Douce A.P. Thermodynamics of the Earth and Planets

Подождите немного. Документ загружается.

438 Thermodynamics of planetary volatiles

0.5 1 1.5

2 2.5

0.5

1.5

1.1

1.2

1.5

0.9

0.85

0.75

liquid + vapor

supercritical fluid

1.05

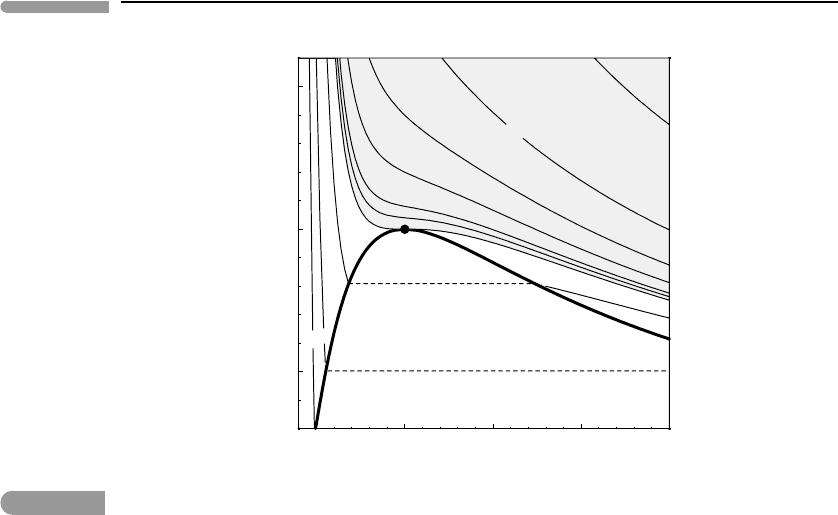
Fig. 9.7

Non-dimensional pressure–volume diagram for a van der Waals ﬂuid. The contours are isotherms (τ given on the

right edge of the diagram). The critical isotherm is tangent to the vapor–liquid loop at the critical point. In a

supercritical ﬂuid there is no discontinuous phase transition separating “low density ﬂuids” (=gases) from “high

density ﬂuids” (=liquids). A discontinuous phase transition between the two phases always exists at temperatures

below the critical temperature.

Close-ups of the neighborhood of the critical point are shown in Figs. 9.7–9.9. The first of

these figures shows the pressure–volume projection, with temperature depicted by isotherms

(the numbers on the right-hand side are the values of τ , the two missing values are 1.01

and 1.02). Figure 9.8shows the temperature–volume projection, with pressure depicted by

isobars (showing values of π ), and Fig. 9.9shows the pressure–temperature projection with

contoured isochores (showing values of φ). The fat dot in all figures is the critical point. The

univariant phase boundary in Fig. 9.9maps the location of the liquid–vapor discontinuous

phase transition, i.e. it tracks the P –T path of the liquid–vapor loop that appears in the

other two projections. The density jump across the first-order phase transition decreases

towards the critical point, and disappears at τ = π = 1. Therefore, the univariant phase

boundary necessarily terminates at the critical point, which is a singular point on the curve,

not an invariant point (see Box 7.1). No discontinuous phase transition is possible beyond

the termination of the univariant phase boundary, but note that the critical isochore (φ =1)

is the continuation of the univariant phase boundary (Exercise 9.6).

The distinction between condensed and non-condensed fluids, expressed, for example,

by their different densities and compressibilities, is discontinuous below the critical point.

The density of the liquid varies relatively little along the vapor–liquid coexistence curve,

but the density of the vapor decreases rapidly with decreasing pressure. Above, but in the

neighborhood of, the critical point there is a sharp decrease in the compressibility as density

increases, but no discontinuity between condensed and non-condensed phases. Where one

chooses to place the boundary between condensed and non-condensed fluid is conventional

(or unimportant): in the supercritical region, which is shaded in Fig. 9.7and 9.8, there is

no discontinuous phase transition between the two states. Supercritical fluid means that the

439 9.2 Liquid–vapor equilibrium

0.5 1 1.5

2.5 3

0.7

0.8

0.9

1.1

1.2

1.3

1.1

1.2

1.5

0.8

0.6

liquid + vapor

supercritical fluid

1.05

0.4

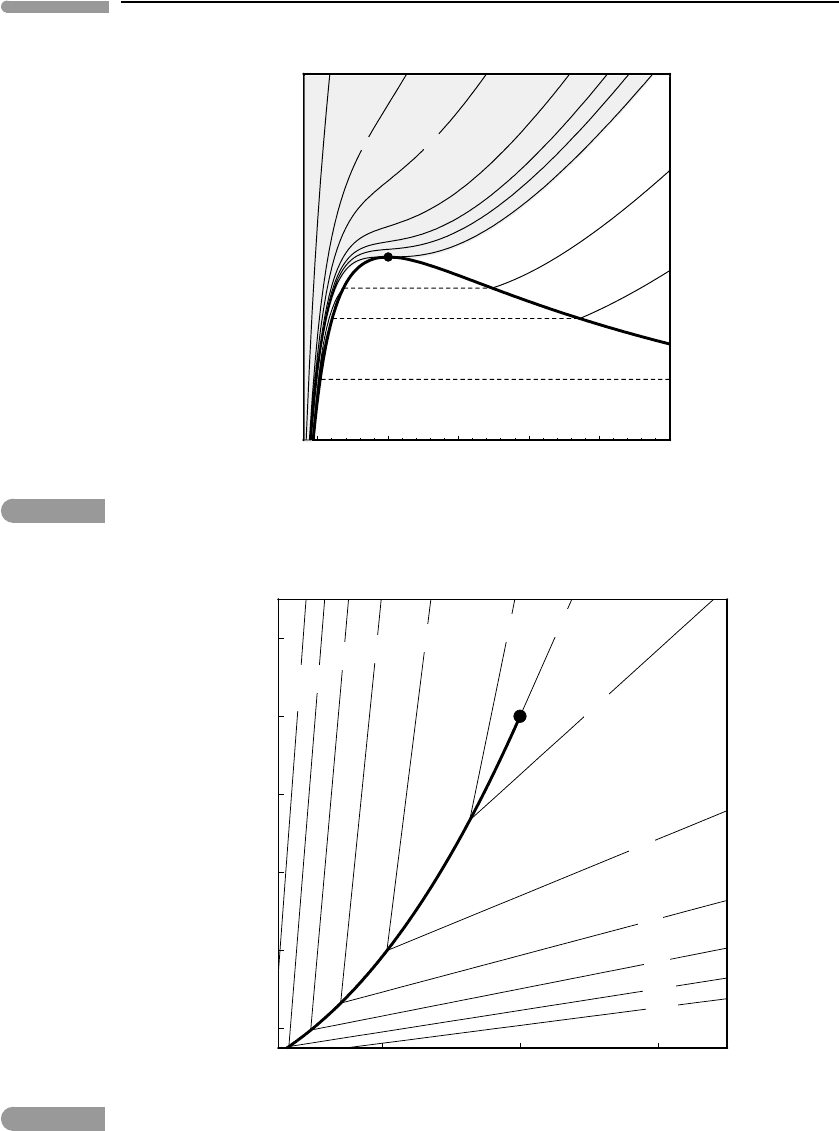
Fig. 9.8

Non-dimensional temperature–volume diagram for a van der Waals ﬂuid. The contours are isobars (π given along

the top and right edges). The critical isobar is tangent to the vapor–liquid loop at the critical point. Liquid–vapor

unmixing at a discontinuous phase transition is possible only if the pressure is less than the critical pressure.

0.8 1 1.2

0.2

0.4

0.6

0.8

1.2

0.65

0.52

0.45

0.48

0.47

0.44

Fig. 9.9

Non-dimensional pressure–temperature diagram for a van der Waals ﬂuid. The contours are isochores. The univariant

phase boundary, i.e. the discontinuous phase transition mapped by the liquid–vapor loop in Figs. 9.7 and 9.8, ends at

the critical point, which is not an invariant point (Box 7.1). The density jump across the discontinuous phase transition

vanishes at the critical point, and the critical isochore is continuous with the univariant phase boundary.

440 Thermodynamics of planetary volatiles

fluid’s properties change continuously from those of a gas to those of a liquid, but carries

no density connotation. For example, given that the critical temperature and pressure for

nitrogen and oxygen are approximately 126 K, 34 bar and 154 K, 50 bar, respectively, air

cannot be made to condense at a discontinuous phase transition by compressing it at room

temperature – it must be cooled below its critical temperature.

9.3 The principle of corresponding states

It is an empirical observation, which was put on solid footing largely by the work of

Guggenheim (for example, 1967, pp. 135–140), that some gases follow the same behavior

if their pressure, temperature and volume are expressed as the non-dimensional reduced

quantities π, τ and φ. This is known as the principle of corresponding states. It suggests that

a universal equation of state may exist, but it does not tell us what form the universal EOS

has, nor, for that matter, whether an analytic function with the properties of a universal EOS

even exists. We can quickly discard the non-dimensional van der Waals EOS, however. If

gases followed this equation as a universal EOS then their critical compressibility factor,

, would be (using (9.31)–(9.33)):

. (9.41)

A compilation of critical parameters for eighteen gases of interest in planetary sciences

(Table 9.1) shows that this is not the case, and that Z

is in every case significantly less than

0.375. The gases in Table 9.1are listed in order of decreasing Z

. There is a group of gases for

which Z

is ∼0.3. These gases are sometimes called simple gases and follow the principle of

corresponding states more or less closely. As we shall see, a simple EOS exists which, if not

completely accurate, is at least acceptable as a first order semi-quantitative approximation

to their behavior.At the other extreme there are substances such as H

O, NH

, HCN and HF

for which Z

is much less than 0.3 and, more importantly, significantly different among the

various gases. This suggests that a universal EOS for these substances does not exist. These

gases do not obey the principle of corresponding states. What distinguishes these substances

from the “simple gases” is that their molecules are strongly polar. In between there are some

gases, most notably CO

and SO

, which depart significantly from the behavior of simple

gases but not as much as, say, H

O. This emphasizes the fact, pointed out by Guggenheim,

that there is no sharp boundary that one can draw between gases that follow the principle

of corresponding states and those that don’t.

One important consequence of the principle of corresponding states is that, if a sufficiently

simple universal EOS can be found, then the adjustable parameters of the EOS can be

calculated from the critical parameters of the gas. To exemplify this, let us assume for the

sake of argument that the van der Waals equation is the universal EOS. We could then pick

any two of the equations (9.31)–(9.33) and solve for a and b in terms of any two of the

critical parameters. If the gas followed the van der Waals EOS exactly then we would get

the same values for a and b regardless of whether we chose to solve in terms of {T

} or {P

}, as the three critical parameters would be related by (9.41). For real

gases this is not the case, however, as Z

=0.375. Because the pressure and temperature of

the critical point are easier to measure accurately than the critical volume, it is customary

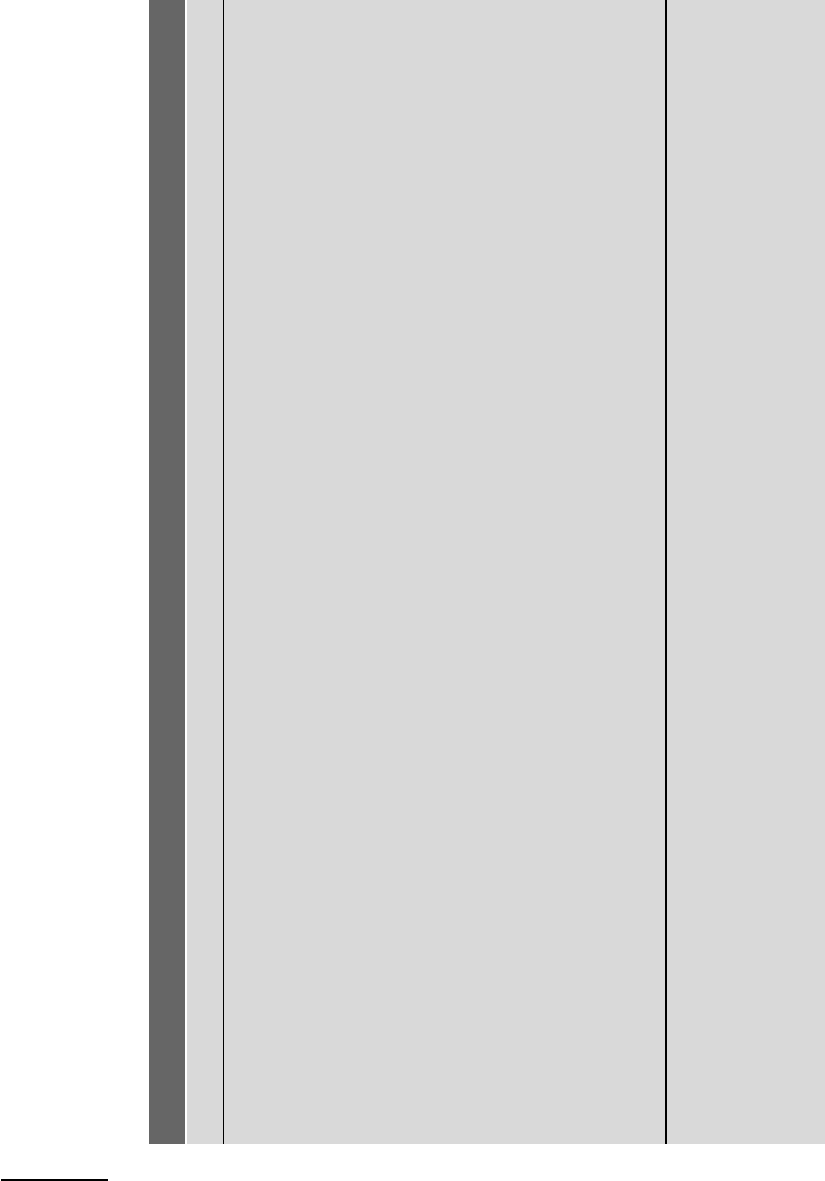
Table 9.1 Critical properties and Redlich–Kwong parameters of some planetary ﬂuids

Species T

k P

bar V

mol

−1

kg m

−3

a (R–K) b (R–K) ρ

Moho

kg m

−3

Moho

/ρ

Ne 44.4 27.6 42.00 519.0 1.4 ×10

0.3140 1.51 ×10

12.0

33.0 12.9 65.00 30.8 9.3 ×10

0.3066 1.81 ×10

15.3

He 5.2 2.3 57.00 70.2 1.1 ×10

0.2998 1.05 ×10

11.1

CO 132.9 35.0 93.10 300.8 6.5 ×10

0.2948 1.72 ×10

27.4 809.1 2.7

Ar 150.9 49.0 75.00 532.6 8.0 ×10

0.2929 1.69 ×10

22.2

126.2 34.0 89.20 313.9 6.7 ×10

0.2890 1.56 ×10

26.7

154.6 50.5 73.40 436.0 8.2 ×10

0.2884 1.74 ×10

22.1

190.5 46.0 98.40 162.6 6.1 ×10

0.2856 3.22 ×10

29.9 432.4 2.7

144.1 51.7 66.00 575.8 9.1 ×10

0.2849 1.43 ×10

20.1

S 373.2 89.4 98.50 345.2 6.1 ×10

0.2838 8.89 ×10

30.1 925.1 2.7

416.9 79.9 123.00 576.4 4.9 ×10

0.2836 1.31 ×10

37.6

304.2 73.8 94.43 466.0 6.4 ×10

0.2756 6.46 ×10

29.7 1257.7 2.7

430.8 78.8 122.00 524.6 4.9 ×10

0.2684 1.33 ×10

37.4 1390.0 2.6

HCl 324.6 83.1 81.00 450.6 7.4 ×10

0.2494 6.75 ×10

28.1 1047.3 2.3

405.6 112.8 72.50 234.5 8.3 ×10

0.2425 8.68 ×10

25.9 525.0 2.2

O 647.1 220.5 56.00 321.4 1.1 ×10

0.2295 8.80 ×10

14.6 944.1 2.9

HCN 456.7 53.9 139.00 194.2 4.3 ×10

0.1973 2.44 ×10

61.0 398.2 2.0

HF 461.0 64.8 69.00 289.9 8.7 ×10

0.1167 7.80 ×10

12.8 345.4 1.2

Critical temperature, pressure and density from Mathews (1972), checked for recent corrections against NIST Chemistry WebBook.

Critical volume and particle number calculated from critical density.

calculated from P

, V

and T

(equation (9.41)).

Redlich–Kwong a and b parameters calculated from T

and P

(equation (9.50)), except values in italics taken from Holloway (1987, Table 1). Units are:

bar cm

1/2

for a,cm

for b.

Estimated density at the Moho, ρ

Moho

, calculated with R–K EOS at 950 K, 10.7 kbar.

441

442 Thermodynamics of planetary volatiles

to solve for a and b in terms of these variables and then we have, from (9.32) and (9.33):

vdW

, b

vdW

. (9.42)

Equations (9.42) are of interest primarily for historical and didactic reasons, as the van

de Waals EOS, even if qualitatively correct, does not provide an accurate quantitative

representation of the behavior of any real gas. For any EOS with two adjustable parameters,

however, the same methodology can be appliedin order to derive the values of the parameters

from measured values of T

and P

9.4 Equations of state for real ﬂuids at P–T conditions typical of the

crusts and upper mantles of the terrestrial planets

There are two basic approaches to constructing EOS applicable over a wide range of tem-

peratures and pressures. The first one, exemplified by the van der Waals equation, is to

start from the ideal gas EOS and add adjustable parameters that account for repulsion and

attraction between molecules, and for the ways in which these forces vary with temperature

and density. These are empirical equations that are cubic in volume and are thus called

cubic equations of state. We will discuss two equations of this type, in addition to the

van der Waals EOS: the Redlich–Kwong EOS (Redlich & Kwong, 1949) and a successful

modification to this equation due to Kerrick and Jacobs (1981).

The other approach is philosophically more satisfying because it can be shown to have

physical fundamentation, but it unfortunately results in an equation of state that performs

very poorly and that can only be improved by empirical tweaks not unlike those used in cubic

EOS. As we did for some of the equations of state for solids, we begin with an expression

for the Helmholtz free energy of the fluid. In this case we consider two contributions to F,

one corresponding to the Helmholtz free energy of the ideal gas and a second one, called

the residual free energy, F

res

, that encapsulates all of the energetic effects that arise from

intermolecular interactions:

F = F

ideal

res

. (9.43)

We now write the residual term as a power series in density or, equivalently, in the inverse

of volume:

res

=RT



+···



, (9.44)

where the coefficients a

, called the virial coefficients, are functions of temperature. The

virial coefficients can be shown to represent the energetic effects arising from interactions

between two molecules (a

), three molecules (a

) and so on (see, for example, Mason &

Spurling, 1969). The name derives from the fact that the distribution of molecular energies

in terms of kinetic and potential energy terms is described by the virial theorem that we

discussed in Chapter 2.

Differentiating (9.43) we get:

P =−



∂F

∂V



=−



∂F

ideal

∂V



−



∂F

res

∂V



ideal

−



∂F

res

∂V



(9.45)

443 9.4 Equations of state for real ﬂuids

and from (9.44) and the ideal gas EOS:

P = RT



+···



(9.46)

or, equivalently:

Z = 1 +

+···. (9.47)

Equation (9.46), or (9.47), with a

(T ), is known as the virial equation of state. Despite

its theoretical foundation the equation is unsatisfactory. Many terms are required in order to

represent the behavior of real gases at even moderate pressures, and then at high densities

(small V) the series may diverge, i.e. terms in progressively higher powers of V become

larger rather than smaller. Its one strong point is that it provides an explicit expression for

the Helmholtz free energy (equation (9.44)), which is convenient when calculating other

thermodynamic functions such as fugacity (Section 9.5.1 ). We will discuss an equation of

state (the Pitzer–Sterner EOS) which is constructed following the same idea as the virial

EOS, i.e. beginning from an empirical function for the Helmholtz free energy, but which

is not a virial equation because the function is not a power series in V , and is therefore

not a physical representation of intermolecular potentials. Finally, we will discuss another

empirical EOS (Brodholt–Wood EOS) that combines a cubic EOS with virial-like terms.

9.4.1 Cubic equations: the van der Waals EOS revisited

We begin our discussion with the van der Waals (VDW) EOS, with the sole purpose of

understanding why it fails and what can be done about it. We will compare the predictions

of VDW and three other EOS with the measured density of H

O at pressures of 0.1–10 kbar.

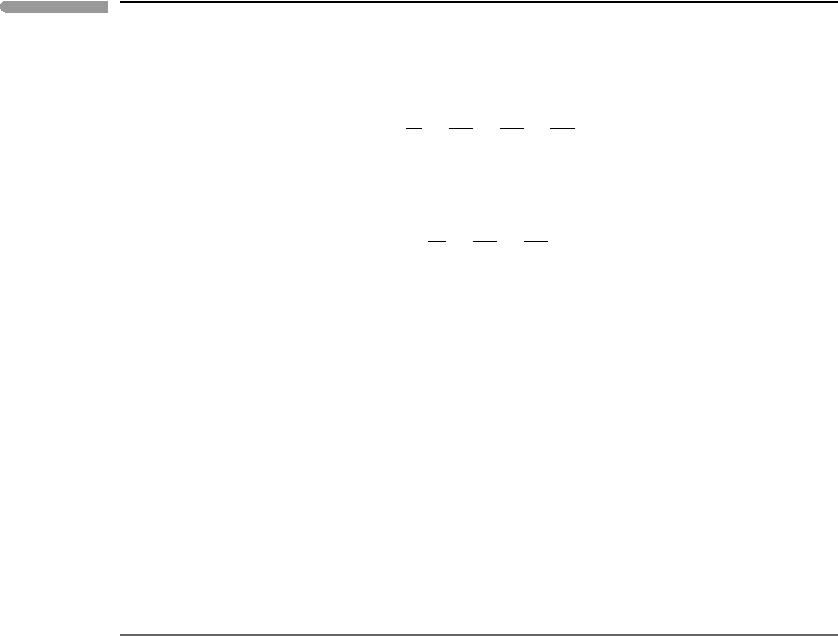
Figure 9.10shows compressibility as a function of reduced density, ρ/ρ

/V . From the

definition of compressibility (equation 9.22) we also get Z =V/V

ideal

. We can thus interpret

the vertical coordinate either as the ratio of the volume of the gas to that of an ideal gas at

the same pressure, or as the ratio of the pressure acting on a given volume of real gas to

the pressure that would be required to take an ideal gas to the same volume. In any case an

ideal gas would plot in this figure as a horizontal line at Z = 1.

Molar volumes of H

O were measured by Burnham et al. (1969) to 8.9 kbar, and extrap-

olated by them to 10 kbar. The symbols in Fig. 9.10represent the density of H

O from

0.1–10 kbar along two isotherms, circles for the critical isotherm (T

= 647.14 K) and

triangles for 700

◦

C, which corresponds closely to 1.5T

. Focusing first on the measured

behavior of H

O we see that at low density it is more compressible than an ideal gas

(Z<1), and that it becomes less compressible than an ideal gas as density increases. This is

the behavior that we should expect from a substance with strongly polar molecules: attrac-

tion predominates at low density, but, as the molecules are squeezed more closely together,

repulsion (or the finite size of molecules) takes over. Attraction becomes less important at

high temperature, as thermal agitation tends to swamp intermolecular potentials, explaining

why the data at 1.5T

plot at higher Z than data at the critical temperature.

At low densities, generally much lower than the critical density, the VDW EOS is mod-

erately successful, but as density increases it fails spectacularly (Fig. 9.10). This behavior

arises because the repulsive term is a constant. As density increases and V approaches b the

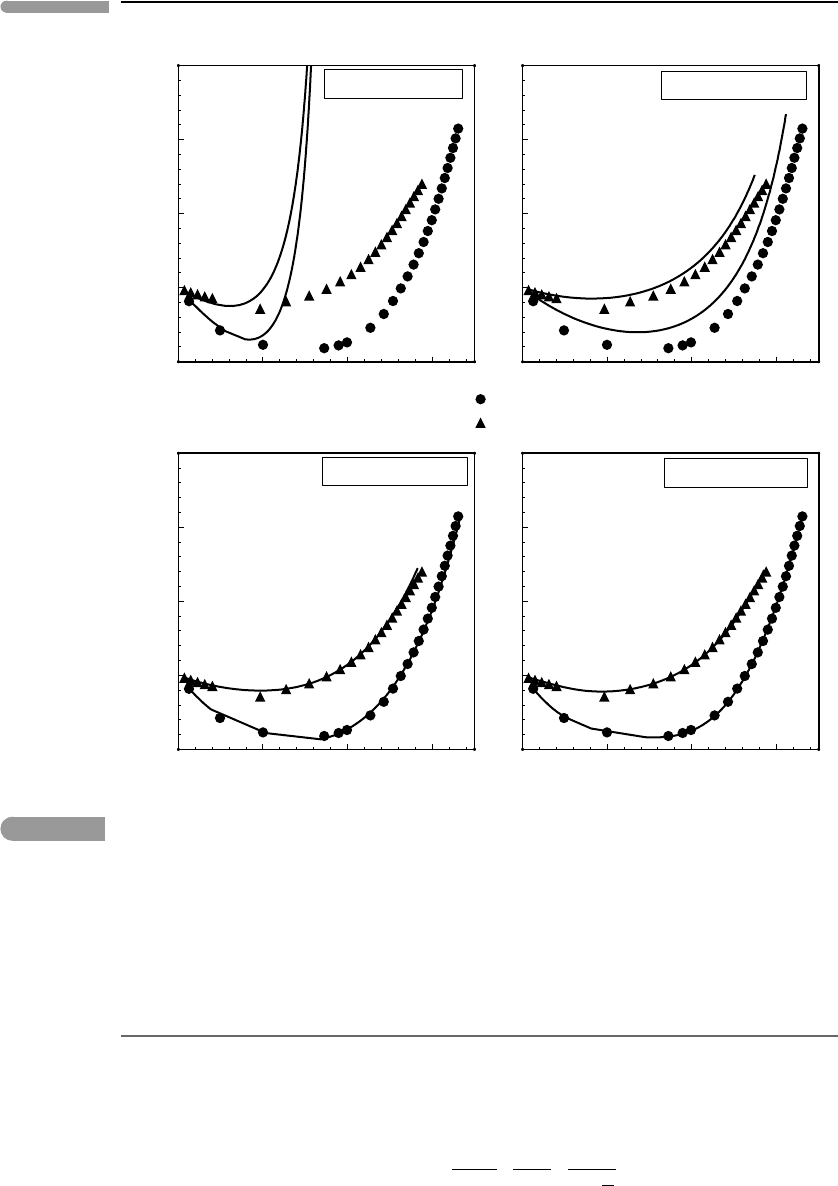
444 Thermodynamics of planetary volatiles

0 1 2 3

O, T = T

O, T = 1.5 T

0 1 2 3

ρ /ρ

1 2 3

van der Waals EOS

Redlich-Kwong EOS

Kerrick-Jacobs EOS

Pitzer-SternerEOS

Fig. 9.10

Comparison of four equations of state for H

O against measured densities at 1 and ∼1.5 T

(experimental data from

Burnham et al., 1969).

pressure required to accomplish further compression diverges (equation (9.27)). An impor-

tant reason for the inadequacy of the VDW EOS is its failure to take into account the fact

that molecules are not rigid.

9.4.2 Cubic equations: the Redlich–Kwong EOS

The first successful modification to the VDW EOS was proposed by Redlich and Kwong

in 1949. In this equation the repulsive term (equation (9.24)) is left unchanged, but the

attractive term (equation (9.25)) is modified as follows:

Z

attraction

RT V

1/2

1 +

. (9.48)

445 9.4 Equations of state for real ﬂuids

The additional factors accomplish two things. The first factor causes the attractive force to

fall off faster with increasing temperature than in the VDW equation. The second factor

makes the attractive force less dependent on density. Redlich and Kwong stated that there

is no theoretical justification for these particular correction terms. Rather, they just happen

to vastly improve the agreement between the EOS and measured densities for many fluids,

especially the simple gases discussed in Section 9.3. As in the VDW equation, a and b

in (9.48) are constants with specific values for each gas. Substituting (9.24) and (9.48)in

(9.23) we get the Redlich–Kwong (RK) EOS:

P =

V −b

−

1/2

(

V +b

)

. (9.49)

Because this equation has only two adjustable parameters it is possible to derive their

values from the critical properties of the gas, by using the condition that the second and

third derivatives of the Helmholtz free energy vanish at the critical point. Applying these

conditions to (9.49) we get:

=0.4274802337

5/2

, b

=0.08664034997

(9.50)

and Z

=1/3. This value for the critical compressibility factor is closer to the value of ∼0.3

measured for the simple gases in Table 9.1. One could then expect that the RK EOS with

parameters calculated from (9.50) may do a reasonable job of representing the properties

of these gases, at least up to moderate pressures. For substances that depart significantly

from corresponding state behavior, however, it is better to obtain the values of the a and b

parameters by fitting the equation to P –V –T measurements spanning as wide a range of

conditions as possible. The parameters for H

O in Table 9.1were obtained in this way (data

from Holloway, 1987).

The RK EOS does not solve the problem of the divergence of pressure at a finite volume

that is a characteristic of the VDW EOS. However, comparing (9.50) with (9.42)wesee

that the value of the b parameter in the RK EOS is about half of that in the VDW EOS. This

means that the useful range of the RK EOS can be expected to extend to higher densities

than the VDW EOS. This is borne out by a comparison of its predictions with the measured

density of H

O up to 10 kbar (Fig. 9.10). The agreement is far from perfect, and generally

inadequate for accurate thermodynamic calculations, but is much better than for the VDW

EOS. The fit improves with increasing temperature. However, at the highest densities shown

in the diagram the tendency for pressure to diverge clearly insinuates itself. This is a problem

with the EOS, not with H

O in particular, which means that its application to simple gases is

also limited to moderate pressures, such that the molar volume remains significantly greater

than b.

9.4.3 Cubic equations: the Kerrick–Jacobs modified Redlich–Kwong EOS

Since Redlich and Kwong’s proposal of equation (9.49) many modifications have been

proposed in order to overcome the limitations of that equation. All of these EOS come under

the general label of modified Redlich–Kwong (MRK) EOS, and they are all empirical fits

of varied complexity. Among the most successful ones for conditions in planetary interiors

446 Thermodynamics of planetary volatiles

is the MRK equation proposed by Kerrick and Jacobs in 1981. This equation leaves the

attractive term in the RK EOS unchanged but: (1) it makes the parameter a a function of

temperature and volume (and hence pressure) and (2) it modifies substantially the repulsive

term. The Kerrick and Jacobs (KJ) EOS is:

P =



1 +y +y

−y



(

1 −y

)

−

(

V ,T

)

1/2

(

V +b

)

(9.51)

where:

y =

(9.52)

and:

a = a

(

)

(

)

(

)

(9.53)

and:

(

)

i,1

i,2

T +c

i,3

. (9.54)

The KJ EOS has ten adjustable parameters, which obviously makes it much easier for it to

reproduce measured volumes over a wide pressure–temperature range. Figure 9.10shows

that, up to ∼10 kbar, it reproduces the measured behavior of H

O almost perfectly, although

at τ = 1.5 there is a hint of the KJ EOS beginning to show divergence in the pressure, as V

approaches b/4.

In contrast to two-parameter equations, it is not possible to estimate the values of the

adjustable parameters of the KJ EOS from the critical properties. The equation can only be

calibrated by fitting it to measured volumes over a pressure–temperature range. Values for

b and the nine c

i,j

parameters are given for H

O and CO

by Kerrick and Jacobs (1981)

and for CH

by Jacobs and Kerrick (1981). Because the KJ EOS is an empirical equation

its performance beyond the range of conditions used to fit the parameters (∼0–10 kbar) is

uncertain, and application to such conditions must be done with caution.

9.4.4 Expansion of the residual Helmholtz free energy: the Pitzer–Sterner EOS

Pitzer and Sterner (1994) proposed an equation of state based on an empirical formulation

for the residual Helmholtz free energy. Their expression is most easily written in terms of

density, ρ = 1/V :

res

ρ +



ρ +a

−



−







−a

−1



−







−a

−1



(9.55)

447 9.4 Equations of state for real ﬂuids

where each of the ten adjustable parameters a

is a function of temperature parametrized

by the following polynomial:

(

)

i,1

−4

i,2

−2

i,3

−1

i,4

i,5

T +c

i,6

. (9.56)

The equation has 60 adjustable parameters, although many of them are found to be zero.

Using (9.45) the Pitzer–Sterner (PS) EOS is:

=ρ +a

−ρ



+2a

ρ +3a

+4a



ρ +a





−a

(9.57)

The equation has no physical justification but is found to reproduce the volumetric proper-

ties of H

O and CO

to very high pressure with a remarkable degree of accuracy. Figure

9.10shows that the agreement with the measured properties of H

O to 10 kbar is essentially

perfect, but the agreement is also excellent to much higher pressures (of order 10

–10

kbar), for which scattered volume measurements obtained by a variety of experimental

methods are available. The form of equation (9.57) keeps the EOS from blowing up at high

densities, as happens with cubic EOS when the molar volume approaches the value of the

excluded volume, b. The equation is calibrated for H

O and CO

only. The coefficients for

the two gases are given by Pitzer and Sterner (1994). Given the large number of adjustable

parameters one would expect that the PS EOS would also work well for other fluid species,

but as of this writing, and to the best of my knowledge, no attempt has been made to per-

form the necessary calibrations. It would be interesting to know whether the PS EOS can

be applied successfully to other important fluid species such as CH

, CO, H

S and NH

9.4.5 The Brodholt–Wood EOS for H

O at high pressure

Brodholt and Wood (1993) performed molecular dynamics simulations of the properties

of H

O to 2500 K and 350 kbar. They then fit the following EOS to the results of their

simulations:

P =

V −b

−

1/2

(

V +b

)

(9.58)

with:

a = a

T +a

−2

(9.59)

and:

b = b

V<0 (9.60)

and c,d, e and f constants (values of all coefficients are given by Brodholt & Wood, 1993).

The Brodholt–Wood (BW) EOS has been described as an MRK equation with virial terms,

but I would argue that this is not correct. In the first place, the b term is always negative,