Douce A.P. Thermodynamics of the Earth and Planets
Подождите немного. Документ загружается.

18 Energy in planetary processes
The negative sign in equation (1.20) insures that K is a positive quantity, as volume always
decreases under an increase in the applied pressure. The symbol ∂ indicates partial differ-
entiation, and is used whenever a variable is a function of more than one other variable (see
Box 1.3). Equation (1.20) defines the dimension of bulk modulus as being the same as that of
pressure. A substance has an infinite quantity of bulk moduli, depending on how one speci-
fies the volume change to take place, but the definition becomes precise if we specify which
variables are to be held constant during the transformation. For example, the bulk modulus
measured at constant temperature, called the isothermal bulk modulus, is defined as:
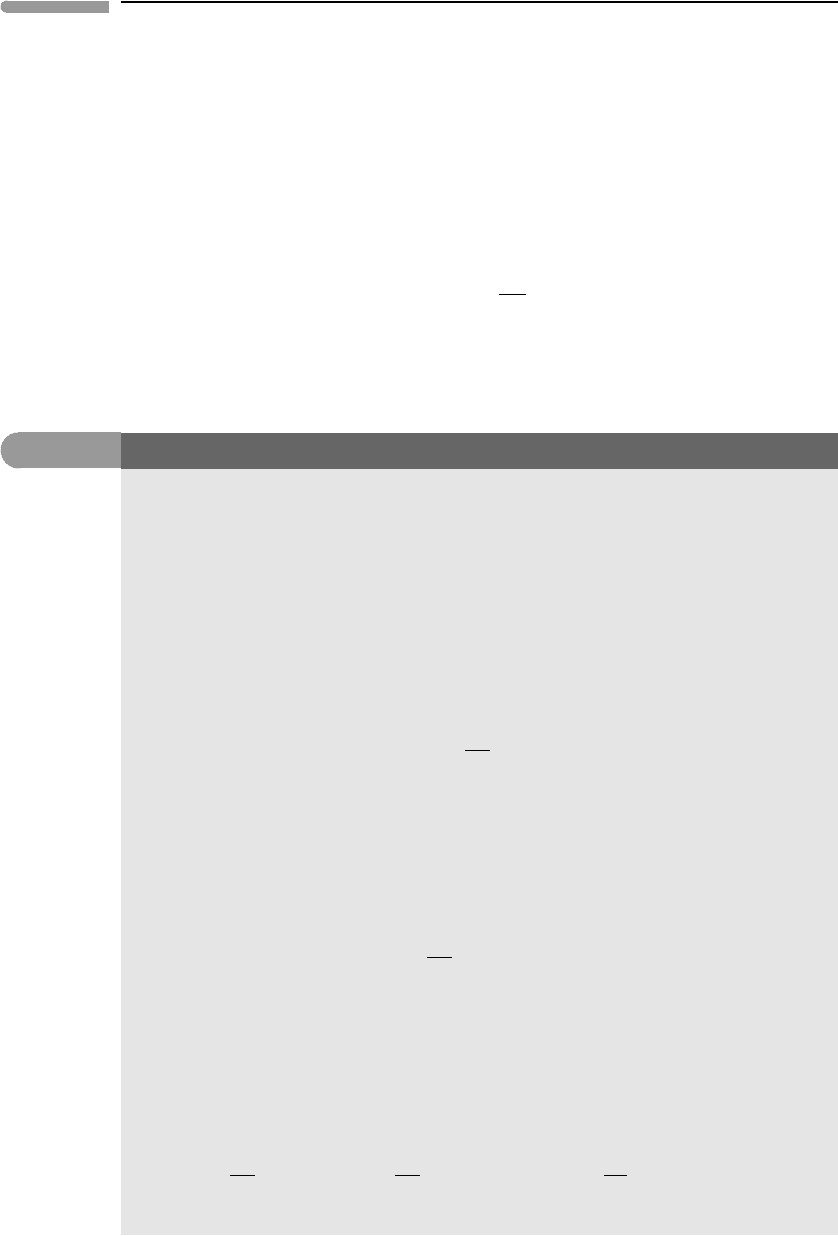
K
T
=−V
∂P
∂V
T
, (1.21)
where the subscript T denotes that temperature is held constant. We shall see that isother-
mal processes are not the only ones that we must consider, so that other bulk moduli will
eventually be introduced.
Box 1.3
Functions of several variables and partial derivatives
Manipulation of functions of several variables, and in particular some basic proficiency in multivariate
calculus, are essential requirements for working in thermodynamics and many other branches of physics and
chemistry. A good source for self-study is the textbook by Sokolnikoff and Redheffer (1966). Here I will cover
only some basic concepts. Consider a function of i variables, y =y( x
1
,x
2
,...,x
i
). The function y has i partial
derivatives, each of which is taken with respect to one of the independent variables, while holding all of the
other independent variables constant. A simple analogy is to think of the two-dimensional surface of the
Earth, and to consider the slope of the terrain along the N–S direction at constant longitude, and then along
the E–W direction at constant latitude. Partial derivatives are denoted with the symbol ∂, and the partial
derivative of y relative to x
n
is written as follows:
∂y
∂x
n
. (1.3.1)
From the operational point of view finding the value of (1.3.1) poses no problems: we just consider all of the
other variables, x
i=n
to be constants, and calculate the derivative following the usual rules. The notation of
(1.3.1) is mathematically complete, but in thermodynamics it is customary to specify which are the variable
or variables that are held constant when performing a calculation. Thus, in thermodynamics (1.3.1) is usually
written as follows:
∂y
∂x
n
x
1
,x
2
,...,x
i=n
, (1.3.2)
where the list of subscripts after the parenthesis specifies the variable or variables that are held constant
during differentiation. Whether or not this notation is strictly necessary is the subject of some contention, to
which I return in Box 1.4.
The total differential of a function of several variables, dy, is the sum of the changes in the value of y
arising from the change in each of the independent variables. It is defined as follows:
dy =
∂y
∂x
1
x
2
,x
3
,...,x
i
dx
1
+
∂y
∂x
2
x
1
,x
3
,...,x
i
dx
2
+···+
∂y
∂x
i
x
1
,x
2
,...,x
i−1
dx
i
. (1.3.3)

19 1.4 Expansion work
Box 1.3
Continued
Recalling the example of the surface of the Earth, we can calculate the total change in elevation between two
points by first moving N–S at constant longitude, and then E–W at constant latitude. Or consider the volume
of a fixed amount of substance, V. This is generally a function of P and T ,so V is a function of two variables,
V = V( P,T ). If we change both pressure and temperature, the total change in volume is given by:
dV =
∂V
∂T
P
dT +
∂V
∂P
T
dP. (1.3.4)
There are a number of identities among partial derivatives that are useful in thermodynamic derivations.
Consider four independent variables, x, y, z and w, such that the following functions exist and are continuous
and differentiable:
w = w(x,z); z = z(x,y); thus, w = w(x,y), (1.3.5)
we can write two different equations for the total differential of w, dw:
d
w =
∂
w
∂x
z
dx +
∂
w
∂z
x
dz (1.3.6)
and:
d
w =
∂
w
∂x
y
dx +
∂
w
∂y
x
dy. (1.3.7)
The total differential of z is given by:
dz =
∂z
∂x
y
dx +
∂z
∂y
x
dy. (1.3.8)
Substituting into the first equation for dw (1.3.6):
d
w =
∂
w
∂x
z
dx +
∂
w
∂z
x
∂z
∂x
y
dx +
∂z
∂y
x
dy
, (1.3.9)
which, collecting terms in dx, dy, becomes:
d
w =
∂
w
∂x
z
+
∂
w
∂z
x
∂z
∂x
y
dx +
∂
w
∂z
x
∂z
∂y
x
dy. (1.3.10)
Now, because x and y are independent variables, the only way in which equations (1.3.7) and (1.3.10) can be
simultaneously true is if the coefficients of dx and dy are equal in each of the equations. In other words:
∂
w
∂y
x
=
∂
w
∂z
x
∂z
∂y
x
(1.3.11)
and:
∂
w
∂x
y
=
∂
w
∂x
z
+
∂
w
∂z
x
∂z
∂x
y
. (1.3.12)
20 Energy in planetary processes
Box 1.3
Continued
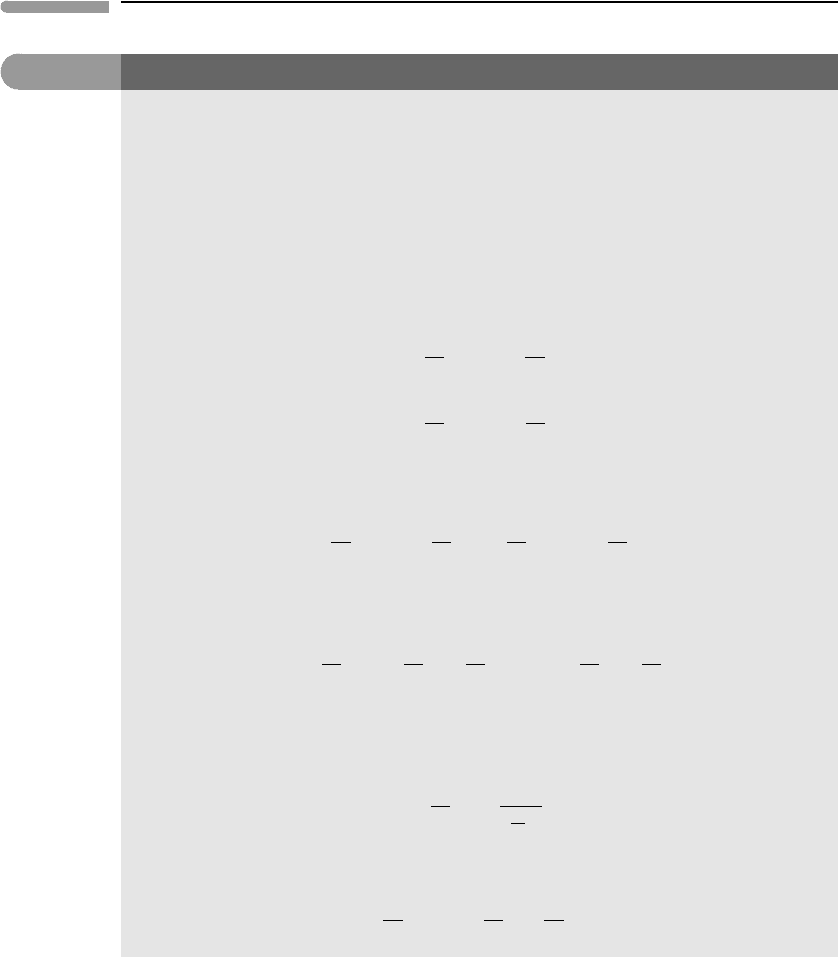
The first of these equations, (1.3.11), is known as the chain rule of partial differentiation and is easy to
remember. The second one has no name, and is harder to memorize – it is best to look it up every time that
one may want to use it. When dealing with equations of state that relate three variables (P, V, T) one is
often faced with the following problem. Here we have three variables, x, y, z, and by virtue of the equation
of state we can write:
x =x(y,z);z =z(x,y), (1.3.13)
we then have the following total differentials:
dx =
∂x
∂y
z
dy +
∂x
∂z
y
dz (1.3.14)
dz =
∂z
∂x
y
dx +
∂z
∂y
x
dy. (1.3.15)
Substituting the second one into the first one:
dx =
∂x
∂y
z
dy +
∂x
∂z
y
∂z
∂y
x
dy +
∂z
∂x
y
dx
(1.3.16)
collecting terms and rearranging:
dx =
∂x
∂y
z
+
∂x
∂z
y
∂z
∂y
x
dy +
∂x
∂z
y
∂z
∂x
y
dx. (1.3.17)
Because x and y are independent variables, the coefficient of dx in the right-hand side of this equation must
be equal to 1, and the coefficient of dy must be 0, so:
∂x
∂z
y
=
1
∂z
∂x
y
(1.3.18)
and:
∂x
∂y
z
=−
∂x
∂z
y
∂z
∂y
x
. (1.3.19)
An equation of state (EOS) is another type of function that relates volume with pressure
and temperature. EOS are of fundamental importance in many branches of planetary sci-
ences, and we will discuss different types of EOS in subsequent chapters. Every substance
has an EOS. This is just another way of saying that a fixed amount of any substance at equi-
librium at a given pressure and temperature has a unique and well defined volume. Some
EOS have physical fundament and at least their general form can be derived from first prin-
ciples. In other cases, EOS are more or less arbitrary best-fit equations to experimentally
measured P–V–T relationships. In some cases equations of state can be mathematically
very complex, or their explicit mathematical form may be unknown, so that experimental

21 1.4 Expansion work
P–V–T data are summarized in tables. There is, however, one EOS that is mathematically
very simple, that can be derived from first principles, and that has important applications in
classical thermodynamics and some areas of planetary sciences. This is the ideal gas EOS:
PV = RT , (1.22)
where V is molar volume, i.e. the volume of one mol of gas, T is absolute temperature, and
R is the universal gas constant (see Appendix 1). The behavior of real gases at low pressure
(≤1 bar) and temperatures well above their condensation temperatures is reasonably well
approximated by this EOS. Up to this point we have been writing volume in bold type, V , but
we have dropped the bold attribute in the ideal gas equation of state, (1.22). This is so because
we need to distinguish between situations in which we are referring to the total volume of a
system (V ) from those in which we are referring to a specific amount of matter, for instance,
1 mol (V ). For variables whose value depends on the amount of substance considered we
will use non-bold uppercase symbols to denote the value normalized to one mol (e.g. V for
molar volume) and bold uppercase symbols to denote the value of the variable for the entire
system (e.g. V for total volume). V is a material property, independent of system size, but
V is not. The scalar variables pressure and temperature, that do not depend on the size of
a system, will also be written with non-bold symbols, whereas other scalar quantities such
as various types of mechanical energy (e.g. U
g
and U
k
, equations (1.6) and (1.12)) that
depend on system size are written in bold type.
In principle, it is always possible to derive the isothermal bulk modulus from the EOS.
This is particularly simple for the ideal gas EOS, as shown in the following Worked Example,
which compares the magnitude of expansion work associated with pressurechanges in gases,
liquids and solids.
Worked Example 1.3 Expansion work of gases, liquids and solids
What is the isothermal bulk modulus of an ideal gas? From the ideal gas EOS:
P =
RT
V
(1.23)
so:
∂P
∂V
T
=−
RT
V
2
. (1.24)
Inserting this expression into equation (1.21) and using the ideal gas EOS to simplify the
result yields K
T
=P . In contrast to gases, liquids and solids have bulk moduli that are nearly
constant over restricted pressure ranges. For example, for pressures of the order of 1 bar,
K
T
for liquid water is approximately 10
4
bar and K
T
for quartz ≈ 4 × 10
5
bar. Compared
to K
T
for an ideal gas at 1 bar, these values mean that liquids and solids are several orders
of magnitude less compressible than gases, and quartz is somewhat less compressible than
water (greater pressure changes are needed to cause a given volume change). The energy
consequences of these differences are important. We seek to compare the expansion work
performed by an ideal gas, a liquid (water) and a solid (quartz) undergoing the same pressure
change.

22 Energy in planetary processes
From the ideal gas EOS:
dV =−
RT
P
2
dP (1.25)
substituting in equation (1.19):
dW =PdV =−RT
dP
P
(1.26)
and integrating:
W = RT ln
P
0
P
, (1.27)
where P
0
is the initial pressure, and P the final pressure. For an expansion process, P
0
>P
and hence W > 0, in agreement with our sign convention for expansion work (a positive
value of W means that the system is performing work on its environment, and hence that
the system’s energy content decreases). Equation (1.27) yields the amount of work for
expansion of 1 mol of ideal gas. For any other amount of gas, it must be multiplied by the
number of mols.
Calculating the expansion work of an ideal gas is straightforward because of the simplicity
of the ideal gas EOS. The general expression for expansion work as a function of bulk
modulus, or, equivalently, for substances with an EOS more complicated than that for ideal
gases, is not that simple (Chapters 8 and 9). If the bulk modulus is large enough and we
are only interested in a rough approximation then the following simplifying assumption
becomes possible. We integrate equation (1.21) assuming constant bulk modulus:
V
V
0
=exp
P
0
−P
K
T
. (1.28)
This equation shows that, for a pressure drop of, say, two orders of magnitude, the volume
of water increases by a factor of ∼ 1.01, and the volume of quartz by a factor of ∼ 1.000 25.
For the same pressure change, an ideal gas increases in volume by a factor of 100. As a first
approximation, we may neglect the volume change of liquids and solids when integrating
equation (1.19), but the attentive reader will immediately notice a seeming contradiction
here: if volume is constant, then there should be no expansion work. The answer to this
riddle is that volume is not constant, but assuming that it is constant leads to a much simpler
mathematical expression that, under certain circumstances, yields a numerical answer that is
an acceptable approximation to the exact answer.The limits of applicability of the simplified
treatment that follows is explored in Exercise 1.9. We re-write equation (1.21) as follows:
dV =−
V
K
dP (1.29)
and substitute into equation (1.19):
dW =PdV =−
V
K
P dP. (1.30)

23 1.5 Isothermal and adiabatic processes
Integrating this expression under the assumption of constant volume yields:
W ≈
V
2K
P
0
2
−P
2
, (1.31)
where expansion implies P
0
> P, so that W > 0.
Consider expansion of 1 mol of each substance from P
0
=10 bar to P =1 bar,at a constant
temperature of 25
◦
C = 298 K. For water and quartz, we need their molar volumes, which
are 1.8 J bar
−1
and 2.27 J bar
−1
, respectively. The values of expansion work are W
water
≈
8.9 × 10
−3
J mol
−1
and W
quartz
≈ 2.8 × 10
−4
J mol
−1
. For an ideal gas, equation 1.27
yields W ≈ 5.7 × 10
3
J mol
−1
. The difference between the amount of work performed
by an expanding gas and the work performed by an equivalent amount of expanding liquid
or solid is huge – six or seven orders of magnitude in the above example. This is a simple
mathematical statement of the greater destructive power of a pyroclastic eruption compared
to a lava flow. Condensed phases (solids and liquids) and non-condensed phases (gases)
differ in many aspects of their physico-chemical behaviors (Section 1.15). At the root of
many of these differences is the wide gap that exists between their respective abilities to
store or dispense energy in response to changes in pressure, which is expressed numerically
in their vastly different compressibilities.
1.5 Isothermal and adiabatic processes. Dissipative vs.
non-dissipative transformations redux
An isothermal volume change is a dissipative transformation. To see why, consider what
must happen in order for the temperature of a gas to remain constant as it is compressed. If
heat were not allowed to escape the system, then the temperature of the gas would increase
during compression. In order for the temperature to remain constant heat must be allowed
to leave the system, and energy dissipation takes place.
Let us repeat our thought experiment more carefully. First, we imagine a system that is
perfectly insulated to heat transfer, such that all of the mechanical energy that we transfer
into the system by compressing it is stored in the system, where it goes to increase its
internal energy. Internal energy is a thermodynamic variable of a kind called state function,
which we will define more precisely later in this chapter. I will symbolize it by E and,
as a first approximation, we may think of temperature as a measure of the internal energy
content of a system. The increase in temperature that we observe in the first part of our
thought experiment measures the increase in its internal energy, which, if the system’s
thermal insulation is perfect and the process is quasi-static, must exactly match the amount
of work that was performed on the system. As long as no heat leaves the system, no energy
dissipation takes place if the compression is quasi-static. Energy dissipation occurs only
when heat is exchanged between the system and its environment. A system that is perfectly
impervious to heat transfer is called an adiabatic system and a process that takes place
without exchange of heat is an adiabatic process. Although a perfectly adiabatic process is
an abstraction, many natural processes entail heat exchanges that are small enough that they
can be considered to be adiabatic to a good approximation. Examples include convection
(e.g. in a planet’s core, mantle, oceans and atmosphere) and mechanical wave propagation

24 Energy in planetary processes
(seismic waves and sound). What is common to all of these processes is that the rate of heat
diffusion is negligible compared to the rate at which mechanical energy is exchanged – this
topic will be explored further in Chapter 3.
Returning to our thought experiment, we now repeat it with a system that allows heat
to move freely across its boundaries, and we compress it quasi-statically once again. In
order for the compression to be isothermal, the mechanical energy that we perform on the
system must be allowed to leave the system at the same rate as it is being added to it. This
energy leaves the system as heat, so that during an isothermal and quasi-static compression
all of the mechanical energy is dissipated as heat. As we shall see, this last statement is
in fact true only for ideal gases, for which internal energy is not a function of volume.
Internal energy varies with volume in real substances, but a slightly modified version of
that statement is true in general: isothermal processes are not just dissipative processes,
they are the processes that result in the maximum possible heat dissipation.
1.6 Elastic energy
The work associated with an adiabatic volume change is calculated by using the adiabatic
bulk modulus, K
S
, to integrate equation (1.19), where K
S
is defined as:
K
S
=−V
∂P
∂V
S
. (1.32)
The subscript S denotes a special type of adiabatic transformation during which a thermo-
dynamic variable known as entropy remains constant. The meaning of this, and the need to
distinguish between different types of adiabatic processes, will become clear in Chapter 4.
The adiabatic bulk modulus is one of a set of parameters that describe the elastic behavior
of materials.Asubstance is said to deform elastically if: (i) the magnitude of the deformation,
or strain, is a linear function of the applied stress (force per unit area) and (ii) the deformation
is reversible, in the sense that the body recovers its initial shape and size once the stress
is removed. A perfectly elastic deformation is also reversible in the sense of being non-
dissipative: when stress is released the substance returns the same amount of mechanical
energy that was used to cause the deformation. If energy is dissipated then by the law of
energy conservation the amount of mechanical energy returned during the process of elastic
rebound will be less than the amount of mechanical energy used to cause the deformation.
The elastic behavior of a material in general is described by a parameter called the elastic
modulus, λ, defined as:
λ =
stress
strain
. (1.33)
Stress is force per unit area and strain is the ratio of the change in the volume, length or
shape of a body (caused by stress), to the value of the corresponding variable in the original,
pre-strained state. Stress has dimension of pressure, and in fact pressure is one particular
type of stress (also called uniform or isotropic stress). Because strain is defined in such a
way as to be dimensionless, the dimension of λ is also that of pressure. Materials can have
three distinct elastic moduli, that measure different properties of a substance.All substances
(solids, liquids and gases) have an adiabatic bulk modulus, K
S
, that relates adiabatic pressure
changes to the corresponding relative changes in volume. The ratio dV/V is the volumetric
strain, and dP is the stress responsible for this strain, so that the definition of adiabatic

25 1.6 Elastic energy
bulk modulus given by equations (1.32) is consistent with the general definition of elastic
modulus given in equation (1.33).
Fluids can undergo elastic volume changes, but they cannot stretch nor shear elastically
over time- and length-scales characteristic of planetary processes. The adiabatic bulk modu-
lus is thus the only elastic modulus that can be defined for fluids. Solids, in contrast, are also
able to deform elastically by stretching and by shearing, in addition to undergoing elastic
volume changes. This fundamental difference between solids and fluids can be understood
on the basis of their microscopic structures. The atoms in a solid are arranged in a crystalline
lattice and are held in well-defined positions in the lattice by a balance of electrostatic forces
acting among neighboring atoms – these forces are what we call chemical bonds. When a
solid is stretched or sheared, the bonds are distorted but, up to a point, they can hold and
will bring back the atoms to their equilibrium positions if the stress is removed. Elastic
strain is the macroscopic expression of the microscopic behavior of the atomic bonds in
a crystal. For solids, then, one can define two other elastic moduli in addition to the bulk
modulus. Young’s modulus, E, measures relative elongation as a function of applied tensile
stress (i.e. stretching). Shear modulus, µ, relates shear stress to shear strain (defined as a
change of shape at constant volume). Fluids, in contrast, do not have crystalline structure
and interatomic attractive forces are weaker than in a crystal (and altogether nonexistent
in an ideal gas). When a fluid is sheared its atoms change position without preserving any
memory of where they were before the stress was applied, so that there is no restorative
force that would cause elastic rebound. The macroscopic expression of this behavior is that
fluids flow under shear, rather than deforming elastically. There are geological environ-
ments, however, in which the distinction between solids and fluids becomes blurred. For
example, mantle convection and glacier flow are two situations in which crystalline solids
flow under shear stress, rather than undergoing elastic strain.
Exact calculation of the energy stored during a generalized elastic deformation is math-
ematically complex and computationally intensive (see, for example, the classic text by
Malvern, 1969), but an order of magnitude estimate is straightforward and useful in many
planetary sciences applications. Consider an elastic body of characteristic linear dimension
x. Application of a force F causes this dimension to change by an amount δx x. The
characteristic stress on the body is given by σ ≈ F/x
2
, and the strain by ε ≈ δx/x, so that:
λ =
σ
ε
≈
F
xδx
. (1.34)
For a perfectly elastic material, i.e. one that can undergo non-dissipative deformation, the
stored elastic energy, U
c
, must equal the work performed in accomplishing the deformation.
Thus:
dU
c
=Fd
(
δx
)
=λxδxd
(
δx
)
. (1.35)
Because δx x we consider x to be constant and integrate with respect to δx, as follows:
U
c
=λx
δx d
(
δx
)
=
1
2
λx
(
δx
)
2
=
1
2
λx
3
ε
2
. (1.36)
The elastic energy stored per unit volume, U
c
, is then given by:
U
c
≈
1
2
λε
2
. (1.37)
For stretching of a thin elastic body, such as a wire or spring, this formula is exact, with
λ = E (Young’s modulus). In the case of shear strain of elastic materials the formula yields
only an order of magnitude approximation with λ = µ, the shear modulus.

26 Energy in planetary processes
Planetary lithospheres store vast amounts of elastic energy as they bend and buckle under
shear stress. A fraction of this energy is released as seismic waves during earthquakes, ulti-
mately to be dissipated as heat: seismic waves are adiabatic as a first approximation, but,
as all natural processes, they are not perfectly adiabatic and energy dissipation takes place.
Changes in ground elevation accompanying an earthquake represent the transformation of
another fraction of the stored elastic energy to gravitational potential energy. The source
of this energy is not the lithosphere itself, however, but the planet’s internal heat. Plane-
tary lithospheres act as energy storage and transfer mechanisms, much as enormous clock
springs. Elastic energy is also stored when a planetary body is deformed by tidal forces,
and its dissipation is in this case a potentially important source of planetary internal energy,
which we will discuss in Chapter 2.
1.7 Two complementary descriptions of nature: macroscopic
and microscopic
The definition of mechanical work, equation (1.1), makes no requirements as to the nature
of the force that is responsible for transfer of mechanical energy. In the present-day universe
there are four distinct forces: gravitational forces, electromagnetic forces, and strong and
weak nuclear forces. By “distinct” we mean that each of these forces arises from a different
property of matter, and each of them is described by its own physical law. The first two are
the most familiar ones. Gravity arises from an object’s mass and is described in classical
physics by equation (1.2); electromagnetic forces arise from an object’s electric charge and
also follow an inverse square law. There are good reasons to believe that, at very high energy
levels (think of this as extremely high temperatures, such as those that prevailed in the very
early Universe), the four forces may become indistinguishable, but this is something that
need not concern us as planetary scientists.
So far I have made explicit mention of the importance of gravitational forces in planetary
processes (e.g. Section 1.3), and I have hinted at the relevance of electrostatic forces as the
explanation for the elastic behavior of solids. There is a fundamental difference between
these two examples, and we must now place this discussion on firmer ground. We begin by
stating explicitly what is the difference between the ways in which we addressed gravita-
tional potential energy on the one hand, and elastic energy in a solid on the other. In the first
case, we used the actual gravitational force to define and calculate potential energy. In the
other case, we defined macroscopic properties of the material (the elastic moduli) and then
explained these properties in terms of electrostatic forces acting at the atomic level. The
description of elasticity in terms of atoms, crystalline structure and electrostatic forces may
be helpful, but is not required in order to have a full quantitative description of elastic strain.
The elastic modulus is an example of a macroscopic property. Macroscopic properties
provide a phenomenological description of the behavior of a system. By this we mean that we
measure and understand the behavior of the system from macroscopic observations (the phe-
nomenathatwecanobserve), withoutregardtotheultimate physicalmechanismthat explains
theobservedbehavior.Inthisexample,thephysicalmechanismcanonlybe understoodfroma
microscopicdescriptionofthesystemattheatomicormolecularlevel.Manynaturalprocesses
besideselasticitycanbeunderstoodfromthesetwodistinctandcomplementarypointsofview,
the macroscopic or phenomenological and the microscopic or atomic. Both descriptions are
correctand useful,andcombiningbothviews usuallyallowsustogain adeeperunderstanding

27 1.8 Energy associated with electric and magnetic fields
of a process. The two descriptions must, of course, be mutually consistent. Thermodynamics
is the phenomenological description of energy conversion processes and of many of its con-
sequences, including chemical equilibrium. The complementary microscopic description is
providedbystatisticalmechanics.Althoughwewill notencounterformal statisticalmechanic
derivations in this book, I will introduce some of the key concepts of statistical mechanics
and use them to understand the physical foundation of some thermodynamic concepts and
relationships that may otherwise appear obscure or arbitrary.
In contrast to our discussion of elasticity, where complementary macroscopic and
microscopic descriptions are possible, our description of gravitational processes is purely
phenomenological and we do not seek a microscopic description of, for example, grav-
itational potential energy. Why this difference? The answer rests partly on the fact that
gravitational and electromagnetic forces have vastly different magnitudes. Physicists
describe this difference by stating that gravity is exceedingly weak (by about 40 orders
of magnitude) compared to electrostatic force. This is not to say that physicists are not
interested in a microscopic description of gravity – they are, but this is still one of the
major unresolved problems of physics. As planetary scientists, we may recast the distinction
between gravitational and electrostatic forces by saying that there is commonly little overlap
between the respective scales of length and mass over which each of these two forces is the
dominant one. Gravity is negligible compared to electrostatic forces fordistances and masses
such as those characteristic of atomic and molecular structure (e.g. the structure of a crystal
or the behavior of a fluid at the molecular level), in other words, what we call the micro-
scopic description of nature. Conversely, gravity is the dominant force when we consider
masses and length scales such as those that are typical of our macroscopic view of nature.
This is certainly true of masses of the order of planetary bodies, but also of much smaller
systems, down to, for example, grains of sand in a clastic sediment – for what is the force that
drives sedimentation? There are macroscopic planetary environments in which electrostatic
forces are important, however. A simple example is that of flocculation of clay particles.
At length scales much smaller than those of individual atoms, such as those character-
istic of the atomic nucleus, both gravitational and electrostatic forces become negligible
compared to the strong nuclear force. Otherwise, how could a nucleus made up of a large
number of equally charged protons be stable? The strong nuclear force, which is responsible
for binding protons and neutrons in atomic nuclei, is involved in the liberation of energy by
nuclear processes. One example of this is the conversion of mass to electromagnetic energy
by nuclear fusion in stellar cores. Another example is spontaneous radioactive decay, in
which mass is converted to either kinetic energy of subatomic particles (during alpha and
beta decays) or electromagnetic energy (gamma radiation). Radioactive decay is of critical
importance in the energy budget of many planetary bodies.
1.8 Energy associated with electric and magnetic fields
1.8.1 Electrostatic forces
The magnitude of the electrostatic force, |
¯
f
e
|, between two electric charges, q
1
and q
2
,
separated by a distance x in vacuum is given by Coulomb’s law:
|
¯
f
e
|=
1
4π/
0
|q
1
||q
2
|
x
2
, (1.38)
