Douce A.P. Thermodynamics of the Earth and Planets

Подождите немного. Документ загружается.

198 The Second Law of Thermodynamics

Box 4.1

Continued

state i. Obviously,



=N. We subdivide the set of N objects into i subsets of n

objects. Two microstates

are said to be equivalent if n

is the same for all i. If two microstates fulﬁll this requirement but, additionally,

each of the individual objects in each subset n

are the same except that they are arranged in a different

order, then they are the same microstate, rather than equivalent microstates. By the same argument that we

used for N, each subset of n

objects can be arranged in n

! different ways. This is true for all n

, so the product



!) is the total number of repeated (i.e. identical) microstates. The product of the number of equivalent

microstates, which we symbolize with O, times the number of repeated microstates,



!) must be equal

to the total number of possible arrangements of the N objects, N!, as there is no other possibility that we

have not considered. The number of equivalent microstates is therefore given by:

O =



, (4.1.1)

which in the case of i = 2 (that we will use frequently), simpliﬁes to:

O =

(

N −n

)

, (4.1.2)

where n objects are in state 1, and (N −n) objects in state 2.

Consider again the example of 5 coins. The number of microstates deﬁned by 3 heads and 2 tails is 5!/(3!

2!) = 10. All of these microstates are equivalent and correspond to the same macrostate. In contrast, the

macrostate corresponding to 4 heads and 1 tail has 5!/(4! 1!) = 5 microstates.

The numbers of objects (molecules, atoms, ions, crystalline sites, etc.) in systems of physical interest

are much larger than this, typically of order 10

. The factorial of 10

is a very large number, beyond

the computational range of everyday calculators and computers. Luckily, however, what we are interested

in is the logarithm of O, rather than in O itself, and this we can easily calculate by means of Stirling’s

approximation, which states that, for N very large:

lnN!≈N lnN −N. (4.1.3)

Where does this come from? First, we can write ln N! as a sum of logarithms:

lnN!=

i=N



i=1

lni. (4.1.4)

Although the factorial function is deﬁned only for integers, which are discrete variables, when N becomes

very large we can approximate the right-hand side of (4.1.4) as an integral, i.e.

i=N



i=1

lni ≈



lnidi = N lnN −N +1 ≈ N lnN −N, (4.1.5)

which is (4.1.3).

A given macrostate can arise from more than one microstate. We shall say that these

microstates are equivalent. We will also postulate that: (i) All individual microstates are

equally probable and (ii) the observed macrostate, which we will call the equilibrium

199 4.6 A microscopic view of entropy

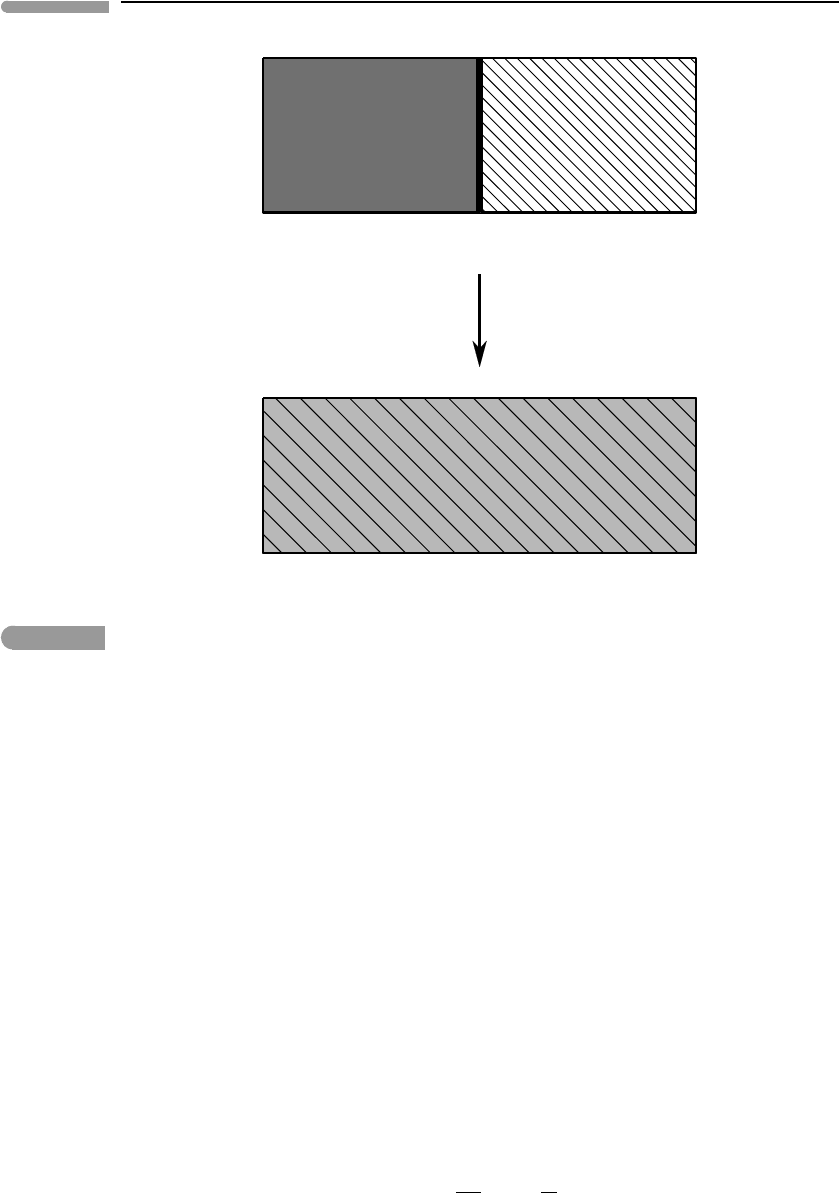
Fig. 4.7

Mixing of two different ideal gases in an isolated container. Each of the gases can be thought of as undergoing a free

expansion from its initial volume to the same ﬁnal volume, V

macrostate, is the one that corresponds to the greatest number of equivalent microstates.

An example will clarify the meaning and implications of these statements and show their

plausibility, although it must be borne in mind that their acceptance as a valid description

of nature relies on the much larger theoretical framework of statistical mechanics, and on

an enormous amount of observations.

Let us consider the process of spontaneous mixing of two different ideal gases at uniform

and constant temperature and pressure. Suppose that we have two containers separated

by a wall, with volumes V

and V

, as shown in Fig. 4.7. Container A is filled with a

moles of ideal gas A, and container B is filled with b moles of ideal gas B. When the

partition is removed the gases mix by diffusion, so that after some time the entire volume,

is filled with a homogeneous mixture in which the mol fractions of the two

gases are X

=a/(a +b) =V

and X

=b/(a +b) = V

(use the ideal gas EOS

to prove these identities). Pressure and temperature were the same for the two gases before

mixing and remain unchanged during mixing.

Diffusive homogenization of the two gases in an isolated system is a spontaneous process,

so it must be accompanied by an increase in entropy, which we calculate as follows. From

equation (4.12) we write the entropy change S from an initial state i to a final state f as

follows:

S =



dV . (4.45)

200 The Second Law of Thermodynamics

Using the ideal gas equation of state and the fact that internal energy of an ideal gas is a

function of temperature only (Section 1.14), we re-write equation (4.45) for an ideal gas as

follows:

S =



dT +R







+R ln





. (4.46)

Diffusive homogenization of ideal gases is an isothermal process, because ideal gases are

made up of non-interacting particles (i.e. there are no forces between the particles, and hence

no work is performed when particles of different gases mix). However, the volume occupied

by each of the gases increases during mixing, from the volume of the respective initial

container to the total final volume, V

. The entropy of each gas therefore increase during

mixing by the same amount that it would increase if it was undergoing a free expansion

(compare equation (4.22)). Because entropy is additive (Section 4.2), the total entropy

increase for the mixing process is given by:

S

mixing

=aS

+bS

=aRln





+bR ln





=−aR ln

(

)

−bR ln

(

)

> 0. (4.47)

As mol fractions in a mixture are always less than 1, spontaneous mixing of gases is always

accompanied by an increase in entropy. I never cease to marvel at this result. We defined

entropy on the basis of heat transfer (equation (4.6)), and yet it can correctly predict the

behavior of a system in which there is no heat transfer involved! As you will soon see,

the importance of equation (4.47) goes beyond merely telling us that ideal gases will mix

spontaneously.

Let us look at this same process microscopically. The two vessels initially contain aN

and bN molecules of different ideal gases, where N ≈ 6.02 ×10

is Avogadro’s number.

We will now count microstates, as described in Box 4.1. You can think of the “objects” that

I refer to in Box 4.1 as being little boxes into which the containers are subdivided, such

that there is exactly one box per molecule. Averaging over a sufficiently long time, there is

always exactly one molecule inside each box, and there are no empty boxes. The boxes can

be in two different states: occupied by either a molecule of gas A or a molecule of gas B.

Before the partition is removed all boxes in side A are filled with molecules of A, and all

boxes in side B are filled with molecules of B. According to equation (4.1.2), the number

of microstates in each of the two vessels, O

and O

, is:

(

)

(

)

!0!

(

)

(

)

!0!

=1. (4.48)

The total number of microstates for the system before the gases are allowed to mix, O

is the product of these two numbers, as any microstate in A can be combined with any

microstate in B, i.e.:

=1. (4.49)

When the gases are allowed to mix there are (a +b)N boxes in a single vessel of volume

, of which aN are in one state (occupied by an A molecule) and bN in the other (occupied

by a B molecule). Using equation (4.1.2), the number of microstates is given by O

,as

201 4.6 A microscopic view of entropy

follows:

[

(

a +b

)

]

(

)

(

)

. (4.50)

We now use Stirling’s approximation (equation (4.1.3)):

ln O

(

a +b

)

N ln

[

(

a +b

)

]

−

(

a +b

)

−

[

(

)

(

)

−

(

)

]

−

[

(

)

(

)

−

(

)

]

, (4.51)

which simplifies to:

ln O

=aN ln



a +b



+bN ln



a +b



(4.52)

or:

ln O

=−aN ln

(

)

−bN ln

(

)

. (4.53)

Consider these results in the light of the two postulates about microstates and macrostates.

According to postulate (i) all microstates are equally probable. Equation (4.49) says that

there is only one microstate that corresponds to the initial macrostate: all boxes on each side

filled with only one kind of molecule. We know that, if the partition is not there, this is not

the equilibrium macrostate. Rather, the equilibrium macrostate is one in which the gases are

mixed uniformly over both containers. According to equation (4.53), for a system size of

order 1 mol this macrostate corresponds to ∼e

equivalent microstates (N ≈6.02×10

By postulate (i) each of these equivalent microstates has the same probability of occurring

as the single microstate that describes the un-mixed gases. There are, however, e

times

more equivalent microstates than in the first case, so it is e

times more likely that we will

observe a homogeneous mixture than separate gases (as long as the partition is not there).

This is what postulate (ii) says, that the equilibrium macrostate, i.e. the final state that the

system will spontaneously evolve to, is the one that corresponds to the most microstates.

Note that we have not proved that equation (4.53) is indeed the maximum, only that it

is (much) larger than (4.49). It happens to be the maximum, though (see, for example,

Glazer & Wark, 2001). The probability that the two gases will spontaneously un-mix and

return to the state they were in before the partition was removed is not zero (this is what

postulate (i) says), but it is so vanishingly small that we can be certain that we will not

observe this phenomenon in the lifetime of the Solar System (quite a bit longer than that,

actually). There is an additional point in this argument. This is the fact that fluctuations

take place in all natural systems, during which the system visits microstates that are very

close, but not identical, to an equilibrium microstate. These fluctuations are so small and

swift that they are not reflected in the macrostate of the system, except when a system is

close to a critical phase transition (more on this later). In principle, another exception could

occur when the system of interest is so small that 1/e

becomes an “observable” number.

In Chapter 14 we will see that this may be a limiting factor for the minimum size of living

organisms.

202 The Second Law of Thermodynamics

4.6.2 Boltzmann’s postulate

The attentive reader might have noticed a striking similarity between equations (4.47) and

(4.53). The two equations are identical, but for a constant multiplicative factor.They suggest,

but do not prove, the following relationship between entropy and number of microstates:

S = k

ln O, (4.54)

where k

= R/N = 1.38 ×10

−23

−1

molecule

−1

is known as Boltzmann’s constant.

Equation (4.54), due to Ludwig Boltzmann and reputedly inscribed on his tombstone, is

known as Boltzmann’s postulate.As with the laws of thermodynamics, it remains a postulate

because it cannot be shown to be valid from simpler statements or observations. We accept

its validity a posteriori, on the basis of the agreement of the theory built upon it with the

observed behavior of nature. Boltzmann’s postulate provides a microscopic interpretation

of entropy that is intuitively satisfying. It says that the highest entropy macrostate is the one

that corresponds to the greatest number of equivalent microstates. The increase in entropy

that accompanies all spontaneous processes in an isolated system is simply a reflection of

the system “falling onto” the most likely set of equivalent microstates. The impossibility

of entropy decreasing in an isolated system should actually be seen as a vanishingly small

probability – “practically impossible” for any conceivable macroscopic system. As to the

relationship between entropy and disorder, which obsesses philosophers, social scientists,

post-modernist writers and other commentators, we shall have more to say about it shortly.

Let us go back to the relationship between (4.53) and (4.47). Using (4.54), we can write

the following equation:

configurational



ln O

−lnO







. (4.55)

The increase in entropy that accompanies mixing of the gases is a function only of the ratio

between the number of microstates in the final and initial states. This increase in entropy is

called the configurational entropy, as it reflects a change in the microscopic configuration

of the system. It is important to realize that equation (4.54) gives the functional relationship

between S and O, but it says nothing about the factors that enter into the definition of the

microstates that are counted by O. The number of microstates in our example was defined

solely on the basis of the identity of the molecules, but in reality other parameters must

be considered too, most importantly temperature. As the temperature increases so does the

number of quantized energy levels available for atomic vibrations, and therefore the number

of accessible microstates and, by (4.54), the entropy. Because we consider an isothermal

transformation the effect of temperature on the value of O cancels out in equation (4.55),

leaving the change in configuration as the only contribution to the entropy of mixing.

There is an important question here: how does the microscopic interpretation of entropy

relate to the concept of energy dissipation and the fact that an entropy increase reflects an

irreversibly lost opportunity to perform mechanical work? The following is a possible way

of looking at this, which traces its origin to James Clerk Maxwell (who was perhaps the

greatest scientist of the nineteenth century). Suppose that rather than a removable partition

the two vessels with different gases in Fig. 4.7 are separated by a fixed wall with a small

opening covered by a revolving trapdoor that will turn only when it is simultaneously hit by a

pair of different molecules coming from opposite directions. The door is just large enough to

allow a single molecule through in each direction at any one time (Maxwell used imaginary

demons for this sort of thought experiment, and his demons were actually asked to perform

203 4.6 A microscopic view of entropy

a task opposite to the one we describe here, see, for example, Baylin, 1994). The door is

connected to an external reservoir of mechanical energy, such as a spring or a weight, so that

rotation in one direction compresses the spring (or raises the weight) by a small amount,

and rotation in the opposite direction releases the spring by the same amount. As long as the

door keeps revolving in the same direction there is net storage of mechanical energy, but if

the door flip-flops then there is no net storage nor release of mechanical energy. When we

first free the revolving door with different gases in each container it will only be hit by A

molecules coming from the left and B molecules coming from the right, so it will spin in only

one direction and store mechanical energy.As the gases mix the door may start flip-flopping,

but as long as there is a gradient in composition across the partition there will be a net storage

of mechanical energy, because the door will rotate more often in one direction than in the

other. When the gas compositions become identical on both sides there will be a net amount

of mechanical energy stored in the spring or weight, which came exclusively from diffusive

mixing of the two gases. If we use a removable partition rather than our little trapdoor this

work potential is lost, and entropy is generated, or equivalently, energy is dissipated.

But the gases were allowed to mix anyway, even if they perform work, so didn’t their

entropy increase, as calculated by (4.47)or(4.53)? No, as one must still abide by the First

Law. The mechanical energy stored in the spring came from somewhere: it must have come

from the kinetic energy of the molecules. If the container is adiabatic then the gas cooled

down. Equation (4.53) is now not a complete description of the number of microstates in

the final state, as it does not account for the fact that, as temperature decreases, the number

of accessible energy levels of the molecules decreases too, and so does the number of

microstates (see Glazer & Wark, 2001). Does the trapdoor example carry over to entropy

generation by diffusive heat flow? Yes, but it is less obvious how. The key is that O changes

with temperature, so we need to imagine some contraption that is able to store mechanical

energy by intercepting packages of molecular kinetic energy exchanged between bodies at

different temperatures.

Worked Example 4.3 Configurational entropy in crystals

Configurational entropy plays an important role in the thermodynamic characterization of

minerals and melts, that we will study in later chapters. Here we focus on the configurational

entropy of minerals of constant composition and variable crystalline structure. Consider the

case of potassium feldspar. A unit cell of potassium feldspar consists of four formula units,

so that it has the composition: K

. Silicon and aluminum occupy tetrahedral

sites in the crystalline structure. There are a total of sixteen tetrahedral sites in a potassium

feldspar unit cell. In all potassium feldspar polymorphs it is possible to distinguish between

two types of tetrahedral sites, on the basis of their sizes and symmetries, which we can call

T1 and T2 sites, and such that there are eight T1 sites and eight T2 sites. In addition, in

some of the crystal forms it is also possible to discriminate between two subsets of the T1

sites, which we will label T1a and T1b. The four Al and twelve Si atoms can occupy the

sixteen tetrahedral sites in a number of different ways, giving rise to minerals with different

crystal symmetries and different amounts of configurational entropy.

Consider microcline first. In this mineral the T1a and T1b sites are distinguishable. The

four T1a sites are occupied by Al, whereas the four T1b sites and the eight T2 sites are

occupied by Si. This regular distribution of Si and Al atoms is said to constitute a structure

with long-range order. It is also a structure with a high information content: we have total

certainty of what we are going to find in each type of site. In sanidine, on the other hand,

204 The Second Law of Thermodynamics

Al and Si distribute themselves randomly over all sixteen tetrahedral sites, such that, one

average (i.e. in a macrsocopic crystal with ∼ N atoms in it) there are six Si and two Al

atoms in the T1 sites and six Si and two Al atoms in the T2 sites. This structure lacks long-

range order, and has a low information content: all we know is that we have a one in three

probability of finding an Al atom in any one tetrahedral site, compared to the certainty that

we had in the case of the ordered structure of microcline.

We will now count the number of equivalent microstates that give rise to each of the two

macrostates, microcline and sanidine. One “unit cell mol” of potassium feldspar contains

N unit cells, i.e. 4N Al atoms and 12N Si atoms. The objects that we will count are

crystalline sites, each of which can be in two possible states: filled with Si or filled with Al.

In the following equations, the numerator is the number of crystalline sites, the first term in

the denominator is the number of those sites occupied by Si, and the second term in the

denominator is the number of sites occupied by Al. For microcline we must consider the

number of microstates of each of the three types of distinguishable sites, so that we have:

T1a

(

)

(

)

T1b

(

)

(

)

!0!

(

)

(

)

!0!

(4.56)

The total number of microstates for the microcline macrostate is:

microcline

T1a

T1b

=1 (4.57)

or:

ln O

microcline

=0. (4.58)

For sanidine:

(

)

(

)

(

)

(

)

(

)

(

)

(4.59)

using Stirling’s approximation we find:

ln O

=ln O

=6N ln





+2N ln 4 (4.60)

and:

ln O

sanidine

=ln O

+lnO

=2N



6ln





+2ln4



. (4.61)

The sanidine macrostate corresponds to a (much) greater number of microstates than the

microcline macrostate, so by Boltzmann’s postulate it must have a higher entropy. This

configurational entropy does not reflect a change in the composition of the phase, as in

the example of the mixing gases, but rather a change in the microscopic configuration of

205 4.6 A microscopic view of entropy

the crystal. We use Bolztmann’s equation, (4.54), to calculate the configurational entropies

of the two polymorphs. Noting that equations (4.58) and (4.61) are based on four formula

units of potassium feldspar, the molar configurational entropies (i.e. per mol of KAlSi

)

are:

configurational, microcline

ln O

microcline

=0 (4.62)

and:

configurational, sanidine

ln O

sanidine



3ln





+ln4



=R ln



256



≈18.7J K

−1

mol

−1

(4.63)

The total entropy of a crystal, S

total

, is in general made up of two contributions:

total

thermal

configurational

. (4.64)

One contribution, S

thermal

, arises from the distribution of (quantized) energy levels of the

constituent atoms, and is called the thermal or vibrational component of entropy. Thermal

entropy increases with temperature, as the vibrational energy of the atoms gets “dispersed”

over a greater number of possible energy levels, which increases the number of accessible

microstates. Configurational entropy, S

configurational

, arises from the distribution of atoms in

the crystalline structure and remains constant with temperature as long as the distribution of

atoms between different crystalline sites does not change (e.g. as long as sanidine does not

invert to microcline). In a crystal with perfect long-range order there is no configurational

entropy, but at all finite temperatures the ordered crystal still has thermal entropy (see

Section 4.7).

The lower “information content” of the crystalline structure with greater configurational

entropy (sanidine in this example) is at the core of the relationship between “entropy and

disorder”: greater order implies greater certainty of what kind of atom is in what kind of

crystalline site, and therefore a lower number of microstates and lower configurational

entropy. It is a tragedy that this concept, that has a strict and unambiguous meaning in the

context of the microscopic structure of matter, has been misunderstood and misappropriated

by non-scientists, who then proceed to apply it erroneously in a wide range of contexts where

it does not belong. Entropy as a measure of disorder is a strictly microscopic concept and is

meaningless as a description of “macroscopic order”. Perhaps the most egregious example

of the misuse of the concept of entropy is its erroneous application to support the spurious

claim that biological evolution, and indeed the existence of life itself, requires a supernatural

explanation. I will dispel these detestable notions in Chapter 14.

There is one final point that you may be wondering about. If sanidine has higher entropy

than microcline (i.e. it is a macrostate that is more likely because it corresponds to a larger

number of equivalent microstates), then why does microcline exist at all? Shouldn’t it

spontaneously invert to sanidine? If the crystal were an isolated system (constant internal

energy and volume) then it would, subject to the removal of constraints (interatomic forces,

which would be the equivalent of the partition in Fig. 4.7). But the direction of a spontaneous

change in a crystal that is not an isolated system (which is the common situation in nature)

is not necessarily determined by an increase in entropy, but rather by a decrease in the

thermodynamic potential appropriate to the constraints on the system. We return to this in

Sections 4.8 and 4.9.

206 The Second Law of Thermodynamics

4.7 The Third Law of Thermodynamics

4.7.1 Statement of the Third Law of Thermodynamics

There is an additional principle, called the Third Law of Thermodynamics, that is inde-

pendent of the First and Second Laws. It is essential in the development of chemical

thermodynamics, although much of classical thermodynamics and its applications to heat

engines and other engineering processes do not require it. We introduce the Third Law

by stating that experimental evidence shows that, as temperature approaches 0 K, heat

capacities (C

and C

) approach zero faster than temperature, i.e.:

lim

T →0





=0. (4.65)

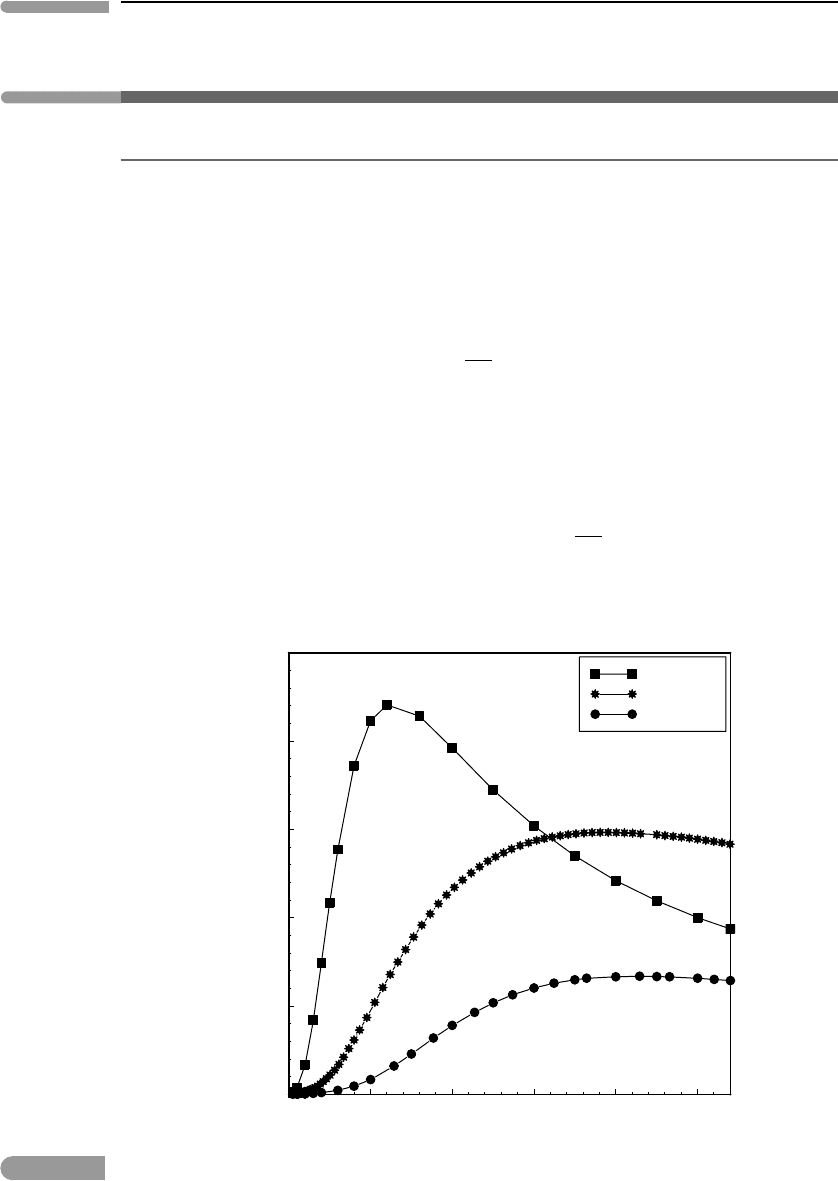
Afew examples are shown in Fig. 4.8. A consequence of (4.65) is that the entropy difference

of a substance between 0 K and any other temperature, T, is a finite value. Assuming that

heating takes place at constant pressure, and that there are no phase transitions between 0

and T, then dQ =C

dT , and we have:

(

)

−S

(

)



dS =



dT (4.66)

with condition (4.65) guaranteeing that the integral does not blow up. An unknown

integration constant remains, however, which is the entropy at 0 K.

0 50 100 150 200 250

0.1

0.2

0.3

0.4

0.5

T (K)

/T(JK

–2

mol

–1

)

KCl

Enstatite

MgO

Fig. 4.8

Low temperature behaviors of C

/T for an ionic crystal (KCl, data from Berg & Morrison, 1957), a crystalline oxide

(MgO, data from Barron et al., 1959) and a crystalline silicate (enstatite, data from Krupka et al., 1985).

207 4.7 The Third Law of Thermodynamics

It is found that the entropy change associated with any transition between equilib-

rium states of perfect crystalline substances vanishes as well as T → 0. The meaning of

“perfect crystalline substance” is that the crystal has no configurational entropy, i.e. that its

atoms are perfectly ordered in the sense that we discussed in Worked Example 4.3. Consider

a transformation A → B between equilibrium states of perfect crystals. This could be, for

instance, a chemical reaction. Using the notation for changes in state variables that we laid

out in Section 1.13.1, we have:



P ,T



P ,T



−



P ,T





P ,0



−



P ,0









dT −







dT (4.67)

and 

P ,T

→0 when T → 0. The low-temperature behavior of heat capacities, equation

(4.65), means that the two integrals in (4.67) also vanish as T → 0. One must therefore

conclude that:

lim

T →0



P ,0



−



P ,0





=0. (4.68)

The integration constant in (4.66), which is the entropy at absolute zero, is therefore the

same for all perfect crystalline substances. This was the original statement of the Third Law,

put forward by the physical chemist W. Nernst in 1906. Note that (4.68) does not fix the

absolute value of S at 0 K, only that the value is the same for all crystalline substances.

Max Planck gave a stronger statement of the Third Law in 1916, by postulating that entropy

vanishes at 0 K. Planck’s statement of the Third Law, which is the one that is widely accepted

today (and which subsumes Nernst’s original statement) is that the entropy of all perfect

crystalline substances vanishes at 0 K. The emphasis on “perfect crystalline substance” is

important. For example, Planck’s statement of the Third Law implies that the entropy of

microcline vanishes at 0 K. The entropy of sanidine, however, does not vanish, because its

configurational entropy remains unchanged with temperature (as long as the atoms don’t

order themselves spontaneously, in which case it is no longer sanidine).

The Third Law remains a postulate (just as the First and Second Laws), but its plausibility

can be demonstrated from quantum mechanical arguments. There is another statement of

the Third Law, which can be shown to be equivalent to Planck’s statement, and which is

also due to Nernst. This is that it is impossible to reach T =0 in a finite number of reversible

steps. We will not be concerned with this statement in this book, but the interested reader

can see Baylin (1994) for a derivation.

4.7.2 An absolute entropy scale

The Third Law of Thermodynamics makes it possible to define absolute values of entropy

for all substances. This is in contrast to energy functions, such as enthalpy. Recall that in

Section 1.13.1 we constructed a scale for enthalpy by setting to zero the enthalpies of all

pure elements in their stable forms at 298.15 K and 1 bar, and then defining enthalpies of

formation for compounds relative to this arbitrary zero. The Third Law says that we cannot

do this for entropy. From equation (4.66) it follows that the entropy of all substances,

including pure elements, at 298.15 K and 1 bar is not zero. Using Planck’s postulate, we

define the reference state entropy, also called the reference state Third Law entropy, and