Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics

Подождите немного. Документ загружается.

7.2 Darboux-covariant Lax pairs in terms of Y-functions 209

where c

is an arbitrary constant. Setting c

= c

=0,weobtain

= c



∂



+ c

, (7.71)

indicating that the simplest Darboux-covariant third-order evolution equation

to be associated with (7.58) has the form (setting c

=0,c

=4)

(ψ

)ψ ≡ 0,

=4∂

+6Q

∂

+3Q

. (7.72)

Together with (7.54) it produces an equiva l ent version of our previous linear

system (7.23) for the KdV equation, obtained by replacing the second equation

by the combination

[∂

+ L

+3∂

− λ)]ψ =0. (7.73)

The operator

corresponds precisely to the third-order op erator which gives

rise to the KdV equation in the Lax formalism [350]:

[∂

]=(Q

+ Q

+3Q

)

=0. (7.74)

The full Darboux-covariant system obtained with expression (7.71) for L

− λ)ψ =0, (∂

+ L

)ψ =0, (7.75)

corresponds, through the map v =lnψ,tothemultipotential Y-system

(v, v + Q)=λ, (7.76)

(v)+c

v, v + Q

(3)

+ c

v, v + Q

(2)

=0,

in which

(3)

(2)

= Q

. (7.77)

An interesting alternative to this system results from an interchange be-

tween Y

(v, v + Q)andc



v, v + Q

(3)



+ c



v, v + Q

(2)



v, v + Q

(3)

+ c

v, v + Q

(2)

= λ,

(v)+Y

(v, v + Q)=0, (7.78)

which corresponds to an alternative Lax-like system with the third-order

eigenvalue equation and second-order time evolution:

ψ ≡ (c

∂

+ c

∂

+ b

∂

+ b

)ψ = λψ,

(∂

+ L

)ψ ≡ ∂

+ ψ

+ Q

ψ =0, (7.79)

where the b

, i =0, 1, are given by (7.57).

210 7 Important links

Let G

be a transformation generated by a (nonvanishing) solution φ of

the system (7.79). It still maps the operators ∂

+ L

and L

−λ onto similar

operators,

(∂

+ L

−1

= ∂



= L

(



), (7.80)

− λ)G

−1



− λ,



= c

∂

+ c

∂



∂



, (7.81)

where the diﬀerences ∆b

≡



− b

are given by (7.60) and (7.61) and where

the following diﬀerential consequences of (7.79) have b een taken into a ccount:

+(σ

+ σ

)

+ Q

=0, (7.82)

(σ

+3σσ

+ σ

)

+ c

(σ

+ σ

)

+(b

σ)

+ b

0,x

=0. (7.83)

Extending the condition (7.64) to r = 0, we ﬁnd by means of the above

analysis that the covariance of L

− λ with ∂

+ L

is guaranteed if

+ c

= c

− Q

)+c

, (7.84)

where c

and c

are arbitrary constants. Setting c

= c

= 0, we ﬁnd

= c



∂

− Q

)



+ c

, (7.85)

yielding the simplest Darboux-covariant system of type (7.79):



ψ = λψ, (∂

+ L

)ψ =0, (7.86)



=4∂

+6Q

∂

+3(Q

− Q

) .

The op erators



and ∂

+ L

are found to constitute the Lax pair for an

equation which is nothing other than the potential version of the Boussinesq

equation (7.26) in which t has been rescaled (t = aτ, a

= −3):

[∂

+ L



]=−(3Q

+ Q

+3Q

)

=(Q

2τ

− Q

− 3Q

)

. (7.87)

It is easy to verify that the system (7.86) taken with t = aτ and a

= −3is

the equivalent version of our previous linear system (7.32) for the Boussinesq

equation which results from subtracting a times the x-derivative of the ﬁrst

equation from the second one. The full Darboux-covariant system obtained

with expression (7.85),

− λ)ψ =0, (∂

+ L

)ψ =0, (7.88)

corresponds, through the map v =lnψ, to a “covariant” version of the system

(7.78) in which

(3)

and Q

(2)

= Q

− Q

). (7.89)

7.2 Darboux-covariant Lax pairs in terms of Y-functions 211

The striking similarity between the covariant Y-systems associated with

the KdV and Boussinesq equations reveals a deep connection between both

soliton systems. It suﬃces to consider the next step which leads us from the

system (7.78) to an alternative version with two evolution equations corre-

sponding to two t-variables (t

has the dimensio n p):

(v)+Y

(v, v + Q)=0, (7.90)

(v)+c

v, v + Q

(2)

+ c

v, v + Q

(3)

=0.

It is clear from the above analysis that the Darboux covariance of the corre-

sponding linear system for ψ =expv,

(∂

+ L

)ψ =0,

∂

+ c

∂

+ c

∂

+3c

(3)

∂

+ c

(2)

ψ =0, (7.91)

is still guaranteed by the conditions (7.89) on Q

(3)

and Q

(2)

.Inparticular,

it is found that the compatibility of the simplest covariant system (setting

=0,c

=4),

(∂

+ L

)ψ =0, (∂



)ψ =0, (7.92)



=4∂

+6Q

∂

+3(Q

− Q

is subjected to the condition

[∂



,∂

+ L

]=[P

x,t

(Q)+3P

(Q)+P

(Q)]

=0, (7.93)

which is a potential version of the KP equation:

KP(u) ≡ (u

+ u

+6uu

)

+3u

=0, (7.94)

obtained by setting u = Q

and by integrating once with respect to x.We

wish to stress that the above derivation of a covariant Lax pair for the KdV

equation produced three closely related Dar boux-covariant systems hinting in

a direct manner at the (well-known) common origin of the KdV and Boussi-

nesq equations as reductions of the K P equation.

We end our discussion with a direct derivation of a Darboux-covariant

equivalent to the linear system (7.39) that we associated with the Lax equation

(7.34). Our starting point is the multipotential Y-system (c

is a constant),

(v, v + Q)=λ, Y

(v)+



i=2

v, v + Q

(i)

=0, (7.95)

or its linear version for ψ =expv,

− λ)ψ =0, (∂

+ L

)ψ =0, (7.96)

= c

∂

+ c

∂

+ b

∂

+ b

∂

+ b

∂

+ b

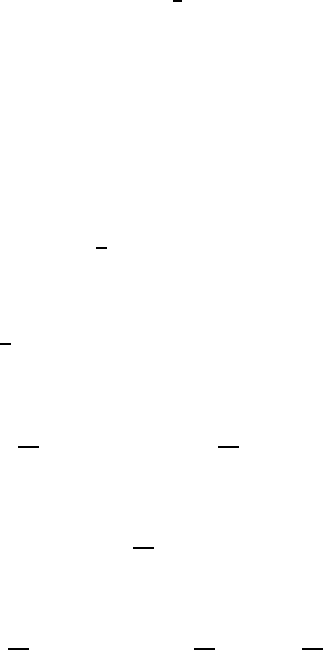
212 7 Important links

with

=10c

(5)

+ c

=6c

(4)

+ c

=3c

(3)

+5c

(

(5)

)

+ c

, (7.97)

= c

(2)

+ c

(

(4)

)

+ c

Let G

be a transformation (7.51) generated by a (nonvanishing) solution φ

of the system (7.96) and (7.97). It maps L

− λ and ∂

+ L

onto the similar

operators (7.60) and ∂



,with



= c

∂

+ c

∂



∂



∂



∂



, (7.98)

where

∆b

≡



− b

=5c

∆Q

∆b

≡



− b

= b

3,x

+ σ∆b

+4c

+10c

∆b

≡



− b

= b

2,x

+ σ∆b

+3σ



+6c

+10c

∆b

≡



− b

= b

1,x

+ σ∆b

+2σ



+3σ



+4c

+5c

(7.99)

In order to ensure the Darboux covariance of ∂

+ L

with L

− λ,wemust

again determine expressions F

for b

, i =0, 1, 2, 3, in terms of Q

and its

derivatives, which are such that condition (7.65) is satisﬁed at i =0, 1, 2, 3,

with (7.64). It is clear from (7.98) that F

can be chosen to b e linear in Q

+ c

, (7.100)

where c

is an arbitrary constant. Equation (7.98) then becomes

∆b

+5c

σσ

+4c

+10c

. (7.101)

Using (7.62), we rewrite it as

∆b

=4c

=2c

∆Q

, (7.102)

indicating that F

can be chosen to be linear in Q

and Q

,so

=2c

+ c

, (7.103)

where c

is an arbitrary constant. Hence, we obtain

∆b

=2c

+4c

σσ

+3c

+15c

+6c

+10c

, (7.104)

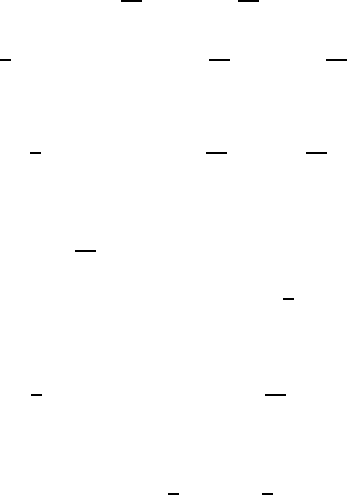
7.2 Darboux-covariant Lax pairs in terms of Y-functions 213

or, using (7.62),

∆b

=2c

∆Q

(2Q

∆Q

+ ∆Q

∆Q

)

∆Q

+2c

∆Q

∆





. (7.105)

It follows that F

can be chosen to be linear in Q

,andQ

,so

+2c

+ c

. (7.106)

It is found from these results and (7.62) that ∆b

becomes

∆b

[∆Q

+2∆(Q

)] (7.107)

+ c



∆Q

+ ∆





∆Q

+ c

∆Q

indicating that the appropriate expression for b

= c

+ c

+ Q

+2Q

)+c

. (7.108)

Setting c

= c

= 0, we obtain the following expression for L

= c

+ c



∂



+ c



, (7.109)

with (choosing c

= 16)



=16∂

+40Q

∂

+60Q

∂

+(50Q

+30Q

)∂

+ 15(Q

+2Q

(7.110)

The rela tions between diﬀerent potentials appearing in the covariant system

(7.95) are determined by (7.97), (7.100), (7.103), (7.106), and (7.108). The

simplest Darboux-covariant ﬁfth-order evolution equation (7.97) to be asso ci-

ated with (7.96) has the form

∂



ψ =0. (7.111)

It is easy to see that the system (7.96) and (7.111) is equivalent to the original

system (7.39):



= L

+15



∂

+(Q

+ λ)∂

+ Q



− λ). (7.112)

Notice that the appearance of the third-order Darboux-covariant operator L

as a part of the general ﬁfth-order covariant operator L

can be regarded

as a direct conﬁrmation of the close relationship between the KdV and Lax

equations as the third- and ﬁfth-order members of the same hierarchy.

214 7 Important links

7.3 B¨acklund transformations and Noether theorem

BTs naturally arise when the Darboux formalism is “projected” to solutions

of nonlinear equations (the potentials o f the corresponding Lax repr esenta-

tion). The action is simple: “wave functions” of the Lax equations should be

excluded [239].

7.3.1 BT and inﬁnitesimal BT

In the previous section we showed that the bilinear BT is a DT covariant form

of the equations of the Hirota method. For the KdV equation it is (7.25), which

is obtained from (7.22). The second relation (7.22) is nothing more than the

ﬁrst equation of the classical BT, relating the ﬁelds w = Q

and w

′

= Q

′

(w + w

′

)

=(w − w

′

)

− κ

, (7.113)

(w + w

′

)

= −2(w − w

′

)(w − w

′

)

+(w

− w

′

)

+3((w − w

′

)

− κ

)

while the second equation (we take the form of [407], the appropriate change

of notations is used) is derived from (7.18) in terms of the Q and Q

′

ﬁelds

of the potential KdV equation (7.15); we denote 2μ = −κ

.Theformofthis

equation is not uni que, because the ﬁrst one ca n be used.

The famous consequence of the BT (7.113) is that both variables w and

′

are solutions of the potential KdV equation

Λw ≡ w

− 6w

+ w

xxx

=0. (7.114)

Steudel [407, 408, 409] derived conservation laws for soliton equations by

application o f the No ether theorem, imposing the BT in a version of the

extended interpretation of

′

= B

w ≡ w + κ[1 + κ

−2

′

+ w

)]

1/2

, (7.115)

which is one of the solutions of the ﬁrst relation in (7.113) with resp ect to

′

− w. The real-valued w is in the realm of the extended BT transform, if

inf[1 + κ

−2

′

+ w

)] ≥ 0, or |w

′

+ w

|≤M

, |κ|≥M.

Theorem 7.1. Let

= B

, (7.116)

then

= B

(7.117)

and

− w

)(w

− w

)=κ

− κ

. (7.118)

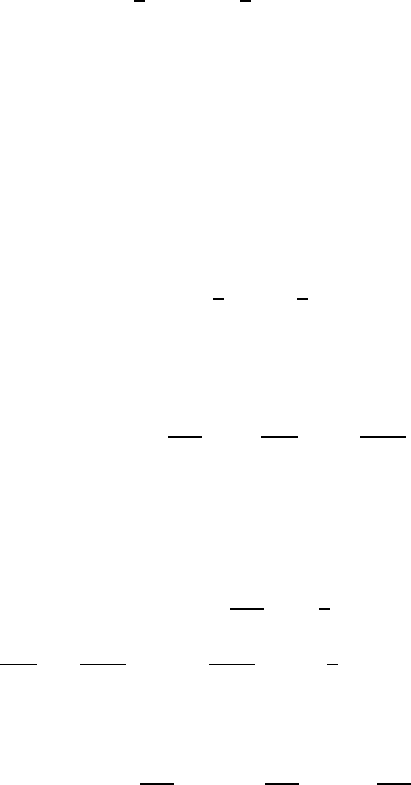
7.3 B¨acklund transformations and Noether theorem 215

The fundamental property of the extension basis is that (7.115) is valid

not only for solutions of the potential KdV equation. In other words, the

Laurent series

δw = κ + A

−1

+ A

−2

+ ... (7.119)

represents the inﬁnitesimal transform at inﬁnity on the κ-plane. Equating the

x-derivative of the right-hand side of (7.119) and the right-hand side of the

ﬁrst relation in (7.113) yields

= w

(n−1)x

−

n−2



r=1

n−r−1

,n=2, 3, 4,.... (7.120)

These formulas were ﬁrst derived by Zakharov and Faddeev [469] in the con-

text of the IST method; see also [385, 445]. Note also that the expansion

(7.119) after diﬀerentiation in x gives an alternative representation of a DT

as δw

∼ u[1] − u. The recurrent relations (7.120) are solved explicitly:

= w

/2,A

= w

xxx

− w

/2,A

= w

xxxx

/8 − w

,.... (7.121)

7.3.2 Noether identity and Noether theorem

A Lagrangian density for the KdV equation is chosen so that

L =

− 2w

(7.122)

gives the po tential KdV equation (7.114) as the Euler equation. A variant of

the Noether theorem for the dependence of L on w

is based on the following

form for the variation (the Frech´et diﬀerential on the prolonged space):

δL ≡

∂L

∂w

δw

∂L

∂w

δw

∂L

∂w

δw

. (7.123)

A decomposition of the right-hand side of (7.134) into a divergence and a term

proportional δw gives the Noether identity

δL = A

+ B

− Λδw, (7.124)

where

A =

∂L

∂w

δw =

δw, (7.125)

B =

(

∂L

∂w

−



∂L

∂w



)

δw+

∂L

∂w

δw



+ w

xxx

− 6w



δw−w

δw

(7.126)

The expression for Λ is given by (7.114). A proof of the theorem follows from

the identity



∂L

∂w

δw





∂L

∂w



δw +

∂L

∂w

δw

, (7.127)

and similar ones for other derivatives and the Euler equation. The identity

(7.124) proves the No ether theorem:

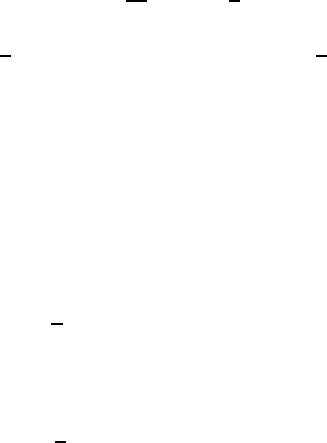
216 7 Important links

Theorem 7.2. If the Lagrangian changes by a divergence

δL = ǫ(Θ

+ Ξ

) (7.128)

under the inﬁnitesimal transformations w → w + ǫf, then, for all solutions of

the potential KdV equation Λw =0, the conservation law

+ X

= 0 (7.129)

exists, with

T = ǫ

−1

A −Θ, (7.130)

X = ǫ

−1

B − Ξ. (7.131)

The following lemma occurs:

Lemma 7.3. Let d

= w

− w

and

L[w

] − L[w

]=Θ

+ Ξ

, (7.132)

with

= −

, (7.133)

= d



−

− 2κ

+ w

− κ

) − 2w

+ w

)



Then the tr ansformation B

is the Noether transformation.

This is proved by the deﬁnitions of the Lagrangian (7.122) and w

(7.116) on

the basis of (7.113). The product B

κ+ǫ

−κ

, being the Noether transformation,

generates the vector (Θ, Ξ) such that

δL = L[w

] − L[w

]=ǫ(Θ

+ Ξ

) (7.134)

determines the variation about the ﬁxed w

. Finally, the part of the vector

(Θ, Ξ),

T = −

,X=2κd

− κ

), (7.135)

which is symmetric with respect to κ →−κ (the symmetry of the BT is

accounted for), contributes indeed to the Noether conservation law:

+2d

(κ

− w

)

. (7.136)

The substitution of expansion (7.119) into (7.136) pro duces the conservation

laws

2r −1

)

+4(A

2r +1

− w

2r −1

)

=0,r=1, 2,... (7.137)

in the form of Wadati et al. [445].

7.4 From singular manifold method to Moutard transformation 217

7.3.3 Comment on Miura map

The ﬁrst relation in (7.113) for imaginary κ =ik in terms of d

= w

− w

∗

is nothing more than the Miura link for u =2w

= σ

+ k

− u,

or, in the notation of this section,

= σ = φ

/φ,

where φ is a solution of

−φ

+ uφ = −k

φ.

This link immediately leads to the continuum conservation law from the cel-

ebrated paper of Miura et al. [335]

(φ

∗

φ)

+(φ

∗

+ φφ

∗

− 4|φ

|−6k

∗

φ)

in the context of the Noether theorem.

Quite similarly the sine– Gordon equation is treated in [409].

7.4 From singular manifold method to Moutard

transformation

Paper [10] contains the so-called Ablowitz–Ramani–Segur conjecture that in-

corporated the Painlev´e property [360]. This result was extended by Weiss

et al. [449] as the Weiss–Tabor–Carnevale theory to check the Painlev´eprop-

erty for a PDE.

Est´evez and Leble [145, 146] developed a pro cedure to derive the Moutard

transformation (and hence the DTs ) in the framework of the singular manifold

method. The generalization of these ideas for the case of two Painlev´e branches

was made in [143].

We will illustrate the idea using the example of the singular mani-

fold method analysis of a version of the 2+1 KdV (Boiti–Leon–Manna–

Pempinelli 1) equation ([59]). Let us write this equation in the form [145]

=(m

xxy

+ m

)

. (7.138)

It is proved that (7.138) has the standard Painlev´e property, i.e., its solutions

can be locally expanded in terms of four arbitrary functions. The truncated

expansion produces the auto-BT

m[1] = m +6

, (7.139)

218 7 Important links

which links two solutions of (7.138) by the “singular manifold” function φ.

The substitution of (7.139) into (7.138) and application of the generalized

procedure [146] leads to the Lax pair

xxx

− φ

+ m

=0, 3φ

+ m

φ =0. (7.140)

A consideration of (7.139) as a transformation m → m[1] and the truncated

expansion for the transformed function ψ[1],

ψ[1] =

, (7.141)

which is the solution of the Lax pair (7.140) with the transform m[1], yields

the following equations for p:

= −2ψφ

= −2φψ

=2ψ

− 2φ

− 2ψφ

. (7.142)

It can be proved that the form

dΩ = −ψφ

dx − φψ

dy +(ψ

− φ

− ψφ

)dt (7.143)

is exact (i.e., dp = −2dΩ) on solutions ψ and φ of the Lax equations and

hence there exists

ψ[1] = ψ − 2

Ω(ψ, φ)

, (7.144)

which coincides with the Moutard transformation [340, 341]. The method

seems to be an eﬀective tool to derive the Moutard transformation formalism

in 2+1 dimensions [140]. It was further applied to generate the DTs for the

Bogoyavlenskii equation in 2 + 1 dimensions [144]. The constructive elements

of the theory are presented in [141].

7.5 Zakharov–Shabat dressing method via operator

factorization

7.5.1 Sketch of IST method

In the “new history” of the soliton theory, half a century after the B¨acklund–

Moutard–Darboux transformations, the notion of dressing appeared within

the inverse scattering pro blem, when solving the Cauchy problem for the KdV

equation [474]. To begin with, let us sketch the IST method and introduce

scattering data for the one-dimensional Sturm–Liouville problem

−∂

ψ + u(x)ψ = k

ψ (7.145)

with a localized potential u(x)(ǫ>0, |x|→∞⇒|u(x)x

(1+ǫ)

|→0) and the

spectral parameter k

. The sca ttering data comprise eigenvalues k

=iκ