Das Sh.P. Statistical Physics of Liquids at Freezing and Beyond

Подождите немного. Документ загружается.

7.2 The compressible liquid 331

Table 7.1 The matrix G

−1

corresponding to the MSR action (7.2.12).

ρ g v ˆρ ˆg ˆv

ρ 00 0−ω q

00 0q

−ωδ

iδ

00 0 0iL

−iρ

ˆρω−q

00 0 0

ˆg

−q

ωδ

02β

−1

ˆv

0 iδ

−iρ

00 0

where D

is obtained as

= ρ

(

−q

)

+i ωq



. (7.2.18)

Similarly, for the response part of the matrix G involving correlations between hatted and

unhatted ﬁelds we obtain

R =

⎡

⎢

⎣

ˆρ ˆg

ˆv

ω +i 

qρ



ωρ

ω

ω i



−q



⎤

⎥

⎦

. (7.2.19)

Let us focus on the expression for the density–density correlation function at zeroth

order:

ρρ

(q,ω) = 2β

−1



∗

(7.2.20)

The poles in the correlation functions represent the corresponding hydrodynamic modes.

The zeros of D

thus indicate the existence of two propagating sound modes. In the

hydrodynamic limit this is given by

z =±c

q − q



(7.2.21)

with c

and 

denoting the speed and attenuation, respectively, of sound waves due to

the density ﬂuctuations. The longitudinal part of the current correlations is related to the

density correlations through the relation

ρρ

(q,ω) = q

(q,ω), (7.2.22)

which follows from the continuity equation (6.2.16). Since in the linear case the momentum

density g and current v are trivially connected, g = v/ρ

, the correlation and response

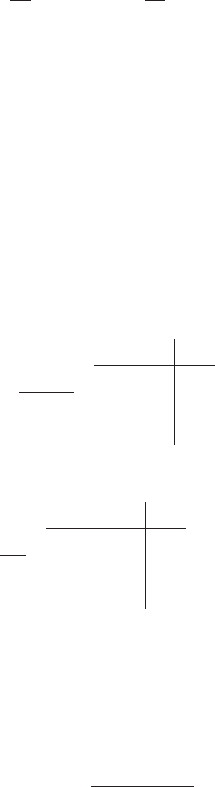
332 Renormalization of the dynamics

functions are trivially connected. Thus, from the inversion of the matrix G

−1

, it directly

follows that

(q,ω) =

(q,ω). (7.2.23)

In a similar way the transverse part of the correlation functions is obtained in block-matrix

form by inverting the matrix G

−1

shown in Table 7.1:



T†





(7.2.24)

involving 2×2 matrices C

and R

. 

denotes the 2×2 null matrix with all the elements

equal to zero. C

and R

denote the matrices of the transverse correlation and response

functions, respectively, and R

T†

is the Hermitian conjugate of R

. From straightforward

inversion of the transverse part of the matrix G

−1

we obtain the following results for C

and R

= 2β

−1

∗

⎡

⎢

⎣

1 v

⎤

⎥

⎦

(7.2.25)

and

⎡

⎢

⎣

1 iω v

⎤

⎥

⎦

, (7.2.26)

where the denominator W

is given by

= ρ

. (7.2.27)

From the above result, let us focus on the typical quantity of transverse-current correlations,

(q,ω) =

2β

−1

+ η

. (7.2.28)

At the level of linear dynamics this represents the diffusive decay mode of transverse shear

in the isotropic liquid. This is expressed in the poles on the RHS of eqn. (7.2.28).Fromthe

explicit form of the zeroth-order (Gaussian) correlation-function matrix we note that the

response function G

αβ

satisﬁes the relation

=−



βα

∗

. (7.2.29)

7.2 The compressible liquid 333

The correlation and response functions in the linear case are related through the ﬂuctuation-

dissipation relations:

αβ

(q,ω) =−2β

−1



ˆαβ

(q,ω)

. (7.2.30)

This follows directly from the Green-function matrix G

presented above. We shall come

back to the issue of ﬂuctuation–dissipation relations in the nonlinear case later on in this

chapter. The expressions for longitudinal and transverse correlations given by eqns. (7.2.17)

and (7.2.25), respectively, are the same as the corresponding expressions for the correlation

functions in Section 5.3.2 (see eqns. (5.3.88) and (5.3.93), respectively).

Nonlinear dynamics

The nonlinear coupling of the hydrodynamic modes in the equations of motion gives rise to

the non-Gaussian part of the action (7.2.8), and their effects on the correlation and response

functions are obtained in terms of the self-energy matrix  deﬁned through the Schwinger–

Dyson equation (7.1.27). Let us ﬁrst consider the cases in which both external indices in

the matrix of eqn. (7.1.27) correspond to the unhatted ﬁelds. In this case we take note of

the following properties of the general structure of the full Green-function matrix G

−1

(a) [G

−1

]

αβ

= 0, which follows from the action (7.2.8) obtained in the MSR ﬁeld theory.

(b) 

αβ

= 0, which follows from the causal nature of the response functions in the MSR

ﬁeld theory. Since each of the vertices in the MSR action functional consists of at least

one hatted ﬁeld, the diagrams corresponding to the self-energy matrix elements for

which both indices are unhatted will involve a closed loop of response functions and

hence will vanish by causality.

We therefore obtain that the elements of the G

−1

matrix corresponding to the unhatted

ﬁelds, [G

−1

]

αβ

= 0, are similar to those in the Gaussian case. The structure of the full

−1

is similar to the form (7.2.16) in the linear case. Upon inverting the matrix G

−1

,we

obtain for the correlation functions of the physical, unhatted ﬁeld variables

αβ

=−



μν

α ˆμ

ˆμˆν

ˆνβ

, (7.2.31)

where Greek-letter subscripts take the values ρ,g, and v, and the self-energy matrix C

ˆμˆν

is given by

ˆμˆν

= 2β

−1



ˆμˆν

ˆμ ˆg

− 

ˆμˆν

. (7.2.32)

The double-hatted self-energies 

ˆμˆν

vanish if either index corresponds to the density.

Equation (7.2.31) is a key relation expressing the correlation functions in terms of the re-

sponse functions. From the Schwinger–Dyson equation (7.1.27) we obtain that the response

functions G

satisfy

(

−1

)

ˆαμ

3) − 

ˆαμ

(

32) = δ(12)δ

ˆα

(7.2.33)

where we use the convention that the barred index 3, is summed over.

334 Renormalization of the dynamics

Table 7.2 The matrix of the coefﬁcients N

in the numerator

on the RHS of eqn. (7.2.34) for the response functions.

ˆρ ˆg ˆv

ρωρ

+iL ρ

qLq

gq(ρ

+ Lγ) ρ

ω Lω

v q(c

+iωγ ) ω + iq

γ i(ω

−q

)

The self-energies 

ˆαμ

are expressed in perturbation theory in terms of the two-point cor-

relation and response functions. Using the explicit polynomial form of the action (7.2.8),

the response functions are expressed in the general form

α ˆμ

(q,ω) =

α ˆμ

(q,ω)

D(q,ω)

, (7.2.34)

where the matrix N is given in Table 7.2 and the determinant D in the denominator is

given by

D(q,ω) = ρ

(ω

−q

) + iL(q, ω)(ω +iq

γ). (7.2.35)

The various quantities are deﬁned such that ρ

, c

, and L are identiﬁed as the corre-

sponding renormalized quantities for the bare density ρ

, speed of sound squared c

and longitudinal viscosity 

, respectively. We have, in terms of single-hatted or response

self-energies,

(q,ω) = ρ

−i 

ˆvv

(q,ω), (7.2.36)

L(q,ω) = 

+i 

ˆgv

(q,ω), (7.2.37)

(q,ω) = qc

+ 

ˆgρ

(q,ω), (7.2.38)

and γ is deﬁned in terms of the self-energy element 

ˆvρ

≡ qγ .

7.3 Renormalization

In statistical mechanics the linear response to an external disturbance on a system in

equilibrium and the corresponding correlation functions of ﬂuctuations are related. These

are generally termed ﬂuctuation–dissipation theorems (FDTs). We have discussed in Sec-

tion 7.3.1 that in the correlation matrix G of the MSR ﬁeld theory the elements G

ψψ

and G

are related through certain ﬂuctuation–dissipation relations. In the case of linear

dynamics such relations follow directly. However, in the case of nonlinear dynamics the

FDT relations are more restricted. Deker and Haake (1975) demonstrated that such FDT

relations exist in a number of speciﬁc situations. Our interest here is focused on the FDT

relations which are satisﬁed for the case of compressible liquids (Das and Mazenko, 1986;

Miyazaki and Reichman, 2005; Andreanov et al., 2006). The formulation of the FDT in the

7.3 Renormalization 335

MSR ﬁeld theory is closely linked to the time-reversal symmetry of the action functional

corresponding to the nonlinear Langevin equation for the dynamics.

7.3.1 Fluctuation–dissipation relations

Let us consider how the MSR action changes under time reversal. This is closely linked

to how the Langevin equation changes under time reversal. Let us deﬁne the time-reversal

operation on the ﬁeld ψ

T ψ

(t) = 

(−t), (7.3.1)

where 

=±1. For simplicity we suppress the spatial dependence of the ﬁelds and focus

on the time dependence only. The Langevin equation for the time evolution of the slow

mode ψ

is obtained as

∂ψ

∂t

− V

[ψ]+L

δF

δψ

(t)

= ζ

, (7.3.2)

where ζ

is the Gaussian random noise correlated in time by a delta function. We adopt

here the convention that the repeated indices are summed over. The reversible part of the

dynamics is obtained in terms of the Poisson bracket Q

between the slow modes,

[ψ]=Q

[ψ]

δF

δψ

(t)

, (7.3.3)

∂ψ

∂t

−



[ψ]−L

δF

δψ

(t)

= ζ

. (7.3.4)

We also assume here that L

is not dependent on the ﬁelds ψ

and can be treated as a con-

stant input in the dynamic description. Under time reversal the irreversible part involving

the dissipative coefﬁcient L

changes sign,

∂ψ

(−t)

∂(−t)

−



[ψ(−t)]+L

δF

−t

δψ

(−t)

= ζ

, (7.3.5)

where we have used the following time-reversal property for Q

which follows from the

deﬁnition of the Poisson bracket in eqn. (5.3.40):

[ψ(−t)]=−



[ψ(t)]. (7.3.6)

We have also assumed that the bare transport coefﬁcients satisfy the following time-reversal

symmetry:

(−t) = 



. (7.3.7)

Therefore the term involving the Poisson bracket Q

remains invariant under time reversal

while the term involving the dissipation coefﬁcient L

changes sign and represents the

336 Renormalization of the dynamics

irreversible part of the dynamics. We now ﬁnd a transformation T that will maintain the

invariance of the MSR action A[ψ,

ψ]:

A[ψ,

ψ]=





(t)β

−1

(t)

+ i

(t)



∂ψ

∂t

−

[ψ]−L

δF

δψ

(t)



. (7.3.8)

In Appendix A7.3 we show that the transformation rules for the ﬁelds {ψ,

ψ} which

maintain this property are given by

(x, −t) → 

(x, t), (7.3.9)

(x, −t) →−



(x, t) − iβ

δF

δψ

(x, t)



. (7.3.10)

We can now use this to obtain the ﬂuctuation–dissipation relation between the response

function (involving one hatted ﬁeld) and a corresponding correlation function. Let us

consider the response function 

(x, t)ψ



, t



). This response function is nonzero for

t > t



. On the other hand, for t →−t and t



→−t



the corresponding response function



(x, −t)ψ

(x, −t



) vanishes since −t < −t



. Now, using the time-reversal properties

and ψ

given by (7.3.10) and (7.3.9), respectively, one obtains

(x, t)ψ



, t



)

−iβ

(x, t)ψ



, t



)

= 0, (7.3.11)

where the ﬁeld θ is deﬁned as

(x, t) =

δF

δψ

(x, t)

. (7.3.12)

The response function is time-ordered and is nonzero for t > t



. The FDT which follows

from eqn. (7.3.11) is expressed as

(t, t



) = iβ(t − t



(t, t



), (7.3.13)

where (t) is the step function.

In the stationary state we assume time translational invariance and obtain the one-sided

Laplace transform (see eqn. (1.3.10) for its deﬁnition) C

of the correlation function

(x, t)ψ

(x, t



)

(q,ω) = β

−1

(q,ω). (7.3.14)

In the above equation we assumed spatial translational invariance and have taken a Fourier

transform at wave vector q with respect to the spatial coordinates. Equation (7.3.14) leads

to the following useful relation between the Fourier transforms of the correlation and

response functions of the MSR ﬁeld theory involving the nonlinear Langevin equation:

(q,ω) =−2β

−1

Im G

(q,ω). (7.3.15)

7.3 Renormalization 337

The form of the FDT (7.3.13) provides a relation between the response functions (involv-

ing a hatted and an unhatted ﬁeld) and the correlation functions (involving two unhatted

ﬁelds). However (unlike in the case of linear dynamics), this is not a relation involving

only two-point functions. It reduces to that form only in certain special situations. Deker

and Haake (1975) described this in terms of processes of classes A and B, respectively.

Class A. In this case the reversible part of the dynamics is zero and we have a purely

dissipative dynamics. So the equation for the dynamics is obtained from eqn. (7.3.2) as

∂ψ

∂t

+ L

δF

δψ

(t)

= ζ

. (7.3.16)

In this case the dissipative part is not necessarily linear. Using the equation of motion

(7.3.16), the FDT relation (7.3.13) reduces to the form

(t − t



) =−iβ(t − t



)



−1



∂ψ

(t)

∂t

− ζ

(t)





)

=−iβ(t − t



)



−1



∂

∂t

(t)ψ



)

−

(t)ψ



)



, (7.3.17)

assuming that the bare-transport-coefﬁcient matrix L

is independent of the ﬁeld vari-

ables {ψ

}. The second term on the RHS of eqn. (7.3.17) is zero. This is justiﬁed

with the following argument: (a) for t < t



it is equal to zero because of the step

function (t−t



) and (b) for t > t



on average

(t)ψ



)

is zero. The delta-function-

correlated noise ζ(t) can have an inﬂuence on the ﬁeld ψ



) only if t



is greater than t.

Therefore the second term is always zero. We obtain the simpliﬁed FDT relation

(t − t



) =−iβ(t − t



)



−1

∂

∂t

(t)ψ



)

≡−i (t − t



)β



−1

∂

∂t

(t − t



) (7.3.18)

linking the response function to the time derivative of the correlation functions. The

above FDT relation therefore holds in purely dissipative systems having (a) constant

bare transport coefﬁcients and (b) a driving free functional that is non-Gaussian. This

FDT relation therefore applies only to cases in which the noise is additive in nature.

Class B. In this case the dissipative term in the equation of motion is linear in the ﬁelds.

This corresponds to the bare transport coefﬁcient L

being independent of ﬁelds and

the free-energy functional being quadratic in the ﬁelds. The Poisson bracket Q

involved depends on the ﬁelds and gives rise to the nonlinear dynamics. Dynamical

equations of this type have been considered by Kawasaki (1970b) for studying critical

dynamics. The form of the dissipative term in the Langevin equation (7.3.2) implies

that, for constant (independent of the ﬁelds ψ

) bare transport coefﬁcients L

, the func-

tional derivative [δF /δψ

]≡θ

can be expressed as a linear combination of the ﬁelds:

δF

δψ

= β

−1

. (7.3.19)

338 Renormalization of the dynamics

The above relation follows directly from the deﬁnition of the equal-time correlation

matrix S

. The FDT (7.3.15) is expressed in the linear form

(t − t



) = i(t − t



)



−1

(t − t



). (7.3.20)

This analysis was further extended by Miyazaki and Reichman (2005) to the case

in which both Q

and L

, controlling the reversible and irreversible parts of the

dynamics, respectively, have a linear dependence on the ﬁelds:

[ψ]=Q

+ Q

ijk

, (7.3.21)

[ψ]=

(1)

ijk

. (7.3.22)

It was shown from a one-loop analysis that the linear FDT given by eqn. (7.3.20) still

holds in the extension of case B. For recent work on the ﬂuctuation dissipation rela-

tion in Nonlinear Langevin equation with ﬁeld dependent transport coefﬁcients see

Kumaran (2011).

The compressible liquid

We now come back to the discussion of the model for compressible liquids. The set of

equations (6.2.16) and (6.2.20) constitutes a dynamics that does not match class A or

class B described above. The bare transport coefﬁcients are taken to be independent of

the ﬁelds but the free energy is not Gaussian due to the presence of the 1/ρ nonlinear-

ity in the kinetic term (see eqn. (6.2.7)). Therefore, this model does not have a complete

set of FDRs linearly relating correlation and response functions. However, using the time-

translational-invariance properties of the action (7.2.8), some useful ﬂuctuation–dissipation

relations between correlation and response functions involving the ﬁeld g are obtained.

This basically involves observation of the invariance of the MSR action under the following

transformations:

ρ(x, −t) → ρ(x, t), (7.3.23)

(x, −t) →−g

(x, t), (7.3.24)

ˆρ(x, −t) →−ˆρ(x, t) + iβ

δF

δρ (x, t)

=−ˆρ(x, t ) + iβπ(x, t), (7.3.25)

ˆg

(x, −t) →−ˆg

(x, t) + iβ

δF

δg

(x, t)

=−ˆg

(x, t) + iβv

(x, t), (7.3.26)

where we have used the result

δF

δg

(x, t)

= v

(x, t) (7.3.27)

following the dependence of the free-energy functional on the momentum density g

given by eqn. (6.2.7). The ﬁeld π(x, t) is deﬁned as

7.3 Renormalization 339

δF

δρ (x, t)

= π(x, t ). (7.3.28)

Using the transformation (7.3.26), we obtain from the relation (7.3.15) the useful FDT

relation

(q,ω) =−2β

−1

Im G

ˆg

(q,ω), (7.3.29)

where α indicates any of the ﬁelds {ρ,g,v}. The second FDT relation, which follows in a

similar manner from the transformation (7.3.25),is

πα

(q,ω) =−2β

−1

Im G

ˆρα

(q,ω), (7.3.30)

where α indicates any of the ﬁelds {ρ,g,v}.

While eqns. (7.3.29) and (7.3.30) constitute the two basic FDT relations in the

ﬂuctuating-hydrodynamics model, they differ in an important way. The ﬁeld δF/δg

= v

which is involved in the ﬁrst FDT relation also appears in the equation of motion (6.2.20)

of the momentum density in the ﬂuctuating-hydrodynamics description. Indeed, it is this

FDT which proves to be most useful in the analysis presented in the next section. We show

that this FDT is crucial in establishing the renormalizability of the dynamics in the hydro-

dynamic limit. The second FDT, on the other hand, involves the introduction of a new

ﬁeld, π = (δF/δρ), which is absent from the equations of motion of the slow variables,

i.e., eqns. (6.2.16) and (6.2.20). The nonlinear part of this ﬁeld π, which is the functional

derivative of the free energy F with respect to density, comes from (δF

/δρ), where F

the kinetic part involving the 1/ρ nonlinearity. However, this term ﬁnally leads to the well-

known Navier–Stokes nonlinearity ∇

) in the equations of motion and π drops out

from the dynamics. As a result the MSR theory does not have a linear FDT-type relation

between G

ρρ

and G

ρ ˆρ

that is valid for all wave vectors. Such a relation can be obtained

only in the hydrodynamic limit (see eqn. (8.3.10) in Section 8.3.2).

7.3.2 Nonperturbative results

The ﬁeld theory outlined above involves a large number of correlation functions between

the different ﬁelds. We divide them broadly into two categories, namely the correlation

functions denoted by G

ψψ

and the “response” functions G

with the symbols ψ and

referring to any one of the ﬁeld variables or the hatted ones, respectively. Several simple

relations are obtained (Das and Mazenko, 1986) in this respect. The ﬁrst important result is

(q,ω) =−G

∗

βα

(q,ω). (7.3.31)

From eqn. (7.3.31) and the Schwinger–Dyson equation (7.1.27) the following nonpertur-

bative result for the self-energy matrix elements then follows directly:



(q,ω) =−

∗

βα

(q,ω). (7.3.32)

As a consequence of the continuity equation we obtain the following relation between the

correlation functions involving the density ﬁeld ρ and those involving the current g:

ωG

ρβ

(q,ω)− q · G

gβ

(q,ω) = δ

β ˆρ

. (7.3.33)

340 Renormalization of the dynamics

Using the FDT relation (7.3.29), we obtain a number of relations between the correlation

functions and the response functions in a nonperturbative manner. For the isotropic ﬂuid

the description of the renormalized theory is facilitated by treating the longitudinal and

transverse parts separately in the same manner as we did for the case of linearized dynam-

ics. The self-energy matrix can also be split into a longitudinal and a transverse part in the

following way:



(q,ω) =ˆq

ˆq



αβ

(q,ω)+ (δ

−ˆq

ˆq

)

αβ

(q,ω), (7.3.34)

where the superscripts L and T denote the longitudinal and transverse parts, respectively.

The transverse case

We will ﬁrst discuss the relatively simpler transverse case in which the transverse compo-

nents do not couple to the density ﬁeld. Since the cubic and quartic vertices in the MSR

action from eqns. (6.2.16) and (6.2.25) do not contain the g ﬁeld, the self-energy matrix

elements with a g

vanish, i.e., 

=

βg

=0. The transverse correlations are obtained

from the inversion of the transverse part of the correlation matrix presented on the RHS

of eqn. (7.1.27). It follows directly from the ﬂuctuation–dissipation theorem (7.3.29) that

the correlation functions G

and G

are related to the response functions G

g ˆg

and G

v ˆg

respectively. In the renormalized theory these correlation functions are obtained as

(q,ω) =

(βρ

)

−1

ω +iη

(q,ω)

, (7.3.35)

where

(q,ω) =

−1

ω +i η

(q,ω)

where the renormalized function η

(q,ω)is deﬁned as

(q,ω) =





ˆgv

(q,ω)



−1

(q,ω), (7.3.36)

(q,ω) = ρ

−i 

ˆvv

(q,ω).

It is useful to check for the static correlation functions from the limiting values of the renor-

malized correlation functions. For example, the equal-time velocity correlation function is

obtained from the following high-frequency limit:

(q) = lim

ω→∞

ωC

(q,ω) = (βρ

)

−1

, (7.3.37)

where we have assumed that the self-energy 

ˆvv

(q,ω)vanishes as 1/ω for large ω. Simi-

larly, we also obtain χ

= β

−1