Das Sh.P. Statistical Physics of Liquids at Freezing and Beyond

Подождите немного. Документ загружается.

3.1 Classical nucleation theory 121

G

∗

16πγ

3|G

. (3.1.17)

By equating the volume of i

∗

of the critical nucleus to the spherical volume of

(4/3)π R

∗

, we obtain for the radius of the critical nucleus

∗

2γ

|G

(3.1.18)

In this case the constants A and B in eqn. (3.1.13) are given by

A =

aγ

, (3.1.19)

B =

|G

. (3.1.20)

The maximum at n = n

∗

in the free-energy barrier and the corresponding radius R

∗

of the

cluster represent a limiting situation. Note that the above expression (3.1.17) for the free

energy computed from thermodynamic consideration of reversible processes represents

the minimum work needed to form the cluster of i monomers. Furthermore, it is also a

maximum with respect to the variation of the number of monomers i. The critical nucleus

is therefore in a state of unstable equilibrium. A cluster of radius less than R

∗

(having

fewer than n

∗

monomers) is subcritical and shrinks spontaneously. On the other hand,

clusters with radius greater than R

∗

(having more than n

∗

monomers) are supercritical and

increasingly likely to grow.

The classical nucleation theory (CNT) described above involves making approximations

that are questionable. First, it assumes that even a cluster of molecular size has bulk-like

properties at the center. Second, the clusters of monomers forming the nucleus are deﬁned

with a sharp interface between the two phases. More speciﬁcally, the CNT uses a sur-

face free energy for the nuclei that is the same as that of the inﬁnite planar interface,

whereas the actual interface for the small nucleus is in fact sharply curved. Thus, while the

basic ideas used in constructing the CNT capture the essential physics, there is a need for

improvement.

3.1.2 The nucleation rate

Since the supercooled liquid can be maintained in the metastable liquid state well below

the freezing point, considerations of the dynamics become important in understanding the

process of crystal formation from the melt. This dynamic process generally involves clus-

ters of various sizes of the new phase gaining or losing more molecules from the bulk

liquid. This process is idealized in terms of rate equations that are local in time, control-

ling the loss or gain of particles from the clusters of different sizes. At the simplest level,

namely the description of the rate process of growth (of small clusters), the role of mem-

ory is ignored. This essentially amounts to ignoring the correlation in the events which

122 Crystal nucleation

cause the growth or decay of the clusters. The temperature is assumed to remain constant

in the steady state as the cluster grows or decays. This implies that the clusters are in ther-

mal equilibrium while the numbers of particles belonging to them change. The latent heat

evolved in the process of phase change does not affect this condition. We also assume that

the clusters grow or decay by attaching or losing single units or monomers. In the fol-

lowing we consider two cases of nucleation of the crystal: (a) transition from a ﬂuid to a

solid, i.e., crystallization from a low-concentration solution; and (b) crystallization from

a high-density melt, whereby the growth of the nucleus takes place through an activated

process.

Nucleation from low monomer concentration

Let the number of n-particle clusters of the crystalline phase present in the parent phase at

time t be denoted by N

(t).If

and 

−

denote the rates of monomer addition and loss

from the n-particle cluster, respectively, the net number of clusters changing per unit time

from size n to size n + 1 at time t is given by

(t) = 

(t) − 

n+1

−

n+1

(t). (3.1.21)

Equilibrium is reached when N

(for all n) no longer changes with time. The equilibrium

distribution is given by J

(t) = 0, i.e.,



= 

n+1

−

n+1

. (3.1.22)

On the other hand, a steady state is reached when the rates of change of the numbers

of differently sized clusters become constant, i.e., independent of time and size. This is

characterized by N

(t) ≡ N

, giving for the steady-state nucleation rate

≡ J

(t) = 

− 

n+1

−

n+1

. (3.1.23)

In the steady state the rate of change of the number of clusters of a particular size N

independent of time but not necessarily equal to zero. The latter is a particular case corre-

sponding to the equilibrium state. Using the relation (3.1.22), the steady-state nucleation

rate J

deﬁned above satisﬁes the relation

= 



− N

i+1



i+1



. (3.1.24)

Since the equilibrium density N

of the clusters of size i is given by eqn. (3.1.2), we obtain

from (3.1.24) the nucleation rate

= 



− N

i+1

exp

G

i+1

− G

4

. (3.1.25)

3.1 Classical nucleation theory 123

Using the Taylor expansions

i+1

= N

+ i

, (3.1.26)

G

i+1

= G

+ i

G

, (3.1.27)

and keeping terms up to lowest order, with i = 1, we obtain from (3.1.25) the following

differential equation for N





+ β N

G



=−J, (3.1.28)

where we drop from now on the superscript s for the steady state for simpliﬁcation and

use the notation β = 1/(k

T ). Now in general the nucleation rate is deﬁned as 

2/3

, where λ

is the dynamic factor denoting the number of monomers arriving at the

nucleus per unit time per unit area and ai

2/3

is the surface area of the nucleus containing i

monomers. Using this result for 

, we obtain

+ β N

G

=−

−2/3

, (3.1.29)

where

J = J/(aλ

). The formal solution of this equation is obtained by multiplying

(3.1.29) by the factor exp(β G

) and then integrating from an initial point i

up to i to

obtain

= e

−βG



−



dw e

βG

−2/3

+ N

βG



, (3.1.30)

where we deﬁne the number of clusters N

= N

for i = i

. Now we can further simplify

the above expression for N

by choosing i

to be small enough relative to the critical

nucleus (i = i

∗

) that the number of critical nuclei can be expressed in terms of the number

of monomers N per unit volume as given in eqn. (3.1.2), i.e., N

= N exp(β G

). Hence

= e

−βG



−



dw e

βG

−2/3

+ N



. (3.1.31)

Now, for the number of clusters N

with a very large number of particles we should

have lim

i→∞

= 0. On the other hand, since G

reaches a maximum at an inter-

mediate i = i

∗

and falls to a negative value for large values of i,wemustalsohave

lim

i→∞

exp(−βG

) =∞. Therefore, in the large-i limit of eqn. (3.1.30), we obtain the

result

lim

i→∞



−



dw e

βG

−2/3

+ N



= 0. (3.1.32)

The nucleation rate J =

Jaλ

is therefore given by

J =

Naλ

∗

, (3.1.33)

124 Crystal nucleation

where the integral I

∗

is obtained as

∗



∞

exp

[

βG

]

−2/3

dn. (3.1.34)

The free energy G

is maximum at n = n

∗

and the dominant contribution of the integral

∗

in the denominator of the RHS of eqn. (3.1.34) comes from values of n close to n

∗

We evaluate this integral by replacing G

with a Taylor expansion up to quadratic terms,

G

= G

∗

−

G



∗

(n − n

∗

)

, (3.1.35)

and take the limit over n from n = n

∗

to ∞ since the contribution from n = i

to n = i

∗

negligible. This gives

∗

= n

∗

−2/3



2πk

G



∗



1/2

exp



G

∗



. (3.1.36)

The steady-state nucleation rate J is obtained as

J = N 

∗



G



∗

2πk



1/2

exp



−

G

∗



, (3.1.37)

where 

∗

= (an

∗

2/3

)λ

represents the number of monomers that hit the nucleus of sur-

face area A

= an

∗

2/3

per unit time. The nucleation rate is thus expressed in the form of

the general expression

J = J

exp

−G

∗

/(k

T )

. (3.1.38)

The prefactor J

in the above expression for the nucleation rate is proportional to the num-

ber of monomers N per unit volume of the mother phase from which the nucleating cluster

is formed. J

also has the following two components.

(i) The dynamic factor 

∗

represents the number of monomers attaching to the nucleus

per unit time. It is given by A

, where A

is the area of the cluster and λ

denotes

the rate of arrival of monomers on the cluster per unit area.

(ii) The statistical prefactor Z , called the Zel’dovich factor (Zel’dovich, 1943),

Z =



G



∗

2πk



1/2

, (3.1.39)

is determined by the shape of the free-energy surface. Using the expression (3.1.13)

for G

∗

and taking the second derivative gives

G



∗

=|μ|/(3n

∗

). Hence the

Zel’dovich factor is expressed in terms of the chemical potential difference as

Z =



μ

6πk

∗



1/2

. (3.1.40)

3.1 Classical nucleation theory 125

The expression for the nucleation rate J is now obtained in the form

J = N 

∗



μ

6πk

∗



1/2

exp



−

G

∗



(3.1.41)

Note that, in the above expression for the nucleation rate per unit volume per unit time,

N denotes the number of monomers in unit volume. In order to further simplify the

above expression, we need to estimate the dynamic prefactor present in the expression

for the nucleation rate.

In the case of transition from a dilute ﬂuid to a solid, the dynamic prefactor is determined

by taking the ideal-gas approximation for the monomers hitting the surface of the nucleus.

The number of particles λ

falling on the surface per unit time is obtained from the simple

kinetic theory of ideal-gas particles in terms of the equilibrium pressure P

= λ



∞

dv φ(v)(mv)(2π)



π/2

d(cos θ)cosθ

= λ

;

(2πmk

T ), (3.1.42)

where φ(v) denotes Maxwell’s velocity distribution for the ideal gas. Using the ideal-gas

equation of state P

= N

T and the monomer concentration N

at pressure P

is reduced

from its value N at the pressure P of nucleation by a factor S, where S = P/P

. Thus we

obtain for 

∗

for the nucleus of area A



∗



2πm



1/2

. (3.1.43)

For a spherically shaped critical nucleus the area is A

= 4π R

∗

with the radius R

∗

given

by (3.1.18).Using(3.1.15), (3.1.16), and (3.1.10), we obtain for the nucleation rate J the

result

J =

2γ

πm





exp



−

16πv

3(k

T )

(ln S)



(3.1.44)

in which the chemical potential difference μ between the two phases is substituted in

terms of the supersaturation as μ = ln S/(k

T ).

Crystallization from the melt

For nucleation from the condensed phase, the dynamic prefactor in the nucleation rate is

approximated using the reaction-rate approach (Turnbull and Fisher, 1949). In this model

the process of addition of the monomer to the nucleus is assumed to take place through

transition into an intermediate activated state that represents an unstable maximum in the

free energy as shown schematically in Fig. 3.2. This maximum at A corresponds to the

nucleus in the activated state and is intermediate between the two minima corresponding

to the nuclei with i and i + 1 particles, respectively. These two minima correspond to

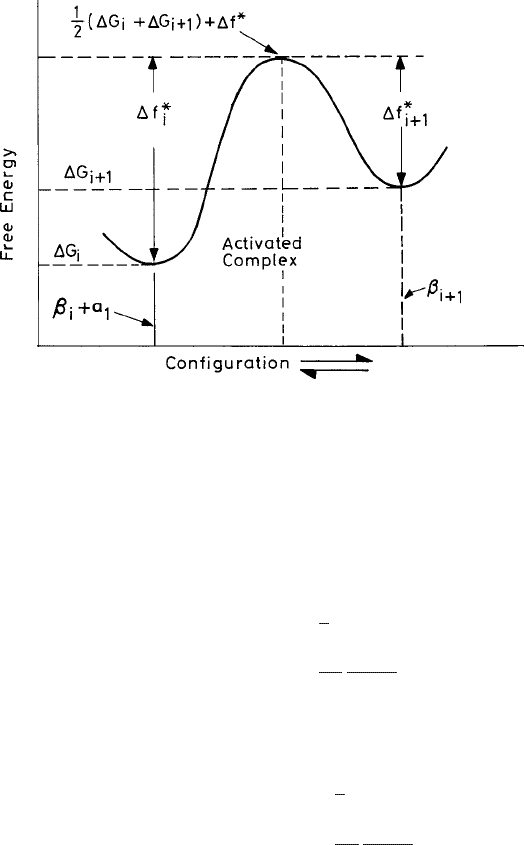
126 Crystal nucleation

Fig. 3.2 A schematic representation of the free energy of activation corresponding to the process of

adding a monomer to a nucleus.

the states of the nucleus before and after the monomer attachment. We assume that the free

energies of formation of the nuclei corresponding to i , A, and i +1 are respectively denoted

by G

, G

, and G

i+1

. The free-energy difference between the states corresponding

to A and i is given by

 f

∗

≡ G

− G

= f

∗

(G

i+1

− G

)

= f

∗

i

dG

, (3.1.45)

where we use the deﬁnition f

∗

= G

−(G

i+1

+G

)/2. Similarly, the free-energy

difference between the nuclei corresponding to A and i + 1is

 f

∗

i+1

≡ G

− G

i+1

= f

∗

−

(G

i+1

− G

)

= f

∗

−

i

dG

. (3.1.46)

The steady-state rate of the nucleation J in this case is obtained in a manner similar to that

used in formulating eqn. (3.1.23), as a sum of two opposite processes. These respectively

correspond to rate processes (at temperature T ) of crossing barriers  f

∗

i+1

and  f

∗

.The

nucleation rate is obtained as

J = ai

2/3

exp



−βf

∗



− exp



−βf

∗

i+1



i+1

, (3.1.47)

where ν

= k

T /h is the primary frequency corresponding to the thermal energy k

T and

2/3

is the surface area of the nucleus with i monomers. Using the Taylor expansions as

3.1 Classical nucleation theory 127

presented in eqn. (3.1.26) and keeping terms of up to lowest order in i, we obtain the

following differential equation for the variation of N



+ β N

G



=−

−2/3

, (3.1.48)

where

J = J/{aν

exp[−f

∗

/(k

T )]exp[− f

∗

/(k

T )]}. Note that (3.1.48) is identical

to eqn. (3.1.29) in the earlier section except that now the rate of monomer attachment per

unit area λ

is replaced by ν

exp[−f

∗

/(k

T )]. Hence the nucleation rate J in this case

is obtained from (3.1.41) in the form

J = N ν

∗

2/3



μ

6πk

∗



1/2

exp



−

G

∗

+  f

∗



, (3.1.49)

where N denotes the number of monomers per unit volume. In the above expression for the

nucleation rate the exponent term involving the free-energy barrier G

∗

is further sim-

pliﬁed. Using (3.1.17), G

∗

is expressed in terms of μ ≡ v

G

, where the chemical

potential difference μ between the two phases is related to the molar enthalpy h and

the molar entropy s through the thermodynamic relation

μ = h − T s = s(T

− T ). (3.1.50)

We have used here the result that close to the melting temperature T

, h = T

s.Using

(3.1.50) in (3.1.17) and the deﬁnition μ = v

G

, we obtain for the free-energy barrier

G

∗

16πv

3 s

− T )

. (3.1.51)

The exponential factor in (3.1.49) simpliﬁes further with the deﬁnition a =



36πv



1/3

and the expression (3.1.16) for i

∗

. We obtain the following result for the nucleation rate:

J = 2N v

T γ

)

1/2

exp



−

 f

∗



exp



−16πγ

T s

− T )



(3.1.52)

for the spherical nucleus. From eqn. (3.1.51) it follows that the free-energy barrier G

be surmounted for the critical nucleus formation falls sharply as the undercooling of the

liquid is increased, i.e., G

∝ T

−2

, where the extent of undercooling is deﬁned as

− T

= 1 −

≡ 1 − T

≡ T

(3.1.53)

in terms of the scaled temperature T

= T /T

.AtT = T

the barrier G

is inﬁnite,

making the nucleation rate zero. To initiate the formation of the crystal seed the temperature

of the liquid has to be well below the freezing point T

, i.e., T

of the liquid has to

be large. We now consider this dependence of the nucleation process on the extent of

undercooling.

128 Crystal nucleation

Dependence on undercooling

The temperature dependences of the two exponential factors in the above expression for the

nucleation rate J result in opposite trends. The ﬁrst factor, exp[−f

∗

/(k

T )] decreases

with lowering of T . On the other hand, the second exponential factor increases as T

decreases, since the difference T = T

− T grows with lowering of the temperature.

In fact, the latter factor in the exponent produces the dominant effect and for an increase

of T by a few degrees the exponential factor in the nucleation rate J changes by orders

of magnitude. To see this, we rewrite eqn. (3.1.52) in the form

J = J

exp



−

G

∗



≡ J

exp



−

(T

)



(3.1.54)

using the deﬁnitions

α =

2/3

h

,ζ=

h

. (3.1.55)

The constant a

= 16π/3 for a spherical droplet. In order to obtain this value of J

the

energy barrier f

∗

has to be estimated. J

is inversely related to the time τ

takenbya

monomer to cross this barrier at the interface and become attached to the nucleus. Let us

write J

in the form C

/τ

, where C

is a constant, and hence the nucleation rate J is

J =

exp



−

G

∗



. (3.1.56)

is inversely proportional to the diffusion constant D

and hence directly proportional

to the viscosity if we assume that the Stokes–Einstein law holds. A lower value of the

viscosity implies a shorter passage time and hence a lower barrier. We ignore the temper-

ature dependence of the viscosity of the undercooled liquid at ﬁrst to keep J

within its

upper bound. The prefactor J

is estimated as 10

(Turnbull and Fisher, 1949), taking

the viscosity of the liquid as 10

−2

P. The effect of undercooling on the crystallization is

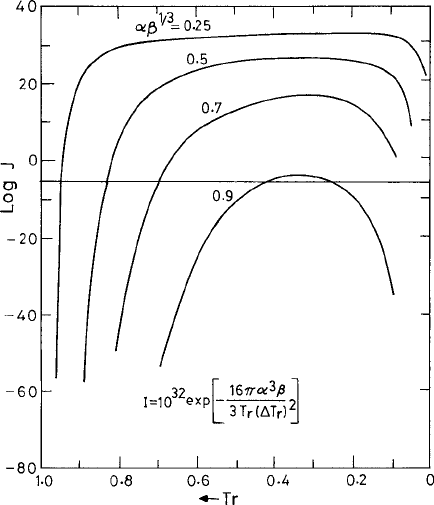
demonstrated through a plot of the nucleation rate with respect to the scaled temperature

.ThisisshowninFig. 3.3 (Turnbull, 1969). The curves are ﬁxed by choosing constant

values of the parameter αζ

1/3

(≡

, say). Close to T

, i.e., at low undercooling (T

≈ 1),

the curve for J corresponding to a ﬁxed 

rises steeply. The curve reaches a maximum at

= 1/3 and falls off to zero as we approach T = 0. In order to be able to observe crys-

tallization experimentally, the rate J must exceed some minimum practical value, say one

nucleus per cubic meter per second (10

−6

−3

−1

). This threshold value for J is shown

by a horizontal line in Fig. 3.3. We notice in this plot of Fig. 3.3 that at low undercooling or

close to T

, corresponding to most values of the parameter 

, the nucleation rate J falls

much below the horizontal line and cannot be observed experimentally. In this case for



> 0.9 the crystallization practically never occurs through a homogeneous nucleation

process. On the other hand, crystallization is almost impossible to suppress for 

< 0.25.

For intermediate values of 

crystallization can remain suppressed only up to moderate

undercooling.

3.1 Classical nucleation theory 129

Fig. 3.3 The logarithm of the rate J



in cm

−3

−1



of homogeneous nucleation in the undercooled

liquid given by (3.1.54) with reduced temperature T

for various values of 

≡ αζ

1/3

. The tem-

perature dependence of the viscosity η present in the prefactor J

in (3.1.54) is ignored here. From

Turnbull (1969).

 Taylor & Francis Group, http://www.informaworld.com

The above analysis is based on two assumptions: ﬁrst, that the Stokes–Einstein relation

holds; and second, that the temperature dependence of the viscosity can be ignored. Both

are violated in the liquid deeply supercooled below T

. A more realistic scenario for the

crystallization process in the supercooled liquid emerges when we take into account (a) the

sharp temperature dependence of the viscosity of the metastable liquid below the freezing

point and (b) the violation of the Stokes–Einstein law. This is considered in the next chapter

in our discussion of the glass transition.

3.1.3 Heterogeneous nucleation

In our discussion of the crystallization process we have so far considered the phenomena

to be taking place in the bulk of the liquid phase. This is generally termed homogeneous

nucleation. In reality, however, the process occurs in a heterogeneous manner around for-

eign particles or impurities distributed in the sample. The surface of the liquid droplet is

also often the source of heterogeneous nucleation. Turnbull (1950) extended the CNT for

homogeneous nucleation to the heterogeneous process by considering the liquid and crys-

talline phases in contact with a solid substrate. The outcome of this is a modiﬁcation of

130 Crystal nucleation

the free-energy barrier to the nucleation as obtained in the CNT in eqn. (3.1.17) in the

following form:

G

∗

16πγ

3|G

(θ). (3.1.57)

The factor f

(θ) is the heterogeneous nucleation factor given by

(θ) =

(2 + cos θ)(1 − cos θ)

, (3.1.58)

where θ is the contact angle between the substrate and the crystal droplet nucleating from

the liquid phase. This contact angle θ is determined in terms of the surface free energies of

the different phases,

cos θ =

− γ

, (3.1.59)

where γ

denotes the surface free energy of the liquid–crystal interface while γ

and γ

respectively, represent the surface free energies for the liquid–substrate interface and the

crystal–substrate interface. If the substrate on which the nucleation is occurring is identical

to the liquid phase we have (γ

− γ

) →−γ

and cos θ → 1, i.e., θ → π. In this limit

we have homogeneous nucleation occurring in the liquid and f

(θ) = 1. The main impli-

cation of the heterogeneous nucleation is therefore represented in terms of a modiﬁcation

of the nucleation barrier. There is also a corresponding modiﬁcation in the pre-exponential

term J

for the nucleation rate as given in (3.4.7) later.

In a bulk heterogeneous process expectedly the factor modifying the nucleation rate

signiﬁes the fraction of the impurity sites which has been used up. Gránásy and Iglól (1997)

assumed a modiﬁed nucleation rate in the following phenomenological form:

J ={ϑ J

}exp



− f

G

∗



, (3.1.60)

where ϑ<1 is the fraction of molecules active in nucleation. f

< 1 is the factor repre-

senting the reduction of the potential barrier due to the presence of heterogeneities, as has

already been discussed above. From eqn. (3.1.17) the exponential factor f

[G

∗

/(k

T )]

in (3.1.60) is proportional to γ





, where γ

is the surface free energy at temper-

ature T . In the CNT the quantity γ

is usually taken to be independent of temperature

and this is rectiﬁed in terms of the quantity χ(T ) = γ

/γ

, which is the ratio of the

temperature-dependent surface tension γ

to the bulk surface tension γ

between the two

phases. Therefore a plot of log[J/J

] against the quantity X ≡ χ(T )





is a straight

line with an intercept on the y axis equal to log ϑ. Using estimates of X from the different

types of theories described above, Gránásy and Iglól (1997) computed the corresponding

prediction for ϑ in each case. Since ϑ should be less than unity by deﬁnition, its values

obtained for the various theoretical models are useful for judging the appropriateness of

the corresponding theory.