Coburn J.W., Herdlick J.D. College Algebra: Graphs and Models
Подождите немного. Документ загружается.

e. f.
Use a calculator to evaluate each expression, rounded to
six decimal places.
31. e
1
32. e
0
33. e
2
34.
35. 36.
Graph each exponential function.
37. 38.
39. 40.
41. 42.
Solve each exponential equation and check your answer
by substituting into the original equation.
43. 44.
45. 46.
47. 48.
49. 50.
51. 52.
53. 54.
55. 56.
57. 58.
59. 60.
61. 62.
Solve the following equations. First estimate the answer by
bounding it between two integers, then solve the equation
graphically. Adjust the viewing window as needed.
63. 64.
65. 66. e
0.5x
8e
x1
9
2
x
2.1253
x
22
e
x
e
x3
1e
x2
2
3
1e
2x4
2
3
e
x5
e
2
e
x
1e
x
e2
e
x
e
3x
e
x
e
4
e
2x
e
3
e
27
2x4
9
4x
25
3x
125
x2
2
2x
1
1
32
2
x3
1
1
9
2
x5
3
3x
1
1
2
2
3x
8
x2
1
1
3
2
2x
9
x6
1
1
4
2
x
641
1
5
2
x
125
100
x2
1000
x
32
x
16
x1
9
x1
278
x2
32
81 27
x
25
x
125
144 12
x
10
x
1000
q1x2 e
x1
2p1x2 e
x2
1
s1t2e
t2
r1t2e
t
2
g1x2 e
x2
1f 1x2 e
x3
2
e
e
12
e
3.2
x
y
54321
54321
1
2
3
4
5
2
3
4
5
1
y
1
(
1, 4)
(0, 2)
x
y
5432154321
1
2
3
4
5
2
3
4
5
1
y
0
(
1, 5)
(0, 1)
Evaluate each function as indicated. Use a calculator
only as needed, rounding results to thousandths.
7. 8.
9. 10.
Graph each function using a table of values and integer
inputs between and 3. Clearly label the y-intercept
and one additional point, then state whether the
function is increasing or decreasing.
11. 12.
13. 14.
Graph each of the following functions by translating the
basic function , sketching the asymptote, and
strategically plotting a few points to round out the
graph. Clearly state the basic function and what shifts
are applied.
15. 16.
17. 18.
19. 20.
21. 22.
23. 24.
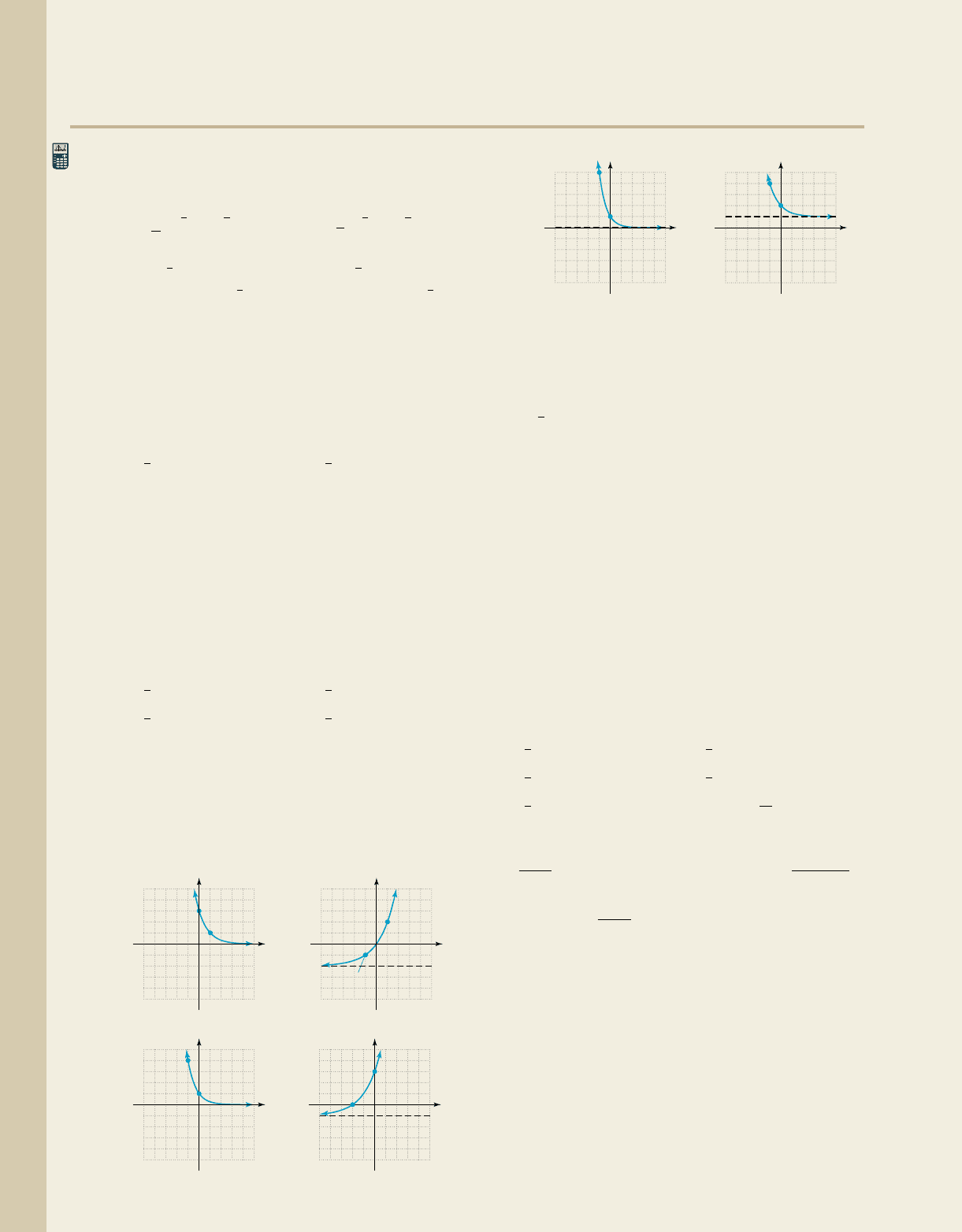
Match each exponential equation to the correct graph.
25. 26.
27. 28.
29. 30.
a. b.
c. d.
x
y
5432154321
1
2
3
4
5
2
3
4
5
1
(0, 3)
(2, 0)
y
1
x
y
54321
54321
1
2
3
4
5
2
3
4
5
1
y
0
(0, 1)
(
1, 4)
x
y
5432154321
1
2
3
4
5
2
3
4
5
1
(1, 2)
(1, 1)
y
2
x
y
y
0
54321
54321
1
2
3
4
5
2
3
4
5
1
(0, 3)
(1, 1)
y 2
x2
1y 2
x1
2
y 3
x
1y 3
x1
y 4
x
y 5
x
y 1
1
3
2
x2
y 1
1
3
2
x2
y 1
1
3
2
x
4y 1
1
3
2
x
1
y 3
x
2y 3
x
y 3
x2
y 3
x3
y 3
x
3y 3
x
2
y ⴝ b
x
y 1
1
4
2
x
y 1
1
3
2
x
y 4
x
y 3
x
ⴚ3
m 2n 2
m
3
2
,m 3,m 0,n
2
3
,n 2,n 0,
W1m2 1
4
9
2
m
;V1n2 1
1
8
2
n
;
t 25
t 13
t
5
3
,t
1
3
,t 2,t
3
2
,t
1
2
,t 2,
Q1t2 8
t
;P1t2 4
t
;
500 CHAPTER 5 Exponential and Logarithmic Functions 5–22
College Algebra G&M—
䊳
DEVELOPING YOUR SKILLS
cob19545_ch05_493-503.qxd 1/7/11 4:58 PM Page 500

䊳
WORKING WITH FORMULAS
67. The growth of bacteria: P(t) ⴝ
If the initial population of a common bacterium is
1000 and the population triples every day, its
population is given by the formula shown, where
P(t) is the total population after t days. (a) Find the
total population 12 hr, 1 day, days, and 2 days
later. (b) Do the outputs show the population is
tripling every 24 hr (1 day)? (c) Explain why this is
an increasing function. (d) Graph the function
using an appropriate scale.
1
1
2
1000
#
3
t
68. Spinners with numbers 1 to 4: P(x) ⴝ ()
x
Games that involve moving pieces around a board
using a fair spinner are fairly common. If the spinner
has the numbers 1 through 4, the probability that any
one number is spun repeatedly is given by the
formula shown, where x represents the number of
spins and P(x) represents the probability the same
number results x times. (a) What is the probability
that the first player spins a 2? (b) What is the
probability that all four players spin a 2? (c) Explain
why this is a decreasing function.
1
4
䊳
APPLICATIONS
69. Depreciation:
The financial
analyst for a large
construction firm
estimates that its
heavy equipment
loses one-fifth of
its value each year.
The current value of the equipment is then modeled
by the function where V
0
represents
the initial value, t is in years, and V(t) represents the
value after t years. (a) How much is a large
earthmover worth after 1 yr if it cost $125 thousand
new? (b) How many years does it take for the
earthmover to depreciate to a value of $64 thousand?
70. Depreciation: Photocopiers have become a critical
part of the operation of many businesses, and due
to their heavy use they can depreciate in value very
quickly. If a copier loses of its value each year,
the current value of the copier can be modeled by
the function where V
0
represents the
initial value, t is in years, and V(t) represents the
value after t yr. (a) How much is this copier worth
after one year if it cost $64 thousand new? (b) How
many years does it take for the copier to depreciate
to a value of $25 thousand?
71. Depreciation: Margaret Madison, DDS, estimates
that her dental equipment loses one-sixth of its
value each year. (a) Determine the value of an
x-ray machine after 5 yr if it cost $216 thousand
new, and (b) determine how long until the machine
is worth less than $125 thousand.
72. Exponential decay: The groundskeeper of a local
high school estimates that due to heavy usage by
the baseball and softball teams, the pitcher’s
V1t2⫽ V
0
1
5
8
2
t
,
3
8
V1t2⫽ V
0
1
4
5
2
t
,
mound loses one-fifth of
its height every month.
Use this information to
find an equation that
models this information
and then determine: (a) If
the mound was 25 cm to
begin, how long until the
height becomes less than
16 cm high (meaning it
must be entirely rebuilt)? (b) If the mound were
allowed to deteriorate further, find the height after
3 months.
73. Exponential growth: Similar to a small town
doubling in size after a discovery of gold, a
business that develops a product in high demand
has the potential for doubling its revenue each year
for a number of years. The revenue would be
modeled by the function where R
0
represents the initial revenue, and R(t) represents
the revenue after t years. (a) How much revenue is
being generated after 4 yr, if the company’s initial
revenue was $2.5 million? (b) How many years
does it take for the business to be generating
$320 million in revenue?
74. Exponential growth: If a company’s revenue
grows at a rate of 150% per year (rather than
doubling as in Exercise 73), the revenue would be
modeled by the function where R
0
represents the initial revenue, and R(t) represents
the revenue after t years. (a) How much revenue is
being generated after 3 yr, if the company’s initial
revenue was $256 thousand? (b) How long until the
business is generating $1944 thousand in revenue?
(Hint: Reduce the fraction.)
R1t2⫽ R
0
1
3
2
2
t
,
R1t2⫽ R
0
2
t
,
5–23 Section 5.2 Exponential Functions 501
College Algebra G&M—
cob19545_ch05_493-503.qxd 11/30/10 3:59 PM Page 501
Use Newton’s law of cooling to complete Exercises 75
and 76: T(x) ⫽ T
R
⫹ (T
0
⫺ T
R
)e
kx
.
75. Cold party drinks: Janae was late getting ready for
the party, and the liters of soft drinks she bought
were still at room temperature ( ) with guests
due to arrive in 15 min. If she puts these in her
freezer at , will the drinks be cold enough
( ) for her guests? Assume .
76. Warm party drinks: Newton’s law of cooling
applies equally well if the “cooling is negative,”
meaning the object is taken from a colder medium
and placed in a warmer one. If a can of soft drink is
taken from a cooler and placed in a room
where the temperature is , how long will it take
the drink to warm to ? Assume .
Photochromatic sunglasses: Sunglasses that darken in
sunlight (photochromatic sunglasses) contain millions of
molecules of a substance known as silver halide. The
molecules are transparent indoors in the absence of
ultraviolent (UV) light. Outdoors, UV light from the sun
causes the molecules to change shape, darkening the
lenses in response to the intensity of the UV light. For
certain lenses, the function models the
transparency of the lenses (as a percentage) based on a
UV index x. Find the transparency (to the nearest
percent), if the lenses are exposed to
77. sunlight with a UV index of 7 (a high exposure).
78. sunlight with a UV index of 5.5 (a moderate
exposure).
79. Given that a UV index of 11 is very high and most
individuals should stay indoors, what is the minimum
transparency percentage for these lenses?
T1x2 0.85
x
k 0.03165°F
75°F
35°F
k 0.03135°F
10°F
73°F
80. Use a trial-and-error process and a graphing
calculator to determine the UV index when the
lenses are 50% transparent.
Modeling inflation: Assuming the rate of inflation is 5%
per year, the predicted price of an item can be modeled
by the function where P
0
represents the
initial price of the item and t is in years. Use this
information to solve Exercises 81 and 82.
81. What will the price of a new car be in the year
2015, if it cost $20,000 in the year 2010?
82. What will the price of a gallon of milk be in the
year 2015, if it cost $3.95 in the year 2010? Round
to the nearest cent.
Modeling radioactive decay: The half-life of a
radioactive substance is the time required for half an
initial amount of the substance to disappear through
decay. The amount of the substance remaining is given
by the formula where h is the half-life,
t represents the elapsed time, and Q(t) represents the
amount that remains (t and h must have the same unit
of time). Use this information to solve Exercises 83
and 84.
83. Some isotopes of the substance known as thorium
have a half-life of only 8 min. (a) If 64 grams are
initially present, how many grams (g) of the
substance remain after 24 min? (b) How many
minutes until only 1 gram (g) of the substance
remains?
84. Some isotopes of sodium have a half-life of about
16 hr. (a) If 128 g are initially present, how many
grams of the substance remain after 2 days (48 hr)?
(b) How many hours until only 1 g of the substance
remains?
Q1t2 Q
0
1
1
2
2
t
h
,
P1t2 P
0
11.052
t
,
502 CHAPTER 5 Exponential and Logarithmic Functions 5–24
College Algebra G&M—
䊳
EXTENDING THE CONCEPT
85. If , what is the value of ?10
x
10
2x
25
87. If , what is the value of ?3
x1
3
0.5x
5
89. The formula gives the probability that “x” number of flips result in heads (or tails). First determine the
probability that 20 flips results in 20 heads in a row. Then use the Internet or some other resource to determine the
probability of winning a state lottery (expressed as a decimal). Which has the greater probability? Were you surprised?
f
1x2 1
1
2
2
x
88. If , what is the value of ?a
1
2
b
x
a
1
2
b
x1
1
3
86. If , what is the value of 5
2x
?5
3x
27
The growth rate constant that governs an exponential function was introduced on page 495.
90. In later sections, we will easily be able to find the growth constant k for Goldsboro, where . For
now we’ll approximate its value using the rate of change formula on a very small interval of the domain. From
the definition of an exponential function, . Since k is constant, we can choose any value of t, say
. For , we have . (a) Use the equation shown to solve for
k (round to thousandths). (b) Show that k is constant by completing the same exercise for and .
(c) Verify that and give approximately the same results.P1t2 1000e
kt
P1t2 1000
#
2
t
t 6t 2
1000
#
2
40.0001
1000
#
2
4
0.0001
k
#
P142h 0.0001t 4
¢P
¢t
kP1t2
P1t2 1000
#
2
t
cob19545_ch05_493-503.qxd 11/27/10 12:27 AM Page 502

5–25 Section 5.3 Logarithms and Logarithmic Functions 503
College Algebra G&M—
䊳
MAINTAINING YOUR SKILLS
91. (1.3) Given determine:
f(a),
93. (R.6) Solve the following equations:
a.
b.
9
x 3
3
12
x 3
21x 3
7 21
f
1a h2f 1
1
3
2,f 112,
f
1x2 2x
2
3x,
92. (2.2) Graph using a shift of a
basic function. Then state the domain and range of g.
94. (R.3) Identify each formula:
a.
b.
c. lwh
d. a
2
b
2
c
2
1
2
bh
4
3
r
3
g1x2 1x 2 1
A transcendental function is one whose solutions are beyond or transcend the
methods applied to polynomial functions. The exponential function and its inverse,
called the logarithmic function, are transcendental functions. In this section, we’ll use
the concept of an inverse to develop an understanding of the logarithmic function,
which has numerous applications that include measuring pH levels, sound and earth-
quake intensities, barometric pressure, and other natural phenomena.
A. Exponential Equations and Logarithmic Form
While exponential functions have a large number of significant applications, we can’t
appreciate their full value until we develop the inverse function. Without it, we’re
unable to solve all but the simplest equations, of the type encountered in Section 5.2.
Using the fact that is one-to-one, we have the following:
1. The function must exist.
2. We can graph by interchanging the x- and y-coordinates of points from f(x).
3. The domain of f(x) will become the range of
4. The range of f(x) will become the domain of .
5. The graph of will be a reflection of f(x) across the line
Table 5.6 contains selected values for . The values for in Table 5.7
were found by interchanging x- and y-coordinates. Both functions were then graphed
using these values.
f
1
1x2f 1x2 2
x
y x.f
1
1x2
f
1
1x2
f
1
1x2.
f
1
1x2
f
1
1x2
f
1x2 b
x
LEARNING OBJECTIVES
In Section 5.3 you will see
how we can:
A. Write exponential
equations in logarithmic
form
B. Find common logarithms
and natural logarithms
C. Graph logarithmic
functions
D. Find the domain of a
logarithmic function
E. Solve applications of
logarithmic functions
5.3 Logarithms and Logarithmic Functions
x y
0 1
1 2
2 4
3 8
1
2
1
1
4
2
1
8
3
Table 5.6
f1x2: y ⴝ 2
x
x (x)
1 0
2 1
4 2
8 3
1
1
2
2
1
4
3
1
8
yⴝ f
ⴚ1
Table 5.7
f
ⴚ1
1x2: x ⴝ 2
y
cob19545_ch05_493-503.qxd 9/2/10 9:29 PM Page 503
504 CHAPTER 5 Exponential and Logarithmic Functions 5–26
College Algebra Graphs & Models—
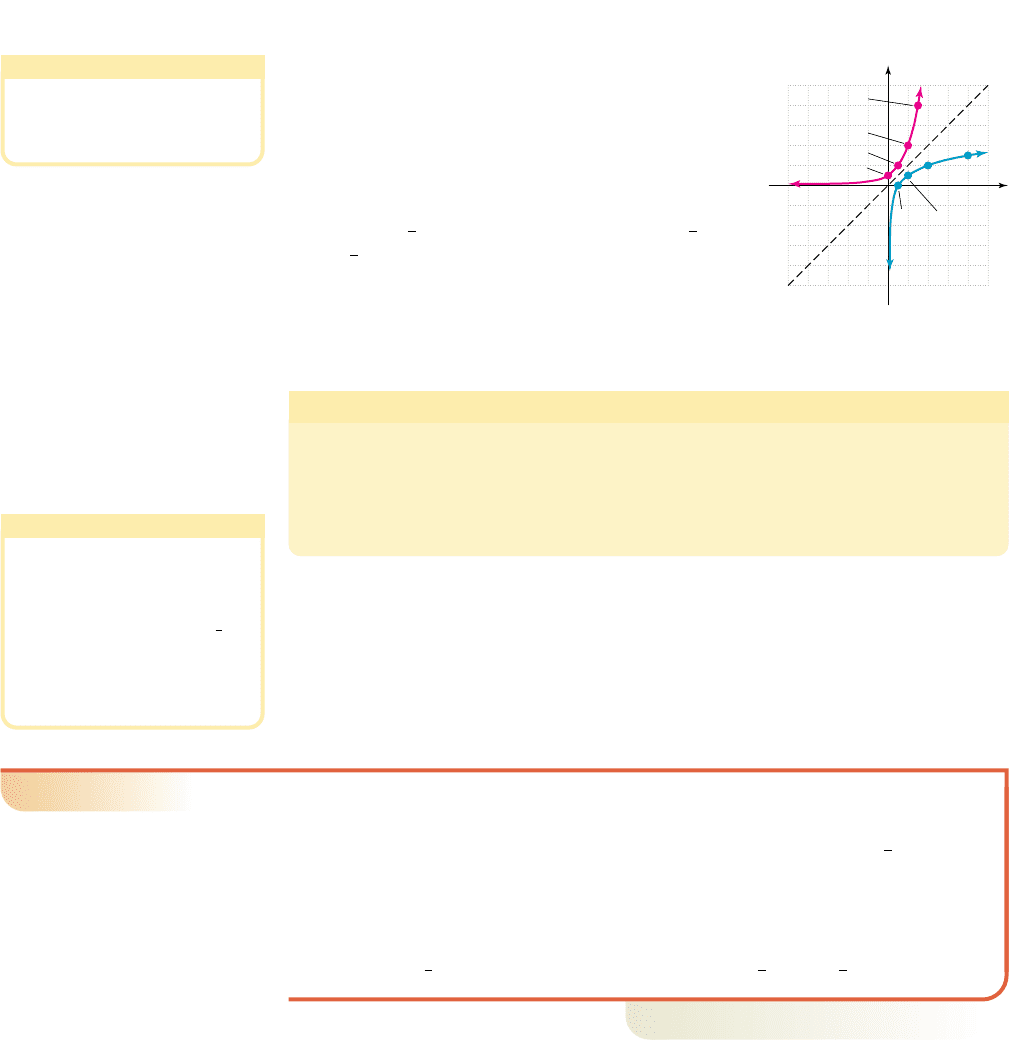
The interchange of x and y and the graphs in Figure 5.23 show that has an
x-intercept of (1, 0), a vertical asymptote at a domain of and a range
of . To find an equation for we’ll attempt to use the algebraic
approach employed previously. For
1. use y instead of f(x): 2. interchange x and y:
At this point we have an implicit equation for the
inverse function, but no algebraic operations that enable
us to solve explicitly for y in terms of x. Instead, we write
in function form by noting that “y is the exponent
that goes on base 2 to obtain x.” In the language of math-
ematics, this phrase is represented by and is
called a logarithmic function with base 2. For example,
from Table 5.7 we have: is the exponent that goes on
base 2 to get , and this is written since
✓. For is the
inverse function, and is read, “y is the logarithm base b
of x.” For this new function, we must always keep in
mind what y represents—y is an exponent. In fact, y is the exponent that goes on base
b to obtain x: .y log
b
x
y b
x
, x b
y
S y log
b
x2
3
1
8
3 log
2
1
8
1
8
3
y log
2
x
x 2
y
x 2
y
.y 2
x
.
f
1x2 2
x
,
f
1
1x2,y 僆 1q, q2
x 僆 10, q2,x 0,
f
1
1x2
WORTHY OF NOTE
The word logarithm was coined by
John Napier in 1614, and loosely
translated from its Greek origins
means “to reason with numbers.”
WORTHY OF NOTE
Since base-10 logarithms occur
so frequently, we usually use only
log x to represent log
10
x. We do
something similar with square
roots. Technically, the “square
root of x” should be written
However, square roots are so
common we often leave off the two,
assuming that if no index is written,
an index of two is intended.
2
2
x.
Logarithmic Functions
For positive numbers x and b, with ,
The function is a logarithmic function with base b. The expression log
b
x
is simply called a logarithm, and represents the exponent on b that yields x.
Finally, note the equations and are equivalent. We say that
is the exponential form of the equation, whereas is written in loga-
rithmic form. Of all possible bases for log
b
x, the most common are base 10 (likely due
to our base-10 number system), and base e (due to the advantages it offers in advanced
courses). The expression log
10
x is called a common logarithm, and we simply write
log x for log
10
x. The expression log
e
x is called a natural logarithm, and is written in
abbreviated form as ln x.
EXAMPLE 1
䊳
Converting from Logarithmic Form to Exponential Form
Write each equation in words, then in exponential form.
a. b. c. d.
Solution
䊳
a. is the exponent on base 2 for 8: .
b. is the exponent on base 10 for 10: .
c. is the exponent on base e for 1: .
d. is the exponent on base 3 for .
Now try Exercises 7 through 22
䊳
To convert from exponential form to logarithmic form, note the exponent on the
base and read from there. For , “3 is the exponent that goes on base 5 for
125,” or 3 is the logarithm base 5 of 125: .3 log
5
125
5
3
125
1
9
: 3
2
1
9
2 log
3
1
1
9
2 S 2
e
0
10 ln 1 S 0
10
1
101 log 10 S 1
2
3
83 log
2
8 S 3
2 log
3
1
1
9
20 ln 11 log 103 log
2
8
y log
b
xx b
y
y log
b
xx b
y
f 1x2 log
b
x
y log
b
x if and only if x b
y
b 1
x
y
y 2
x
x 2
y
y x
108642108 6 4 2
2
4
6
8
10
4
6
8
10
2
(0, 1)
(1, 0)
(4, 2)
(8, 3)
(2, 1)
(1, 2)
(2, 4)
(3, 8)
Figure 5.23
cob19545_ch05_504-516.qxd 11/27/10 12:30 AM Page 504
EXAMPLE 2
䊳
Converting from Exponential Form to Logarithmic Form
Write each equation in words, then in logarithmic form.
a. b. c. d.
Solution
䊳
a. 3 is the exponent on base 10 for 1000, or
3 is the logarithm base 10 of 1000: .
b. 1 is the exponent on base 2 for , or
is the logarithm base 2 of .
c. 2 is the exponent on base e for 7.389, or
2 is the logarithm base e of 7.389: .
d. is the exponent on base 9 for 27, or
is the logarithm base 9 of 27: .
Now try Exercises 23 through 38
䊳
B. Finding Common Logarithms and Natural Logarithms
Some logarithms are easy to evaluate. For example, since , and
since . But what about the expressions log 850 and ln 4?
Because logarithmic functions are continuous on their domains, a value exists for log 850
and the equation must have a solution. Further, the inequalities
tell us that log 850 must be between 2 and 3. Fortunately, modern calculators can
compute base-10 and base-e logarithms instantly, often with nine-decimal-place
accuracy. For log 850, press , then input 850 and press . The display should
read 2.929418926. We can also use the calculator to verify (see
Figure 5.24). For ln 4, press the key, then input 4 and press to obtain
1.386294361. Figure 5.25 verifies that .e
1.386294361
4
ENTER
LN
10
2.929418926
850
ENTER
LOG
2 6 log 850 6 3
log 100 6 log 850 6 log 1000
10
x
850
10
2
1
100
log
1
100
2
10
2
100log 100 2
3
2
log
9
27
3
2
3
2
9
3
2
27 S
2 ⬇ ln 7.389
e
2
⬇ 7.389 S
1
2
: 1 log
2
1
1
2
21
1
2
2
1
1
2
S
3 log 1000
10
3
1000 S
9
3
2
27e
2
⬇ 7.3892
1
1
2
10
3
1000
5–27 Section 5.3 Logarithms and Logarithmic Functions 505
College Algebra Graphs & Models—
A. You’ve just seen how
we can write exponential
equations in logarithmic form
Figure 5.24 Figure 5.25
EXAMPLE 3
䊳
Finding the Value of a Logarithm
Determine the value of each logarithm without using a calculator:
a. log
2
8 b. c. ln e d.
Solution
䊳
a. log
2
8 represents the exponent on 2 for 8: , since .
b. represents the exponent on 5 for , since .
c. ln e represents the exponent on e for e: , since .
d. represents the exponent on 10 for ,
since
Now try Exercises 39 through 50
䊳
10
1
2
110.
110
: log 110
1
2
log 110
e
1
eln e 1
5
2
1
25
1
25
: log
5
1
25
2log
5
1
1
25
2
2
3
8log
2
8 3
log 110
log
5
1
1
25
2
cob19545_ch05_504-516.qxd 9/2/10 9:31 PM Page 505
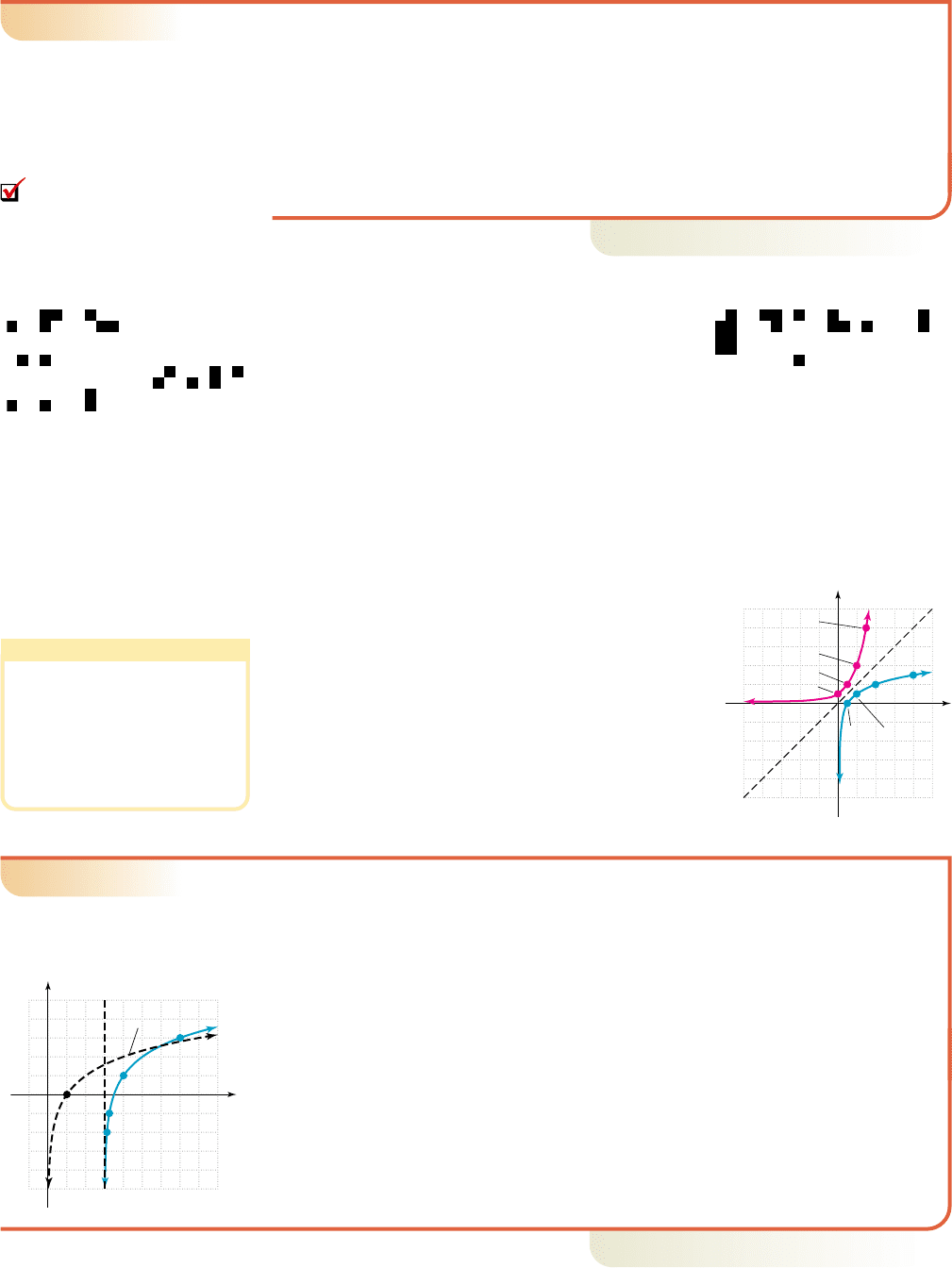
4
4
4
x
y
(4, 1)
(7, 3)
y log
2
(x 3) 1
y log
2
x
x 3
506 CHAPTER 5 Exponential and Logarithmic Functions 5–28
College Algebra Graphs & Models—
EXAMPLE 4
䊳
Using a Calculator to Find Logarithms
Use a calculator to evaluate each logarithmic expression. Verify the result.
a. log 1857 b. log 0.258 c. ln 3.592
Solution
䊳
a. c.
✓✓
b.
✓
Now try Exercises 51 through 58
䊳
Finally, note that if , the value of log x is
greater than 1, but if the value of log x is
less than 1. Also, if , the expression log x does
not represent a real number (the domain of
does not include negative numbers). See Figures 5.26
and 5.27.
y log
b
x
x 6 0
0 6 x 6 10
x 7 10
10
0.588380294
0.258
log 0.258 0.588380294,
e
1.27870915
⬇ 3.59210
3.268811904
1857
ln 3.592 ⬇ 1.27870915log 1857 3.268811904,
B. You’ve just seen how we
can find common logarithms
and natural logarithms
Figure 5.26 Figure 5.27
C. Graphing Logarithmic Functions
For convenience and ease of calculation, our first ex-
amples of logarithmic graphs are done using base-2
logarithms. However, the basic shape of a logarithmic
graph remains unchanged regardless of the base used,
and transformations can be applied to for
any value of b. For , a contin-
ues to govern stretches, compressions, and vertical
reflections, the graph will shift horizontally h units
opposite the sign, and shift k units vertically in the
same direction as the sign. Our earlier graph of
was completed using as the inverse
function for (Figure 5.23). For reference, the
graph is repeated in Figure 5.28.
EXAMPLE 5
䊳
Graphing Logarithmic Functions Using Transformations
Graph using transformations of (not by simply
plotting points). Clearly state what transformations are applied.
Solution
䊳
The graph of f is the same as that of , shifted 3 units right and 1 unit up.
The vertical asymptote will be at and the point (1, 0) from the basic graph
becomes . Knowing the graph’s basic shape, we compute
one additional point using :
The point (7, 3) is on the graph, shown in the figure.
Now try Exercises 59 through 72
䊳
3
2 1
log
2
4 1
f
172 log
2
17 32 1
x 7
11 3, 0 12 14, 12
x 3
y log
2
x
y log
2
xf 1x2 log
2
1x 32 1
y 2
x
x 2
y
y log
2
x
y a log1x h2 k
y log
b
1x2
x
y
y 2
x
x 2
y
y x
108642108 6 4 2
2
4
6
8
10
4
6
8
10
2
(0, 1)
(1, 0)
(4, 2)
(8, 3)
(2, 1)
(1, 2)
(2, 4)
(3, 8)
Figure 5.28
WORTHY OF NOTE
As with the basic graphs we
studied in Section 2.2, logarithmic
graphs maintain the same
characteristics when transforma-
tions are applied, and these graphs
should be added to your collection
of basic functions, ready for recall
or analysis as the situation requires.
cob19545_ch05_504-516.qxd 9/2/10 9:31 PM Page 506
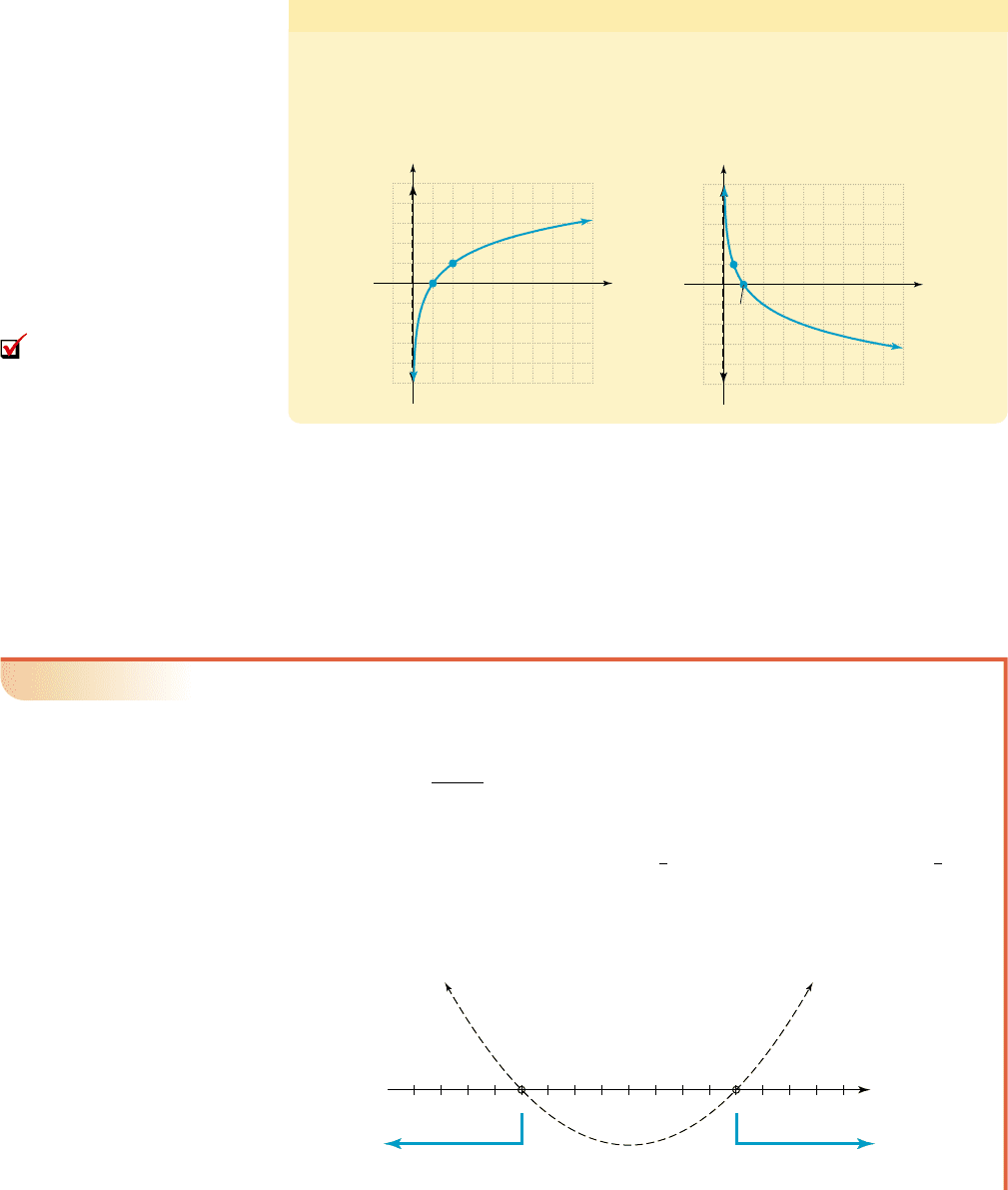
5–29 Section 5.3 Logarithms and Logarithmic Functions 507
College Algebra Graphs & Models—
As with the exponential functions, much can be learned from graphs of logarith-
mic functions and a summary of important characteristics is given here.
and
• one-to-one function
• domain:
• increasing if b 7 1
x 僆 10, q2
b ⴝ 1f 1x2ⴝ log
b
x, b ⬎ 0
• x-intercept (1, 0)
• range:
• decreasing if 0 6 b 6 1
y 僆 ⺢
• asymptotic to the y-axis (the line )x 0
C. You’ve just seen how
we can graph logarithmic
functions
48
4
4
x
y
(1, 0)
(b, 1)
f(x) log
b
x
b 1
48
4
4
x
y
(1, 0)
(b, 1)
f(x) log
b
x
0 b 1, b 苷 1
D. Finding the Domain of a Logarithmic Function
Examples 5 and 6 illustrate how the domain of a logarithmic function can change when
certain transformations are applied. Since the domain consists of positive real num-
bers, the argument of a logarithmic function must be greater than zero. This means
finding the domain often consists of solving various inequalities, which can be done
using the skills acquired in Sections 3.2 and 4.6.
EXAMPLE 6
䊳
Finding the Domain of a Logarithmic Function
Determine the domain of each function.
a. b.
c. d.
Solution
䊳
Begin by writing the argument of each function as a “greater than” inequality.
a. Solving for x gives , and the domain of p is .
b. For , we note is a parabola, opening upward, with
zeroes at and (see Figure 5.29). This means will be
positive for and . The domain of q is .x 僆 1q, 02 ´ 12, q2x 7 2x 6 0
x
2
2xx 2x 0
y x
2
2xx
2
2x 7 0
x 僆 1
3
2
, q2x 7
3
2
2x 3 7 0
f
1x2 ln
冟
x 2
冟
r 1x2 log a
3 x
x 3
b
q1x2 log
5
1x
2
2x2p1x2 log
2
12x 32
When x 0,
the graph is above
the x-axis
y 0
When x 2,
the graph is above
the x-axis
y 0
Graph is below the x-axis
for 0 x 2.
a 0
x
2 3
101
Figure 5.29
cob19545_ch05_504-516.qxd 9/2/10 9:31 PM Page 507
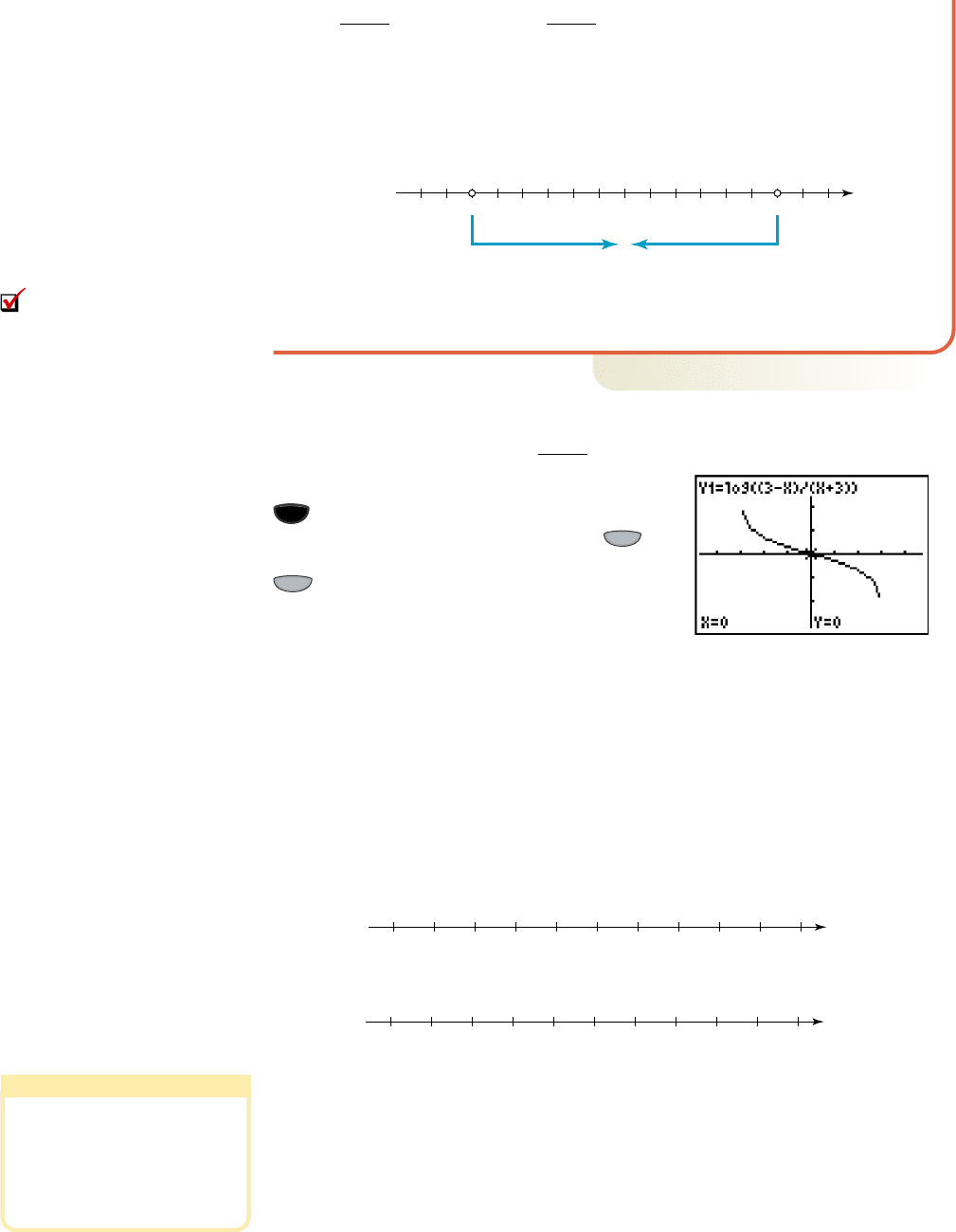
508 CHAPTER 5 Exponential and Logarithmic Functions 5–30
College Algebra Graphs & Models—
c. For , we note has a zero at , with a vertical
asymptote at and here we opt to use the interval test method to solve the
inequality. Outputs are positive when (see Figure 5.30), so y is positive in
the interval ( , 3) and negative elsewhere. The domain of r is .x 僆 13, 323
x 0
x 3
x 3y
3 x
x 3
3 x
x 3
7 0
d. For , we note is the graph of shifted 2 units
right, with its vertex at (2, 0). The graph is positive for all x, except at .
The domain of f is .
Now try Exercises 73 through 78
䊳
x 僆 1q, 22 ´ 12, q2
x 2
y
冟
x
冟
y
冟
x 2
冟冟
x 2
冟
7 0
D. You’ve just seen how
we can find the domain of a
logarithmic function
Figure 5.30
When x 3, y 0 When x 3, y 0
When 3 x 3,
y 0
(interval test)
x
234
4 3 2 101
The domain for from
Example 7c can also be confirmed using the
key on a graphing calculator. Use this
key to enter the equation as Y
1
on the
screen, then graph the function using the
4:ZDecimal option. Both the graph (Fig-
ure 5.31) and TABLE feature help to confirm
the domain is .x 僆 13, 32
ZOOM
Y=
LOG
r 1x2 log a
3 x
x 3
b
3.1
3.1
4.7 4.7
Figure 5.31
0123456789
years
10
0 (10
1
) (10
2
) (10
3
) (10
4
) (10
5
) (10
6
) (10
7
) (10
8
) (10
9
) (10
10
)
0123456789
years
10
Figure 5.32
Figure 5.33
E. Applications of Logarithms
The use of logarithmic scales as a tool of measurement is primarily due to the range of
values for the phenomenon being measured. For instance, time is generally measured on
a linear scale, and for short periods a linear scale is appropriate. For the time line in Fig-
ure 5.32, each tick-mark represents 1 unit, and the time line can display a period of 10 yr.
However, the scale would be useless in a study of geology or the age of the universe. If
we scale the number line logarithmically, each tick-mark represents a power of 10 (Fig-
ure 5.33) and a scale of the same length can now display a time period of 10 billion years.
WORTHY OF NOTE
The decibel (dB) is the reference
unit for sound, and is based on the
faintest sound a person can hear,
called the threshold of audibility. It
is a base-10 logarithmic scale,
meaning a sound 10 times more
intense is one bel louder.
In much the same way, logarithmic measures are needed in a study of sound and
earthquake intensity, as the scream of a jet engine is over 1 billion times more intense
than the threshold of hearing, and the most destructive earthquakes are billions of
times stronger than the slightest earth movement that can be felt. Similar ranges exist
in the measurement of light, acidity, and voltage. Figures 5.34 and 5.35 show logarith-
mic scales for measuring sound in decibels ( ) and earthquake
intensity in Richter values (or magnitudes).
1 bel 10 decibels
cob19545_ch05_504-516.qxd 11/27/10 12:31 AM Page 508
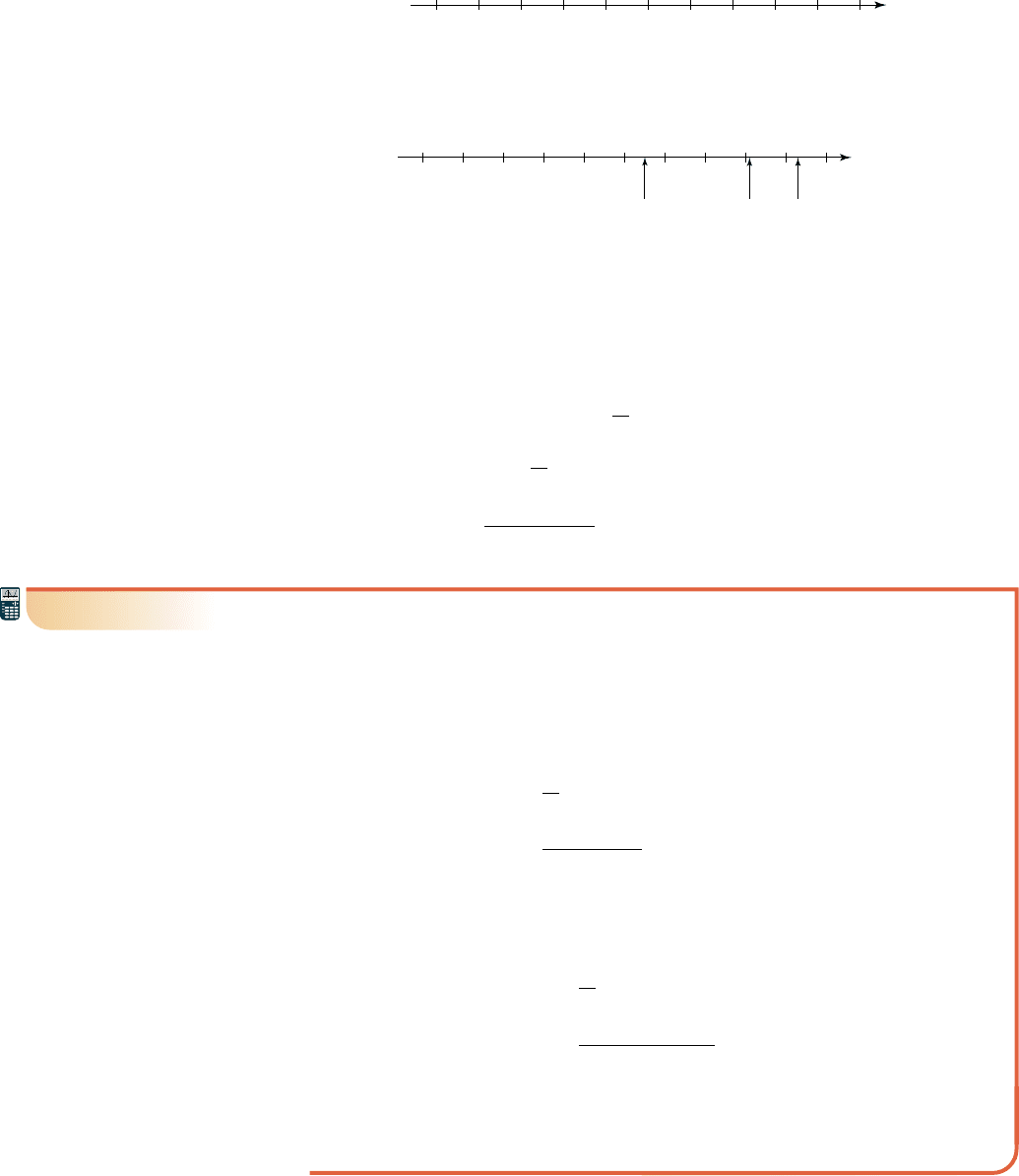
5–31 Section 5.3 Logarithms and Logarithmic Functions 509
College Algebra Graphs & Models—
Figure 5.34
threshold
soft whisper
hum of an
appliance
conversations
city traffic
loud motorcycle
jet fly-over
0123456789
bels
10
1992
San Jose,
CA (5.5)
1906
San Fran,
CA (8.1)
2004
Indian
Ocean (9.3)
threshold
barely felt
noticeable
shaking
destructive
devastating
catastrophic
never recorded
0123456789
magnitudes
10
Figure 5.35
The slightest earth movement perceptible is called the reference intensity I
0
, with the
intensity Iof stronger earthquakes expressed as a multiple of I
0
. The earthquake that struck
Haiti in January of 2010 was measured at over 10,500,000 times this reference intensity, or
. To find the Richter value (magnitude) of this earthquake, we simply
take the base-10 logarithm of the ratio to express these values on a logarithmic scale. In
function form, , and we find that the Haitian earthquake had a magnitude
of just over 7.0:
EXAMPLE 7A
䊳
Finding the Magnitude of an Earthquake
Find the magnitude of the earthquakes (rounded to hundredths) with the intensities given.
a. Eureka earthquake; January 9, 2010, near Humboldt county, California:
.
b. Sumatra-Andaman earthquake; December 26, 2004, near the west coast of
Sumatra, Indonesia: .
Solution
䊳
a. magnitude equation
substitute 3,162,000
I
0
for
I
simplify
result
The earthquake had a magnitude of about 6.5.
b.
magnitude equation
substitute 1,995,260,000
I
0
for
I
simplify
result
The earthquake had a magnitude of about 9.3.
⬇ 9.3
log 1,995,260,000
M11,995,260,000I
0
2 log a
1,995,260,000I
0
I
0
b
M1I2 log
a
I
I
0
b
⬇ 6.5
log 3,162,000
M13,162,000I
0
2 log a
3,162,000I
0
I
0
b
M1I2 log
a
I
I
0
b
I 1,995,260,000I
0
I 3,162,000I
0
log a
10,500,000I
0
I
0
b log 110,500,0002⬇ 7.0.
M1I2 log
a
I
I
0
b
I
I
0
I 10,5000,000I
0
cob19545_ch05_504-516.qxd 9/2/10 9:32 PM Page 509
