Coburn J.W., Herdlick J.D. College Algebra: Graphs and Models
Подождите немного. Документ загружается.

ISBN: 0-07-351954-5
Author: John W. Coburn
Title: College Algebra, 3e
Front endsheets
Color: 5
Pages: 2, 3
▼
13 ⬇ 1.732112 ⬇ 1.4142e ⬇ 2.7183 ⬇ 3.1416
Special Constants
▼
Special Products
1a b2
3
a
3
3a
2
b 3ab
2
b
3
1a b2
3
a
3
3a
2
b 3ab
2
b
3
1a b2
2
a
2
2ab b
2
1a b2
2
a
2
2ab b
2
1a b21a b2 a
2
b
2
1x a21x b2 x
2
1a b2x ab
▼
Special Factorizations
a
3
b
3
1a b21a
2
ab b
2
2a
3
b
3
1a b21a
2
ab b
2
2
a
2
2ab b
2
1a b2
2
a
2
2ab b
2
1a b2
2
a
2
b
2
1a b21a b2x
2
1a b2x ab 1x a21x b2
▼
Formulas from Plane Geometry: P S perimeter, C S circumference, A S area
Rectangle Square Regular Polygon
A
a
2
P
P ns
A s
2
P 4s
A lw
P 2l 2w
Parallelogram Trapezoid Triangle
A
1
2
bhA
h
2
1a b2
A bh
Triangle
Sum of angles
Right Triangle
Pythagorean Theorem
Circle
C 2r d
A r
2
a
2
b
2
c
2
A B C 180°
Ellipse Right Parabolic Segment
A
4
3
ab
C ⬇ 221a
2
b
2
2
A ab
▼
Formulas from Solid Geometry: S S surface area, V S volume
Rectangular Solid Cube Right Circular Cylinder
S 2r1r h2
V r
2
h
S 6s
2
V s
3
S 21LW LH WH2
V LWH
Right Circular Cone Right Square Pyramid Sphere
S 4r
2
V
4
3
r
3
S b
2
b2b
2
4h
2
V
1
3
b
2
h
S r1r s2
V
1
3
r
2
h
w
l
s
s
a
b
h
b
h
a
b
h
a
b
c
A
B
C
r
a
b
a
b
▼
Distance between P
1
and P
2
Slope of Line Containing P
1
and P
2
m
¢y
¢x
y
2
y
1
x
2
x
1
d 21x
2
x
1
2
2
1y
2
y
1
2
2
Formulas from Analytical Geometry: P
1
S (x
1
, y
1
), P
2
S (x
2
, y
2
)
Equation of Line Containing P
1
and P
2
Point-Slope Form
Equation of Line Containing P
1
and P
2
Slope-Intercept Form (slope m, y-intercept b)
where b y
1
mx
1
y mx b,y y
1
m1x x
1
2
Parallel Lines
Slopes Are Equal:
Perpendicular Lines
Slopes Have a Product of m
1
m
2
11:m
1
m
2
Intersecting Lines
Slopes Are Unequal:
Dependent (Coincident) Lines
Slopes and y-Intercepts Are Equal: b
1
b
2
m
1
m
2
,m
1
m
2
Interest Compounded n Times per Year Interest Compounded Continuously
A Pe
rt
A Pa1
r
n
b
nt
Accumulated Value of an Annuity Payments Required to Accumulate Amount A
P
AR
11 R2
nt
1
A
P
R
冤11 R2
nt
1冥
Arithmetic Sequences Geometric Sequences
冟
r
冟
6 1S
q
a
1
1 r
;
S
n
a
1
a
1
r
n
1 r
a
3
a
1
r
2
, ... , a
n
a
1
r
n 1
a
2
a
1
r,a
1
,
S
n
n
2
冤2a
1
1n 12d冥
S
n
n
2
1a
1
a
n
2
a
3
a
1
2d, ... , a
n
a
1
1n 12da
2
a
1
d,a
1
,
▼
Logarithms and Logarithmic Properties
log
b
M
P
P
#
log
b
Mlog
b
a
M
N
b log
b
M log
b
Nlog
b
1MN 2 log
b
M log
b
N
log
c
x
log
b
x
log
b
c
b
log
b
x
xlog
b
b
x
x
log
b
1 0log
b
b 1y log
b
x 3 b
y
x
▼
▼
▼
Applications of Exponentials and Logarithms
Sequences and Series:
r S common ratiod S common difference,S
n
S sum of n terms,a
n
S nth term,a
1
S 1st term,
Binomial Theorem
a
n
k
b
n!
k!1n k2!
0! 1n! n1n 121n 22
###
132122112;
1a b2
n
a
n
0
ba
n
b
0
a
n
1
ba
n 1
b
1
a
n
2
ba
n 2
b
2
###
a
n
n 1
b a
1
b
n 1
a
n
n
b a
0
b
n
t S time in yearsR S interest rate per time period a
r
n
br S interest rate per year
n S compounding periods/yearP S periodic paymentP S initial deposit,A S amount accumulated
H
W
L
s
h
r
h
r
h
b
r
cob19545_es.indd Page Sec1:2 28/01/11 3:41 PM s-60usercob19545_es.indd Page Sec1:2 28/01/11 3:41 PM s-60user /Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles/Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles
▼
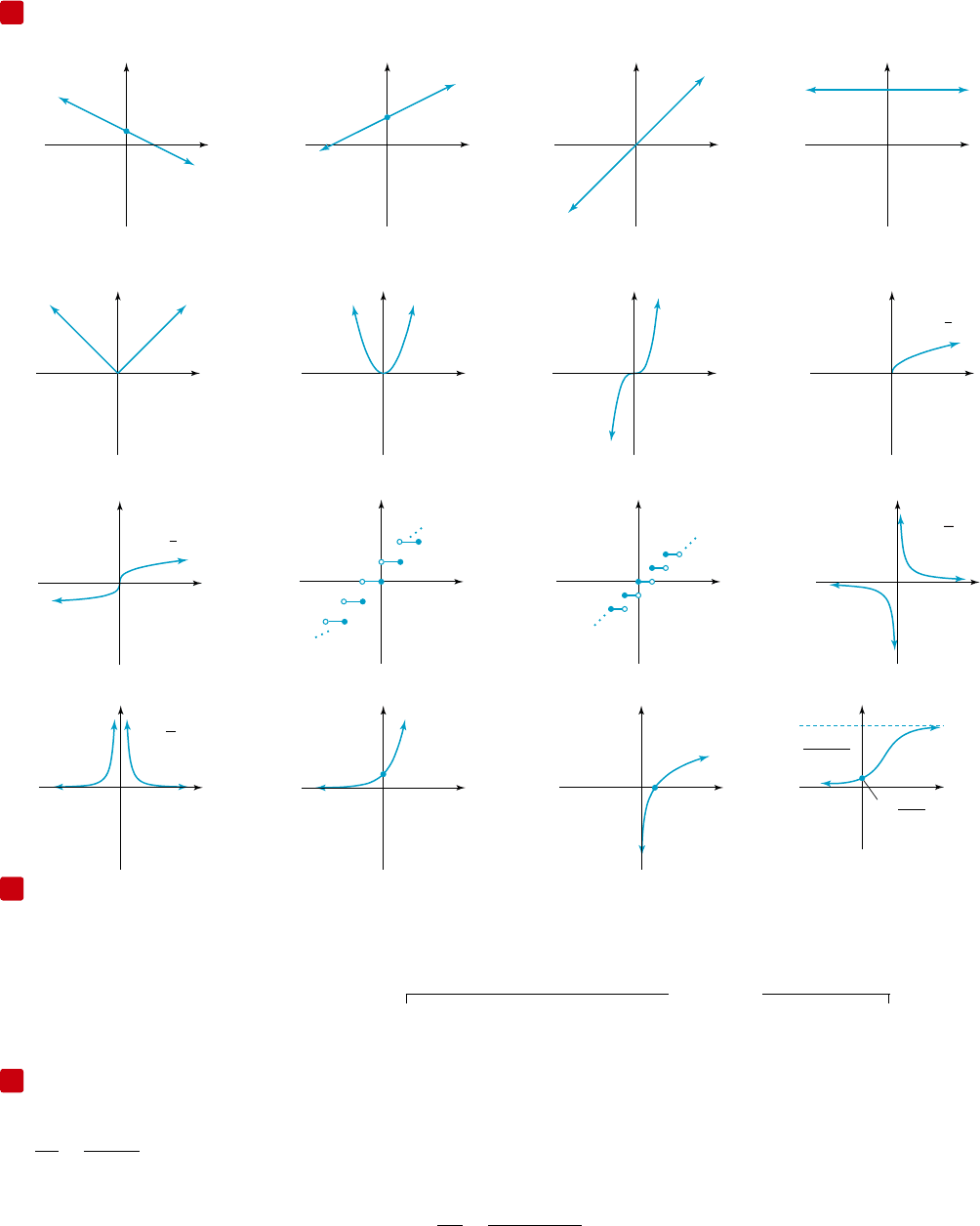
The Toolbox and Other Functions
▼
Transformations of Basic Graphs
Given Function
y f 1x2
Transformation of Given Function
y a f 1x h2 k
vertical reflections
vertical stretches/compressions
horizontal shift h units,
opposite direction of sign
vertical shift k units,
same direction as sign
S
S
S
▼
Average Rate of Change of f(x)
For linear function models, the average rate of change on the interval is constant, and given by the slope formula:
The average rate of change for other function models is nonconstant. By writing the slope formula in function form
using and we can compute the average rate of change of other functions on this interval:
⌬y
⌬x
ⴝ
f(x
2
) ⴚ f(x
1
)
x
2
ⴚ x
1
y
2
f 1x
2
2,y
1
f 1x
1
2
¢y
¢x
y
2
y
1
x
2
x
1
.
3x
1
, x
2
4
linear linear identity constant
absolute value squaring cubing square root
cube root ceiling function floor function reciprocal
reciprocal square exponential logarithmic logistic
y
mx b
(0, b)
x
m 0, b 0
y
(0, b)
m 0, b 0
x
y
y mx b
m 1, b 0
x
y
y x
m 0, b 0
x
y
y b
x
y
x
y
x
y x
2
y
x
y x
3
y
x
y
y
兹
x
y
兹
x
y
x
3
y x
x
y
y x
y
x
21
y
y
x
x
1
y
x
2
1
x
y
y b
x
(b 0)
x
1
y
y log
b
x
(b 0)
1
x
y
y
y
c
1 ae
bx
c
(
0,
)
1 a
c
y
x
ISBN: 0-07-351954-5
Author: John W. Coburn
Title: College Algebra, 3e
Front endsheets
Color: 5
Pages: 6, 7
▼
Quick-Counting and Probability
Fundamental Counting Principle: Given an experiment with two tasks completed in sequence, if the first can be
completed in m ways and the second in n ways, the experiment can be completed in ways.
Permutations—Order Is a Consideration: (Al, Bo, Ray) and (Ray, Bo, Al) finish the race in a different order.
The permutations of r objects selected from a set of n (unique) objects is given by
Combinations—Order Is Not a Consideration: (Al, Bo, Ray) and (Ray, Bo, Al) form the same committee.
The combinations of r objects selected from a set of n (unique) objects is given by
Basic Probability: Given S is a sample space of equally likely events and E is an event defined relative to S.
The probability of E is where and represent the number of elements in each.
For any event and P1E
1
2 P1~E
1
2 1.E
1
: 0 P1E
1
2 1
n1S2n1E2P(E) ⴝ
n(E)
n(S)
,
n
C
r
ⴝ
n!
r!(n ⴚ r)!
.
n
P
r
ⴝ
n!
(n ⴚ r)!
.
m ⴛ n
Probability of E
1
and E
2
Probability of E
1
or E
2
P1E
1
´ E
2
2 P1E
1
2 P1E
2
2 P1E
1
傽 E
2
2
1E
1
, E
2
independent2
P1E
1
傽 E
2
2 P1E
1
2P1E
2
2
▼
Conic Sections
r
r
h
k
(x, y)
x
2
y
2
r
2
(x h)
2
(y k)
2
r
2
(h, k)
(0, 0)
x
y
central
circle
circle with center
at (h, k)
x
y
ellipse with cente
r
at (h, k), a b
central
ellipse
If a b, the ellipse
is oriented vertically.
(h, k b)
(h, k b)
(h a, k)
(h a, k)
(0, b)
(h, k)
(a, 0)
(c, 0) (c, 0)
(0, b)
(a, 0)
k
h
c
2
|a
2
b
2
|
x
2
a
2
y
2
b
2
1
a
2
b
2
(x h)
2
1
(y k)
2
x
y
hyperbola with cente
r
at (h, k)
central
hyperbola
If term containing y leads, the
hyperbola is oriented vertically.
x
2
a
2
(h, k)
(c, 0)
(a, 0)
(c, 0)
(a, 0)
k
h
y
2
b
2
1
a
2
b
2
(x h)
2
1
(y k)
2
c
2
a
2
b
2
x
y
(0, p)
y
p
p 0
x
2
4py
vertical parabola
focus (0, p)
directrix y p
x
y
y
2
4px
horizontal parabola
focus (p, 0)
directrix x p
(p, 0)
x p
p 0
cob19545_es.indd Page Sec1:3 28/01/11 3:42 PM s-60usercob19545_es.indd Page Sec1:3 28/01/11 3:42 PM s-60user /Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles/Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles
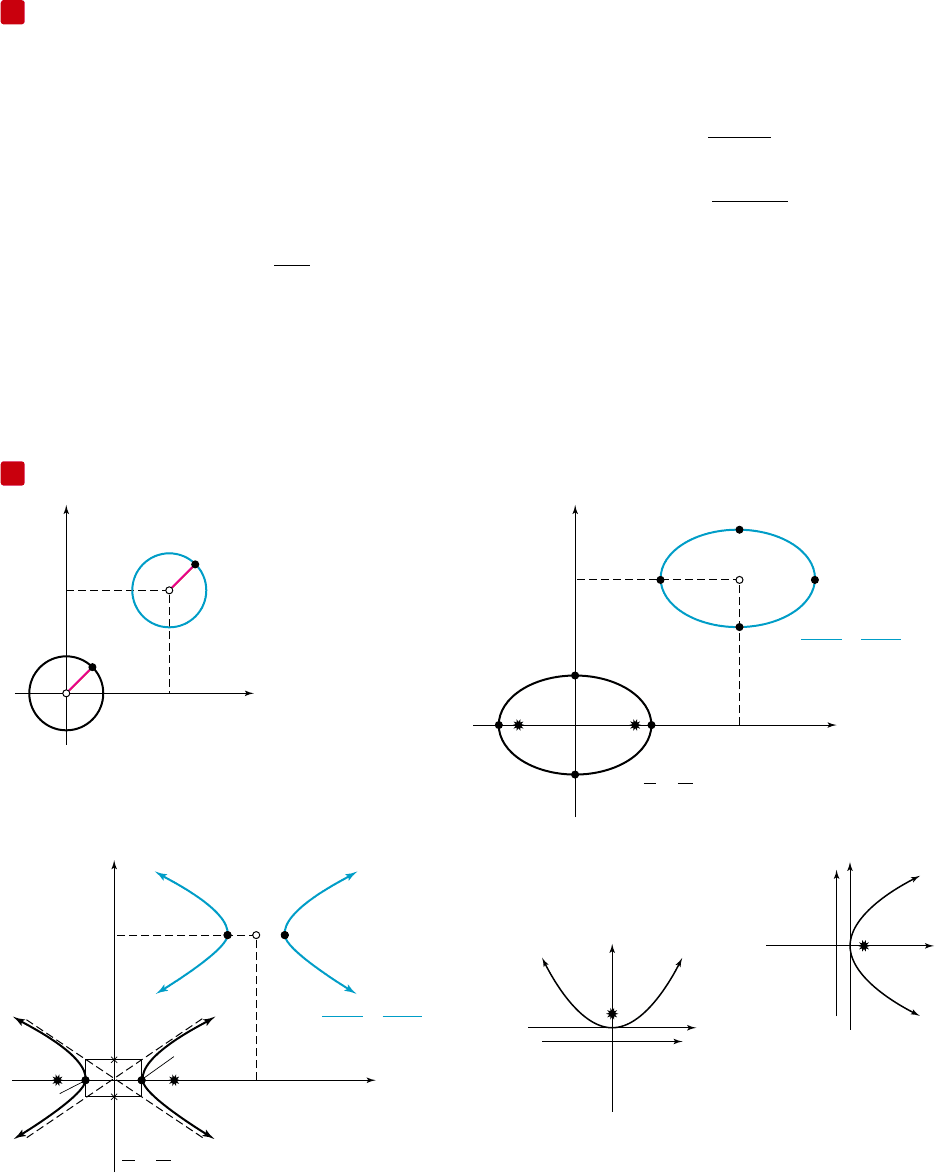
▼
The Toolbox and Other Functions
▼
Transformations of Basic Graphs
Given Function
y f 1x2
Transformation of Given Function
y a f 1x h2 k
vertical reflections
vertical stretches/compressions
horizontal shift h units,
opposite direction of sign
vertical shift k units,
same direction as sign
S
S
S
▼
Average Rate of Change of f(x)
For linear function models, the average rate of change on the interval is constant, and given by the slope formula:
The average rate of change for other function models is nonconstant. By writing the slope formula in function form
using and we can compute the average rate of change of other functions on this interval:
⌬y
⌬x
ⴝ
f(x
2
) ⴚ f(x
1
)
x
2
ⴚ x
1
y
2
f 1x
2
2,y
1
f 1x
1
2
¢y
¢x
y
2
y
1
x
2
x
1
.
3x
1
, x
2
4
linear linear identity constant
absolute value squaring cubing square root
cube root ceiling function floor function reciprocal
reciprocal square exponential logarithmic logistic
y
mx b
(0, b)
x
m 0, b 0
y
(0, b)
m 0, b 0
x
y
y mx b
m 1, b 0
x
y
y x
m 0, b 0
x
y
y b
x
y
x
y
x
y x
2
y
x
y x
3
y
x
y
y
兹
x
y
兹
x
y
x
3
y x
x
y
y x
y
x
21
y
y
x
x
1
y
x
2
1
x
y
y b
x
(b 0)
x
1
y
y log
b
x
(b 0)
1
x
y
y
y
c
1 ae
bx
c
(
0,
)
1 a
c
y
x
ISBN: 0-07-351954-5
Author: John W. Coburn
Title: College Algebra, 3e
Front endsheets
Color: 5
Pages: 6, 7
▼
Quick-Counting and Probability
Fundamental Counting Principle: Given an experiment with two tasks completed in sequence, if the first can be
completed in m ways and the second in n ways, the experiment can be completed in ways.
Permutations—Order Is a Consideration: (Al, Bo, Ray) and (Ray, Bo, Al) finish the race in a different order.
The permutations of r objects selected from a set of n (unique) objects is given by
Combinations—Order Is Not a Consideration: (Al, Bo, Ray) and (Ray, Bo, Al) form the same committee.
The combinations of r objects selected from a set of n (unique) objects is given by
Basic Probability: Given S is a sample space of equally likely events and E is an event defined relative to S.
The probability of E is where and represent the number of elements in each.
For any event and P1E
1
2 P1~E
1
2 1.E
1
: 0 P1E
1
2 1
n1S2n1E2P(E) ⴝ
n(E)
n(S)
,
n
C
r
ⴝ
n!
r!(n ⴚ r)!
.
n
P
r
ⴝ
n!
(n ⴚ r)!
.
m ⴛ n
Probability of E
1
and E
2
Probability of E
1
or E
2
P1E
1
´ E
2
2 P1E
1
2 P1E
2
2 P1E
1
傽 E
2
2
1E
1
, E
2
independent2
P1E
1
傽 E
2
2 P1E
1
2P1E
2
2
▼
Conic Sections
r
r
h
k
(x, y)
x
2
y
2
r
2
(x h)
2
(y k)
2
r
2
(h, k)
(0, 0)
x
y
central
circle
circle with center
at (h, k)
x
y
ellipse with cente
r
at (h, k), a b
central
ellipse
If a b, the ellipse
is oriented vertically.
(h, k b)
(h, k b)
(h a, k)
(h a, k)
(0, b)
(h, k)
(a, 0)
(c, 0) (c, 0)
(0, b)
(a, 0)
k
h
c
2
|a
2
b
2
|
x
2
a
2
y
2
b
2
1
a
2
b
2
(x h)
2
1
(y k)
2
x
y
hyperbola with cente
r
at (h, k)
central
hyperbola
If term containing y leads, the
hyperbola is oriented vertically.
x
2
a
2
(h, k)
(c, 0)
(a, 0)
(c, 0)
(a, 0)
k
h
y
2
b
2
1
a
2
b
2
(x h)
2
1
(y k)
2
c
2
a
2
b
2
x
y
(0, p)
y
p
p 0
x
2
4py
vertical parabola
focus (0, p)
directrix y p
x
y
y
2
4px
horizontal parabola
focus (p, 0)
directrix x p
(p, 0)
x p
p 0
cob19545_es.indd Page Sec1:3 28/01/11 3:42 PM s-60usercob19545_es.indd Page Sec1:3 28/01/11 3:42 PM s-60user /Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles/Volume/204/MHDQ234/cob19545_disk1of1/0073519545/cob19545_pagefiles
