Cao Z. (Ed.) Thin Film Growth: Physics, materials science and applications
Подождите немного. Документ загружается.

318 Thin film growth
© Woodhead Publishing Limited, 2011
observation is thus enhanced by the use of high magnication devices such
as scanning electron microscopy (SEM) and atomic force microscopy (AFM).
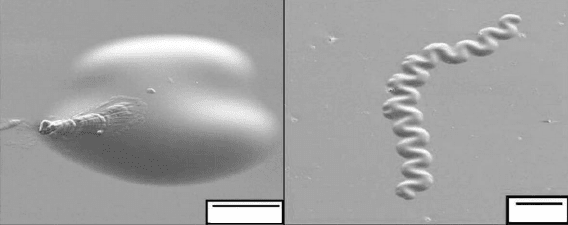
Many types of morphologies are observed: circular, straight-sided (linear
structure with a sinusoidal prole) or telephone cords (streamers forming
zigzags). Whatever the shape, the deection is one order of magnitude higher
than the width, giving the buckles a at aspect. It is worth noting that AFM
images are generally strongly dilated in the vertical direction in such a way
that this at aspect is not shown. A couple of examples of buckling structures
are displayed in Fig. 13.1.
The formation of buckling structures results from two interacting
phenomena: delamination and buckling. Evidence for this interaction comes
from buckles propagating in a lm with a thickness gradient. Since the energy
for buckling is directly proportional to the lm thickness, the buckles stop
when their elastic energy becomes lower than the adhesion energy, as shown
in Fig. 13.2.
Most of the common structures can be modelled based on the Föppl–von
Kármán (FvK) theory of thin plates (Föppl, 1907; von Kármán, 1910).
Analytical solutions for circular and straight-sided buckles have thus been
obtained (Hutchinson and Suo, 1992). However, the experiments show that
the most widely observed structures are the telephone cord patterns (Figs
13.1(b) and 13.2). Parry et al. (2006) demonstrated that the predominance
of these structures is explained by the isotropy of the internal stresses
generally observed in thin lms. This kind of structure is relatively
complex and can only be modelled using numerical methods (Crosby and
Bradley, 1999; Audoly, 2003; Parry et al., 2004, 2006; Jagla, 2006, 2007).
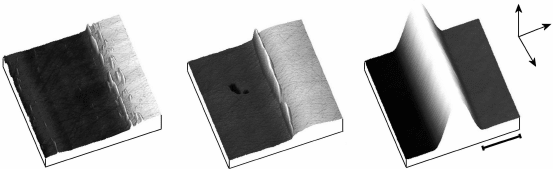
Experimentally, the formation of straight-sided buckles was observed during
uniaxial compression of coated substrates (Coupeau et al., 1999a) as shown
in Fig. 13.3.
(a) (b)
10 µm
100 µm
13.1 Diamond-like thin carbon film deposited on a glass substrate.
SEM images of buckling structures with (a) a circular shape and (b) a
telephone cord shape (after Moon et al., 2002).
ThinFilm-Zexian-13.indd 318 7/1/11 9:44:40 AM
319Understanding substrate plasticity and buckling of thin films
© Woodhead Publishing Limited, 2011
This chapter will focus on the effect of crystalline substrate plasticity on
the generation of buckling structures. In the rst part, general experimental
observations of the phenomenon are described and the mechanisms involved
during the lm buckling and the crystal plastic deformation are explained.
The second part deals with the modelling of the phenomenon in the
framework of the FvK theory of thin plates to obtain the equilibrium shapes
20 µm
13.2 AFM image of telephone cord buckling patterns observed on a
Y
2
O
3
thin film of varying thickness (30–50 nm) deposited on a GaAs
substrate. The structures terminate in the bottom of the image when
the film thickness is too small (after Paumier et al., 2003).
5 µm
13.3 Straight-sided buckles observed by AFM on a 100 nm thick
film of nickel deposited on a uni-axially strained polycarbonate
substrate (after Coupeau et al., 1999a). The structures are oriented
perpendicular to the applied stress.
ThinFilm-Zexian-13.indd 319 7/1/11 9:44:40 AM

320 Thin fi lm growth
© Woodhead Publishing Limited, 2011
of the structures and of the critical stress for buckling. The localization of
the buckling structures on the area of crystal strain is discussed and the
asymmetry of the buckles observed on crystalline substrate is explained. The
model is generalized for situations where several slip systems are activated
under the same buckle and the equilibrium shape of the lm is determined
whatever the initial internal stress and the substrate deformation. Finally, it
is shown in the last part of the chapter how buckling structures can be used
to characterize the mechanical properties of thin lms.
13.2 Experimental observations
13.2.1 Buckling structures
The rst observations of spontaneously buckling structures in thin lms
were made on carbon thin lms deposited on glass substrates (Matuda et al.,
1981). It was shown that spontaneous buckling was driven by the relaxation
of the internal stresses in the lm. Internal stresses are due to the method
of deposition itself (intrinsic stresses) but also to the thermal expansion
mismatch between the lm and the substrate (thermal stresses), as given by
hutchinson et al. (2000):
s
n
a
ther
f
f
t
=
-
E
f
E
f
T
1
DD
a
DD
a
t
DD
t
[13.1]
where E
f
is the Young’s modulus of the lm, n
f
its Poisson’s ratio, Da
t
the
difference in coef cients of linear thermal expansion (a lm – a substrate)
and DT the drop in temperature from the deposit temperature to room
temperature. these stresses are mostly compressive and isotropic and favour
the formation of more or less complex structures, especially telephone cord
buckles (c.f. Figs. 13.1b and 13.2).
During uniaxial compressive tests of metallic thin lms deposited on
polymeric substrate, the formation of straight-sided structures perpendicular
to the compression axis was observed (Coupeau et al., 1999a) (cf Fig. 13.3).
During these mechanical tests, the elastic deformation of the substrate is
totally transmitted to the lm. This deformation creates high stress anisotropy
in the lm, given by Colin et al. (2000):
D
s
nn
n
s
xx
0
f
s
fs
nn
fs
nn
f
2
yy
nn
fs
nn
–
nn
fs
nn
1 –
=
E
f
E
f
E
ex
p
[13.2]
D
s
nn
n
s
yy
0
f
s
fs
nn
fs
nn
f
2
yy
ex
p
=
–
–
E
f
E
f
E
1
1
[13.3]
the stress
s
yy
ex
p
corresponds to the stress applied to the sample along the
(Oy) axis, E
s
and n
s
are the Young’s modulus and the Poisson’s ratio of the
ThinFilm-Zexian-13.indd 320 7/1/11 9:44:41 AM
321Understanding substrate plasticity and buckling of thin films
© Woodhead Publishing Limited, 2011
substrate, respectively. In the lm, the compressive stress along the (Oy)
axis is strongly increased while, for most metallic thin lms deposited on
polymeric substrate, the stress is relaxed along the (Ox) axis since n
f
<
n
s
. This anisotropy easily explains the formation of straight-sided buckles
perpendicular to the applied stress as shown in Fig. 13.3, instead of telephone
cords (parry et al., 2006).
13.2.2 Buckling induced by substrate plasticity
Similar compressive tests were carried out by replacing the polymeric substrate
by a crystalline substrate (Foucher et al., 2006). In this case the substrate is
plastically strained during compression. Crystalline plasticity is driven by the
activation and gliding of dislocations along particular crystal planes, which
lead to the shearing of the sample. A slip system is described by a Burgers
vector and a slip plane, the Burgers vector representing the magnitude and
the direction of the lattice distortion induced by the dislocation in the crystal
lattice. The primary slip systems (with the higher Schmidt’s factor) will
be activated as a function of the compression axis, crystal orientation and
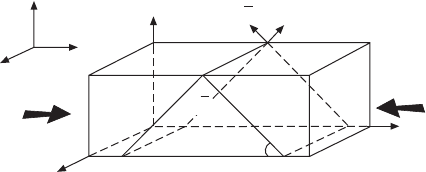
crystalline structure. For instance, assuming a parallelepiped sample with
a cubic crystalline structure (lattice parameter a), oriented along the planes
{100} and compressed along the [010] direction, the activated slip systems
will be given by {110}<110>, corresponding to dislocations with a Burgers
vector a/2<110> gliding in the planes {110}. On the observed (001) surface,
two of the slip systems will create steps as shown in Fig. 13.4.
During plastic strain, the dislocations emerge in groups and form sub-
micrometric steps at the crystal surface. The number and height of these steps
increase with the increasing strain, as shown by Coupeau and Grilhé (2001).
The total height of a surface step is given by H = N · h
e
with h
e
the height
of an elementary step, formed by the emergence of one single dislocation,
z
x
y
0
[100]
[010]
(011)
(001)
(011)
[001]
a/2[011]
a/2[011]
q
13.4 Orientation of the primary slip systems creating step structures
on the surface (001) of a cubic lattice crystal during uni-axial strain.
The stress is applied along the (Oy) axis corresponding to the [010]
crystal plane (large arrows). The slip planes are symmetric and form
an angle q with the surface (001).
ThinFilm-Zexian-13.indd 321 7/1/11 9:44:41 AM
322 Thin film growth
© Woodhead Publishing Limited, 2011
and N the number of emerged dislocations. AFM is particularly suited for
characterizing the mechanisms taking place within the crystal through the
study of these nanostructures (coupeau et al., 1999b; 2004; Coupeau and
Grilhé, 2001).
In the case of coated crystals, the dislocations are locked at the interface
between the lm and the substrate (Coupeau et al., 2000; Foucher and
Coupeau, 2007; Foucher et al., 2006). The formation of steps is observed
on the lm surface corresponding to the pile-up of several dislocations at
the interface. Note that the lm is elastically strained and not sheared by the
dislocations. With increasing strain of the sample, the step height increases
and the formation of straight-sided buckles following the surface steps is
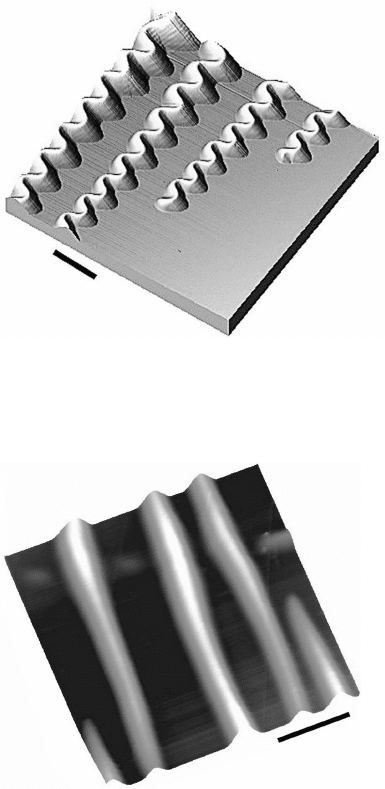
nally observed, as shown in Fig. 13.5.
Figure 13.5 shows that the size of the buckles is directly related to the lm
thickness, the thicker the lm the larger the buckles. The buckles are only
observed above the steps and are characterized by a vertical shift between
their edges corresponding to the height of the underlying step, as clearly
shown by the prole in Fig. 13.6.
13.3 Modelling
13.3.1 The Föppl–von Kármán theory of buckling
The modelling of the buckling phenomenon is based on the FvK theory of
thin plates (Hutchinson and Suo, 1992). A buckled part of lm is considered
initially separated from the substrate. For a straight-sided buckle, the lm
is considered to be initially separated over an innite length along the (Ox)
axis, and over a width 2b, between y = – b and y = b, along the (Oy) axis.
The lm strip is then modelled as a thin plate clamped at its edges whose
displacements are imposed by the substrate. in the case of spontaneous
(a) (b) (c)
10 µm
z
y
x
0
13.5 Nickel thin films deposited on LiF single crystals plastically
strained at –2% (film thickness (a) 20 nm, (b) 75 nm and (c) 150
nm). AFM images show the localization of the buckles on the steps
formed during the emergence of dislocations from the substrate. The
size of the buckles is proportional to the film thickness.
ThinFilm-Zexian-13.indd 322 7/1/11 9:44:41 AM

323Understanding substrate plasticity and buckling of thin fi lms
© Woodhead Publishing Limited, 2011
buckling, these displacements are related to the internal stresses after
deposition. these initial stresses are supposed to be isotropic, they are
labelled s
yy
0
= s
xx
0
= – s
0
in the middle plane of the lm (using this notation,
s
0
is positive in compression). The change in the stresses after buckling is
given by Ds
ii
= s
ii
+ s
0
.
Föppl (1907) and von Kármán (1910) developed the theory of thin plate
buckling in the general framework of linear elasticity. Since buckling
is associated with high out-plane displacement and very small in-plane
displacements (within the plate thickness), the second-order terms for the
out of plane displacement in the Hooke’s equations are considered. In this
case, the FvK equilibrium equation that must be veri ed by the out of plane
displacement w, is given by:
D
h
w
w
x
w
y
w
xy
——
∂
∂
x∂x
∂
∂
y∂y
∂
∂∂
xy∂∂xy
22
——
22
——
xx
2
2
yy
2
2
xy
=
+
–
ss
ss
w
ss
w
∂
ss
∂
∂
ss
∂
xx
ss
xx
2
ss
2
ss
xx
xx
ss
xx
xx
2
2
ss
2
2
+
ss
+
s
2
2
[13.4]
with —
2
the laplacian, h the lm thickness and D the bending stiffness given
by Hutchinson and Suo (1992):
D
h
E
=
–
f
E
f
E
f
2
12
1
n
[13.5]
For a straight-sided buckle, the shape is invariant along the (Ox) axis, i.e. w
does not depend on x, and the FvK equation can be written as:
dw
dy
dw
dy
4
dw
4
dw
4
2
+
=
0
a
a
2
dw
2
dw
2
2
[13.6]
z (µm)
2
1
0
H
–10 0 10
y (µm)
13.6 Nickel thin fi lm 150 nm thick deposited on LiF single crystal
strained at –2%. AFM profi le of a straight-sided buckle formed above
a step structure of height H.
ThinFilm-Zexian-13.indd 323 7/1/11 9:44:42 AM
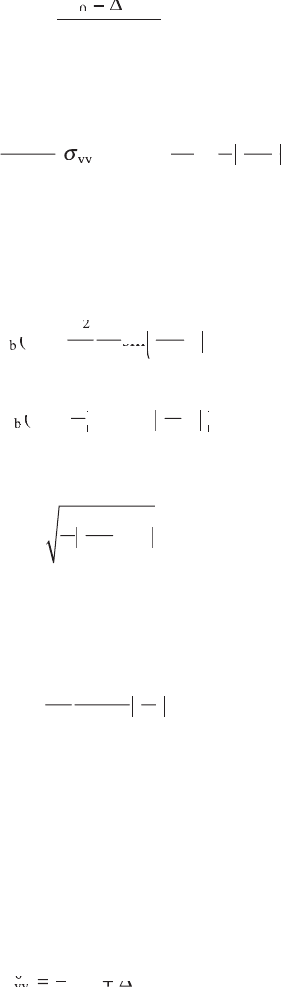
324 Thin fi lm growth
© Woodhead Publishing Limited, 2011
with
a
ss
2
0
ss
0
ss
=
(
ss
–
ss
)
yy
h
D
D
ss
D
ss
[13.7]
In the framework of elasticity, it is shown that the transverse displacement
in the buckle v must satisfy the following equation:
1
1
2
0
2
–
(
+
)
=
+
f
2
f
yy
(
yy
(
n
ss
(
ss
(
+
ss
+
(
yy
(
ss
(
yy
(
E
f
E
f
dv
dy
dw
dy
Ê
Ë
Ê
Ë
Ê
Ê
Á
Ê
Ë
Á
Ë
Ê
Ë
Ê
Á
Ê
Ë
Ê
ˆ
¯
ˆ
¯
ˆ
ˆ
˜
ˆ
¯
˜
¯
ˆ
¯
ˆ
˜
ˆ
¯
ˆ
[13.8]
The pro le of the buckle is given by the parametric equation (y + v(y),
w(y)). Solving Eqs 13.6 and 13.8 leads to two solutions. The rst one is the
planar fundamental solution corresponding to w = 0 and the second one is
the buckled solution given by:
vy
bb
y
vy
b
vy
()
vy()vy
=
pd
p
22
pd
22
pd
12
2
si
bb
si
bb
n
bb
n
bb
Ê
Ë
bb
Ë
bb
Á
Ê
Á
Ê
Ë
Á
Ë
bb
Ë
bb
Á
bb
Ë
bb
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.9]
wy
b
y
wy
b
wy
()
wy()wy
=
1 + cos
d
p
2
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
È
Î
Í
È
Í
È
Î
Í
Î
˘
˚
˙
˘
˙
˘
˚
˙
˚
[13.10]
with d the maximal de ection of the buckle:
d
s
s
=
4
3
– 1
0
c
h
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.11]
The fundamental solution is valid whatever the value of the internal stress
s
0
, but the buckled solution is only valid when s
0
is higher than a critical
value s
c
(also taken positive in compression) given by:
s
p
n
c
f
f
2
=
12
–
2
2
1
E
f
E
f
h
b
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.12]
The pro le of the buckle (y + v
b
(y), w
b
(y)) is plotted in Fig. 13.7. It
corresponds to a sinusoid slightly deformed by the transversal displacement
v
b
which leads to a widening of the upper part and a shrinking of the lower
part of the structure.
In the case of buckling on polymeric substrates induced during compressive
tests, the deformation of the substrate imposes displacements on the edges
of the considered lm strip. These displacements can be seen as an increase
of the internal stress intensity now given by:
ss
s
yy
ss
yy
ss
0
ss
0
ss
0y
s
0y
s
y
0
ss
=
ss
ss
ss
+
0y
+
0y
ss
–
ss
D
0y
D
0y
[13.13]
with
D
s
yy
0
given by Eq. 13.3.
ThinFilm-Zexian-13.indd 324 7/1/11 9:44:44 AM

325Understanding substrate plasticity and buckling of thin fi lms
© Woodhead Publishing Limited, 2011
in this case, the critical stress refers to the stress –
s
yy
0
(–
s
yy
0
>s
c
) and
the stress s
0
needs to be replaced by –
s
yy
0
in Eqs 13.7–13.11. The uniaxial
compressive tests induce an increase of the transversal stress and thus favour
the formation of straight-sided buckles.
13.3.2 Buckling on crystalline substrates
Dislocations emerge on strained crystalline substrates at the lm/substrate
interface leading to the formation of steps above which straight-sided buckles
are localized (cf Fig. 13.4). The presence of the step beneath the buckle
modi es the boundary conditions on the edges of the thin lm strip. For a thin
lm strip of initial width 2b, the formation of dislocations induces an out of
plane displacement w(b) = H = N · h
e
and an in-plane displacement v(b) =
– H/tanq , where q is the angle between the slip plane and the crystal surface
(0° < q £ 90°) as shown in Fig. 13.8. Note that the imposed displacements
are relatively small with respect to the strip width (H << b).
The imposed displacements lead to the following compatibility equation
derived from linear elasticity:
1
2
=
1 –
+
b
+b
f
2
yy
–
ta
n
Ú
–
Ú
–
Ê
Ë
Ê
Ë
Ê
Ê
Á
Ê
Ë
Á
Ë
Ê
Ë
Ê
Á
Ê
Ë
Ê
ˆ
¯
ˆ
¯
ˆ
ˆ
˜
ˆ
¯
˜
¯
ˆ
¯
ˆ
˜
ˆ
¯
ˆ
dw
dy
dy
b
E
H
2
2
n
s
q
D
[13.14]
The transversal displacement is now given by:
vy
dw
dy
dy
E
y
()
vy()vy
= –
1
2
+
1 –
f
2
yy
Ê
Ë
Ê
Ë
Ê
Ê
Á
Ê
Ë
Á
Ë
Ê
Ë
Ê
Á
Ê
Ë
Ê
ˆ
¯
ˆ
¯
ˆ
ˆ
˜
ˆ
¯
˜
¯
ˆ
¯
ˆ
˜
ˆ
¯
ˆ
¥
Ú
2
n
s
D
[13.15]
d
0
z
–b 0 b
y
13.7 Theoretical profi le of a straight-sided buckle given by (y + v
b
(y),
w
b
(y)), of width 2b and height d. The arrows show the orientation of
the transversal displacement v
b
in the upper and lower parts of the
buckle.
ThinFilm-Zexian-13.indd 325 7/1/11 9:44:45 AM
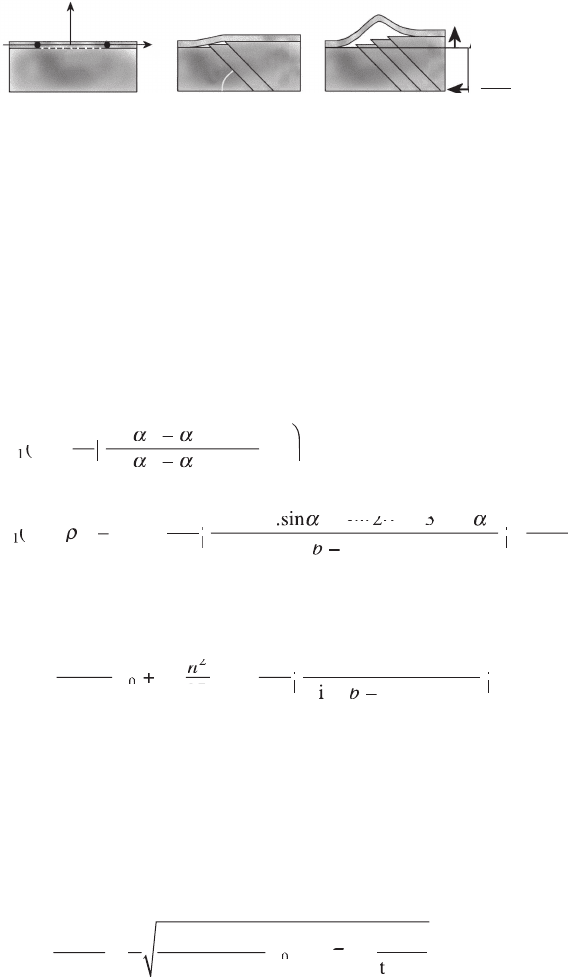
326 Thin fi lm growth
© Woodhead Publishing Limited, 2011
Solving the FvK equation 13.2 leads to two solutions (Foucher et al., 2006).
The rst one corresponds to an unbuckled fundamental solution (similar to
the planar solution in the classical case) and the second one to a buckled
solution. The unbuckled solution is given by:
wy
H
yy
b
bb
b
wy
1
wy
()
wy()wy
=
2
+ 1
si
nc
yyncyy
nc
os
si
nc
bbncbb
nc
os
aa
yy
aa
yy
nc
aa
nc
yyncyy
aa
yyncyy
yy – yyncyy – yy
aa
yy – yyncyy – yy
a
b
a
b
aa
bb
aa
bb
aa
bb
aa
bb
nc
aa
nc
bbncbb
aa
bbncbb
bb – bbncbb – bb
aa
bb – bbncbb – bb
a
b
a
b
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆˆˆ
¯
˜
ˆˆˆ
˜
ˆˆˆ
¯
˜
¯
[13.16]
vy
by
H
by
y
vy
1
vy
2
()
vy()vy
=
(
–)
by–)by
+
32
8cos
byinby
––
2
ra
by
ra
by
(
ra
(
–)
ra
–)
by–)by
ra
by–)by
+
ra
+
aa
by
aa
by
by.sby
aa
by.sby
byinby
aa
byinby
a
si
––si––
ns
ynsy
––ns––
a
ns
a
in
23
a
23
a
ns23ns
ynsy23ynsy
––ns––23––ns––
y––ynsy––y23y––ynsy––y
––ns––23––ns––
a
ns
a
23
a
ns
a
y
a
ynsy
a
y23y
a
ynsy
a
y
a
ns
a
23
a
ns
a
––
a
––ns––
a
––23––
a
––ns––
a
––
aaa
aa
a
q
b
aaa
b
aaa
bb
aa
bb
aa
bb
aa
bb
aa
b
a
b
a
H
(
)
–
si
nc
aa
nc
aa
bbncbb
aa
bb
aa
nc
aa
bb
aa
aa
bb
aa
–
aa
bb
aa
nc
aa
bb
aa
–
aa
bb
aa
os
ta
n
2
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.17]
with
r
n
sa
a
a
= –
1 –
+
sa
+
sa
sa
sa
+
2 + cos
2
(
f
2
f
0
sa
0
sa
22
a
22
a
+
22
+
E
f
E
f
hH
22
hH
22
a
22
a
hH
a
22
a
+
22
+
hH
+
22
+
b
a
b
a
22
hH
22
hH
12
12
16
s
in
iini
co
s
aa
a
bb
aa
bb
aa
b
a
b
a
aa
bb
aa
–
aa
bb
aa
)
2
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.18]
a
2
is given by Eq. 13.7 and can be obtained from the compatibility equation
(Eq. 13.14). The pro le of the lm is nally given by the parametric curve
(y + v
1
(y), w
1
(y)). This pro le is plotted in Fig. 13.9 for various step heights
H. It is shown that H must be smaller than a critical value H
c
otherwise
the out of plane displacement would be associated with negative values
(H
2
in Fig. 13.9). This critical value is associated with a = p/b and is given
by:
H
b
b
E
h
b
c
2
f
2
f
E
f
E
22
2
=
4
3tan
–
2
3
12
(
–
)
+
4
q
n
sp
h
sp
h
22
sp
22
–
sp
–
1
0
sp
0
sp
ta
ttat
n
2
q
[13.19]
When H > H
c
, the fundamental solution w
1
is no longer acceptable due to
the presence of the substrate.
The second solution, labelled buckled solution, for a = p/b is valid for
–b
z
b
y
0
e
0
e
1
> e
0
e
2
> e
1
H
tanq
–
H = N · h
e
q
13.8 Evolution of a delaminated strip of the fi lm of initial width 2b
above a step structure formed during plastic strain of a crystalline
substrate. The underlying step induces an out of plane displacement
H and an in-plane displacement –H/tanq on the right side of the
thin fi lm strip. Above a critical strain (or stress), the formation of a
straight-sided buckle is observed above the step.
ThinFilm-Zexian-13.indd 326 7/1/11 9:44:46 AM
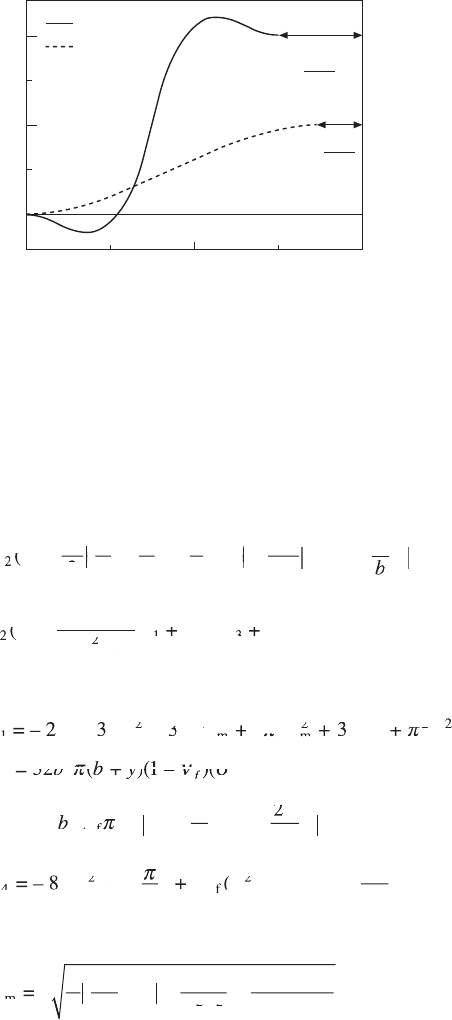
327Understanding substrate plasticity and buckling of thin fi lms
© Woodhead Publishing Limited, 2011
H > H
c
, preventing thus model from any discontinuity, and is given by
Foucher et al. (2006):
wy
H
b
y
y
b
w
wy
2
wy
m
()
wy()wy
=
s
s
in
+
b
b
+
1
+
1 + cos
2
s
2
s
1
2
p
p
p
Ê
s
Ê
s
Ë
s
Ë
s
s
Ê
s
Ë
s
Ê
s
Ê
Á
Ê
s
Ê
s
Á
s
Ê
s
Ë
Á
Ë
s
Ë
s
Á
s
Ë
s
s
Ê
s
Ë
s
Ê
s
Á
s
Ê
s
Ë
s
Ê
s
ˆ
1
ˆ
1
¯
1
¯
1
1
ˆ
1
¯
1
ˆ
1
ˆ
˜
ˆ
1
ˆ
1
˜
1
ˆ
1
¯
˜
¯
1
¯
1
˜
1
¯
1
1
ˆ
1
¯
1
ˆ
1
˜
1
ˆ
1
¯
1
ˆ
1
bbb
y
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
[13.20]
vy
bE
aa
aa
vy
2
vy
2
bE
2
bE
f
bE
f
bE
12
aa
12
aa
34
aa
34
aa
()
vy()vy
=
32
(
aa( aa
12
(
12
aa
12
aa( aa
12
aa
+
aa+ aa
aa
12
aa+ aa
12
aa
+
aa aa
aa
34
aa aa
34
aa
+
aa+ aa
aa
34
aa+ aa
34
aa
)
1
p
[13.21]
with
aE
bH
wH
yw
1f
aE
1f
aE
mm
wH
mm
wH
22
wH
22
wH
m
= – 2
aE = – 2aE
aE
1f
aE = – 2aE
1f
aE
(
wH wH
mm
mm
wH
mm
wH wH
mm
wH
wH+ wH
wH
22
wH+ wH
22
wH
+
yw +yw
pp
bH
pp
bH
wb
pp
wb
bHwbbH
pp
bHwbbH
mm
pp
mm
wb
mm
wb
pp
wb
mm
wb
(
pp
(
bH( bH
pp
bH( bH
wb +wb
pp
wb +wb
wb
mm
wb +wb
mm
wb
pp
wb
mm
wb +wb
mm
wb
mm
mm
pp
mm
mm
wb
mm
wb wb
mm
wb
pp
wb
mm
wb wb
mm
wb
p
yw
p
yw
33
bH33bH
( 33(
bH( bH33bH( bH
pp
33
pp
(
pp
( 33(
pp
(
bH( bH
pp
bH( bH33bH( bH
pp
bH( bH
+
pp
+ 33+
pp
+
wH3wH
22
3
22
wH
22
wH3wH
22
wH
22
bH
22
bH
wb
22
wb
bHwbbH
22
bHwbbH
wb +wb
22
wb +wb
pp
22
pp
bH
pp
bH
22
bH
pp
bH
wb
pp
wb
22
wb
pp
wb
bHwbbH
pp
bHwbbH
22
bHwbbH
pp
bHwbbH
wb +wb
pp
wb +wb
22
wb +wb
pp
wb +wb
33
22
33
( 33(
22
( 33(
+ 33+
22
+ 33+
pp
33
pp
22
pp
33
pp
(
pp
( 33(
pp
(
22
(
pp
( 33(
pp
(
+
pp
+ 33+
pp
+
22
+
pp
+ 33+
pp
+
22
yw
22
yw
22222
20
c
3
)
20
(
20
20
)
20
)(
20
)(
20
)
= – 2
y
ab
20
ab
20
= 32ab = 32
20
= 32
20
ab
20
= 32
20
by
20
by
20
20
(
20
by
20
(
20
a
f
20
f
20
22
(
22
(
)
22
)
by
22
by
( by(
22
( by(
22
1
22
pn
20
pn
20
20
–
20
pn
20
–
20
22
pn
22
pn
20
pn
20
pn
20
pn
20
)
pn
)
20
)
20
pn
20
)
20
pn
20
pn
20
(
pn
(
20
(
20
pn
20
(
20
by
pn
by
20
by
20
pn
20
by
20
( by(
pn
( by(
20
(
20
by
20
(
20
pn
20
(
20
by
20
(
20
+ by+
pn
+ by+
20
+
20
by
20
+
20
pn
20
+
20
by
20
+
20
22
pn
22
)
22
)
pn
)
22
)
22
pn
22
(
22
(
pn
(
22
(
by
22
by
pn
by
22
by
( by(
22
( by(
pn
( by(
22
( by(
+ by+
22
+ by+
pn
+ by+
22
+ by+
1
pn
1
20
1
20
pn
20
1
20
22
1
22
pn
22
1
22
ss
20
ss
20
–
ss
–
22
(
22
20
(
20
pn
(
pn
20
pn
20
(
20
pn
20
22
pn
22
(
22
pn
22
bH
bbHb
Ew
bHEwbH
b
y
b
y
ab
HE
fm
Ew
fm
Ew
4
ab
4
ab
2
HE
2
HE
f
HE
f
HE
4cos
+ cos
ab = – 8ab
Ew
p
Ew
fm
p
fm
Ew
fm
Ew
p
Ew
fm
Ew
pp
2
pp
2
pp
Ê
Ë
Á
Ê
Á
Ê
Ë
Á
Ë
ˆ
¯
˜
ˆ
˜
ˆ
¯
˜
¯
si
n
ppp
p
p
b
yb
Ew
p
Ew
p
H
b
y
yb +yb
(
yb (yb
Ew (Ew
–
)
2
f
(
f
(
Ew (Ew
f
Ew (Ew
2
Ew
2
Ew
m
22
H
22
H
si
n
and
wh
H
Hb
h
m
wh
m
wh
0
c
wh =wh
4
3
– 1
–
3
+
s
s
pp
h
pp
h
q
Ê
Ë
Ê
Ë
Ê
Ê
Á
Ê
Ë
Á
Ë
Ê
Ë
Ê
Á
Ê
Ë
Ê
ˆ
¯
ˆ
¯
ˆ
ˆ
˜
ˆ
¯
˜
¯
ˆ
¯
ˆ
˜
ˆ
¯
ˆ
2
22
h
22
h
pp
22
pp
h
pp
h
22
h
pp
h
22
h
22
h
8
ta
n
q
n
q
[13.22]
The pro le of the buckle (y + v
2
(y), w
2
(y)), is plotted in Fig. 13.10.
H = H
2
>H
c
H = H
1
<H
c
H
2
H
1
0
z
–b 0 b
y
– H
2
tanq
– H
1
tanq
13.9 Theoretical profi le associated with the fundamental solution
(y + v
1
(y), w
1
(y)) of a fi lm undergoing displacements imposed by
deformation of the substrate. When the step height H is higher than
a critical value H
c
(H
2
in the fi gure), the profi le takes negative values
incompatible with the presence of the substrate.
ThinFilm-Zexian-13.indd 327 7/1/11 9:44:47 AM
