Cain G., Meyer G.H. Separation of Variables for Partial Differential Equations: An Eigenfunction Approach
Подождите немного. Документ загружается.

page_102
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_102.html[22/02/2009 23:52:22]
< previous page page_102 next page >
Page 102
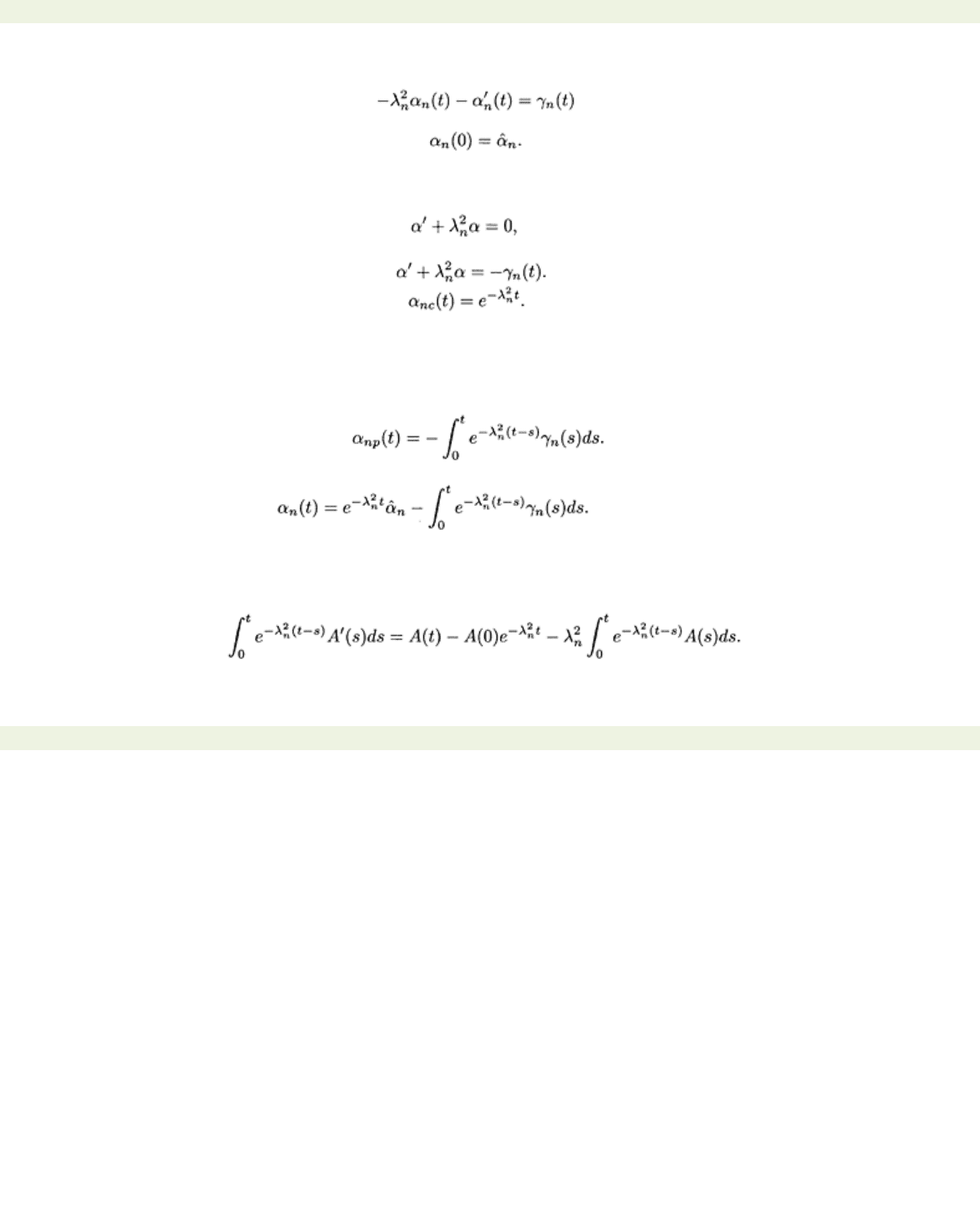
and use of the eigenvalue equation show that
The solution of this equation has the form
αn(t)=dnαnc(t)
+
αnp(t)
where
dn
is a constant,
αnc(t)
is a complementary solution of the equation
and
αnp(t)
is a particular integral of the inhomogeneous equation
For
αnc(t)
we choose
Our ability to find the particular integral analytically will depend crucially on the form of the source term
γ
n
(t)
. If it is the product of a real or complex exponential and a polynomial, then the method of
undetermined coefficients suggests itself. Otherwise the variation of parameters solution can be used
which is
The solution
αn(t)
is then
(5.8)
The approximation to the solution of the original problem is
uN(x, t)=wN(x, t)
+
v(x, t).
We note that the derivatives of the boundary data
A(t)
and
B(t)
occur only under the integral in (5.8). If
we apply integration by parts, then the boundary data need be only integrable. For example
Hence the assumption that
A(t)
and
B(t)
be differentiable may be dispensed with after applying
integration by parts to (5.8) (see also Exercises 5.12 and 5.16).
< previous page page_102 next page >

page_103
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_103.html[22/02/2009 23:52:22]
< previous page page_103 next page >
Page 103
Let us next consider the vibration of a driven uniform string of length
L.
The problem to be solved is
with initial conditions
u
(0,
t
)=
u
0
(x)
ut
(
x,
0)
=u
1
(x).
We shall assume that the boundary and initial conditions are consistent and smooth so that the problem
has a unique smooth solution.
A simple function satisfying the given boundary conditions is
If we set
w(x, t)=u(x, t)−v(x, t),
then
w
satisfies the problem
The associated eigenvalue problem is
which has the solution
These functions are orthogonal in
L
2(0,
L
). The approximating problem is
< previous page page_103 next page >

page_104
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_104.html[22/02/2009 23:52:23]
< previous page page_104 next page >
Page 104
where
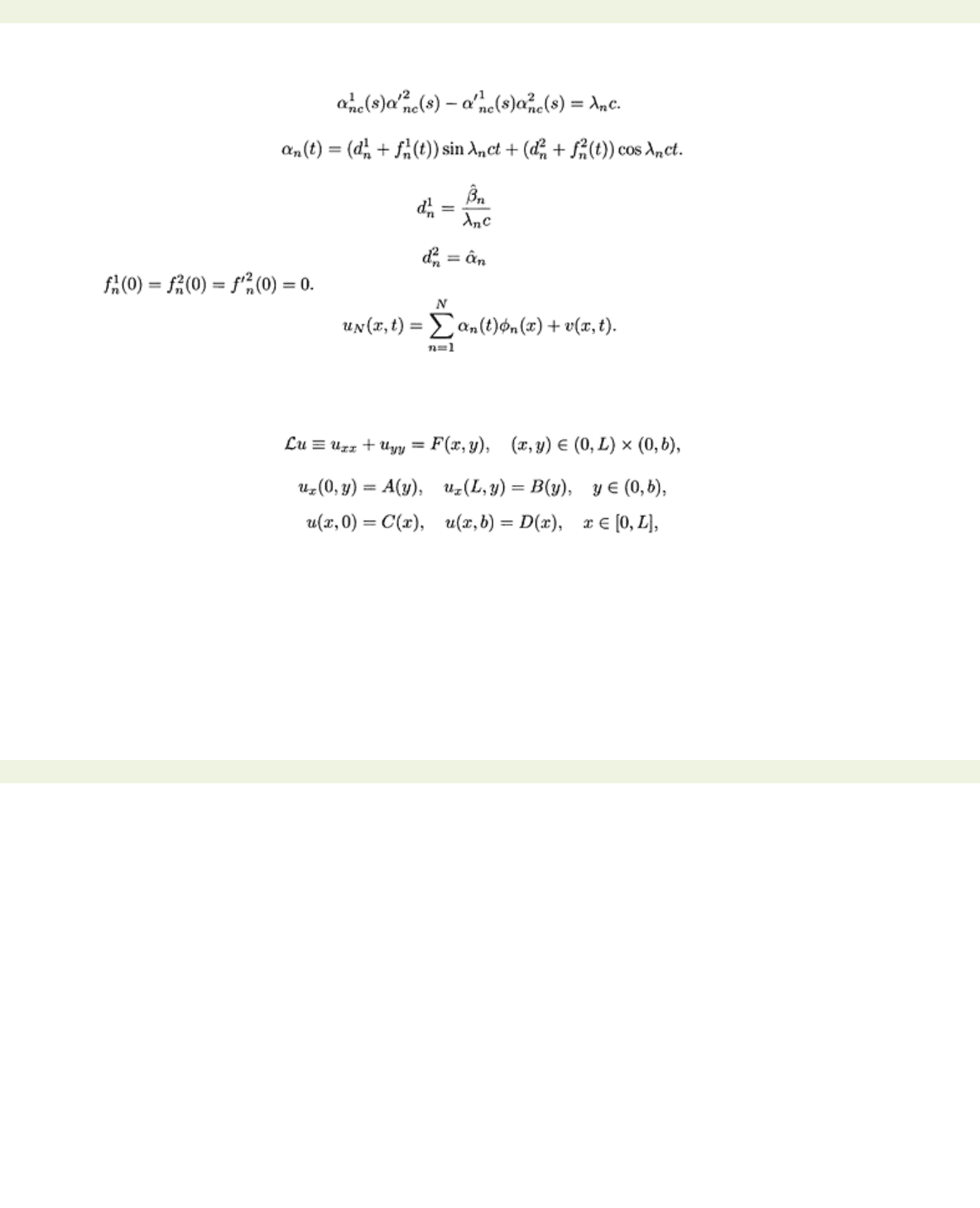
The solution is
where
This equation has a unique solution of the form
where and are two linearly independent solutions of
taken here to be
αnp(t)
is a particular integral of the equation. Its form will depend on the structure of
γn(t)
. If possible,
the method of undetermined coefficients should be applied; otherwise the method of variation of
parameters must be applied which yields the formula
where
< previous page page_104 next page >
page_105
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_105.html[22/02/2009 23:52:24]
< previous page page_105 next page >
Page 105
These integrals will simplify because
The variation of parameters solution for
αn(t)
is
The initial conditions require that
because The solution of the approximating problem is
We point out that just about the same equations result if we consider the case of a simply supported
uniform vibrating beam; see Example 7.8.
For the last illustration of the general eigenfunction expansion approach we turn to the potential
problem
which may be interpreted as a steady-state heat flow problem in a rectangular plate with prescribed
temperatures and fluxes on the boundary. As before we shall assume that the data are smooth
functions.
For an actual calculation it would be simpler to employ eigenfunctions in the
y
-direction, but for this
illustration we shall again choose eigenfunctions in the x-direction. To obtain homogeneous boundary
conditions at
x=
0 and
x=L
we need to find a
v(x, y)
satisfying
vx(0, y)=A(y), vx(L, y)=B(y).
An extensive discussion of the proper choice of
v(x, y)
for making the boundary data homogeneous may
be found in Examples 8.1 and 8.2. Here we simply observe that this time we cannot succeed with a
function which is linear in
x
< previous page page_105 next page >

page_106
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_106.html[22/02/2009 23:52:25]
< previous page page_106 next page >
Page 106
because its derivative only has one degree of freedom. Instead we shall choose the quadratic in
x
The equivalent problem for
w(x, y)=u(x, y)−v(x, y)
is
with
The associated eigenvalue problem is
Its first
N+
1 eigenfunctions and eigenvalues are
which are orthogonal in
L
2 (0,
L
). The approximating problem is
where, e.g.
< previous page page_106 next page >

page_107
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_107.html[22/02/2009 23:52:25]
< previous page page_107 next page >
Page 107
The approximating problem is solved by
if
an(y)
is a solution of the two-point boundary value problem
Note that a solution of this boundary value problem is necessarily unique. This observation follows from
the maximum principle of Section 1.3. Indeed, the difference
en(y)
of two solutions would solve the
problem
The second derivative test now rules out an interior positive maximum or negative minimum so that
en(y)=
0. The solution of the differential equation is again
where now
The particular integral is the same as given above for the wave equation provided
c
2 is replaced by −1
and
t
by
y.
Hence
and must now be determined from the boundary conditions
The final approximate solution of the potential problem is
uN(x, y)=wN(x, y)
+
v(x, y).
It is natural to ask how
wN
is related to the analytic solution
w(x, t)
of the original problem. For several
of the problems considered below we shall prove the following remarkable result:
wN(x, t)
=
PNw(x, t).
< previous page page_107 next page >

page_108
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_108.html[22/02/2009 23:52:26]
< previous page page_108 next page >
Page 108
Hence the computed solution is exactly the projection of the unknown analytic solution. In general one
has a fair amount of information from the theory of partial differential equations about the smoothness
properties of
w.
In particular,
w
is nearly always square integrable. The general Sturm-Liouville theory
can then be invoked to conclude that, at least in the mean square sense,
wN
converges to w as
This implies that when our finite sums are replaced by infinite series, then the resulting function is, in a
formal sense, the analytic solution
w(x, t).
Some quantitative estimates for the quality of the
approximation can be found for specific problems as outlined in the chapters to follow.
Exercises
5.1) Apply the solution process of this chapter to find an approximate solution of the problem
i.e.
i) What do you choose for
v
?
ii) What is the problem satisfied by
w=u−v
?
iii) What is the associated eigenvalue problem?
iv) What is the approximating problem?
v) for
wN.
vi) Find the analytic solution
w.
vii) Show that
wN=PNw.
viii) What is the approximate solution
uN
of the original problem?
ix) Compare
uN
with the analytic solution u as
5.2) Apply the solution process of this chapter to find an approximate solution of the problem
Follow all the steps detailed in problem 5.1.
5.3)
Show that the problem
< previous page page_108 next page >

page_109
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_109.html[22/02/2009 23:52:27]
< previous page page_109 next page >
Page 109
has no solution. Show that the solution process of this chapter breaks down when we try to find an
approximate solution.
5.4)Show that the problem
has infinitely many solutions. Find an approximate eigenfunction solution when the boundary
conditions are zeroed out with
5.5)Apply the solution process of this chapter to find an approximate solution of the problem
5.6)Apply the solution process of this chapter to find an approximate solution of the problem
5.7)Apply the solution process of this chapter to find an approximate solution of the problem
5.8)Apply the solution process of this chapter to find an approximate solution of the problem
< previous page page_109 next page >

page_110
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_110.html[22/02/2009 23:52:28]
< previous page page_110 next page >
Page 110
5.9) Determine
F(x, t), u
0
(x), A(t),
and
B(t)
such that
u(x, t)−xe−t
is a solution of
Use the solution process of this chapter to find an approximate solution
uN.
Compute
e(x,
t)=PNu(x, t)−uN(x, t).
5.10)Determine
F(x, t), u
0
(x), A(t),
and
B(t)
such that
u(x, t)=(x−t)
2
solves
Use the solution process of this chapter to find an approximate eigenfunction solution
uN.
Compute
e(x, t)=PNu(x, t)−uN(x, t).
5.11)Apply the solution process of this chapter to find an approximate solution of the problem
5.12)Let
C
be continuously differentiate with respect to
t
for
t>
0. Show that the initial value problem
< previous page page_110 next page >

page_111
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_111.html[22/02/2009 23:52:28]
< previous page page_111 next page >
Page 111
can be solved in terms of
C(t)
without ever computing
C′(t).
5.13)Apply the solution process of this chapter to find an approximate solution of the problem
5.14)Apply the solution process of this chapter to find an approximate solution of the problem
5.15)Determine
F(x, t), u
0
(x), u
1
(x), A(t),
and
B(t)
such that
u(x, t)
=(1−
x
)
sinωt
solves
Then use the solution process of this chapter to find an approximate solution
uN
of this problem
and compare it with the analytic solution of the original problem.
5.16)Let
C
be twice continuously differentiate with respect to
t
for
t>
0. Show that the initial value
problem
< previous page page_111 next page >
