Brewster H.D. Fluid Mechanics
Подождите немного. Документ загружается.

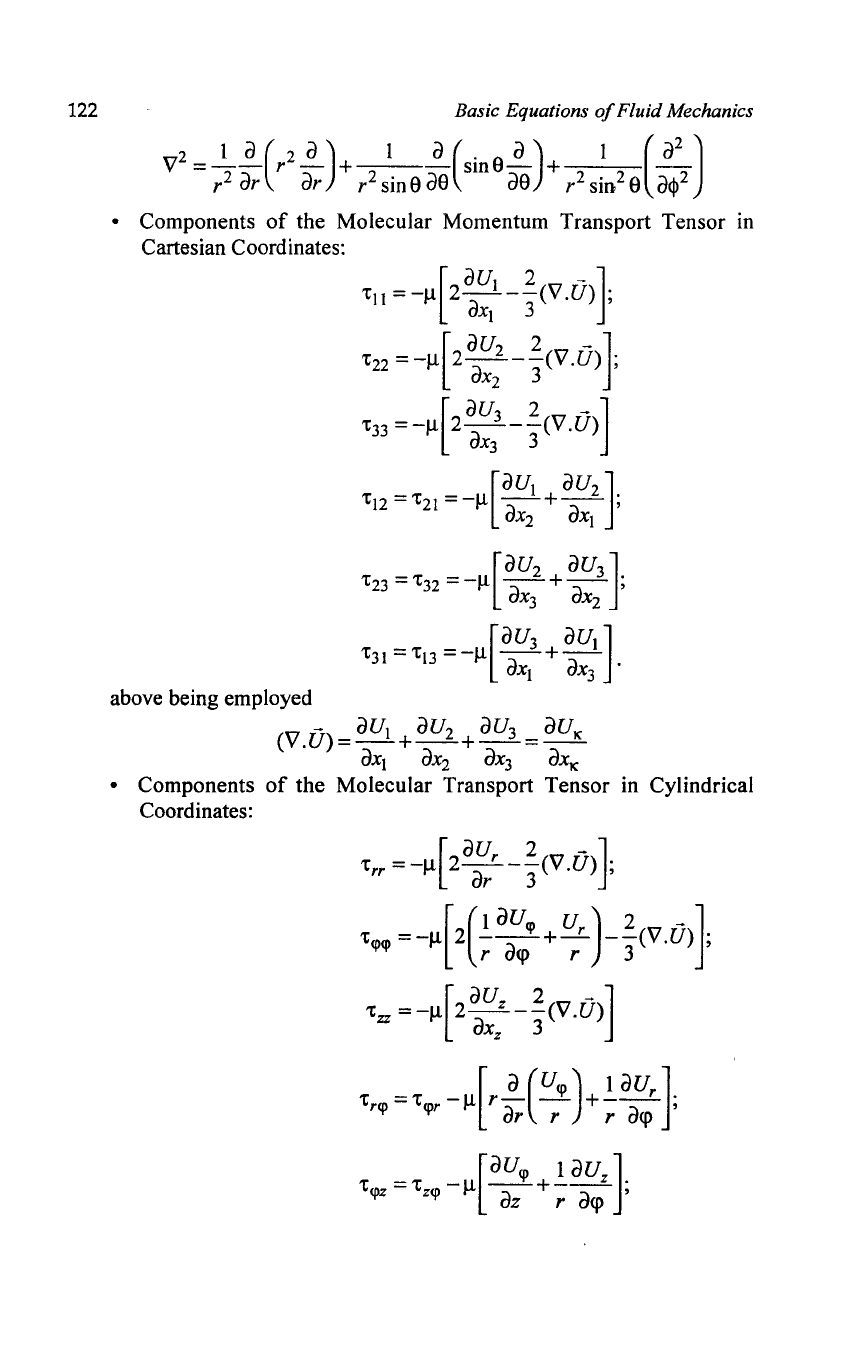
122 Basic Equations
of
Fluid Mechanics
V2
=_1
~(r2~)+
1 a
(Sine~)+
1
(~J
r2
ar
ar
r2
sin e
ae ae
r2
sin
2
e
a<j>2
•
Components
of
the
Molecular
Momentum
Transport
Tensor
in
Cartesian Coordinates:
'tIl
=-Jl
2---(V.u)
;
[
aUI 2 - ]
aXI
3
't22
=
-Jl
2---(V.U)
;
[
aU2
2 - ]
aX2
3
't33
=-Jl
2---(V.u)
[
aU3
2 - ]
aX3
3
above being employed
(V.U)=
aU
I
+
aU
2
+
aU
3
=
au
lC
aXI
aX2
aX3
axlC
•
Components
of
the
Molecular
Transport
Tensor
in Cylindrical
Coordinates:
[
au
2 - ]
't
=-Jl
2_r
--(V
U)
.
rr
ar 3 . ,
[
(
1
au
IP
U)
2 - ]
't
=-Jl
2
___
+_r
--(V.u);
<PIP
r
acp
r 3
't
zz
=-Jl
2---(V.U)
[
aU
z
2 - ]
ax
z
3
't
='t
-Jl
--<P
+
___
z •
[
au
1
au
]
<pz
Z<P
az
r
acp
,
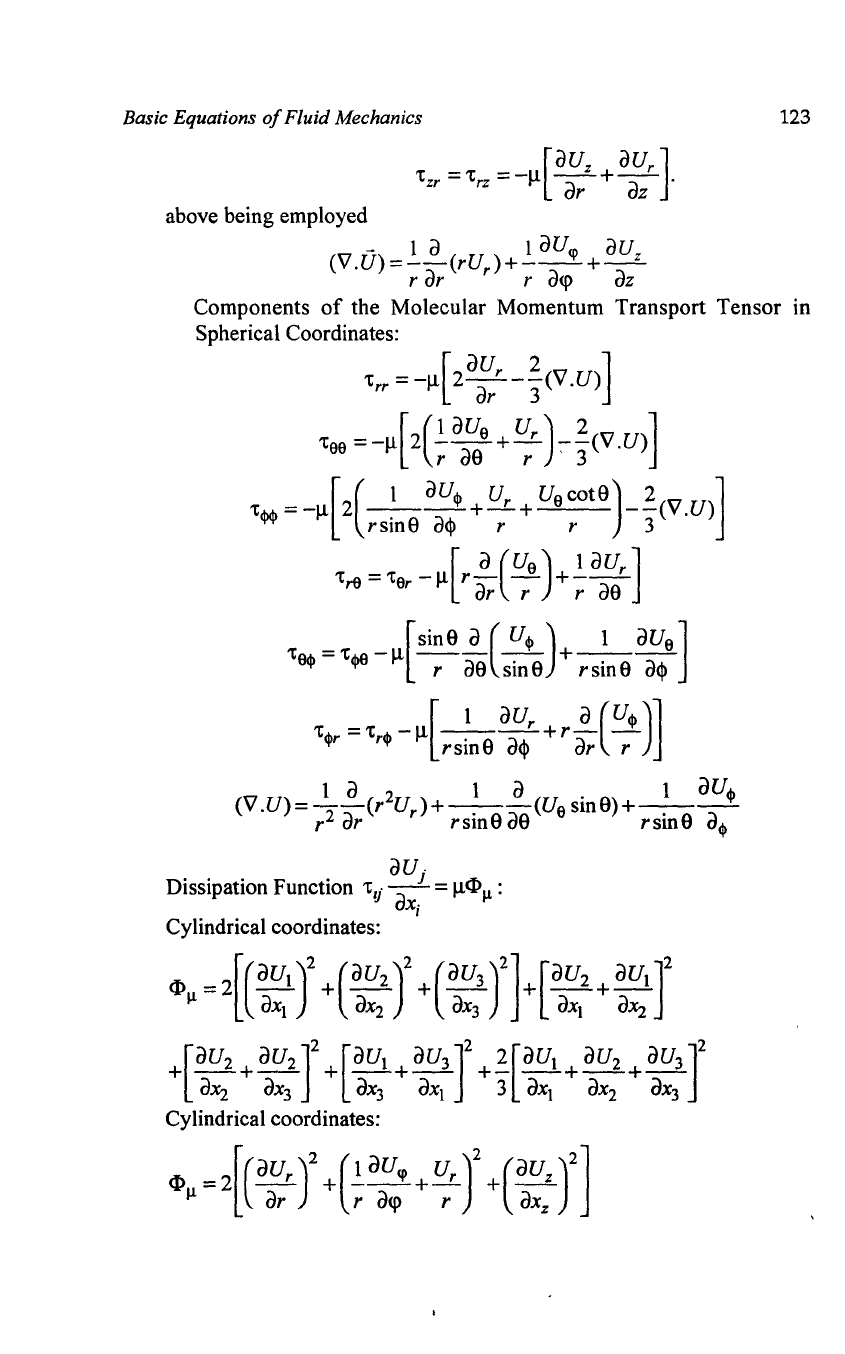
Basic Equations
of
Fluid Mechanics
123
above being employed
- 1 a 1
aUq>
au
(V
.U)
= --(rU
r
)
+
___
+_z
r
ar
r
a<p
az
Components
of
the Molecular Momentum Transport Tensor in
Spherical Coordinates:
[
aUr 2
rT
]
't
rr
=
-11
2-a;:--
3(
v
.U)
[
(
1
aUe
Ur ) 2 ]
'tee
=-11 2
--+-
-:--(v.u)
r
ae
r 3
't<l*
=
-11[2(-~
_aucp
+ U
r
+ U
e
cote)_3.(v.u)]
rsme
a<l>
r r 3
'tre
='ter
_11[r~(Ue)+~
au
r
]
ar
r r
ae
[
sine
a (
Ucp
) 1
aUe]
't
e
= 't -11
---
--
+
----
cp
cpa
r
ae
sine
rsine
a<l>
[
1
aU
r
a
(Ucp)]
'tcpr
='t
rcp
-11
-.---+r-
--
rsme
a<l>
ar
r
1 a 2 1 a . 1
aucp
(V.u)=--(r U
)+---(Uesme)+----
r2
ar
r
rsine
ae
rsine
acp
au·
Dissipation Function
't1J·
-a
J =
11<I>~
:
Xi
Cylindrical coordinates:
~"=2[(~~'
r
+(~
r
+(~:
)}[aa~2
+
":,'
r
+[aU
2
+
au
2
]2
+[aU
l
+
au
3
]2
+3.[aU
l
+
aU
2
+
au
3
]2
a~
aX3
aX3
aXl
3
aXl
aX2
aX3
Cylindrical coordinates:
<I>
=
2[(au
r
)2
+(~
aucp
+ U
r
)2
+(au
z
)2]
~
ar
r
a<p
r
ax
z
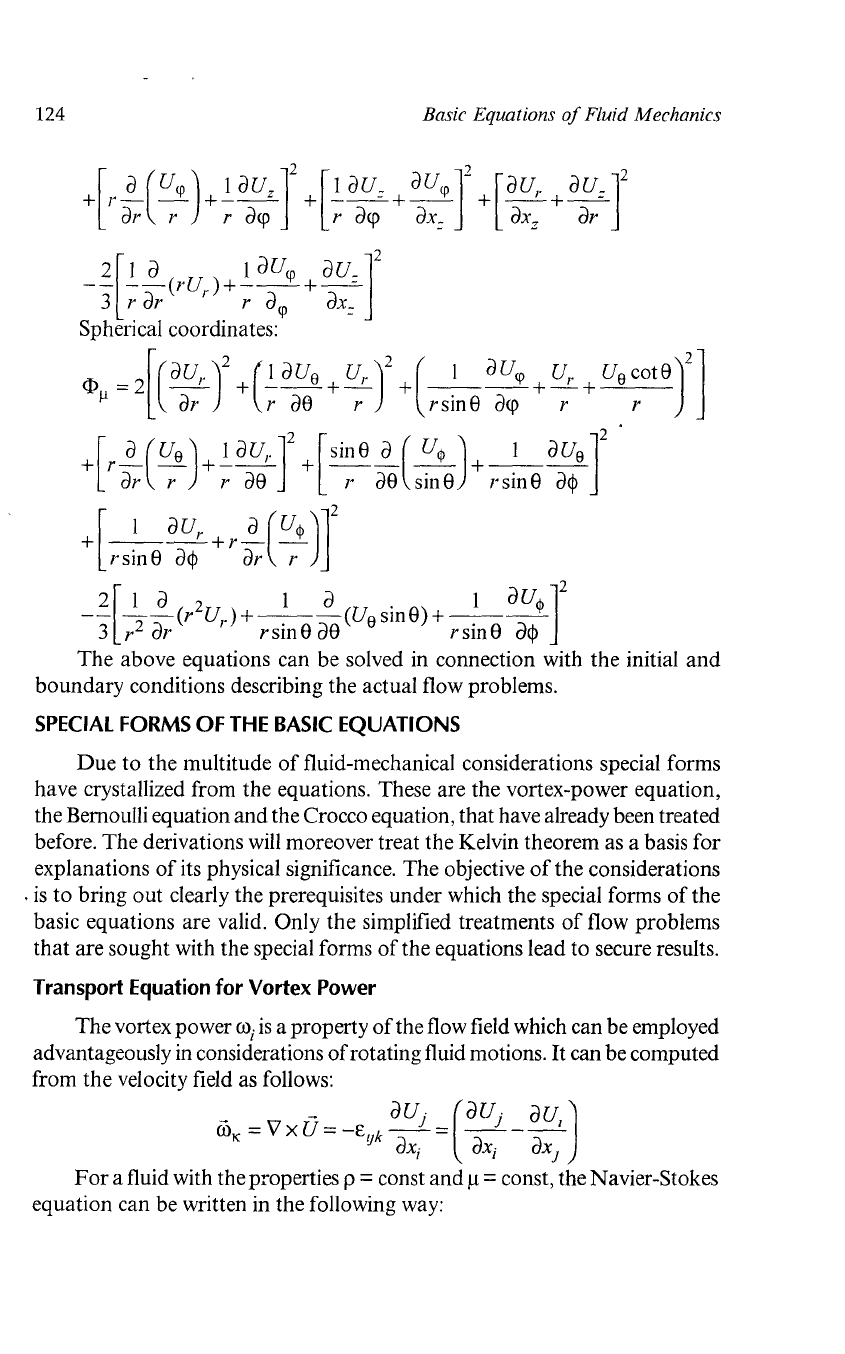
124
Basic Equations
of
Fluid Mechanics
+[r~(U<fJ)+l
aU
Z
]2
+[l
au::
+
aU<fJ]2
+[aU
r
+
aU::]2
ar
r r
a<p
r
a<p
ax::
ax
z
ar
-~[l~(rUr)+l
aU<fJ
+
au~]2
3 r
ar
r
a<fJ
ax::
Spherical coordinates:
<I>
=
2[(au
r
)2
+(l
au
s
+ U
r
)2
+(_?
_au<fJ
+
u,.
+
Us
cote)2]
11
ar
r
ae
r r
sm
e
a<p
r r
+
:r(~·
)+~
a~
r
+[
si~8
:8C~'8)+
rs:n8
a~.
r .
{Si~8
a~
+r
:r(~')J
2
[1
a 2 1 a . 1
aUcp]2
--
--(r
U.)+---(Ussme)+----
3
r2
ar
1
rsine
ae
rsine
acj>
The above equations can be solved in connection with the initial
and
boundary conditions describing the actual flow problems.
SPECIAL
FORMS
OF
THE
BASIC
EQUATIONS
Due
to the multitude
of
fluid-mechanical considerations special forms
have crystallized from the equations. These are the vortex-power equation,
the Bernoulli equation and the Crocco equation, that have already been treated
before. The derivations will moreover treat the Kelvin theorem as a basis for
explanations
of
its physical significance. The objective
of
the considerations
.
is
to bring out clearly the prerequisites under which the special forms
of
the
basic equations are valid.
Only the simplified treatments
of
flow problems
that are sought with the special forms
of
the equations lead to secure results.
Transport
Equation
for Vortex
Power
The vortex power
Wi
is
a property
of
the flow field which can be employed
advantageously in considerations
of
rotating fluid motions. It can be computed
from the velocity field as follows:
- -
au)
(au)
au,
J
wJ(
=
VXU=-Cljk--=
-----
aXi
aXi
ax;
For
a fluid with the properties p = const
and
/l = const, the Navier-Stokes
equation can be written in the following way:

Basic Equations
of
Fluid Mechanics
125
or
in vector form:
[
aU
-
-]
1 2 -
-+(U.\1)U
=--\1P+v\1
U+pg
at
p
Considering
that
this vector form
of
the Navier-Stokes equation can also
be written as:
au
(1
-
-)
- - I 2 -
-+\1
-U.U
Ux(\1xU)=--\1P+v\1
U+g
at
2 p
When one applies
the
operator
\1
x (
...
) .
to
each
of
the
terms appearing
in the above equation
one
obtains:
a& _
\1x
(Ux
&) = v\1
2
&
at
Making
use
of
the
relation valid for vectors:
\1
x (U x
&)
= U(\1.&) - &(\1.U) - (U. \1)& + (&. \1)U
where
\1.
& = 0 as
the
divergence
of
the
rotation
of
each vector
is
equal
to
zero,
and
where
at
the
same time p = const
\1.0
= 0 holds owing to the
continuity equation.
When
one
introduces all this
into
the
above equations,
the
transport equation for the vortex power reads:
a& + (U\1)& = (&\1)U + v\1
2
&
at
or
in tensor notation:
Dro
j
aro
j
aro
j
aU
I
a
2
ro
j
--=--+U1-a-=ro
j
-
a
+v--
2
-
Dt
at
Xi
Xj
aXi
The
equation does
not
contain
the
pressure term, from this it
is
apparent
that
the
vortex-power field can
be
determined
without
knowledge
of
the
pressure distribution.
To
be able to
compute
the
pressure,
one
forms the
divergence
of
the
Navier-Stokes equatiorl
and
obtains for
gj
=
0:
~(P)ro2+U
a
2
u
j
_!
a
2
u;
ax; p j j ax; 2 ax;
Thus
yields
the
Poisson equation for the
computation
of
the
pressure.
For
two-dimensional flows, for which the vortex-power vector stands vertical
on
the flow plane, (&.\1)U =
o.
The
transport
equation
for the vortex power
therefore reads:
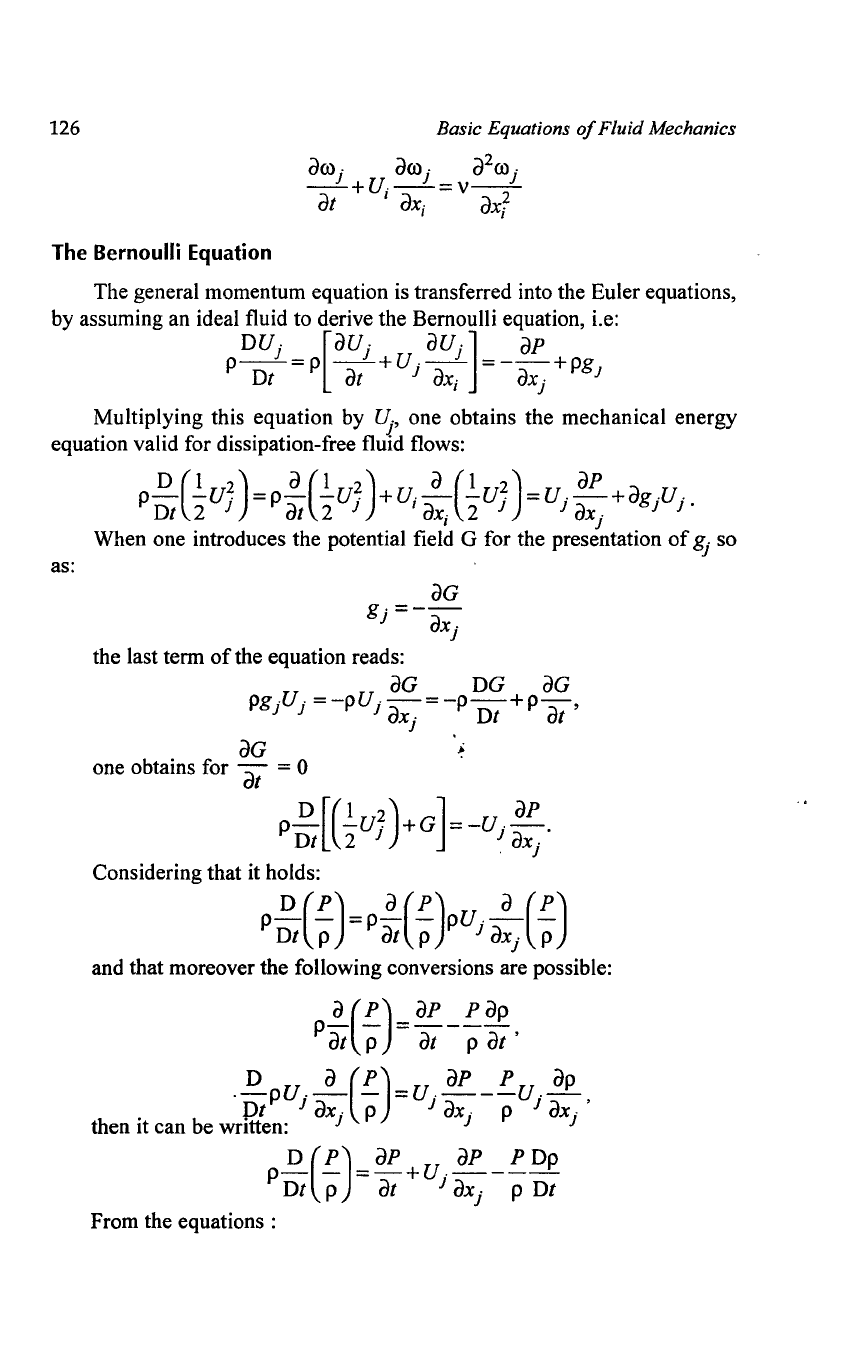
126
Basic Equations
of
Fluid Mechanics
aO)
.
aO)
. (
2
0)
.
__
J
+U.
__
J
=v
__
J
at
I
ax;
ax;
The Bernoulli Equation
The general momentum equation is transferred into the Euler equations,
by assuming an ideal fluid to derive the Bernoulli equation, i.e:
p
__
J
=p
__
J
+u.
__
J =
__
+pg
DU·
[au.
au.]
ap
Dt
at J
ax;
ax
j J
Multiplying this equation by
~,
one obtains the mechanical energy
equation valid for dissipation-free fluid flows:
as:
p~(!u~)=p~(!u~)+U.~(!U2)=U.
ap
+ag.u.
Dt
2 J
at
2 J I
ax.
2 J J
ax.
J
J.
I J
When one introduces the potential field G for the presentation
of
gj
so
aG
g.=--
J
ax.
J
the last term
of
the equation reads:
aG
DG
aG
pgjU
j
=-pU
j
ax.
=-p
Dt
+Pat'
J
aG
~
one obtains for
at
= 0
p~[(!U~)+GJ=-u.
ap.
Dt
2 J J
ax.
. J
Considering that it holds:
p~(P)
=
p~(P)pu.
~(P)
Dt
P
at
p J ax j p
and that moreover
the
following conversions are possible:
p~(P)=
ap
_ p ap
at
p at p
at
'
.~Pu.~(P)=u.
ap
_ p
U.
ap
Dt
J
ax.
p J
ax.
p J
ax.
'
then it can be written: J J J
p~(P)=
ap
+U.
ap
_ p
Dp
Dt
P at J ax j p
Dt
From the equations :
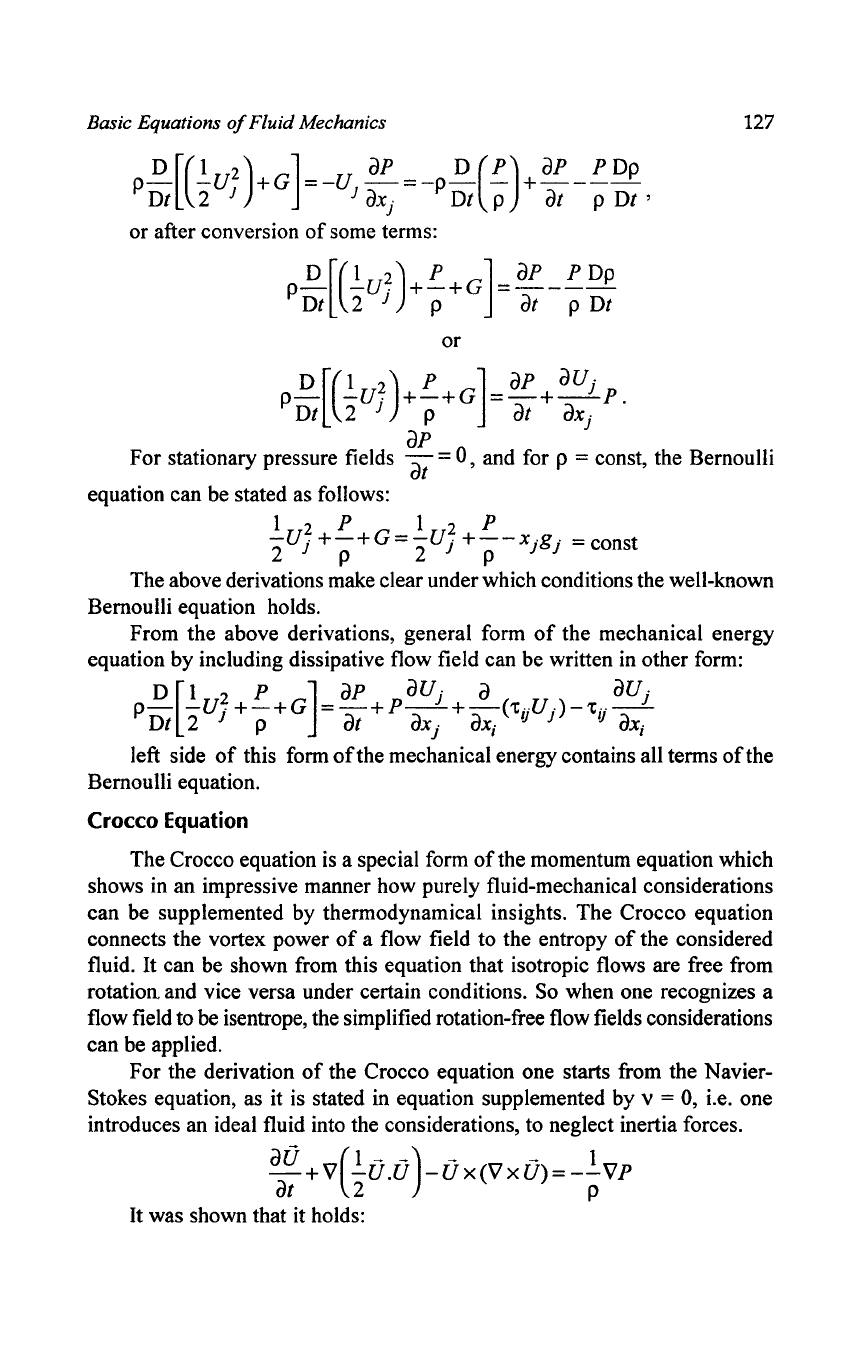
Basic Equations
of
Fluid Mechanics
127
p~[(!U2)+G]=-U
ap
=_p~(P)+
ap
_
PDp
Dt
2 1 1
ax
f Dt P
at
p Dt '
or after conversion
of
some terms:
p~[(!U~)+
P
+G]=
ap
_ P
Dp
Dt 2 1 P
at
p
Dt
or
p~[(!U~)+
P
+G]=
ap
+
aUf
P.
Dt 2 1 P
at
aXf
ap
For stationary pressure fields at =
0,
and for p = const, the Bernoulli
equation can be stated as follows:
1 2 P 1 2 P
-U·
+-+G=-U·
+--x.g.
=const
21
p
21
P
11
The above derivations make clear under which conditions the well-known
Bernoulli equation holds.
From the above derivations, general form
of
the mechanical energy
equation by including dissipative flow field can be written in other form:
D
[1
2 P ]
ap
aUf
a
aUf
PDt
"2
Uj
+-p +G
=at+
P
ax.
+ ax. ('CijUj)-'Cij ax.
l'
,
left side
of
this form
of
the mechanical energy contains all terms
ofthe
Bernoulli equation.
Crocco
Equation
The Crocco equation
is
a special form
of
the momentum equation which
shows in an impressive manner how purely fluid-mechanical considerations
can be supplemented by thermodynamical insights. The Crocco equation
connects the vortex power
of
a flow field to the entropy
of
the considered
fluid.
It
can be shown from this equation that isotropic flows are free from
rotation and vice versa under certain conditions.
So when one recognizes a
flow field to be isentrope, the simplified rotation-free flow fields considerations
can be applied.
For the derivation
of
the Crocco equation one starts from the Navier-
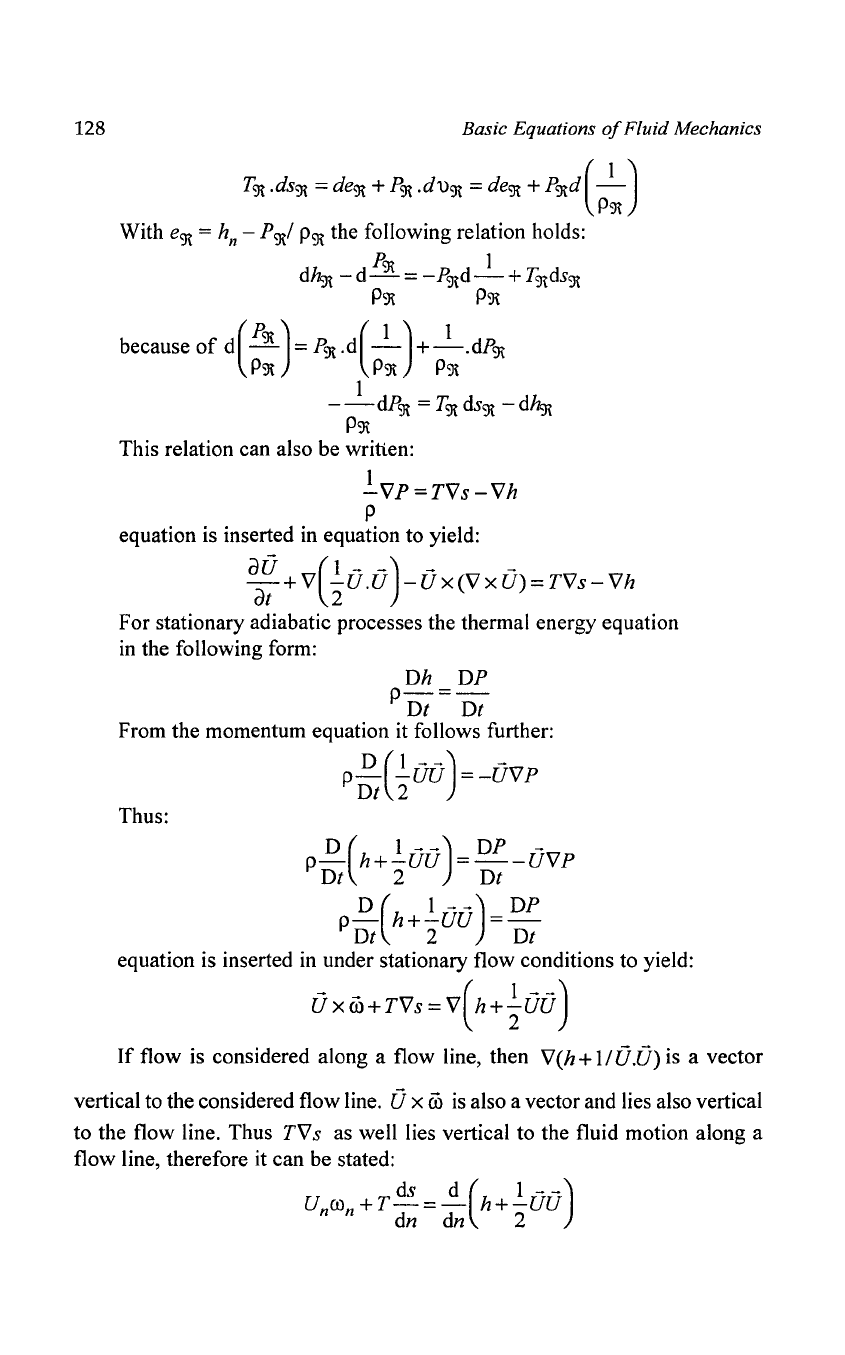
Stokes equation, as it is stated in equation supplemented by v = 0, i.e. one
introduces an ideal fluid into the considerations, to neglect inertia forces.
au
(1
-
-)
- - 1
-+v
-U.U
-Ux(VxU)=--VP
~
2 P
It was shown that it holds:
128 Basic Equations
of
Fluid Mechanics
T9t
.ds
9t
=
de9t
+ P
9t
.
du
9t
=
de9t
+
P9td(p~)
With
e9t
= h
n
-
P
9t
/
P9t
the following relation holds:
P
9t
1
d~
-d-=-P
9t
d-+T
9t
ds
9t
P9t
P9t
because
of
d ( P
9t
) = P
9t
. d
(_1_)
+ _1_.
dP
9t
P9t P9t P9t
1
--dP
9t
=
T9t
ds9t
-d~
P9t
This relation can also be written:
!...VP=TVs-Vh
P
equation is inserted in equation to yield:
au
(1
-
-)
- -
-+V
-U.U
-Ux(VxU)=TVs-Vh
at
2
For stationary adiabatic processes the thermal energy equation
in the following form:
Dh DP
P-=-
Dt Dt
From the momentum equation it follows further:
D(1
--)
-
P-
-UU
=-UVP
Dt
2
Thus:
D ( 1
-
-)
DP -
P-
h+-UU
=--UVP
Dt
2
Dt
D ( 1 -
-)
DP
P-
h+-UU
=-
Dt
2
Dt
equation is inserted in under stationary flow conditions to yield:
- ( 1
--)
UXOJ+TVs=
V h+"2UU
If
flow is considered along a flow line, then
V(h
+
11
U.u)
is a vector
vertical to the considered flow line.
U x
OJ
is also a vector and lies also vertical
to the flow line. Thus
TV
s as well lies vertical to the fluid motion along a
flow line, therefore it can be stated:
U
co
+T-=-
h+-UU
ds
d ( 1
--)
nn
dndn
2
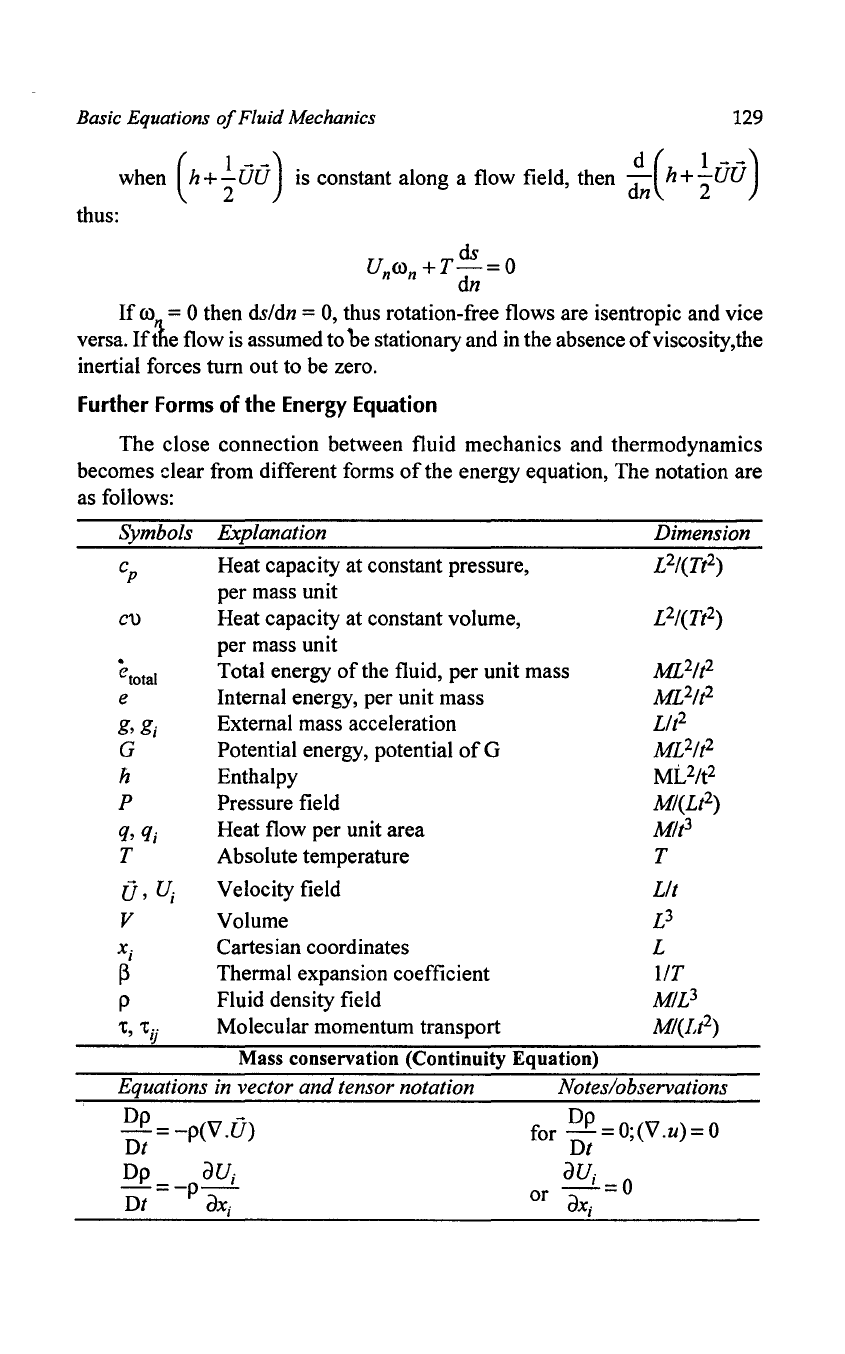
Basic Equations
of
Fluid Mechanics
129
(
1
--)
when
h+"2UU
d ( 1
--)
is constant along a flow field, then dn h +
"2
UU
thus:
ds
U
(0
+T-=O
n n dn
If
(0
= 0 then dsldn =
0,
thus rotation-free flows are isentropic and vice
versa.
Ifdle flow is assumed to
be
stationary and in the absence
of
viscosity, the
inertial forces turn out to be zero.
Further
Forms
of
the
Energy
Equation
The close connection between fluid mechanics and thermodynamics
becomes clear from different forms
of
the energy equation, The notation are
as follows:
Symbols Explanation
Dimension
c
p
Heat capacity at constant pressure,
per mass unit
L2/(Tt2)
c\)
Heat capacity at constant volume,
L2/(Tt
2
)
per mass unit
.
Total energy
of
the fluid, per unit mass
ML21t
2
e
total
e Internal energy, per unit mass
ML21t
2
g,gj
External mass acceleration
Llt
2
G Potential energy, potential
of
G
ML21t
2
h
Enthalpy
ML2/t
2
P Pressure field MI(Lt
2
)
q,
qj
Heat flow per unit area
Mlt
3
T Absolute temperature
T
O,U
j
Velocity field
LIt
V
Volume
L3
Xj
Cartesian coordinates
L
~
Thermal expansion coefficient
liT
p
Fluid density field
MIL3
't,
'tij
Molecular momentum transport MI(Lt
2
)
Mass conservation (Continuity Equation)
Equations
in
vector and tensor notation Notes/observations
Dp
__
-p('['7.U-)
Dp
0
('['7
) 0
v for - = ;
v.u
=
Dt Dt
Dp
aU
j
au
j
_
o
-=-p-
or
-
lli
~ ~
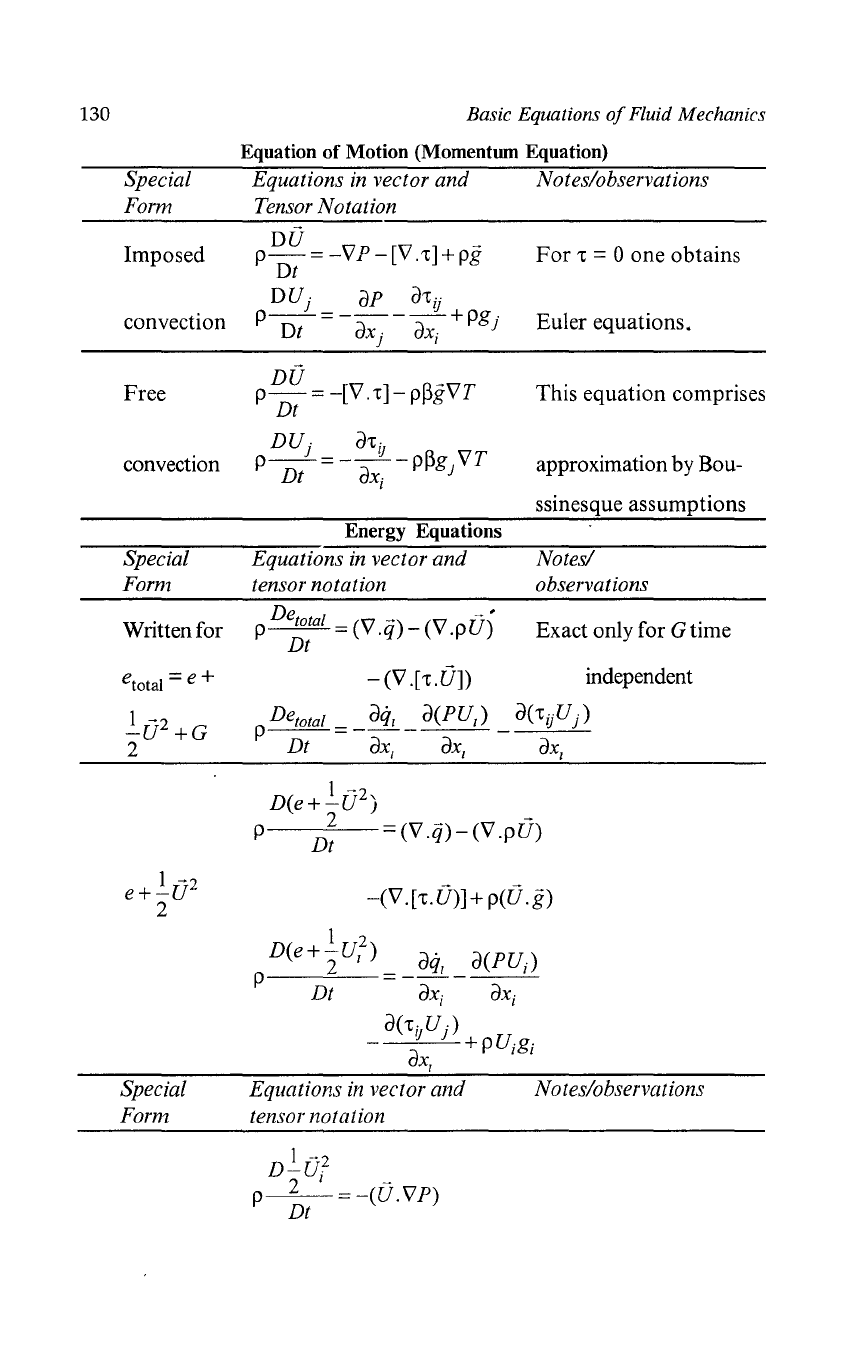
130
Special
Form
Imposed
convection
Free
convection
Special
Form
Written for
etotal = e +
1
-2
e+-U
2
Special
Form
Basic Equations
of
Fluid Mechanics
Equation of Motion (Momentum Equation)
Equations
in
vector
and
Notes/observations
Tensor Notation
P D U =
-\7
P _
[\7
. 't] + P g
Dt
DU)
dP d'tij
p--=----+pg)
Dt
dX)
dX
l
P
DU
= -[\7. 't] -
p~g\7T
Dt
DU)
d'tij
p-=---p~g
\7T
Dt
dXi
}
Energy Equations
Equations in vector
and
tensor notation
p
Detotal
= (\7 .
q)
- (\7 . p
U)
Dt
-(\7.['t.U])
For't
= 0 one obtains
Euler equations.
This equation comprises
approximation by Bou-
ssinesque assumptions
Notes!
observations
Exact only for G time
independent
d('t··U .)
Ij
}
dX
z
1
-2
D(e+-U
)
p 2 = (\7
.q)
- (\7
.pO)
Dt
-(\7
.['t.0)] + p(U.
g)
Equations
in
vector
and
tensor notation
D~02
P 2 1
=-(U.\7P)
Dt
Notes/observations
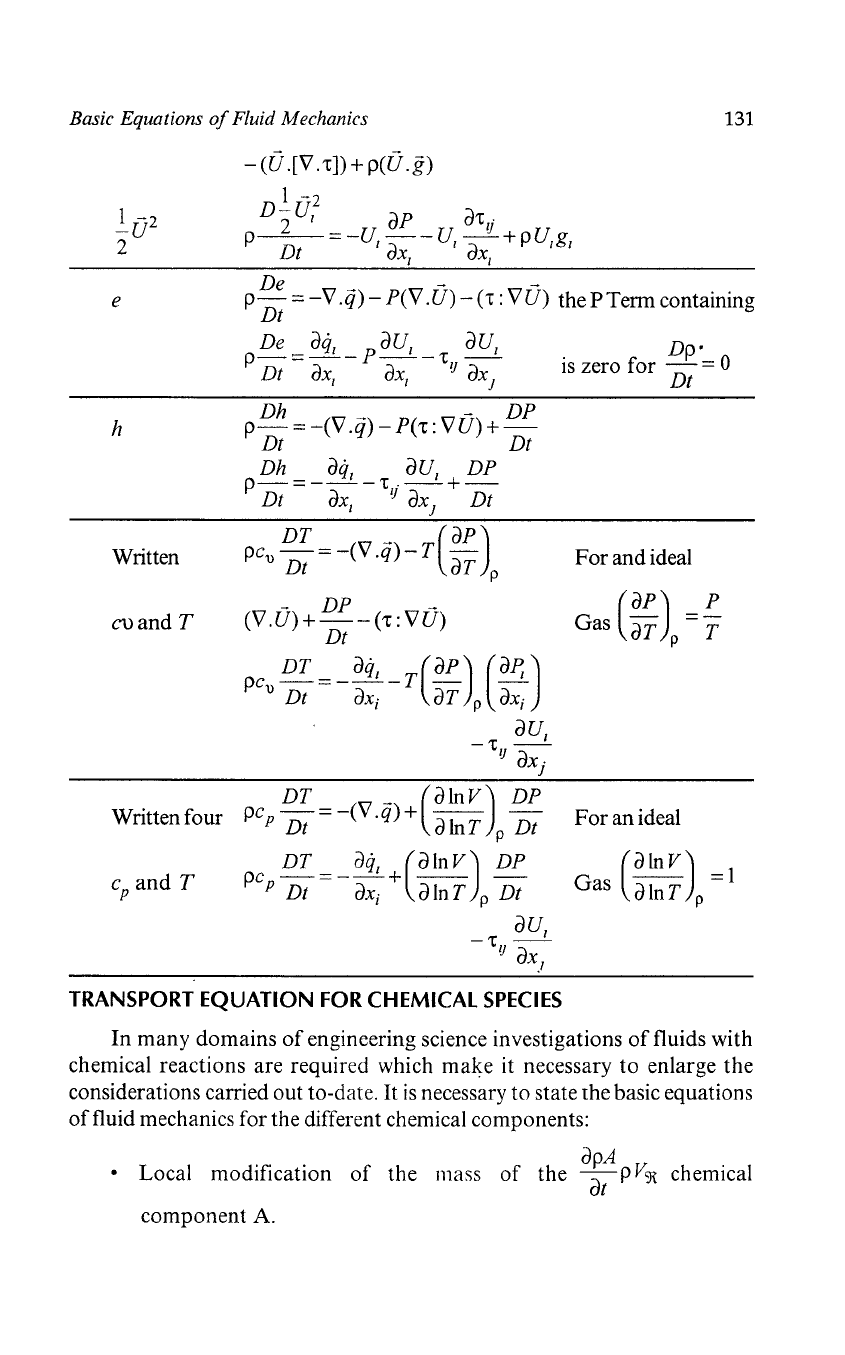
Basic Equations
of
Fluid Mechanics
131
- (0 .[V.
1:])
+ p(O
.g)
1
-2
D-U
l
dP
d1:·
P
2
=-U
--U
-.-iL+pUg
Dt 1
dX
l
1
dX
l
1 1
e
De
--
p-
=
-V.q)
- P(V.U)
-('t:
VU)
thePTerm
containing
Dt
h
Written
cuand
T
Written four
C
p
and
T
pDe::::
dql
_pdUI
-1:
dU
I
Dt
dX
I
dX
l
1)
dX]
Dh
_ -
DP
p-
=
-(V.q)
- P(
1:
: V
U)
+-
Dt Dt
Dh
dql
dU
I
DP
P-=---1:
.--+-
Dt
dX
I
1)
dX]
Dt
pc
DT
=_(V.q)_T(dP)
U Dt dT p
-
DP
-
(V.
U)
+ - -
(1:
: V
U)
Dt
pc DT
__
dql
_T(dP)
(d~)
U Dt -
chi
dT P
dXi
dU
I
-1:
--
1)
-,
OXj
TRANSPORT
EQUATION
FOR
CHEMICAL
SPECIES
Dp'
is
zero for Dt = 0
For
and ideal
For
an ideal
(
dlnV)
-1
Gas
dInT
-
p
In
many domains
of
engineering science investigations
of
fluids with
chemical reactions are required which make it necessary
to
enlarge the
considerations carried out to-date.
It
is
necessary
to
state the basic equations
of
fluid mechanics for the different chemical components:
dpA
• Local modification
of
the mass
of
the
Tt
P
V9\
chemical
component
A.
