Brewster H.D. Fluid Mechanics
Подождите немного. Документ загружается.


112
Basic Equations
of
Fluid Mechanics
from the another. From the derivations resulted the equation in the following
form:
[
D(1
2)]
acpu·)
au·
aC1:
r
u.)
aru.
p _
-u.
= J
+p
__
J _
r.J
J +1:.
~+pg.u.
Dt
2 J
ax ax
.
ax
lj
ax
J J
J J , ,
When
one
sets up
the
equation
for
the
total
energy
balance,
the
consideration stated below results, which starts from the entire internal kinetic
and potential energy
of
a fluid element and considers its evolution as a function
of
time
I:
~(om~
['!'u
J
2
+e+GJ)
=
om~
~[
...
]+[
...
]
dom~
dt 2 dt
dt
For the temporal change
of
the total energy
of
a fluid element results
d
with
om~
= const, d.h. dt
Com~)
=
0:
:t(
8m~
[~u]
+e+G])
=
8m~
~t(~U]
+e+G
)
This is the total energy change which has to be considered concerning
the derivation
of
the total energy equation.
The change
of
the total energy
of
the fluid element can emanate from the
heat conduction, which yields the following inputs minus the discharges
of
heat:
ai]i
--a
8V~
= Energy input per unit time
by
heat conduction can originate
xi
from the convective transport
of
pressure energy and by the input
of
kinetic
energy due to molecular transport into the fluid element:
ai]i
--a
C'tijUj)OV~
= molecular-dependent input
of
kinetic energy
xi
The following total energy balance is thus resulting:
D
[1
2 J ai].
acPU
j
)
aC'tiju
j
)
p8V~-
-U.
+e+G
=--'
8V~
-
8V~
-
8V~
Dt
2 J
aXi
ax
j
aXi'
as 0 V
~
"*
0 follows:
D [ ( 1 2
)]
ai].
aCPU
j
)
aC'tiju
j
)
p-
e+
-U
j
+G
=--'
- - .
Dt
2
ax·
ax·
ax·
,.
J '
When one deducts from this the mechanically derived parts, i.e.
by
subtracting the equation from the equation for the mechanical energy, given
here once again:
p~J~uJ
+G]=
acpu·)
au·
ac't··u.)
au·
_---=:J_
+ p
__
J _
lj
J +
't
..
__
J
aX
j
aX
j
aXi
lj
aX
i
Basic Equations
of
Fluid Mechanics
113
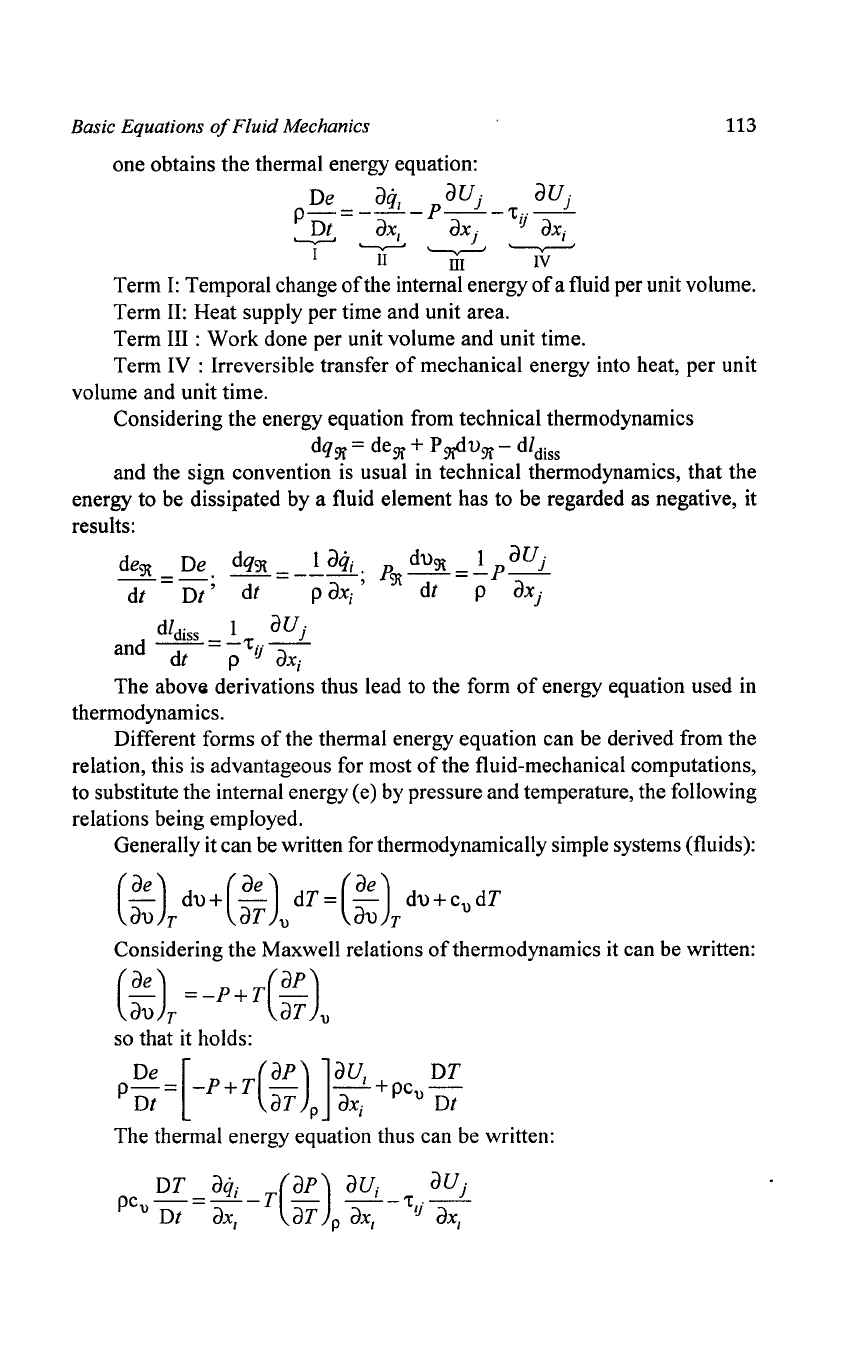
one obtains the thermal energy equation:
De
aq
aUj
aUj
p_
=
__
I _ p
__
_
'tij--
Dt
aX
1
ax
j
ax;
'---,,---'
'---v---'
'--v--'
I
II
'JiI"'
IV
Term
I:
Temporal change
of
the internal energy
of
a fluid per unit volume.
Term
II:
Heat supply per time and unit area.
Term
III:
Work done per unit volume and unit time.
Term IV : Irreversible transfer
of
mechanical energy into heat, per unit
volume and unit time.
Considering the energy equation from technical thermodynamics
dq9i=
de9i+ P9flv
9i
-
d1diss
and the sign convention
is
usual in technical thermodynamics, that the
energy to be dissipated by a fluid element has to be regarded as negative, it
results:
de~
De
dq~
_ 1
aq;
.
Tt=
Dt;
dt
-pax;'
d1diss
-..!.'t
aUj
and dt - p
;j
ax;
The
abov@
derivations thus lead to the form
of
energy equation used in
thermodynamics.
Different forms
of
the thermal energy equation can be derived from the
relation, this is advantageous for most
of
the fluid-mechanical computations,
to substitute the internal energy (e) by pressure and temperature, the following
relations being employed.
Generally it can
be
written for thermodynamically simple systems (fluids):
(
ae)
dV+(~)
dT=(aae)
du+cudT
au T
aT
u u T
Considering the Maxwell relations
of
thermodynamics it can be written:
(
ae)
=_p+T(ap)
au T
aT
u
so that it holds:
pDe
=[_p+T(ap)
]aU
1
+pc
u
DT
Dt
aT
pax;
Dt
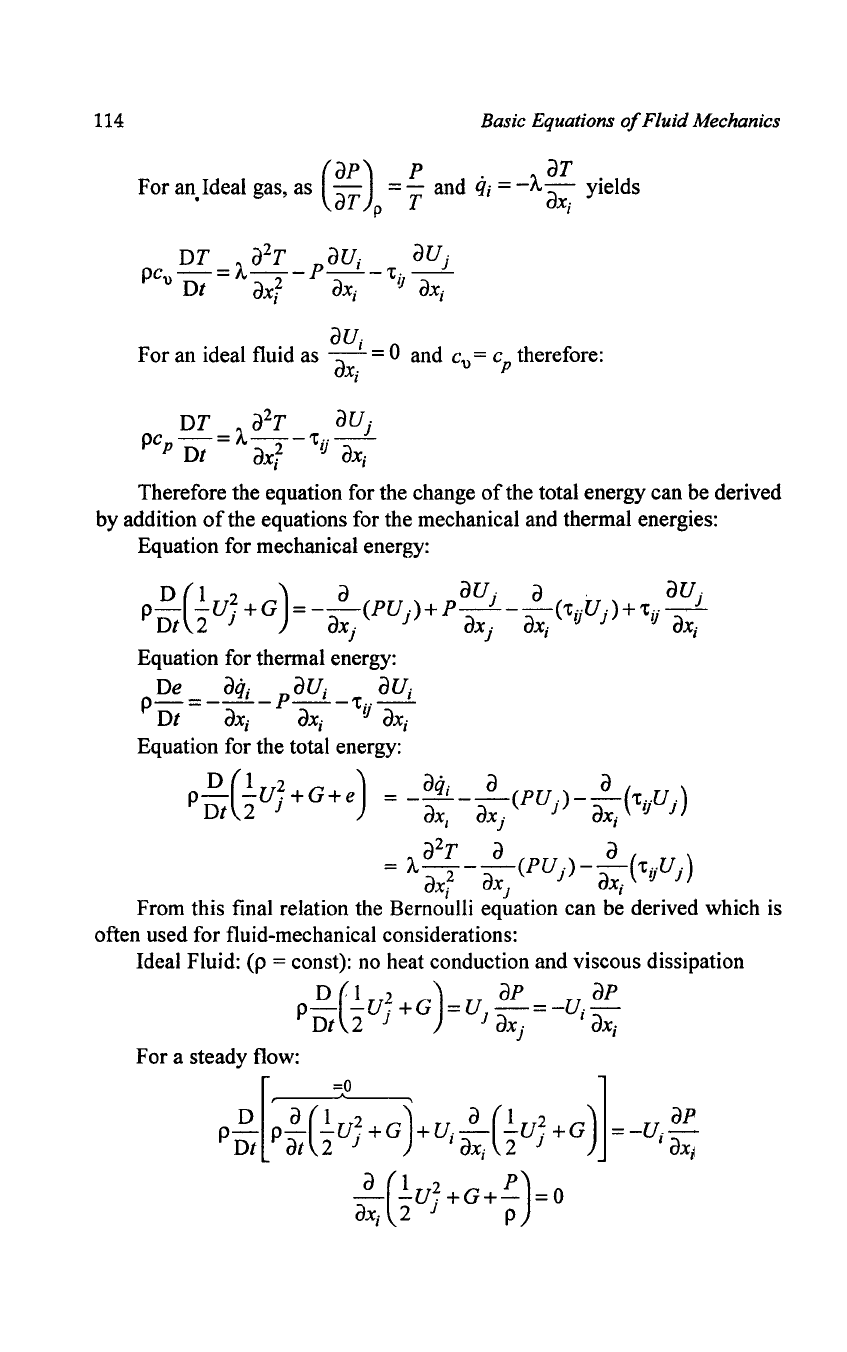
The thermal energy equation thus can be written:
114
Basic Equations
of
Fluid Mechanics
(
ap)
p .
aT
For
an,Ideal gas, as
-a
= - and
q;
=
-A-a
. yields
T p T X,
au·
For
an
ideal fluid as
ax.'
= 0 and C
u
= c
p
therefore:
I
DT
a
2
T
aU
j
pCp
Dt = A
axl
-'tij
ax;
Therefore the equation for the change
of
the total energy can be derived
by addition
of
the equations for the mechanical and thermal energies:
Equation for mechanical energy:
D ( 1 2 ) a
aU
j
a·
aU
j
p-
-U.
+G
=--(PU·)+P---('t··U.)+'t··-
Dt 2 J
ax.
J
ax.
ax.
1J
J
1J
ax.
J J I I
Equation for thermal energy:
pDe
=_
ag;
_pau;
-'t
..
au;
Dt
ax;
ax;
1J
ax;
Equation for the total energy:
D
(1
2 )
ag.
a a
p-
-U
j
+G+e
=
--'
--(PU
.)--('t
..
U.)
Dt
2,
ax
ax
. J
ax.
1J
J
I J I
a
2
T a a
=
A---(PU.)--('t
..
U.)
a
~
ax
J
ax.
1J
J
:.
x,
J I
From this final relation the Bernoulli equation can be derived which is
often used for fluid-mechanical considerations:
Ideal Fluid:
(p
= const): no heat conduction and viscous dissipation
~(!U~+G)=U
ap
=-U.
ap
PDt 2 J J
ax·
'
ax.
J I
For a steady flow:
[
=0 ]
, A ,
D a 1 2 a 1 2
ap
P-
p-(-U.
+G)+U.-(-U.
+G)
=-U·-
Dt
at
2 J I
ax;
2 J I
ax,
~(!U~+G+
P)=o
ax;
2 J P
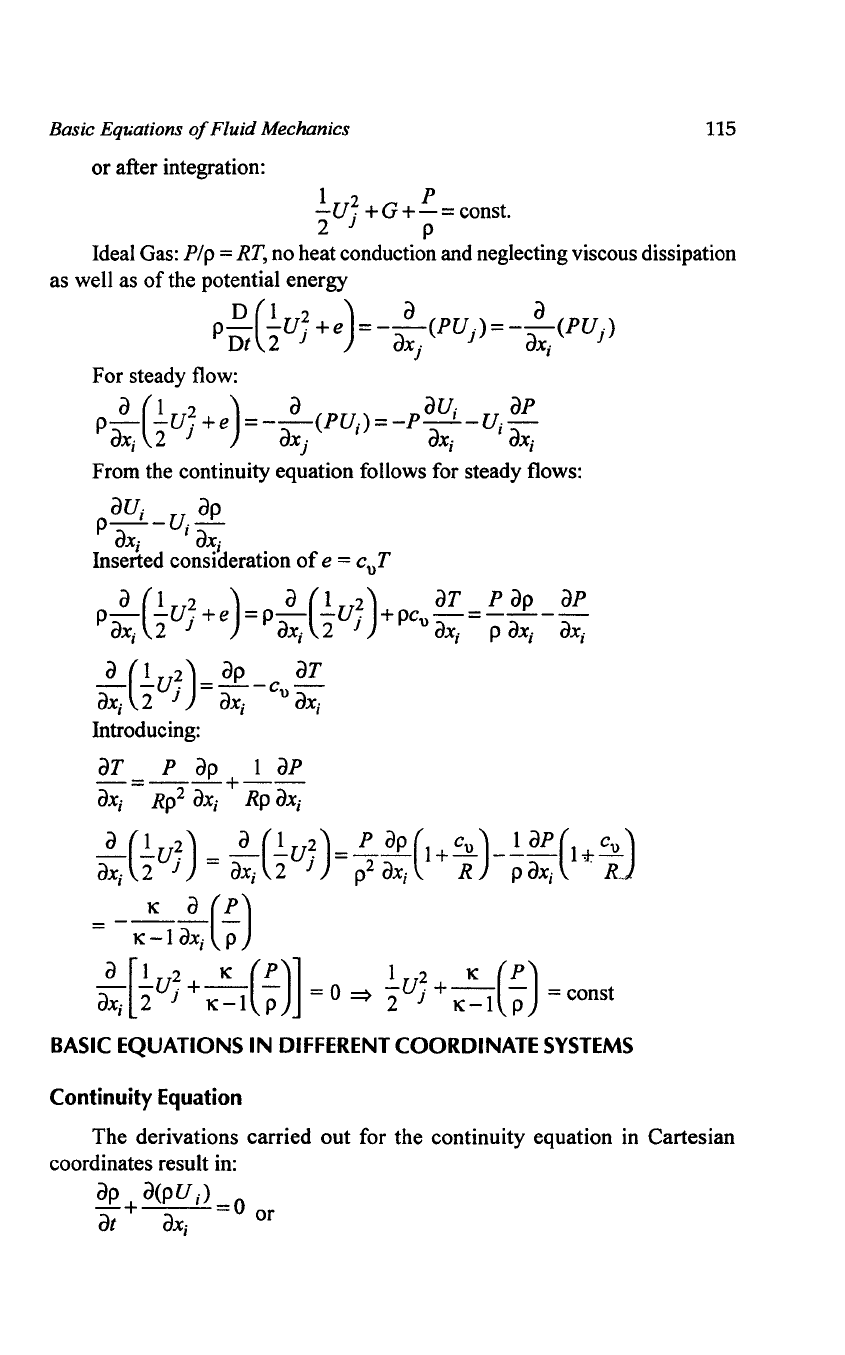
Basic Eql4ations
of
Fluid Mechanics
or after integration:
1 2 P
-U
j
+G+-=
const.
2 P
115
Ideal Gas:
Pip
=
RT,
no heat conduction and neglecting viscous dissipation
as well as
of
the potential energy
D(l
2 ) a a
PDt
"2
Uj
+e
=-ax.
(PUj)=-ax.
(PU
j
)
J I
For steady flow:
a
(1
2 ) a
au; ap
p-
-U·
+e
=--(PU)=-P--U.-
ax;
2 J ax j I
ax;
I
ax;
From the continuity equation follows for steady flows:
pau;
-U~
ax·
I
ax.
Inserted consiaeration
of
e =
cuT
p~(.!.u~
+
e)
=
p~(.!.u~)
+ pc
aT
= P ap _
ap
ax;
2 J
ax;
2 J u
ax;
p
ax; ax;
~(.!.u~)=~-c
aT
ax;
2 J
ax;
u
ax;
Introducing:
aT
=~~+_1
ap
ax;
Rp2
ax;
Rp
ax;
~(.!.u~)
=
~(.!.u~)
=
.!..~(1
+ C
u
)
_.!.
ap
(1
-+
C
u
)
ax;
2 J
ax;
2 J
p2
ax;
R pax;
£j
= -
K~la~J:)
a
[1
u2
K
(P)]
.!.U~
+~(P)
_
ax;
"2
j + K
-1
P = 0
~
2 J K
-1
P - const
BASIC
EQUATIONS
IN
DIFFERENT
COORDINATE
SYSTEMS
Continuity
Equation
The derivations carried out for the continuity equation in Cartesian
coordinates result in:
ap + a(p U
;)
= 0
at
ax;
or
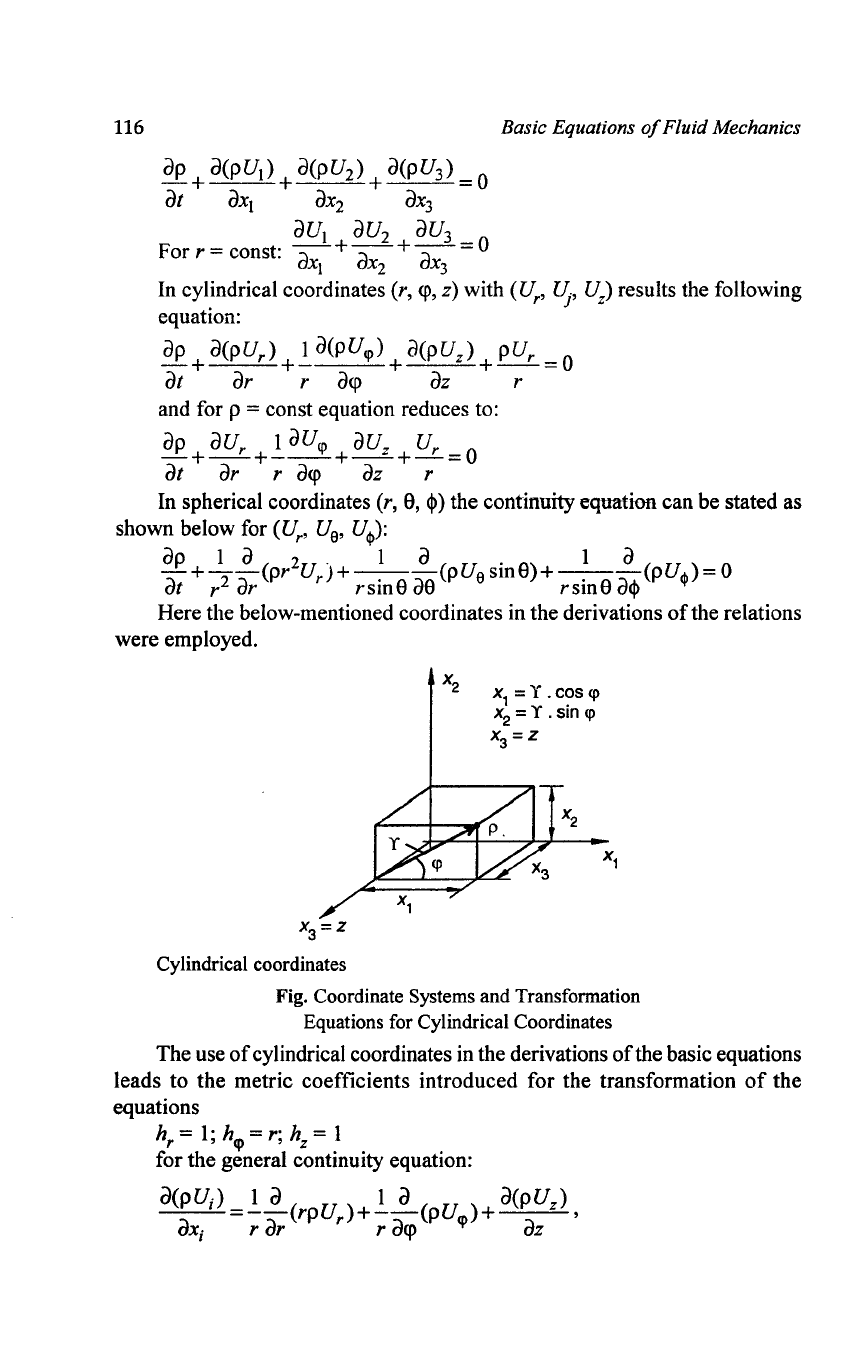
116
ap
+
a(pU
I
)
+
a(pU
2
)
+
a(pU
3
)
= 0
at
aXI
aX2
aX3
aU
I
aU
2
aU
3
Forr=
const:
-a
+-a
+-a-=O
xl
X2
x3
Basic Equations
of
Fluid Mechanics
In cylindrical coordinates (r,
<p,
z) with
(U
r
,
~,
U
z
)
results the following
equation:
ap
+
a(pU
r
)
+ I a(puq» +
a(pU
z
)
+ pUr = 0
at
ar
r
a<p
az r
and for p
= const equation reduces to:
ap
+ aUr
+.!..
aUq>
+
au
z + Ur
=0
at
ar
r
a<p
az
r
In spherical coordinates
(r,
S,
~)
the continuity equation can be stated as
shown below for
(U
r
, U
a
'
Ucp):
ap
1 a
2.
I a . 1 a
-+--(pr
U
r
)+-.
--(pUasmS)+-.
--(pUcp)
= 0
at
r2
ar
rsmS
as
rsmS
acj>
Here the below-mentioned coordinates in the derivations
of
the relations
were employed.
X3=Z
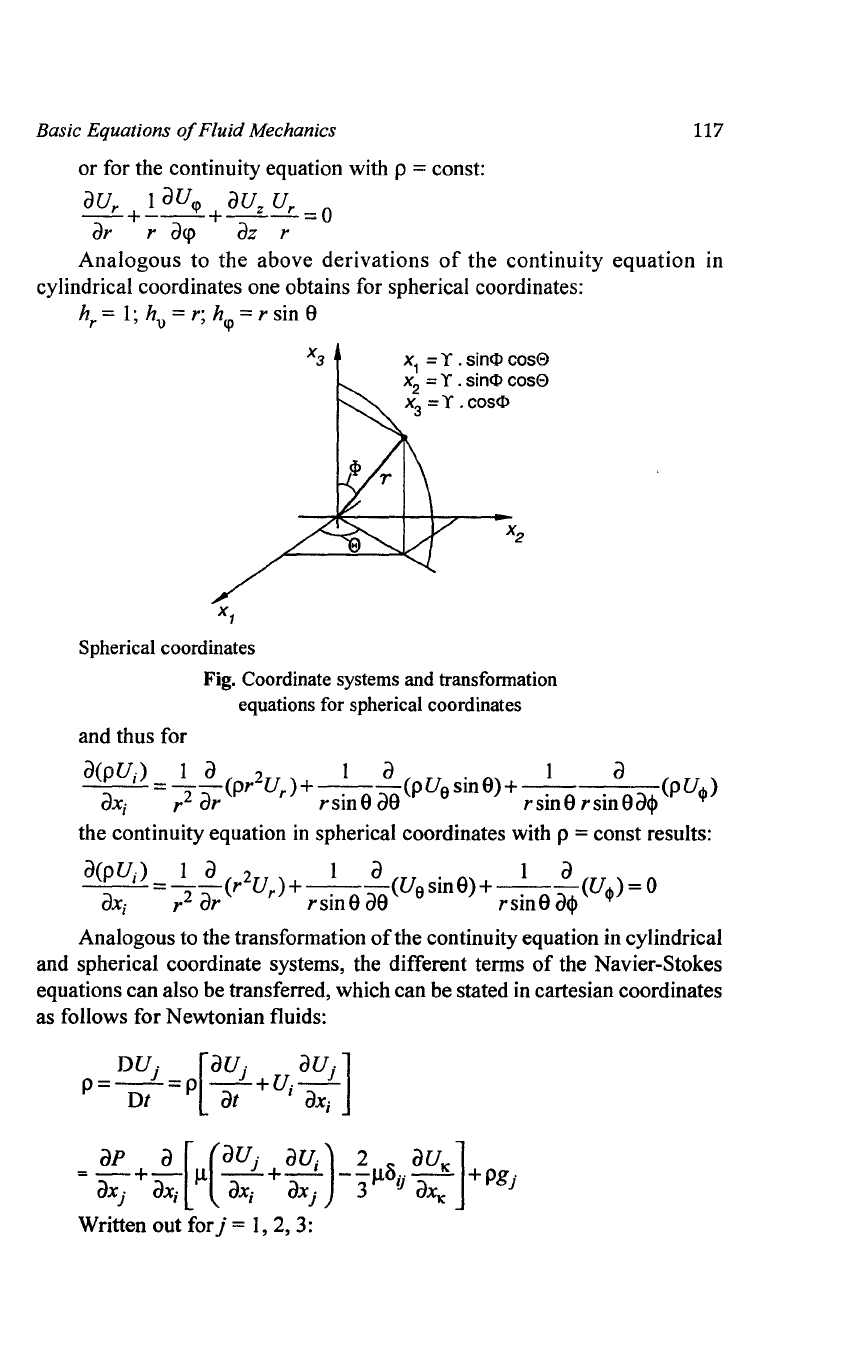
Cylindrical coordinates
X
1
= Y .
C?S
<p
x
2
=
Y.
sin
<p
x3=z
Fig. Coordinate Systems and Transformation
Equations for Cylindrical Coordinates
The use
of
cylindrical coordinates in the derivations
of
the basic equations
leads
to
the metric coefficients introduced for the transformation
of
the
equations
hr
=
I;
hq>
=
r;
h
z
= I
for the general continuity equation:
a(pU;)
L.~-(rPUr)+.!..~(PU)+
a(pU
z
)
ax; r
ar
r
a<p
q>
az
Basic Equations
of
Fluid Mechanics
117
or for the continuity equation with p = const:
aU
r
+.!.
aUf{>
+
au
z
U
r
:::::
0
ar r
aq>
az r
Analogous to the above derivations
of
the continuity
equation
in
cylindrical coordinates one obtains for spherical coordinates:
h =
l'
h = r h = r sin S
r
'1>
'cp
X3
Xl
= Y .
sinet>
cose
x
2
= Y .
sinet>
cose
x3
= Y .
cos<1>
X
t
Spherical coordinates
and thus for
Fig. Coordinate systems and transformation
equations for spherical coordinates
a(pu.)
1 a 2 1 a . 1 a
a 1
=2''';-(pr
U
r
)+-.
--;-(pUesmS)+.
. a
(pUcp)
xi r
or
rsmS
oS
rsmS
rsmS
<I>
the continuity equation
in
spherical coordinates with p = const results:
a(p
U
i
) 1 a 2 1 a . 1 a
aXi
= r2
a/
r
U
r
)+
rsinS
as
(UesmS)+
rsinS
a<l>
(Ucp)=O
Analogous to the transformation
of
the continuity equation in cylindrical
and spherical coordinate systems, the different terms
of
the Navier-Stokes
equations can also be transferred, which can be stated in cartesian coordinates
as follows for Newtonian fluids:
p=
__
l
=p
__
1 +u.
__
1
DU·
[au.
au.]
Dt
at
1
ax;
ap
a
[(aU
j
au.
) 2
au]
=
-+-
J.1
__
+
__
1 --J.10ij
__
lC
+pgj
aXj
aXi aXi
aXj 3
a~
Written out for j =
1,2,3:
118
Basic Equations
of
Fluid Mechanics
DU
3
ap
a
[(aU
3
au
l
)]
a
[(aU
3
au
2
)]
Plli=-
aX3
+
aXI
~
aXI
+
aX3
+
aX2
~
aX2
+
aX3
+~[2~
aU
3
-~~(V.U)]+pg3
aX3
aX3
3
_
aUK·
where it holds
V.U
=
-a-
X
K
• Momentum Equations in Cartesian Coordinates - Momentum
equations with
'eij -terms:
xl
-Component:
(
aU
I
U
aU
I
u
aU
I
u
au
l)
p
-+
1-+
2--+
3-
at
aXI
aX2
aX3
ap
(ar
ll
ar21
ar31)
=---
-+-+-
+pgl
aXI aXI
a~
aX3'
Basic Equations
of
Fluid Mechanics
• Navier-Stokes equations for
rand
11
equally constant:
xI
-Component:
(
aU
I
U
aU
I U
aU
I
U
au
l
)
p
-+
1-+
2-+
3-
at
aXI
aX2
aX3
ap
(a
2
U
1
a
2
u
I
a
2
U
1
)
=--
+11
-2-+-2-+-2-
+pgl
aXI
aXI
aX2
aX3
119
• Momentum Equations in Cylindrical Coordinates - Momentum
equations with
T.ij
-terms:
r -Component:
p(au
r
+U
aU
r
+
U~
aU
r
_
U~
+U
au
r
)=_
ap
at
r
ar
r
aq>
r z
az
ar
(
1 a 1
arr~
rqxp
rqxp
ar.)
-
__
("")+
______
+-2!..
+pgr
rar
raq>
r r
az
<p--Component:
(
au~
au~
U~
au~
UrU~
au~)
p
--+U
--+---+--+U
--
at
r
ar
r
aq>
r z
az
1
ap
(1
a 2 1
ar
qxp
ar
~
)
=
----
--(r
rr
)+---+-
+pg
r
aq>
r2
ar
~
r
aq>
az
~
z-Component:
(
au
z
u
au
z
U~
au
z
u
au
z
)
p
--+
--+---+
--
at
r
ar
r
aq>
Z
az
120
Basic Equations
of
Fluid Mechanics
ap
(1
a 1
ar<pz
ar~z
)
=---
--(rrrz)+--·-+-~
+pgz
az r
ar
r
acp
az
Navier-Stokes equations for p
and
~
equally constant:
p-Component:
p(au
r
+U
aUr +
U<p
aUr
_
U~
+U
aUr]
at r
ar
r
acp
r z a
z
ap
[a(la
) 1 a
2
u
r
2
au
<p
a
2
u
r
]
=
--+/1
-
--(rU
r
)
+-+------+--
+pgr
ar
ar
r
ar
r2
acp2:
r2
acp
az
2
<j>-Component:
(
au<p au<p
U<p
au<p
UrU<p
au
z
)
p
--+U
--+---+--+U
--
at
r
ar
r
acp
r z a
z
'
lap
[a(l
a ) 1 a
2
ucp
2
au
a
2
ucp]
---+/1
-
--(rU
) +
___
+
___
r
+--
+pg
r
acp
ar
r
ar
<p
r2
acp2
r2
acp
az
2
cp
z-Component:
p(au
z
+U
au
z
+
Ucp
au
z
+U
au
z
)
at r
ar
r
acp
z a
z
ap
[1 a
(au
z
) 1 a
2
u
z
a
2
u
z
]
=
--+/1
--
r--
+---+--
+pgz
az r
ar
ar
r2
acp2
az
2
• Momentum Equations
in
Spherical Coodinates
Momentum equations with
'tij
-terms
p(au
r
+U
aUr + U
e
aUr
+~
aUr
_
U~
+U~]
at
r
ar
r
ae
r sin e
a<j>
r
ap
(1
a 2 1 a .
=---
--(r't
)+---('t
esme)
ar
r2
ar
rr
rsine
ae
r
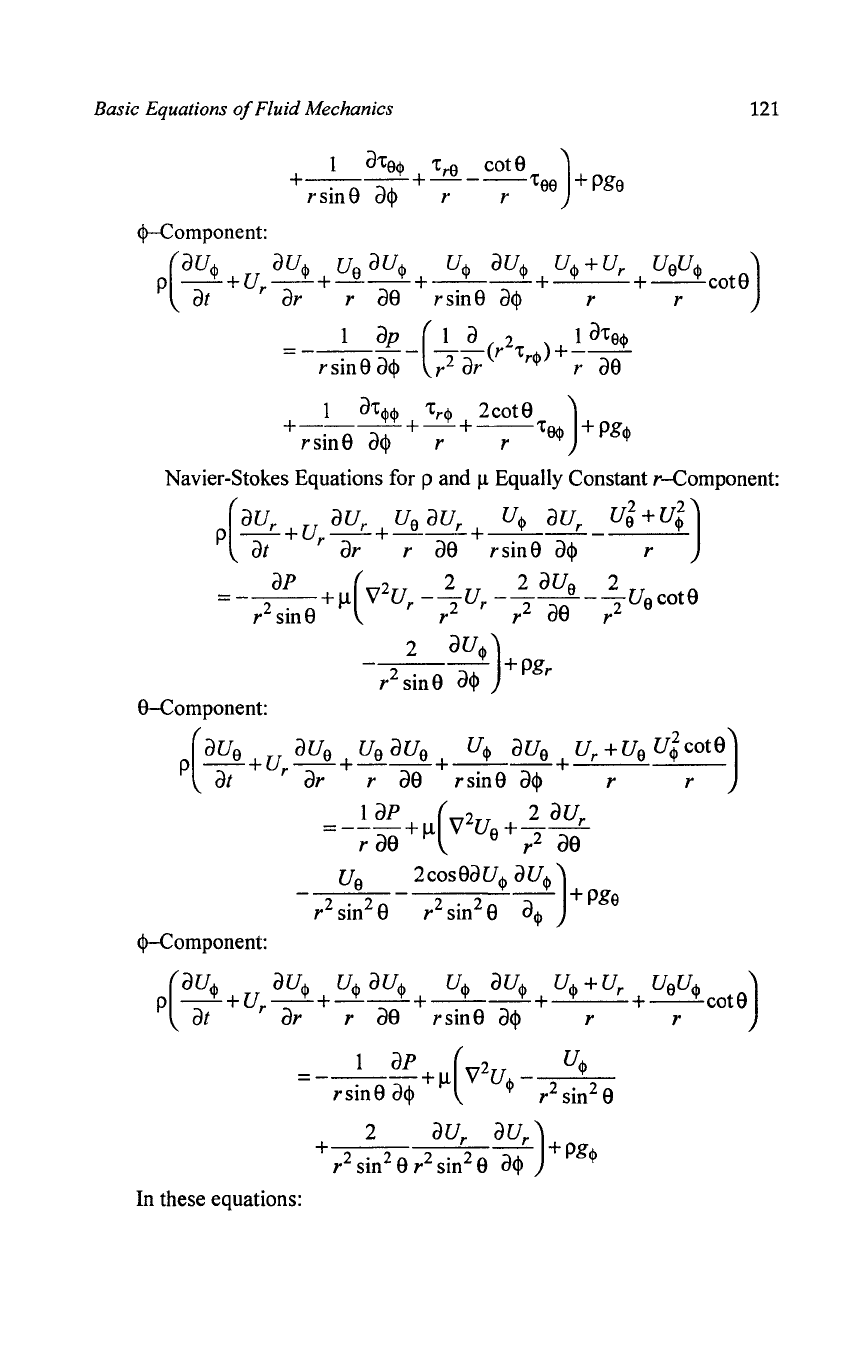
e-Component:
1 a't
r<j)
'tee
+ 'tee)
+-----
+pg
rsine
a<j>
r r
(
aU
e
aUe U
e
aUe
U<p
aUe
U
r
+ U
e
U~
cote]
p
--+U
--+---+----+
---'---
at
r
ar
r
ae
r
sin
e
a<j>
r r
1
ap
(1
a 2 1 a .
=----
--(r'tre)+-.--('tee
sme
)
rae
r2
ar
rsme
ae
Basic Equations
of
Fluid Mechanics
121
1
a'taq,
't
ra
cot S )
+-.---+----'t
aa
+pga
rsmS
a<l>
r r
<!>-Component:
(
auq,
auq,
U
a
auq,
Uq,
auq,
Uq,
+ U
r
UaUq,
)
p
--+U
--+---+----+
+--cotS
at r
ar
r
as
r sin S
a<l>
r r
=
__
1_
ap
_
(_1
~(r2't
q,)
+.!.
a'taq,
rsinS
a<l>
r2 ar r r
ae
1
a'tq,q,
'trq,
2cotS )
+-.---+-+--'t9<P
+pgq,
rsmS
a<l>
r r
Navier-Stokes Equations for p and
p.
Equally Constant r-Component:
p(au
r
+U
aU
r
+ U
e
aU
r
+!!..!L
aU
r
_
U~
+U~J
at
r
ar
r
ae
r sin e
a<l>
r
ap
(2
2 2
aUe
2
-::---+Jl
V U
--U
------UecotS
r2 sin e r r2 r r2
as
r2
=
2 auq,)
r2
sine
a<l>
+
pgr
<!>-Component:
(
auq, auq,
Uq,
auq,
Uq,
auq,
Uq,
+ U
r
UeUq,
)
p
--+U
--+---+----+
+--cotS
at
r
ar
r
as
rsinS
a<l>
r r
=
__
1_
ap
+
Jl(V
2
U _
Uq,
rsine
a<l>
q,
r2 sin
2
e
2
aU
r
au
r
)
+ r2 sin
2
S r2 sin
2
S
a<l>
+
pgq,
In
these equations:
