Brewster H.D. Fluid Mechanics
Подождите немного. Документ загружается.

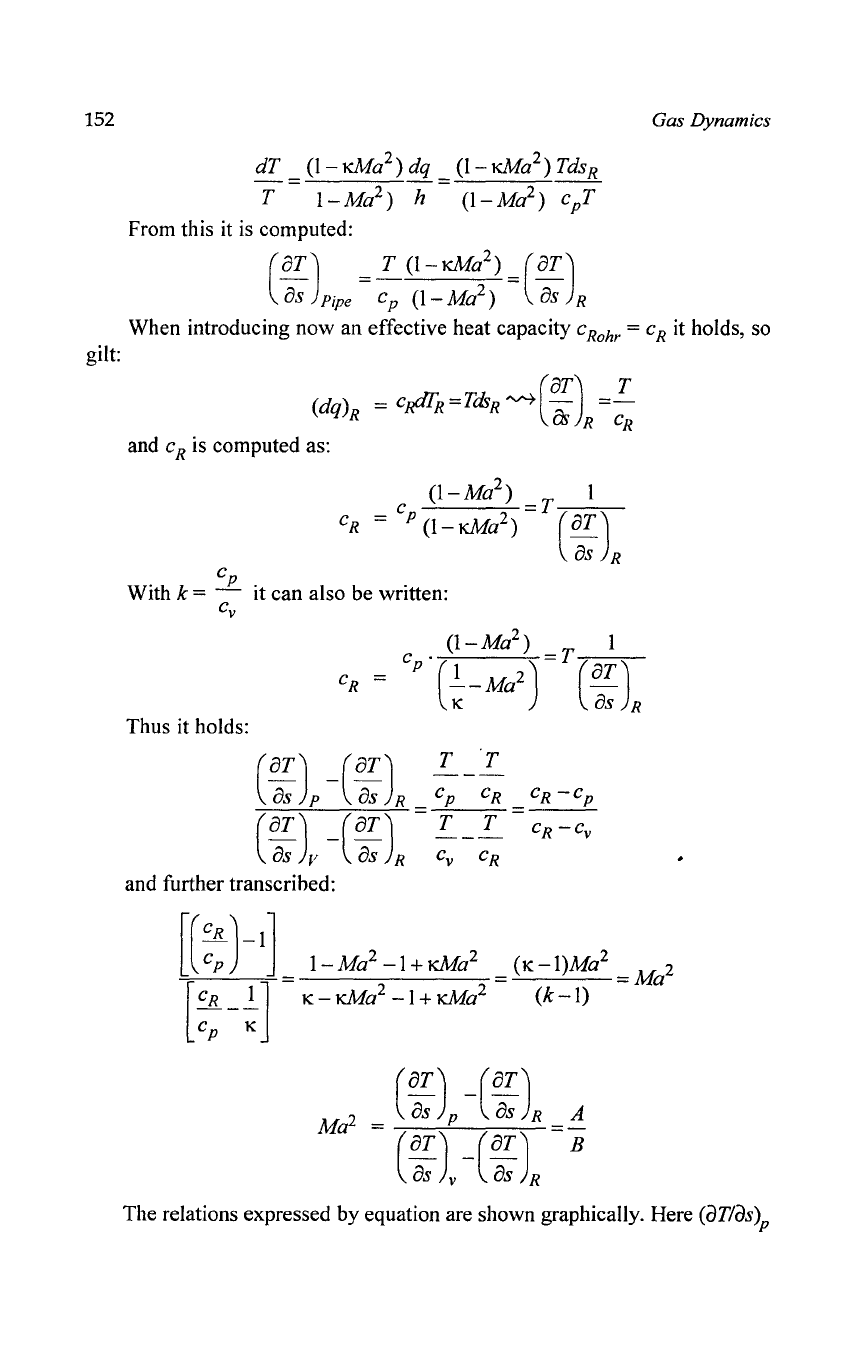
152
dT
(1-
KMa
2
)
dq
(1-
KMa
2
)
Tds
R
T=
I-Ma
2
)
h =
(I-Ma
2
)
cpT
From this it
is
computed:
(
aT)
T
(1-
KMa
2
)
(aT)
as
Pipe = C
p
(I-Ma
2
)
=
8;
R
Gas Dynamics
When introducing now an effective heat capacity c
Rohr
= c
R
it holds, so
gilt:
and
C R is computed as:
C
p
With k = - it can also be written:
C
v
Thus it holds:
(~)p
-(~)R
(~:l-(~:)R
and further transcrihed:
=
T T
---
c
p
cR
T
T
---
C
v
CR
CR
-c
p
CR
-C
v
The relations expressed by equation are shown graphically. Here caTlas)p
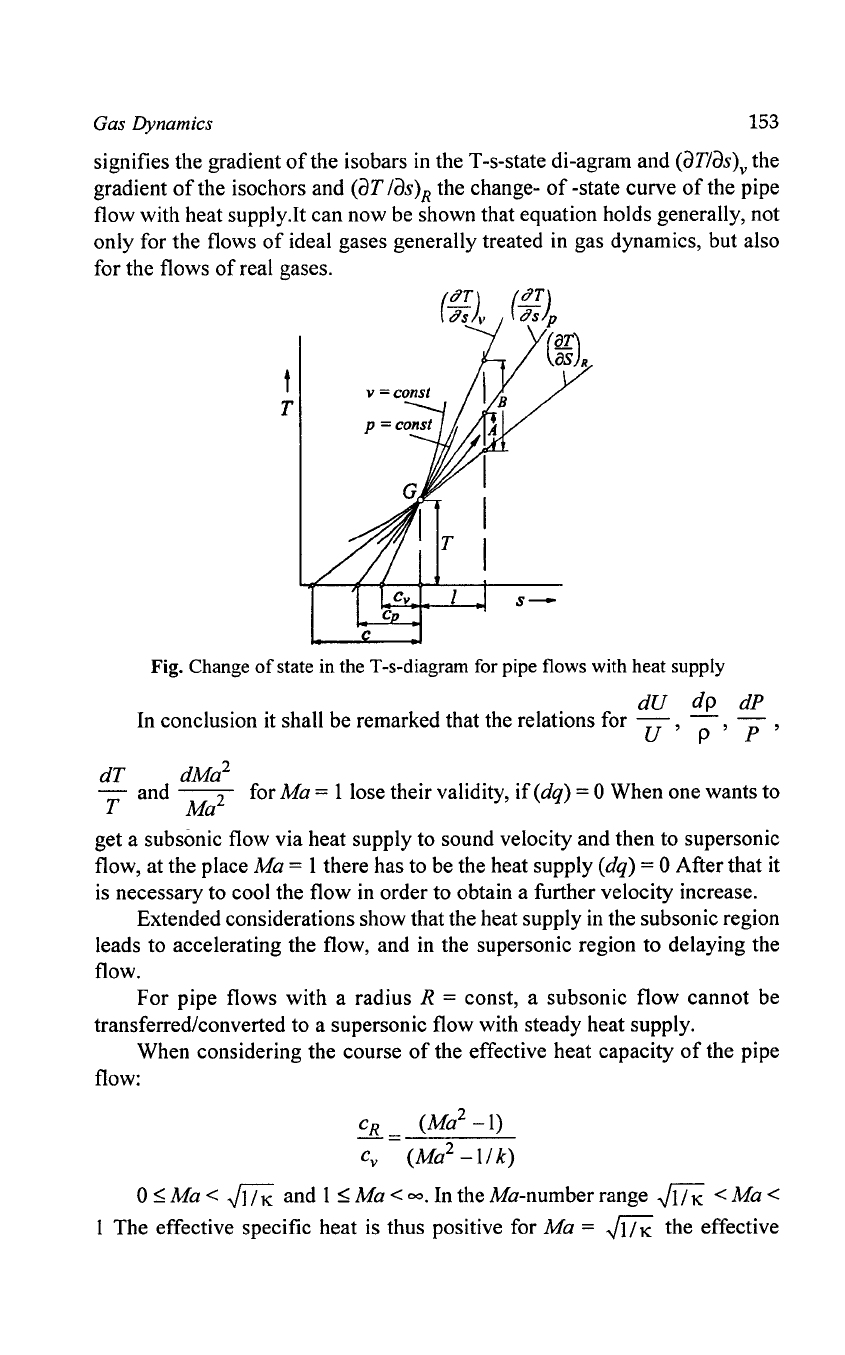
Gas Dynamics
153
signifies the gradient
of
the isobars
in
the T-s-state di-agram and
(aTlas)v
the
gradient
of
the isochors and (aT
/as)R
the change-
of
-state curve
of
the pipe
flow with heat supply.!t can now be shown that equation holds generally, not
only for the flows
of
ideal gases generally treated in gas dynamics, but also
for the flows
of
real gases.
Fig. Change
of
state in the T-s-diagram for pipe flows with heat supply
dU
dp
dP
In
conclusion it shall be remarked that the relations for
-U
'
-,
- ,
p P
dT
dMa
2
T and Ma
2
forMa = 1 lose their validity,
if
(dq) = 0 When one wants to
get a subsonic flow via heat supply to sound velocity and then to supersonic
flow, at the place Ma
= 1 there has to be the heat supply (dq) = 0 After that it
is
necessary to cool the flow in order to obtain a further velocity increase.
Extended considerations show that the heat supply
in
the subsonic region
leads to accelerating the flow, and
in
the supersonic region to delaying the
flow.
For pipe flows with a radius
R = const, a subsonic flow cannot be
transferred/converted to a supersonic flow with steady heat supply.
When considering the course
of
the effective heat capacity
of
the pipe
flow:
CR (Ma
2
-1)
~=
(Ma
2
-11k)
o
~
Ma < J]i";. and 1
~
Ma <
00.
In the Ma-number range J]i";. < Ma <
1 The effective specific heat is thus positive for Ma = J]i";. the effective

154
Gas Dynamics
heat capacity is negative. At c
R
~
00.
tends to go to infinity. At
Ma
= .JIIK
the local flow velocity has the value
of
the isothermal sound velocity. Because
of
the relation for the effective heat capacity
cJc
v
for the developments
of
subsonic
and
supersonic
flows in
heated
and
cooled
pipe
flows
the
thermodynamic state behaviours, at the same thermo-dynamical initial state.
Starting from state
A, one gets to state C by heating and after that by cooling
up to state
B,
where a supersonic flow predominates. When on the other hand
heating up at supersonic conditions, one gets from state point A to C, and
after that by cooling into state point
B, where a subsonic flow predominates.
Chapter 6
Hydrostatics
and
Aerostatics
HYDROSTATICS
Hydrostatics deals with the laws to which fluids are subjected that do not
show motions in hydrostatic coordinate system in which the considerations
are carried out, i.e. fluids which are at rest in the coordinate system employed
for the considerations. General laws
of
fluid motions they are also applicable
to the case
of
fluids at rest, i.e. non-flowing fluids. Thus from the continuity
equation
ap
+~(pU;)=
0
at
ax;
It
can be shown that for p = const and
U;"*
j{x;) the continuity equation is
given by:
ap
+U
j
ap
+p
au;
=0.
at
ax;
aXj
'----v----'
~
DplDt=O
=0
This means that for
U;
= 0 the simple partial differential equation holds:
ap = 0
at
whose general solution can be stated as follows:
p =
F(x).
The density p in a fluid
at
rest thus is only a function
of
the
spatial
coordinates
xi"
When time variations
of
the density
of
the fluid occur, these
lead inevitably to motions within the fluid because
of
the relation between the
flow and density fields attributable to the continuity equation.
The general equations
of
momentum can be expressed as:
]
U ] - ] I)
[
au.
au.]
au·
a't··
P
--+
;--
------+pgj
at
ax; ax; ax;
and
its
special
form
is
deduced
for
a
fluid
at
rest
(~
= 0
and
156
Hydrostatics and Aerostatics
d't
.
moleculardependent
momentum
transport
:I
lj
= 0)
to
the
following
OX,
system
of
partial 1606 Hydrostatics
and
Aerostatics differential equations.
This represents the set
of
basic equations
of
hydrostatics
and
aerostatics:
dP
dx
=pgJ(j=
1,2,3)
J
or
written
out
for all three directions:
dP
dP
ap
-;-=pgj,
;-=pg2'
;-=pg3·
OXj
OX2
oX3
In
this section the pressure distribution in a fluid, mainly defined by the
field
of
gravity, will
be
considered
more
closely. Restrictions are
made
concerning
the
possible
fluid
properties;
the
fluid is
assumed
to
be
incompressible
for
hydrostatics, i.e p = const. This condition
is
in general
quite
well
fulfilled by liquid, so that the following derivations can be considered
as valid for liquids.
For
the derivation
of
the pressure distribution in liquid
at
rest a rectangular
Cartesian coordinate system
is
introduced, whose position
is
chosen such that
the
mass acceleration
{g)
given by the field
of
gravity only shows one
component in the negative
x
2
direction, i.e. the following vector holds
{gi}
=
{O,
- g,
O}.
Then
the
differential equations given above generally
for
the pressure
can be written as follows:
dP
=0
aXj ,
dP
ap
-=-pg,
-=0.
aX2
dX3
Fig. Coordinate system for the derivation
of
the pressure distribution in fluids
From
dPldx
j
= 0 follows P =
!(x2
,x
3
)
and
from
dP
Idx
3
= 0 follows
P =
!(x
j
,
x
2
).
Thus
a comparison yields P =
!(x
2
)
and
this shows
that
the
pressure
of
a fluid within a plane
is
constant when the same
is
vertical
to
the
direction
of
the field
of
gravity. The free surface
of
a fluid stored in a container
is
a plane
of
constant pressure
and
all planes parallel to it are also planes
of
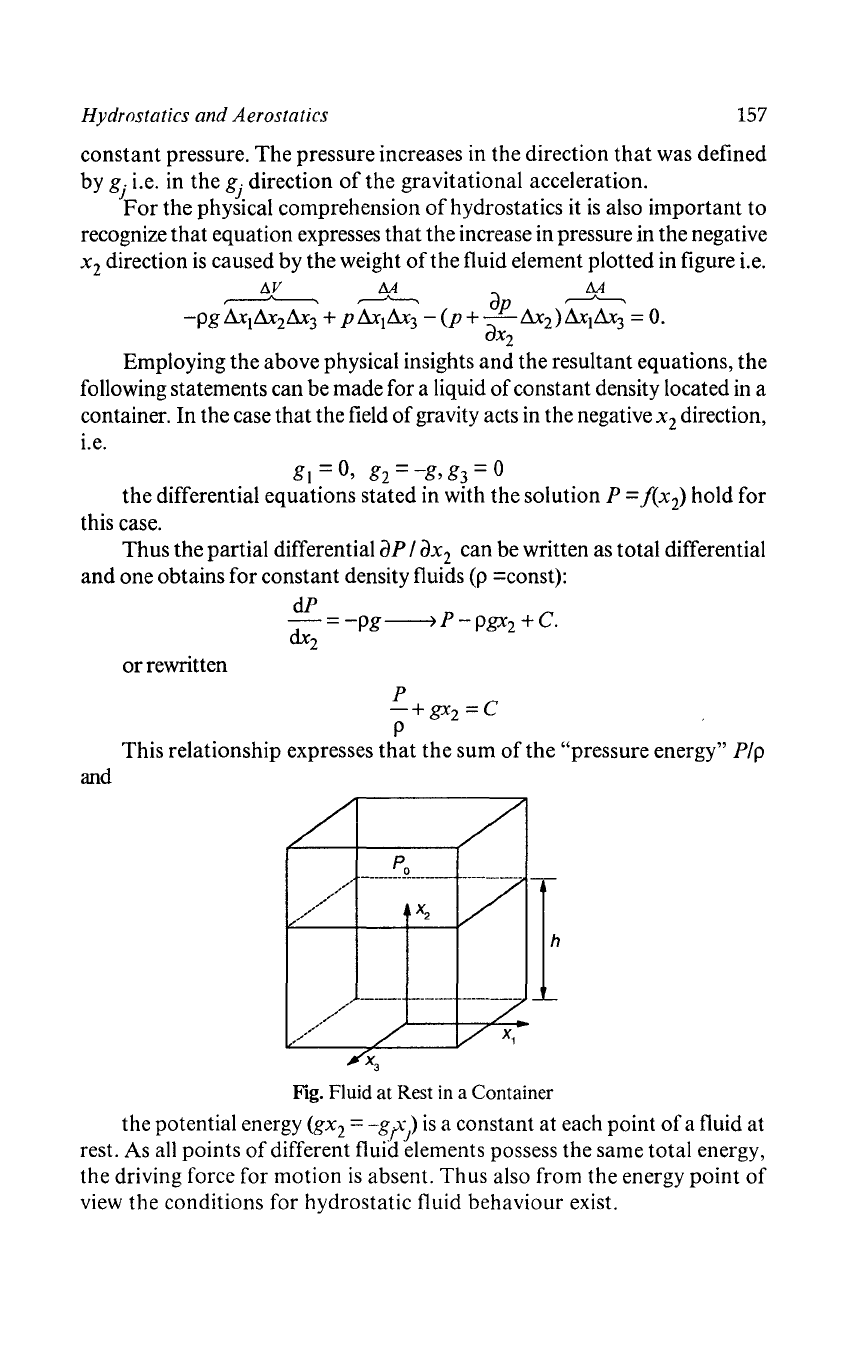
Hydrostatics and Aerostatics
157
constant
pressure.
The
pressure increases in the direction
that
was defined
by
g. i.e. in
the
gj
direction
of
the
gravitational acceleration.
lFor
the physical comprehension
of
hydrostatics it
is
also
important
to
recognize
that
equation expresses
that
the increase in pressure in the negative
x
2
direction
is
caused by the weight
of
the
fluid element plotted in figure i.e.
L'1V
M
~
M
~
r--"---,
op
r--"---,
-pg
&(&2&3
+ P
&(&3
-
(p
+
-&2)&(&3
=
O.
aX2
Employing the above physical insights
and
the resultant equations, the
following statements can be made for a liquid
of
constant density located in a
container. In the case
that
the field
of
gravity acts in the negative x
2
direction,
i.e.
gl
= 0, g2 =
-g,
g3 = 0
the
differential equations stated in with the solution P =
!(x
2
)
hold for
this case.
Thus
the
partial differential
ap
I
aX
2
can be written as total differential
and
one obtains for constant density fluids (p =const):
or
rewritten
dP
-=-pg~P-Pgx2
+c.
dx
2
P
-+gx2
=c
p
This relationship expresses
that
the
sum
of
the
"pressure energy" Pip
and
Fig. Fluid
at
Rest in a Container
the potential energy
(gx2
=
-g;)
is
a constant at each point
of
a fluid at
rest. As all points
of
different fluid elements possess the same
total
energy,
the
driving force for motion
is
absent.
Thus
also from the energy
point
of
view the conditions for hydrostatic fluid behaviour exist.
158
Hydrostatics and Aerostatics
When
the
fluid in
the
height h has a free surface
on
which
an
equally
large pressure
Po
acts
at
all points, it represents, because
of
the
relation
P =
!(x
2
),
a plane x
2
= const, i.e. a horizontal plane.
For
the pressure distribution one obtains with the
boundary
c9Hdition
P
=
Po
for x
2
= h
'V-t
C =
Po
+ pgh
P
=Po+pg(h-x2)0~x2~h.
This relationship expresses the known hydrostatic law, according to which
the
pressure in a fluid increases in a linear way with the depth below the free
surface.
When one rewrites equation one obtains:
Po
P
- +
gh
= - +
gx2
= const
p p
b
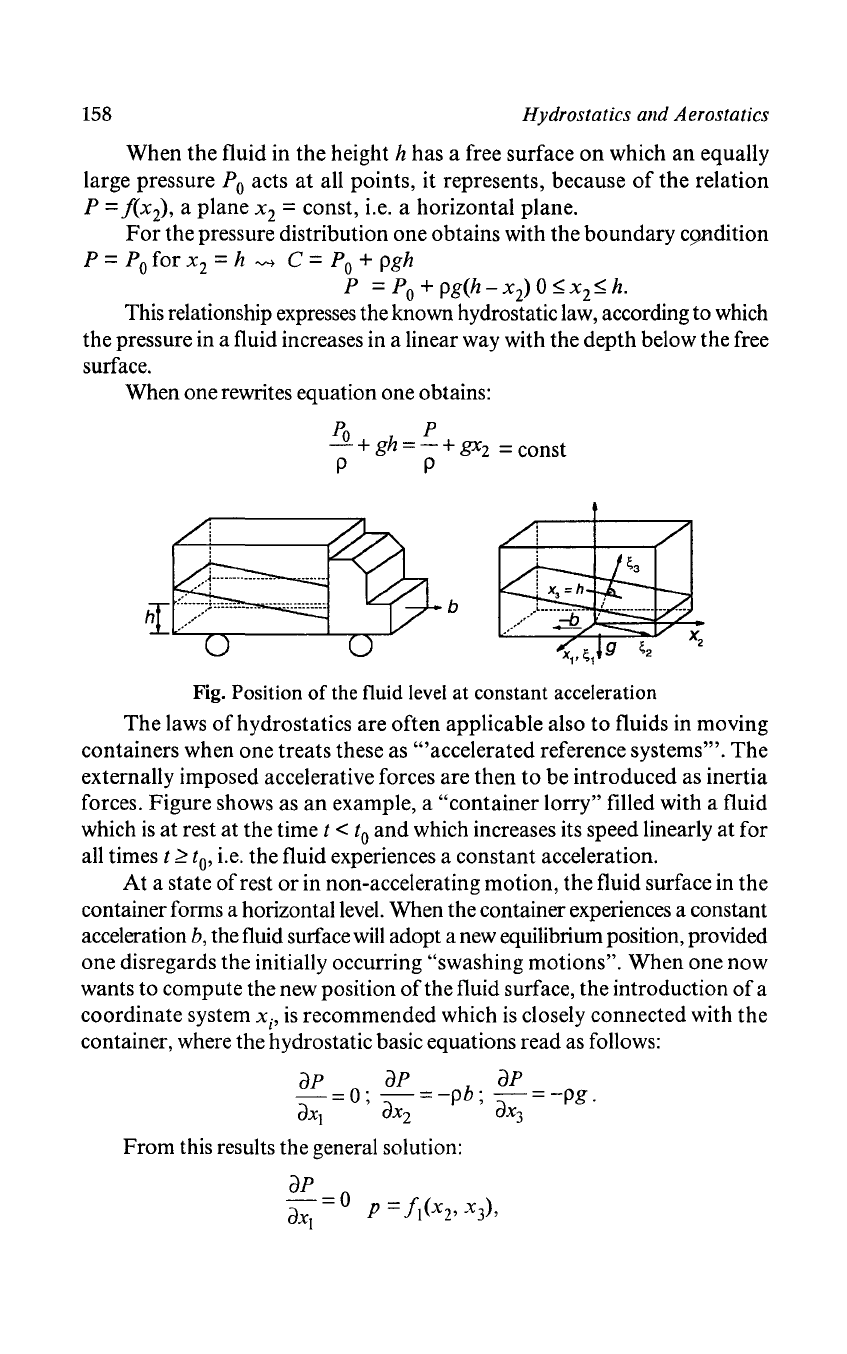
Fig. Position
of
the fluid level at constant acceleration
The
laws
of
hydrostatics are often applicable also
to
fluids in moving
containers when one treats these as
"'accelerated reference systems'''.
The
externally imposed accelerative forces are
then
to
be
introduced as inertia
forces. Figure shows as
an
example, a
"container
lorry" filled with a fluid
which
is
at
rest at the time t <
to
and
which increases its speed linearly at for
all times
t
~
to'
i.e. the fluid experiences a constant acceleration.
At
a state
of
rest
or
in non-accelerating motion, the fluid surface in the
container forms a horizontal level. When the container experiences a constant
acceleration
b,
the fluid surface
will
adopt a new equilibrium position, provided
one disregards the initially occurring
"swashing motions".
When
one now
wants to compute the new position
of
the fluid surface, the introduction
of
a
coordinate system
Xi'
is
recommended which
is
closely connected with
the
container, where the hydrostatic basic equations read as follows:
dP dP dP
-=0;
-=-pb;
-=-pg.
dX}
dX2
dX3
From
this results the general solution:
dP
=0
dX}
P =
!}(x
2
'
x
3
),

Hydrostatics and Aerostatics
159
ap
aX3
=
-pg
p =
-pgx3
+ 13
(X
I'
x2)·
By comparing the solutions one obtains
that/l,J2J3
can only be the sum
of
the terms obtained
by
partial integration plus a constant:
p = C - P(bx2 +
gx
3
)·
Along the free surface exists the pressure P =
Po
and
thus the equation
of
the
plane in which
the
free surface lies reads:
h 1
x3
=
--x2
+
-(C
-
Po)
for-=
<
xI
<
+00.
g gp
The
integration constant C
is
determined by the condition
that
the fluid
volume before
and
after the onset
of
the
acceleration
is
the same. Therefore
the same relation holds
for
C as for the container with the fluid at rest:
C=gph
+
Po.
Thus
the
equation
for
the plane
of
the free surface reads:
b
x3 = h
--x
2
for-=
<
xI
<
+00.
g
As
the
solution
of
the
problem
has
to
be independent
of
the
chosen
coordinate system, a coordinate system
~i
can be introduced which
is
rotated
against
the
system
xi
in such a way
that
the
following equations for
the
coordinate transformations hold:
~I
=
xI
(axis
of
rotation),
1
~2
= I (gx2 +
bX3)
Vb2 + g2
and
~
- I (bx2 + gx3)
S3
-
Vb
2
+g
2
This
is
equivalent
to
the
introduction
of
a resulting acceleration
of
the
quantity
~b2
+ g2 in the direction
~3.
Thus
the basic hydrostatic equations
read:
Thus
P = F(x
3
)
holds
and
P =
p~b2
+
g2~3
The
integration
constant
C results
frt>m
the
boundary
condition:
P =
Po
+
gph
for
~3
= 0
160
Hydrostatics and Aerostatics
p =
PO+Pg(h-l+(!)\3)
All further statements concerning the problem
of
the
accelerated fluid
container can also be made in the coordinate system
~i"
Along the free surface
P =
Po
holds
and
h
S3=
FIT!
Thus
is
the equation
of
the plane in which the free surface lies.
By the above treatment it becomes clear
that
it
is
possible to employ the
hydrostatic basic laws also in accelerated reference systems, provided
the
inertia forces are taken into consideration that are attributable to the external
motions. The occurring accelerations (inertial and gravitational) are to be added
to a total acceleration in vectorial manner in order to
obtain the direction
and
the quantity
of
the total acceleration. The free surface appears vertical to the
vector
of
the total acceleration.
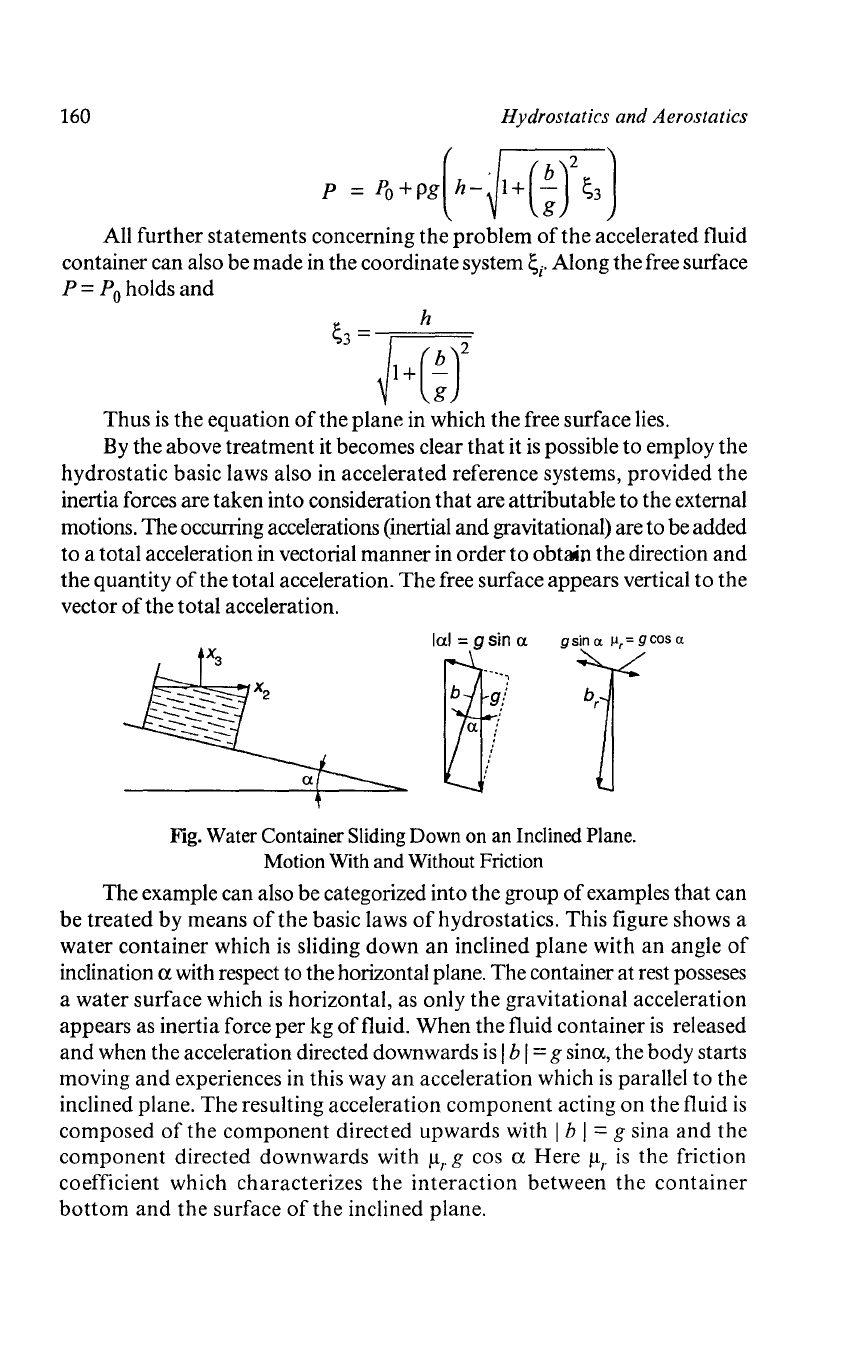
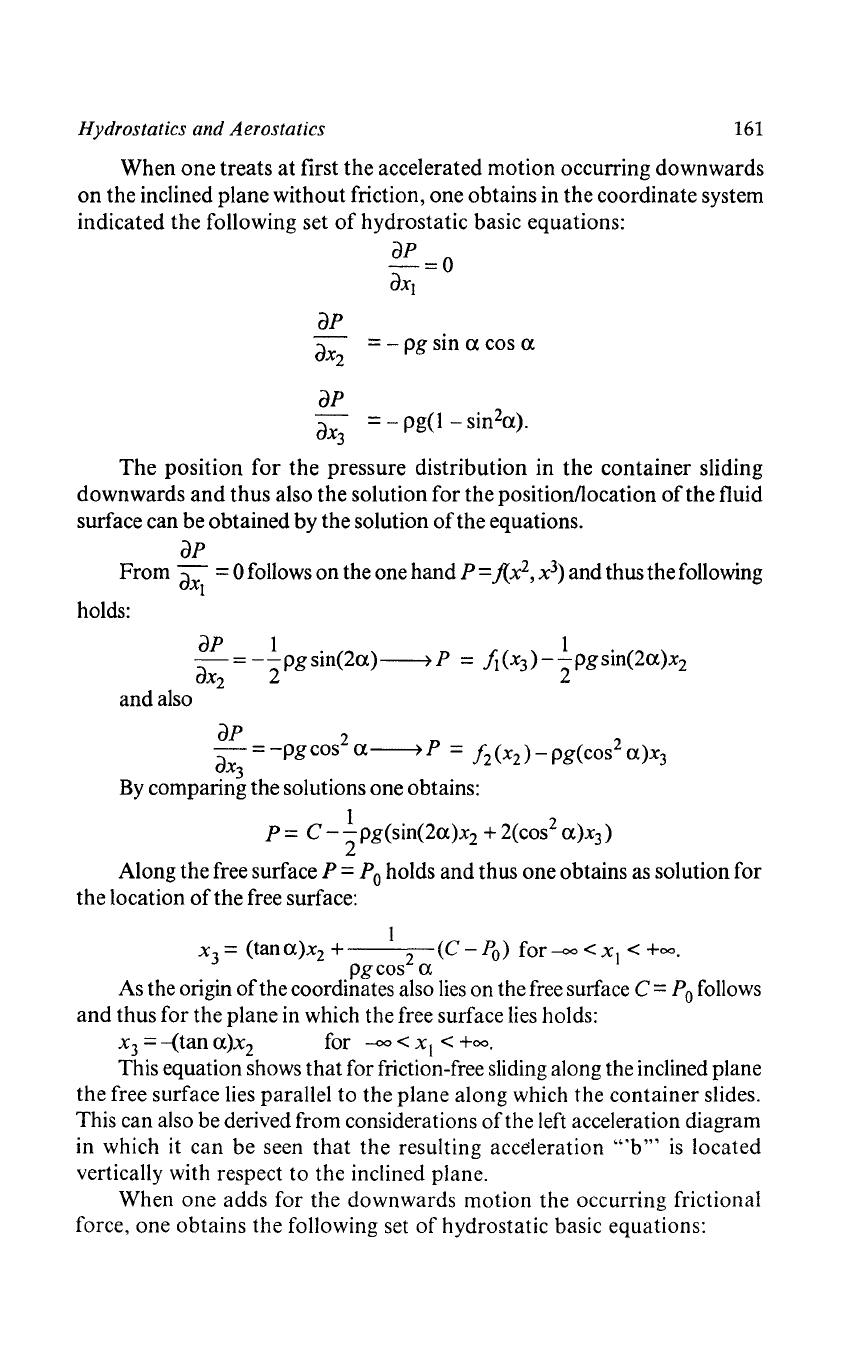
lal = g
sin
a
gsin
a
11,=
geas
a
Fig. Water Container Sliding
Down
on
an
Inclined Plane.
Motion With
and
Without Friction
The example can also be categorized into the group
of
examples
that
can
be
treated by means
of
the
basic laws
of
hydrostatics. This figure shows a
water container which
is
sliding
down
an
inclined
plane
with
an
angle
of
inclination
ex
with respect to the horizontal plane. The container at rest posseses
a water surface which
is
horizontal, as only
the
gravitational acceleration
appears as inertia force per kg
of
fluid. When the fluid container
is
released
and when the acceleration directed downwards
is
I b I = g
sinex,
the body starts
moving
and
experiences in this
wayan
acceleration which
is
parallel
to
the
inclined plane.
The
resulting acceleration component acting
on
the fluid
is
composed
of
the
component directed upwards with I b I = g sina
and
the
component directed downwards with
Ill'
g cos
ex
Here
Ill'
is
the friction
coefficient which characterizes
the
interaction
between
the
container
bottom
and
the
surface
of
the
inclined plane.
Hydrostatics and Aerostatics
161
When
one
treats
at
first
the
accelerated motion occurring downwards
on
the inclined plane without friction, one obtains in the coordinate system
indicated
the
following set
of
hydrostatic basic equations:
i)p
=0
i)xl
= - pg sin a cos a
i)p
:I
= - pg(l - sin
2
a).
oX3
The
position
for
the
pressure distribution in
the
container sliding
downwards
and
thus also
the
solution for the positionflocation
of
the fluid
surface can be obtained by the solution
of
the equations.
i)p
From
-;-
= 0 follows on the one hand P =
j{x
2
,
x3)
and thus the following
OXI
holds:
i)p
2
-=-pgcos
a----:;P
=
f2(X2)-pg(cos
2
a)X3
i)
x
3
By
comparing the solutions one obtains:
P=
c-.!..pg(sin(2a)x2
+ 2(cos
2
a)x3)
2
Along the free surface P =
Po
holds and thus one obtains as solution for
the
location
of
the free surface:
I
x3
= (tana)x2 + 2 (C -
Po)
for-oo <
Xl
<
+00.
pgcos a
As the origin
of
the coordinates also
lies
on the free surface C =
Po
follows
and
thus for the plane in which the free surface lies holds:
x3
= -{tan
a)x
2
for
-00
<
Xl
<
+00.
This equation shows that for friction-free sliding along the inclined plane
the free surface lies parallel
to
the plane along which the container slides.
This can also be derived from considerations
of
the left acceleration diagram
in which it can be seen
that
the
resulting acceleration
"'b'"
is
located
vertically with respect
to
the inclined plane.
When one adds for the downwards motion the occurring frictional
force, one obtains
the
following set
of
hydrostatic basic equations:
