Braibant S., Giacomelli G., Spurio M. Particles and Fundamental Interactions: An Introduction to Particle Physics
Подождите немного. Документ загружается.

10.3 Elastic Electron-Proton Scattering 269
10.3 Elastic Electron-Proton Scattering
10.3.1 Kinematic Variables
Figure 10.3 illustrates the kinematics of an ep elastic scattering in the laboratory
system using energy-momentum covariant four-vectors with „Dc D 1. The four-
momenta of the involved particles are
P D .E; p/I P
0
D .E
0
; p
0
/ for the incident and scattered electron (10.4)
P
0
D .M; 0/ I W D .E
0
0
; p
0
0
/ for the proton before and after impact: (10.5)
M is the proton mass. The square of the four-momentum of the incident electron
and of the proton at rest are
P
2
D .E
2
=c
2
p
2
/ D m
2
e
c
2
cD1
! m
2
e
I P
2
0
D M
2
: (10.6)
The four-momentum transferred by the e
in the scattering is
q D P P
0
D .E E
0
; p p
0
/ D .; q/ (10.7)
and its square t D q
2
is
t D q
2
D .P
0
P/
2
D .E
0
=c E=c/
2
.p
0
p/
2
cD1
! 2m
2
e
2E
0
E C 2p
0
p cos : (10.8)
At high energy, the electron mass can be neglected .m
e
D 0; p ' E/,thatis,
q
2
DQ
2
'2EE
0
.1 cos / D4EE
0
sin
2
.=2/: (10.9)
P
P'
P
0
W
q
θ
p
ab
p'
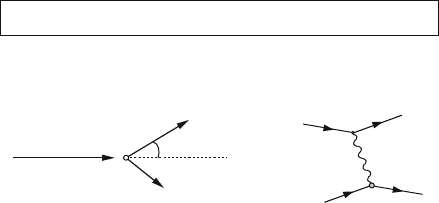
Fig. 10.3 (a) The kinematics of elastic e
p ! e
p scattering in the laboratory system. p e p
0
are
the electron momenta before and after collision; is the scattering angle. In (b), the corresponding
Feynman diagram is shown: P; P
0
and P
0
;W are, respectively, the energy-momentum four-vectors
of the electron and of the hadronic system before and after collision. q is the transferred four-
momentum
270 10 High Energy Interactions and the Dynamic Quark Model
Since q
2
is negative, the quantity Q
2
Dq
2
is often used. For scattering at high
energy and small angles, one has p
0
' p; sin ' and t D q
2
'p
2
2
.Interms
of the four-momentum transferred to the proton, one has
t D q
2
D .M E
0
0
/
2
.0
!
p
0
/
2
D 2M
2
2M W D2M T
p
(10.10)
where T
p
D W M is the kinetic energy of the recoiling proton.
The total energy in the center of mass is
s D .P CP
0
/
2
D p
2
CP
2
0
C2PP
0
D m
2
e
CM
2
C2EM ' M
2
C2EM: (10.11)
Finally, one has
P
0
q D M with D E E
0
; (10.12)
representing the condition for elastic scattering.
The numerical values of the squared four-vector, for example, q
2
and s,arethe
same in any reference system; they can be calculated in the laboratory system as
shown here. For an elastic scattering, one has q
2
<0; this condition is called space-
like. In annihilation processes, one has q
2
>0; this situation is called time-like.
As shown below, the cross-section for elastic ep scattering can be calculated using
successive approximations.
10.3.2 Proton Form Factors
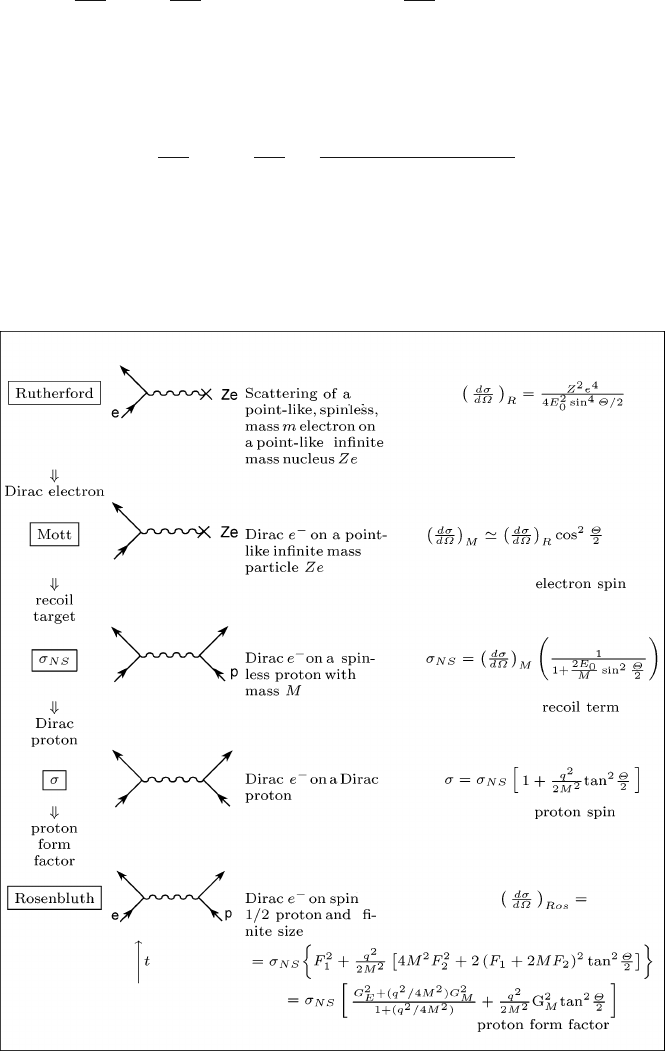
Rutherford scattering. The simplest calculation concerns the elastic scattering of
a spinless, point-like electron with mass m
e
and charge e by a point-like infinitely
massive nucleus of charge Ze (for the proton Z D1). The elastic cross-section is
described by the Rutherford formula seen in Sect. 4.7.1 (for z D 1), that is,
d
d˝
R
D
Z
2
e
4
q
4
D
Z
2
e
4
t
2
D
Z
2
e
4
4E
2
0
sin
4
2
: (10.13)
The formula and the corresponding Feynman diagram are shown in the Box here
below. Note that the wave line representing the exchanged photon ends (on the right)
in the massive charge Ze.
Mott formula. The next approximation introduces the electron spin through the
relativistic Dirac equation. The proton spin is still ignored. For relativistic electrons,
the spin vector is aligned with the electron momentum p.Thehelicity is the
projection of the spin along the direction of the momentum (Appendix A.4). The
electron helicity can be D˙1. The electron is right-handed or left-handed if
the helicity is DC1 or D1, respectively. The electromagnetic interaction
conserves the helicity. This implies constraints on the shape of the final state wave
function [P87], which introduces a factor cos
2
.
2
/ in the cross-section:
10.3 Elastic Electron-Proton Scattering 271
d
d˝
M
D
d
d˝
R
.1 ˇ
2
sin
2
=2/ '
d
d˝
R
cos
2
.=2/: (10.14)
Proton with mass M. In the previous formulas, the proton was considered as
infinitely massive. The Feynman diagram must be modified (see Box) to include
its finite mass M , leading to the following formula, that is,
d
d˝
NS
D
d
d˝
M
1
1 C .2E
0
=M / sin
2
.=2/
; (10.15)
reducing to (10.14)forM !1.
Box. Differential e
p cross-section with increasing accuracy in the description of
the proton and electron structures. Note that in these Feynman diagrams, the time
axis goes from bottom to top.
272 10 High Energy Interactions and the Dynamic Quark Model
Proton with spin. The next approximation includes the spin of the proton, which
is assumed to be point-like (Dirac proton). The introduction of the spin induces an
additional term due to the magnetic dipole interaction between an electron and a
proton at a distance r:
0
4
r
r
3
. The cross-section becomes
d
d˝
D
d
d˝
NS
1 C
q
2
2M
2
tan
2
.=2/
: (10.16a)
However, the magnetic moment of the proton (or neutron) is different from
that predicted by the Dirac theory for spin 1=2 particles, i.e.,
0
D e„=2Mc
(Sect. 7.14.4). In 1950, Rosenbluth obtained
d
d˝
D
d
d˝
NS
1 C
q
2
4M
2
2.1 C /
2
tan
2
.=2/ C
2
(10.16b)
for a “point-like” nucleus, where is the anomalous part of the magnetic moment,
and equal to 1.79 for the proton and 2.91 for the neutron,
p;n
D .1 C /
0
: (10.17)
Formula (10.16b) can be extended to the case of a nucleon with an internal structure
by introducing the so-called form factors F
1
.q
2
/ and F
2
.q
2
/.
Proton with finite size. Finally, the finite size of the proton (10
15
m) must be
taken into account. A spatial form factor f.r/is introduced to remove the point-like
approximation and to describe the electric charge spatial distribution, that is,
%.r/ D ef .r / (10.18)
where % D dq=dv, with the condition that
R
f.r/dv D 1,thatis,
R
%.r/d v D e.
In the simplest case, the distribution function f.r/ can be interpreted as the
classical spatial distribution of electric charge, or the probability distribution of
finding the charged point-like constituents in the proton volume. The form factor
F.q/ is defined as the Fourier transform of the spatial distribution f.r/ and
correspond to f.r/in the momentum space
F.q/ D
Z
e
iqx
f.r/d
3
x: (10.19)
Examples of some form factors are listed in Table 10.1 and shown in Fig. 10.4,
assuming in all cases a spherically symmetric f.r/.
The Fourier transform (10.19) of the Yukawa potential has been explicitly derived
in Sect. 4.4. This potential, except for numerical constants, has a similar dependence
in r than that of the Yukawa charge spatial distribution given in Table 10.1.
We may notice that F.q/ depends in this case only on the scalar quantity q
2
.

10.3 Elastic Electron-Proton Scattering 273
Table 10.1 Charge spatial distributions and corresponding form factors
expressed as a function of the transferred four-momentum q D
p
jt j
Charge spatial distribution Form factor
Point-like f.r/ D ı.r r
0
/ F.q
2
/ D 1 constant
Exponential f.r/ D
a
3
8
e
ar
F.q
2
/ D
h
1
1Cq
2
=a
2
i
2
dipole
Yukawa f.r/ D
a
2
4r
e
ar
F.q
2
/ D
1
1Cq
2
=a
2
pole
Gaussian f.r/ D
a
2
2
1
2
e
.a
2
r
2
=2/
F.q
2
/ D e
.q
2
=2a
2
/
Gaussian
The fact that F.q/ D F.q
2
/ is true even if the charge distributions are exponential
or Gaussian, as shown in the table. Hereafter, the form factor will always be referred
to as F.q
2
/.
Equation 10.16b can be generalized to take into account the proton (or neutron
structure) due to charge distribution and magnetization, that is,
d
d˝
D
d
d˝
NS
(
F
1
.q
2
/ C
q
2
4M
2
"
2.F
1
.q
2
/ CF
2
.q
2
//
2
tan
2
2
C
2
F
2
2
.q
2
/
#)
: (10.20)
The form factors are different for electron collisions on protons or neutrons: they
will be listed in the following with a p or n superscript. The q
2
dependence indicates
that the form factors are expected to vary with the square of the transferred four-
momentum. For low q
2
, the form factors are normalized to
F
p
1
.0/ D F
p
2
.0/ D F
n
2
.0/ D 1I F
n
1
.0/ D 0:
F
p
1
;F
p
2
and F
n
1
;F
n
2
(sometimes called Dirac and Pauli form factors) can be more
conveniently expressed as a linear combination, producing the so-called electric and
magnetic form factors. For the proton and the neutron, they are defined as
G
p;n
E
.q
2
/ D F
p;n
1
.q
2
/
q
2
4M
2
F
p;n
2
.q
2
/ (10.21a)
G
p;n
M
.q
2
/ D F
p;n
1
.q
2
/ C F
p;n
2
.q
2
/: (10.21b)
The electric form factor G
E
.q
2
/ describes the electric charge distribution inside the
proton or the neutron. The magnetic form factor G
M
.q
2
/ describes the distribution
of the magnetic dipole moment. It should be noted that the interpretation of form
factors in terms of charge spatial distribution has no meaning in the high energy
limit because the incident electron does not see a static charge distribution, but a
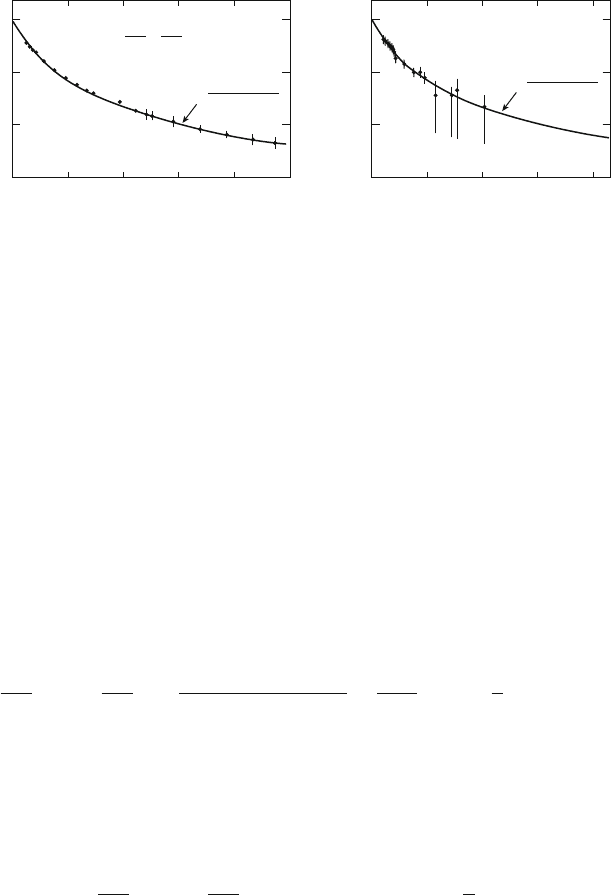
274 10 High Energy Interactions and the Dynamic Quark Model
1
(1 + q
2
/ 0.71)
2
1
(1 + q
2
/ 0.71)
2
G
p
M
μ
p
G
n
M
μ
n
G
p
E
G
p
E
=
0.001
0.01
0.1
ab
1
0.001
0.01
0.1
1
0246810
q
2
,GeV
2
02468
q
2
,GeV
2
G
p
M
/ μ
p
Fig. 10.4 Electric and magnetic form factors of the proton and magnetic form factor of the
neutron. The solid line represents a fit with a dipole form factor (see Table 10.1), corresponding to
a Yukawa-like charge spatial distribution
moving one. The electric and magnetic form factors are normalized to the electric
charge and magnetic moment of each particle, for example,
G
p
E
.q
2
/ is normalized to: G
p
E
.0/ D 1
G
p
M
.q
2
/
00
G
p
M
.0/ D 2:79
G
n
E
.q
2
/
00
G
n
E
.0/ D 0
G
n
M
.q
2
/
00
G
n
M
.0/ D1:91:
(10.22)
With the introduction of form factors in (10.20), the Rosenbluth formula for the ep
elastic scattering is obtained, namely,
d
d˝
Ros
D
d
d˝
NS
G
2
E
C .q
2
=4M
2
/G
2
M
1 C.q
2
=4M
2
/
C
q
2
2M
2
G
2
M
tan
2
2
: (10.23)
Note that there is no interference between the electric and magnetic form factors.
If the Rosenbluth cross-section is plotted at q
2
D constant and for different
energies and scattering angles, a linear dependence on tan
2
.=2/ is obtained,
namely,
d
d˝
Ros
d
d˝
NS
D A.q
2
/ C B.q
2
/ tan
2
2
: (10.24)
The experimental proof of such a linear dependence on tan
2
.=2/ is evidence that
the scattering is mediated by the exchange of a single photon.
Measurement of proton and neutron form factors are shown in Fig. 10.4.They
were first obtained in the 1960s by R. Hofstadter for the proton. Because free
neutrons are not available in nature, the neutron form factors were derived from
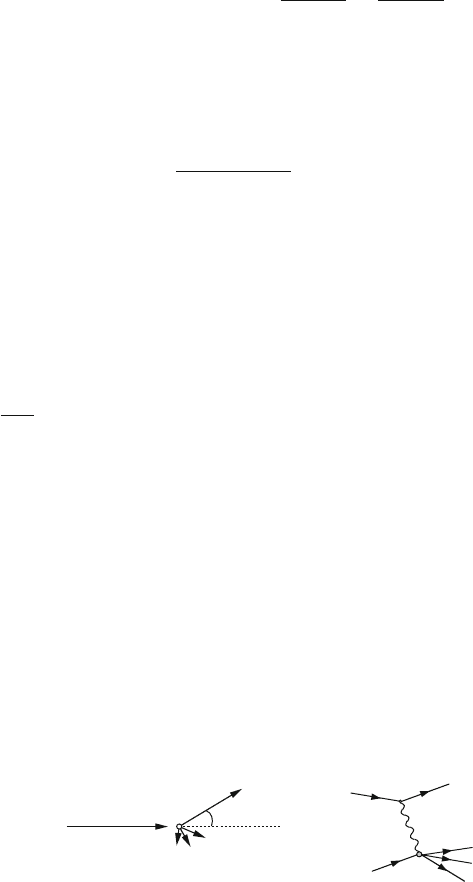
10.4 Inelastic ep Cross-Section 275
measurements on deuterium (the pn bound state) after subtracting the contribution
of the proton. The results can be parameterized by the following empirical expres-
sion, the scaling law and the dipole formula. Scaling law:
G.q
2
/ D G
p
E
.q
2
/ D
G
p
M
.q
2
/
p
D
G
n
M
.q
2
/
j
n
j
(10.25a)
G
n
E
.q
2
/ D 0: (10.25b)
Dipole formula:
G.q
2
/ D
1
1 C .q
2
=0:71/
2
Œq
2
in .GeV=c/
2
: (10.26)
The Fourier transform of the dipole form factor in the coordinate space gives (see
Table 10.1,rinfm)
f.r/
dipole
D 3:06 e
4:25r
: (10.27)
For small transferred momentum, one has
G
p
E
.q
2
/ ' f.0/Œ1 .1=6/q
2
hr
2
i; (10.28)
with
p
hr
2
iD0:81 fm. For high transferred momenta, the elastic form factors are
very small and inelastic scattering of the incident electron becomes much more
likely than elastic scattering.
10.4 Inelastic ep Cross-Section
If the reaction (10.1) is inelastic, the target fragments in a final state of mass
W>M. The lepton energy and scattering angle in the final state are independent
variables (Fig. 10.5). The mass of the system X is
W
2
D .P
0
C q/
2
D M
2
C q
2
C 2M D M
2
Q
2
C 2M > M
2
: (10.29)
P
P'
P
0
W
q
p
θ
p'
Fig. 10.5 Kinematics of inelastic scattering e
p ! e
p in the laboratory system and as a
Feynman diagram. See Fig. 10.3 for a description of the variables

276 10 High Energy Interactions and the Dynamic Quark Model
In this case,
2M > Q
2
: (10.30)
For inelastic scattering, the squared transferred four-momentum q
2
and the trans-
ferred energy are independent variables. The elastic limit is characterized by the
condition W
2
D M
2
, i.e., 2M D Q
2
. The inelastic cross-section can be expressed
in terms of these two variables as
d
2
dQ
2
d
D
4˛
2
Q
4
E
0
E
cos
2
2
W
2
.Q
2
;/C W
1
.Q
2
;/2tan
2
2
: (10.31)
Equation 10.31 is similar to Eq. 10.16a for elastic scattering. The difference is that
now W
1
;W
2
are arbitrary structure functions, which generally depends on the two
kinematic variables Q
2
;.
Deep inelastic scattering (DIS) experiments were used to investigate the inner
structure of protons and neutrons, and to test the quark hypothesis. The deep
inelastic scattering conditions are defined by
Q
2
M
2
I D E E
0
M: (10.32)
Deep inelastic interactions of elementary particles like electrons, muons or neutrinos
with a nucleon will be the result of a superposition of elastic scattering with the
constituents if the nucleon is composed of point-like particles. If these partons
have mass m and if the transferred energy is much larger compared to their binding
energy, the cross-section (10.31) will be the sum of the contributions of the lepton
elastic scattering on the different partons,thatis,
d
2
dQ
2
d
ela
D
4˛
2
Q
4
E
0
E
cos
2
2
1 C
Q
2
4m
2
2 tan
2
2
ı
Q
2
2m
: (10.33)
Note that (1) ı. Q
2
=2m/ expresses the condition that the impact is elastic
(W D m) from (10.29); (2) since the collision with the parton is elastic, Eq. 10.33
has the same structure as the elastic ep cross-section (10.16a) after replacing M with
the parton mass m; (3) comparing Eq. 10.33 with Eq. 10.31, the following conditions
on the structure functions can be written as
W
2
.Q
2
;/!
1
ı
Q
2
2m
I W
1
.Q
2
;/!
Q
2
4m
2
ı
Q
2
2m
: (10.34)
In 1967, Bjorken showed that in deep inelastic scattering, the structure functions
describing the nucleon depend on dimensionless variables. In particular, they do
not depend on the transferred four-momentum Q
2
, on the transferred energy and
on the nucleon size, as in the case of elastic scattering. This property was derived
assuming that the collisions of electrons, muons and neutrinos on p and n occur with
10.4 Inelastic ep Cross-Section 277
Fig. 10.6 Differential
cross-section for ep
interactions as a function of
the hadronic energy W
Elastic peak
d
2
σ/dΩdE’ (μb / GeV)
their point-like fermionic constituents; it is called the Bjorken scaling law.Bjorken
scaling assumes that for Q
2
!1;!1, the quantity
x D
Q
2
2M
remains finite: (10.35)
Let us assume that the elastic scattering condition Q
2
D 2m,foramassm
parton at rest in the laboratory system, is valid. In this case, Eq. 10.35 becomes
x D m=M .Inthisway,thevariablex can be interpreted as the fraction of the
nucleon mass carried by the parton interacting with the lepton. Consequently, the
structure functions W
1
;W
2
, in the high energy limit, do not separately depend on
Q
2
and , but only by the dimensionless ratio x,thatis,
W
2
.Q
2
;/
Q
2
!1;!1
! F
2
.x/I MW
1
.Q
2
;/
Q
2
!1;!1
! F
1
.x/: (10.36)
The proof of this hypothesis was obtained starting in 1968 with a series of
experiments lead by Friedman, Kendall and Taylor (Nobel laureate in 1990) and
collaborators at the Stanford University Linear Accelerator (SLAC) in California.
In these experiments, a linear electron accelerator of approximately 3 km long
produced electron beams up to 20 GeV that were directed on hydrogen and
deuterium targets. The energy E
0
and the scattering angle of the electron in the
final state were measured; from these measurements, Q
2
; and W were obtained.
Figure 10.6 shows the differential cross-section d
2
=d˝dE
0
as a function of the
hadronic energy W . The peak of the elastic scattering on the proton, at W D M ,
has been removed for clarity. At W 1:2 1:8 GeV, the excitation of baryonic
resonances (the first is the usual with a mass of 1230MeV) are visible; no exited
states are present in the continuous distribution for W>1:8GeV. At a fixed W ,the
cross-section decreases rapidly with increasing Q
2
due to the form factor F.Q
2
/:
assuming that the scattering is elastic on the parton constituents, it is expected that
the cross-section decreases with increasing Q
2
, similar to the elastic form factors
(Fig. 10.4).
278 10 High Energy Interactions and the Dynamic Quark Model
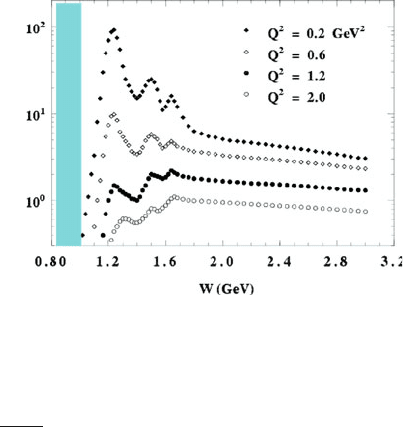
Fig. 10.7 ep interaction:
ratio between the elastic
(solid curve) and inelastic
cross-sections (points)and
the Mott cross-section (on a
point-like and spinless target)
as a function of the
transferred four-momentum
(d
2
σ/dΩdE’) / (d
2
σ/dΩdE’)
Mott
Elastic scattering
With increasing transferred four-momentum Q
2
, the total electron-proton cross-
section decreases: inelastic scattering becomes increasingly important compared to
the elastic one. This is evident in Fig. 10.7, where the inelastic and elastic cross-
sections are compared to a function of Q
2
. The inelastic cross-section becomes
larger than the elastic one when Q
2
is larger than the values corresponding to
the resonance formation (Q
2
O.1GeV
2
/). In addition, at fixed W
2
D M
2
C
2M Q
2
, the inelastic cross-section remains approximately constant and does not
depend on Q
2
, as can be seen in Fig.10.7 for W D 3 GeV. The Bjorken scaling
law is verified in the Q
2
region where the production of baryonic resonances is no
longer important.
10.4.1 Partons in the Nucleons: Their Nature and Spin
The meaning of the Bjorken x variable (10.35) and of the functions F
1
.x/ and F
2
.x/
becomes more clear if the cross-section (10.31) is expressed as a function of x (the
variable x D Q
2
=2M implies that
dx
d
D
x
and thus
d
dx
D
x
d
d
), that is,
d
2
dQ
2
dx
D
x
d
2
dQ
2
d
D
4˛
2
Q
4
E
0
E
1
x
cos
2
2
W
2
.Q
2
;/C W
1
.Q
2
;/2tan
2
2
D
4˛
2
Q
4
E
0
E
1
x
cos
2
2
F
2
.x/ C
F
1
.x/
M
2 tan
2
2
D
4˛
2
Q
4
E
0
E
1
x
cos
2
2
F
2
.x/ C 2xF
1
.x/
Q
2
4M
2
x
2
2 tan
2
2
:
(10.37)
