Boyd T.J.M., Sanderson J.J. The Physics of Plasmas

Подождите немного. Документ загружается.

7.8 Test particle in a Vlasov plasma 287

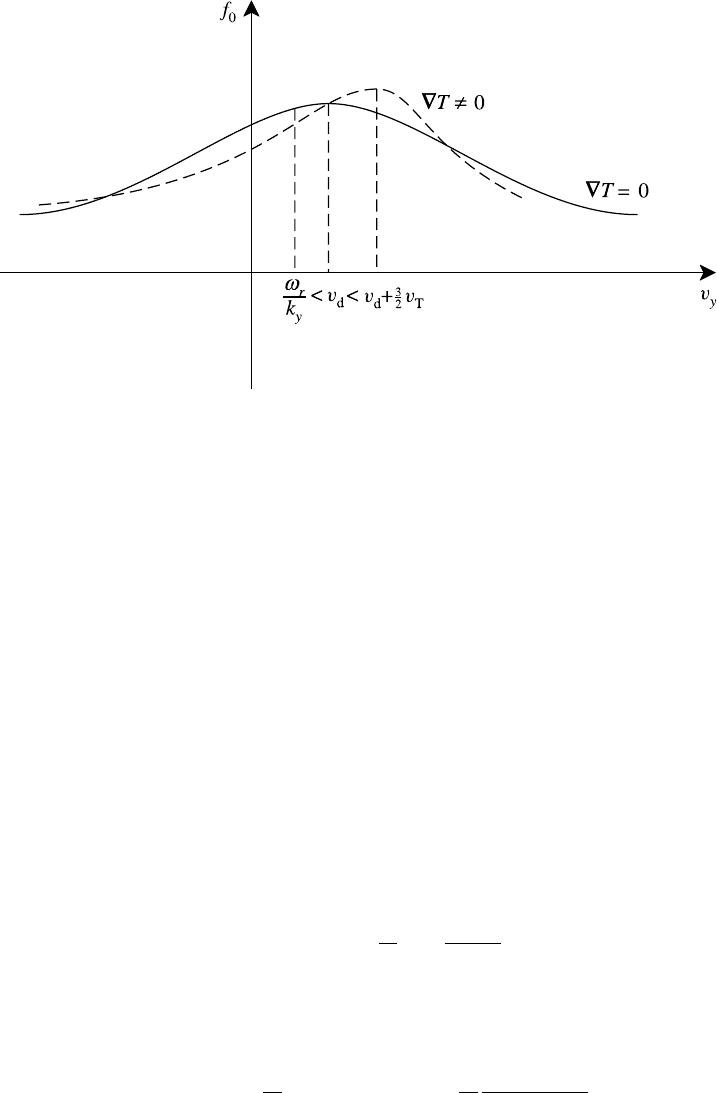
Fig. 7.17. Temperature gradient distortion of the electron distribution function. The slope

of f

at v

= ω

is proportional to (k

−ω

) for v

= 0 and to (k

+3v

/2)−ω

)

for v

= 0 (after Priest and Sanderson (1972)).

7.8 Test particle in a Vlasov plasma

In the next chapter we deal with the kinetic theory of plasmas allowing for colli-

sional effects. We shall see there that the important collisional effects in plasmas

are long range many-body interactions rather than short range binary collisions. In

this section we outline an approach to particle–plasma interactions that focuses on

the interaction between a discrete charged particle, or test particle and the other

charges in the plasma using the Vlasov–Poisson equations. We begin by isolating

a single particle of charge q

which is injected at t = 0 (at r

= 0) and moves

through the plasma with velocity u

, assumed constant. We suppose that our test

charge causes only a small perturbation in the plasma electron density so that we

may reasonably describe the effect of the test charge on the rest of the plasma

electrons using the linearized Vlasov equation (7.22)

( p + ik · v) f

(k, v, p) =−



k ·

∂ f

(v)

∂v



φ(k, p) (7.71)

where we now take f

(k, v, t = 0) = 0. From Poisson’s equation

φ(k, p) =−



(k, v, p)dv +

( p + k · u

)

(7.72)

288 Collisionless kinetic theory

From (7.71) and (7.72) proceeding in parallel with Section 7.3 we ﬁnd

φ(k, p) =

D(k, p)( p + ik · u

)

(7.73)

When it comes to inverting the Laplace time transform we have now an additional

pole at p =−ik · u

. As before we consider the long time behaviour of φ(k, t )

and, since we are principally concerned with the effect of the test electron on the

plasma, only the contribution from the pole at p =−ik · u

is taken into account

so that

φ(k, t) =

−ik·u

D(k,ω = k ·u

)

(7.74)

In the special case u

 V

we ﬁnd φ(k, t) = (q

/ε

)(k

+ k

)

−1

where k

is the

reciprocal of the Debye length. From this it is straightforward to retrieve the Debye

shielding potential (see Exercise 7.14)

φ(r, t →∞) =

4πε

exp(−r/λ

)

(7.75)

7.8.1 Fluctuations in thermal equilibrium

When we discuss collective effects in radiation from plasmas in Chapter 9 we

shall need a representation for electric ﬁeld ﬂuctuations in a plasma. Since the

electric ﬁeld itself is a random variable in both space and time what we want is the

ensemble average of the energy density of the electric ﬁeld. Using the concept of a

test charge we allow each plasma particle in turn to take the role of the test particle

and sum the contributions of each. From (7.73),

E(r, t) =−



ik·[r−r

(t)]

D(k,ω = k ·u

)

dk (7.76)

where r

(t) = u

t. Allowing each particle in turn to be the test particle we then

ﬁnd the ensemble average of the electric ﬁeld by introducing the distribution func-

tion f

, v

) which is the probability density for particles to have velocity v

position r

, i.e.

E(r, t)=



E(r, t) f

, v

) dr

For a uniform isotropic plasma clearly E(r, t)=0.

On the other hand for the ensemble average of the energy density of the electric

ﬁeld we have

W =



[E(r, t) · E

∗

(r, t)] f

, v

) dr

Exercises 289

Introducing the Fourier–Laplace transform of the ensemble average energy density

W (k,ω)it is straightforward to show (see Exercise 7.14)

W (k,ω)=

(ω/k)

|D(k,ω)|

(7.77)

Exercises

7.1 Show that ∇

· a = 0 when the acceleration a is due to the self-consistent

electromagnetic ﬁeld, E +v ×B. Why can we not assume that ∇

·a = 0

when the acceleration is caused by collisional interactions with neighbour-

ing particles?

7.2 Show that the Maxwell distribution function satisﬁes the Vlasov equation

identically. Explain this in terms of (i) constants of the motion and (ii)

the Maxwell distribution being the asymptotic solution of the collisional

kinetic equation.

7.3 In the weak coupling approximation the potential energy of particle inter-

actions is very much smaller than particle kinetic energy. Show that this

approximation is equivalent to the condition for the number of particles in

the Debye sphere being very large.

7.4 Show that the dispersion relation (6.97) for electron plasma waves, derived

from the warm plasma wave equations, is equivalent to the result (7.34)

obtained from kinetic theory. What assumptions and approximations have

to be made to obtain this equivalence?

Obtain (7.37) from (7.36). Explain mathematically and physically why

this result cannot be obtained from the warm plasma wave equations.

7.5 The plasma used in the measurements of the dispersion characteristics

and Landau damping of Langmuir waves in Figs. 7.4 and 7.5 formed a

column 2.3 m long with an axial electron density typically 10

–10

−3

Electron temperature ranged between 5 and 20 eV and the pressure of the

background gas (mostly hydrogen) was ∼ 10

−3

pascals.

Estimate λ

and nλ

. Determine the mean free path for both electron–

ion and electron–neutral collisions.

Note that in this experiment Malmberg and Wharton did not measure

electron density directly but chose a value which normalized the theoretical

dispersion curve to the data points at low frequencies. Why is this justiﬁed?

Why does it appear that in the limit of small k, ω → 0 rather than ω

Plot ω versus k using (7.36) with T

= 9.6 eV and compare your re-

sults with Fig. 7.4. Interpret the discrepancy between this result and the

corresponding line in Fig. 7.4.

290 Collisionless kinetic theory

In the experiment two probes were used, one serving as transmitter

while the detector was moved along the axis. The data recorded consisted

of the real and imaginary parts of k. Solve the dispersion relation in the

form k = k(ω) for real ω and obtain an expression for the attenuation of

Langmuir waves as a result of Landau damping. Plot k/k as a function

of (v

)

, where v

denotes the phase velocity, and compare your results

with Fig. 7.5.

7.6 Dawson (1962) devised a physical model of Landau damping based on

considerations of wave–particle interactions. The rate of change of the

kinetic energy T

of particles resonant with the wave, computed in the wave

frame, in which the wave electric ﬁeld is represented as E = E

sin kx,is



∞

−∞

∂ f 

∂t



v +



dv (E7.1)

where  f  denotes the spatially averaged distribution function. It follows

from the Vlasov equation that

∂ f 

∂t



∂ f

∂v

sin kx



(E7.2)

The solution to the linearized Vlasov equation with the initial condition

(x,v,0) = f

(v, 0) cos kx is

(x,v,t) = f

(v, 0) cos(kx − vt) +

mkv

∂

∂v

[

cos k(x − vt) − cos kx

]

(E7.3)

Use (E7.3) in (E7.2) to determine ∂ f /∂t and substitute this in (E7.1)

to show that

=−

neE



∞

−∞

(v, 0)



v +



sin kvt dv

−



∞

−∞

∂ f

∂v



v +



sin kvt

dv (E7.4)

The ﬁrst term in (E7.4) decays through phase mixing. In the second term

the only particles that make a contribution to dT

/dt are those moving

slowly in the wave frame and lim

v→0

sin kvt/kv → πδ(kv). Show that

=−πω

∂ f

∂v



v=0

W (E7.5)

where W =

is the wave energy density. Now

=−

=−2γ

W (E7.6)

Exercises 291

so that the linear damping rate for the electrostatic ﬁeld set up by the initial

perturbation is, after transforming back to the laboratory frame,

∂ f

∂v



v=ω/k

(E7.7)

This is the Landau damping rate (7.37).

7.7 Obtain the dispersion relation (7.45) for ion acoustic waves and the ap-

proximation to the Landau damping decrement (7.46). Compare results

from (7.45), (7.46) with the numerical solution to the dispersion relation

in Fig. 7.7.

Consider a plasma consisting of two ion species and electrons. Suppose

that the temperature of the two ion populations is the same and that one of

the ions is much heavier than the other. Obtain a dispersion relation for ion

acoustic waves in this plasma.

7.8 Deﬁnitive measurements of the dispersion characteristics of ion acoustic

waves were made in stable plasmas in Q machines (Wong, Motley and

D’Angelo (1964)). In this work an alkali metal plasma was created by

contact ionization. The plasma was almost completely ionized and close to

thermal equilibrium. Magnetic ﬁelds of the order of 1 T meant that 

∼

100 MHz which is very much higher than the mode frequencies studied.

Measurements were made using potassium and caesium plasmas with T

 2300 K.

Conﬁrm that to a good approximation the ions are collisionless. Since

the plasma is produced at one end of the column, recombination losses

induce a drift of plasma away from the producing plate. Accordingly,

phase velocities of waves moving both upstream and downstream were

measured:





= 1.3 × 10

−1





= 0.9 × 10

−1





down

= 2.5 × 10

−1





down

= 1.3 × 10

−1

Allowing for a drift velocity V

(negligible compared with electron

thermal velocity), show that the upstream (−) and downstream (+) phase

velocities

= 2.05





1/2

± V

0.72(k

)

1/2

2.05 ± V

/(k

)

1/2

(k = k

+ ik

). Use this expression to compare predicted and measured

phase velocities.

292 Collisionless kinetic theory

Table 7.1. Ion wave damping





theory





expt





theory





expt

Downstream wave 0.65 0.55

Upstream wave 0.14 0.25

Damping of the ion wave was also measured. The damping distance δ

is that distance over which the wave amplitude is attenuated by a factor

e = exp(1). The damping constant was calculated and found to be

2π

= 0.39 ± 0.19

)

1/2

Verify this result and use it to complete Table 7.1.

7.9 Many plasmas both in the laboratory and in space are characterized by

non-Maxwellian distributions. As an example consider a two-component

electron distribution function, f = f

+ f

where both hot (h) and cold (c)

components are Maxwellian.

Show that for |ζ

|1, |ζ

|1 (7.45) remains valid provided T

replaced by an effective temperature deﬁned by

eff

+ n

If n

 n

and T

 1 another acoustic-like mode, the electron

acoustic mode, appears. Refer to Gary (1993) (Section 2.2.3) for a sum-

mary of the characteristics of this mode.

7.10 By taking zero-, ﬁrst-, and second-order moments of (7.69), obtain (7.66),

(7.68) and (7.70), respectively. [Hint: Keep only linear terms in small

quantities.]

7.11 How is the growth rate for the BTI instability changed when k and v

are

not parallel?

We shall ﬁnd in Chapter 10 that the one-dimensional BTI evolves to

produce a plateau distribution across a range of velocities

f (v

<v<v

) = const.

f (v > v

) = 0

Show that this distribution is unstable in the case of Langmuir waves

propagating at an angle θ(= 0) to the direction of the beam.

Exercises 293

7.12 In a plasma in which electrons drift relative to ions with a drift velocity

 V

, the electron thermal velocity, show that the growth rate of

the ion acoustic instability is given by (7.54). Determine the instability

threshold.

7.13 In magnetized plasmas there is an ion counterpart to electron Bernstein

modes, the ion Bernstein modes. In this case we have to distinguish be-

tween two possible outcomes. One corresponds to the electron mode in that

 0 and these so-called pure ion Bernstein modes mirror the electron

modes having no damping for exact orthogonal propagation. Moreover, in

the ﬂuid limit they collapse to the lower hybrid mode. However, unlike

the electron case with ﬁnite k

such that ω/k

 1, electrons can now

maintain a Boltzmann distribution by ﬂowing along the magnetic ﬁeld

lines to cancel charge separation.

Show that the dispersion relation for these neutralized ion Bernstein

waves may be written

1 + k

∞



n=1



(ω

− n



)

−λ

(λ

) (E7.8)

Take the ﬂuid limit of (E7.8), i.e. λ

→ 0, and assuming quasi- neutral-

ity, kλ

 1, retrieve the dispersion relation for electrostatic ion cyclotron

waves

= 

+ 2k





(E7.9)

7.14 Obtain (7.75).

In Chapter 9 it will prove helpful to decompose φ(k, p) in (7.73) into

two parts, the ‘self-ﬁeld’ of the test charge and the ﬁeld due to polarization

induced in the plasma by the test charge. Show that the induced ﬁeld is

determined by

ind

( p + ik · u

)



D(k, p)

− 1



Establish the expression for the ensemble-averaged energy density

W (k,ω)in (7.77).

7.15 Plasma kinetic theory developed in this chapter on the basis of the Vlasov–

Maxwell equations relies on linearization, and even then one generally

has to solve dispersion relations numerically. By way of illustration we

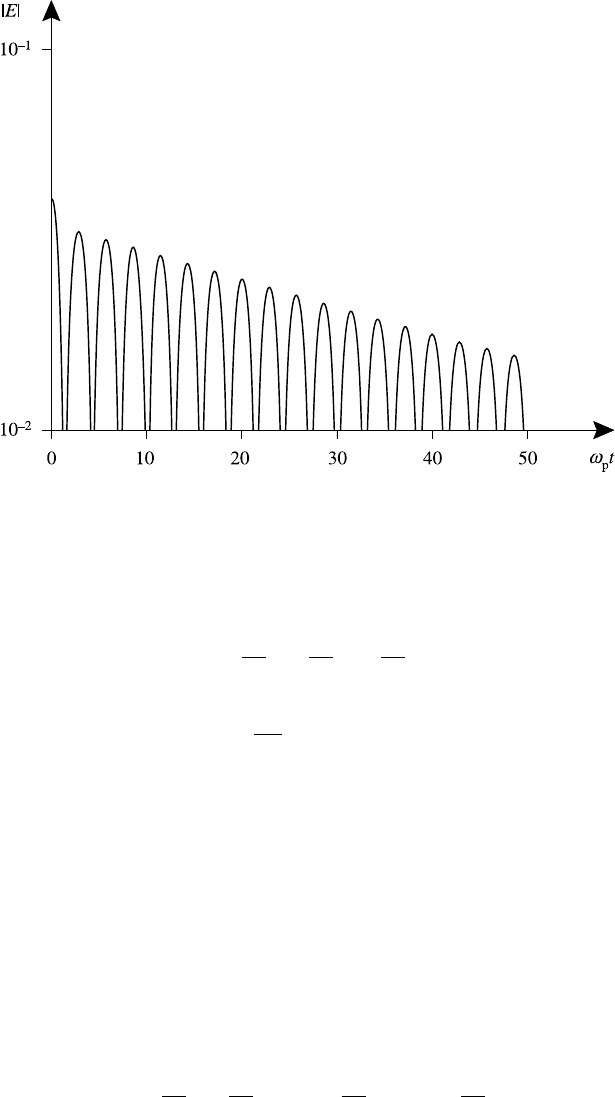
294 Collisionless kinetic theory

Fig. 7.18. Time evolution of a Langmuir wave from a 1D Vlasov code.

consider a direct numerical integration of the 1D normalized Vlasov–

Poisson equations

∂ f

∂t

+ v

∂ f

∂x

− E

∂ f

∂v

= 0 (E7.10)

∂ E

∂x

= 1 −



∞

−∞

f dv (E7.11)

The Vlasov equation (E7.10) is an advective equation in the 2D phase

space (x,v). If we set about differencing (E7.10) directly we ﬁnd that the

numerical diffusion that is characteristic of difference schemes in conﬁgu-

ration space now gives rise to diffusion in velocity space as well. We shall

see in the following chapter that velocity space diffusion is a property of

the Fokker–Planck collision operator. Clearly a numerical solution to the

Vlasov equation that mimics collisional effects is to be avoided.

An alternative approximation ﬁrst used by Cheng and Knorr (1976)

introduces a splitting technique in which the Vlasov equation is replaced

by the pair of equations

∂ f

∂t

+ v

∂ f

∂x

= 0

∂ f

∂t

+ E(x, t)

∂ f

∂v

= 0 (E7.12)

Exercises 295

The integration of (E7.12) was reduced to a shifting of the distribution

function:

∗

(x,v) = f

(x −

vt

,v) (E7.13)

∗∗

(x,v) = f

(x,v− E(x)t) (E7.14)

n−1

(x,v) = f

∗∗

(x −

vt

,v) (E7.15)

in which f

denotes the distribution function evaluated at t = nt.The

shifts are generated by interpolating f in both x and v. Periodic boundary

conditions are applied at boundaries in the x direction while in v the dis-

tribution function is assumed to vanish at the boundaries. The procedure is

straightforward for a 2D phase space.

Construct a 1D Vlasov code and use it to study the evolution of a Lang-

muir wave in time. In particular estimate the Landau damping and compare

this estimate with the predicted damping. Figure 7.18 is output from a 1D

code showing the time evolution of a Langmuir wave.

Collisional kinetic theory

8.1 Introduction

In Section 7.1 we used a simple heuristic argument to obtain the collisional kinetic

equation

∂ f

∂t

+ v ·

∂ f

∂r

∂ f

∂v



∂ f

∂t



(8.1)

in which the left-hand side is the same as in the Vlasov equation and the right-

hand side, in some way yet to be determined, represents the rate of change of the

distribution function, f , due to collisions. We then argued that, since in plasmas

the force, F, includes the self-consistent electric ﬁeld which gives rise to plasma

oscillations, the frequency typical of non-collisional changes in f is ω

. Thus, a

dimensional comparison of the left- and right-hand sides of (8.1) suggests that

collisional effects may be ignored provided that the collision frequency ν

 ω

which is almost always the case. This is, of course, no more than a hand-waving

argument and, in this chapter, we shall examine this matter more carefully.

To begin with we may ask what is meant by the collision frequency. We shall

discover that there are, in fact, several collision frequencies, differing by many or-

ders of magnitude, and that the choice of an appropriate one depends on what kind

of collisions have the greatest inﬂuence on the physical effects under investigation.

One effect, for which collisions are crucial, is the establishment of thermody-

namic equilibrium in a plasma. This was touched on in Chapter 3 where ν

−1

was

the time scale for the distribution function to relax to its minimum energy state, the

Maxwell distribution. Aside from this, the main area of plasma physics in which

collisions are important is transport theory. Matter, momentum and energy may be

transferred by the action of collisions. Since plasma heating and containment are

crucial goals of controlled thermonuclear reactor physics, a proper understanding

of plasma transport is fundamental to the success of this programme.

296