Boyd T.J.M., Sanderson J.J. The Physics of Plasmas
Подождите немного. Документ загружается.

5.2 Magnetic relaxation and reconnection 147
2L
B
B
B
B
u
u
2d
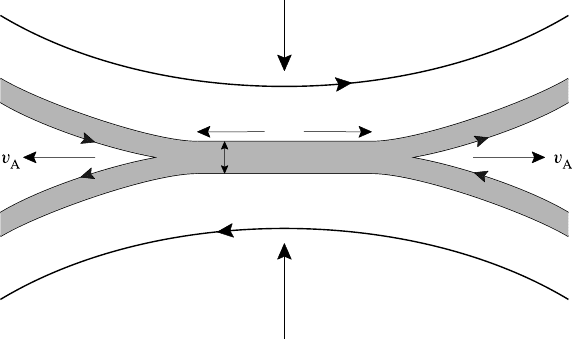
Fig. 5.4. Driven reconnection at X-type neutral point.
current sheet by the plasma flow is balanced by the rate of ohmic dissipation so
that
u = η/2µ
0
d (5.4)
This equation combined with the expression of mass conservation gives
u = v
A
/S
1/2
d = L/S
1/2
(5.5)
Identifying M
dr
= u/v
A
= M
A
(the Alfv
´
en Mach number) as a dimensionless
index for the driven reconnection rate, it follows from (5.5) that M
dr
= S
−1/2
.
From Table 5.1 we find reconnection that is many orders of magnitude too slow
to characterize the evolution of solar flares. By the same token an inverse aspect
ratio d/L ∼ 10
−6
implies a current sheet only metres thick for typical values of L,
which is unrealistic.
The realization that the Sweet–Parker reconnection rate was much too slow
pointed to the need for incorporating some faster mechanism into the current sheet
model. Petschek (1964) attempted to do this by means of a slow MHD shock model
which again involves a current sheet but leads to a reconnection rate now effectively
independent of resistivity. Petschek reasoned that the magnetic fields would meet
at a relatively narrow apex, rather than across the entire region envisaged in the
Sweet–Parker model and found a maximum reconnection rate
M
dr
∼ (ln S)
−1
(5.6)
This weak dependence on resistivity seemed to fit the bill and led to the model
being widely adopted despite a longstanding debate about its validity, over issues
148 Resistive magnetohydrodynamics
such as precisely how to define a Petschek regime and the boundary conditions
governing it. These misgivings were strengthened by insights gained from nu-
merical experiments on reconnection by Biskamp (1986). Biskamp (1993) has
given a detailed critique of the Petschek slow-shock model. On general grounds
Petschek’s model has been seen as counter-intuitive in that two plane regions of
highly conducting plasma with oppositely directed magnetic fields pushed together
might be expected to generate a flat current sheet configuration rather than the
cone required by the Petschek model. However Biskamp’s criticism centres on
the treatment of the diffusion region where a boundary layer solution, matching
the ideal MHD solution outside the diffusion zone to the resistive MHD solution
within, is required. Biskamp’s numerical experiments of driven reconnection do
not show a Petschek-like configuration in the small η limit. Although features
characteristic of slow shocks are confirmed by the simulations, Biskamp found
that as the reconnection rate increases, both the length and width of the diffusion
region increase, counter to Petschek’s predictions.
Whatever doubts persist over models for magnetic reconnection in solar flares,
observations by Innes et al. (1997) have provided the first direct evidence for
reconnection. They report ultraviolet observations of explosive events in the so-
lar chromosphere which point to the presence of oppositely directed plasma jets
ejected from small sites above the solar surface. Observations of these jets show
signs of some anisotropy in that jets directed away from the solar surface may
stream freely up to the corona while downward jets should suffer attenuation on
account of the increasing density of the chromosphere. The stream exhibiting a
blue shift, indicative of plasma flowing away from the solar surface, is of very
much greater extent than the red stream.
5.3 Resistive instabilities
The ability of a plasma, through magnetic reconnection, to reach lower energy
states means that ideal MHD stability theory needs re-examination. Modification
of the theory by the introduction of a small but finite resistivity leads to the dis-
covery of new instabilities. These resistive instabilities were first derived in the
seminal paper of Furth, Killeen and Rosenbluth (1963). In this paper the resistive
MHD equations were solved in the boundary layer in which S 1 and field line
diffusion takes place; the ideal MHD equations were solved outside this region
and the solutions matched at the boundary. Three instabilities were discovered
with growth times much smaller than τ
R
but much greater than τ
A
. One of these,
the tearing instability, arises spontaneously while the others are driven instabili-
ties.
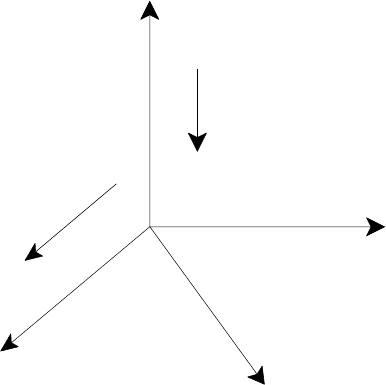
5.3 Resistive instabilities 149
g
x
y
z
k
B
0
(y)
Fig. 5.5. Coordinate axes for calculation of resistive instabilities.
A discussion of the original calculation may be found in Miyamoto (1989) and
accounts of both the linear and non-linear theory of resistive instabilities have been
given by Bateman (1978) and by White (1983). In what follows we make no at-
tempt to repeat details of the full calculation in the original paper but derive instead
the basic equations from which the linear dispersion relation is obtained and, in
the spirit of Wesson (1981), use heuristic arguments to determine the parametric
dependence of the linear growth rates.
The procedure followed is close to that used in Chapter 4, in discussing linear
stability in ideal MHD, first obtaining (4.67) and then (4.111) for the Rayleigh–
Taylor instability. We use the geometry illustrated in Fig. 5.5 and again assume
that equilibrium quantities vary only in the y direction. Incompressibility is also
assumed so that (4.107) holds. This is justified since the growth rates of the resistive
instabilities are very small on the hydromagnetic time scale τ
A
and this means that
fluid and magnetic pressure changes tend to be compensating, having a negligible
effect on the dynamics of the instabilities. Two generalizations of the assumptions
used to derive (4.111) are required. However, one of these, the replacement of the
ideal by the resistive equation for P, turns out to be of no consequence since we
shall eliminate the ∇P term.
The other generalization is important since it introduces the driving term for one
of the resistive instabilities. We want to allow for variable resistivity in which case,
following the usual derivation of the induction equation from the Maxwell equation
150 Resistive magnetohydrodynamics
and Ohm’s law, we get
∂B
∂t
=−∇ × E
= ∇ × (u × B) − ∇ ×
η
µ
0
∇ × B
= ∇ × (u × B) +
η
µ
0
∇
2
B − ∇η ×
(∇ × B)
µ
0
(5.7)
Since we are now treating η as a variable we need an extra equation to determine
it and for this we assume that it does not change within the fluid element but only
on account of its advection so that
Dη
Dt
=
∂η
∂t
+ u · ∇η = 0 (5.8)
Linearizing (5.7) and (5.8) we have
∂B
1
∂t
= ∇ × (u
1
× B
0
) +
η
0
µ
0
∇
2
B
1
− ∇η
1
×
(∇ × B
0
)
µ
0
− ∇η
0
×
(∇ × B
1
)
µ
0
(5.9)
and
∂η
1
∂t
=−u
1
· ∇η
0
(5.10)
Integrating (5.10) gives
η
1
=−ξ
y
η
0
(y) (5.11)
where we have used (4.64) and the prime denotes differentiation with respect to y.
Now substituting (5.11) in (5.9), the y component is
∂ B
y
∂t
= i(k ·B
0
)u
y
+
η
0
µ
0
∇
2
B
y
− i
(k · B
0
)
µ
0
η
0
ξ
y
where we have dropped the subscript 1 on first-order variables. Assuming, as in
our discussion of the Rayleigh–Taylor instability, that all variables are of the form
A(y)e
ikz+γ t
this equation may be integrated to obtain
B
y
= i(k ·B
0
)ξ
y
+
η
0
µ
0
γ
(B
y
− k
2
B
y
) − i
(k · B
0
)
µ
0
γ
η
0
ξ
y
(5.12)
This is one of the basic equations from which the dispersion relation is obtained.
The second equation comes from the linearized equation of motion which is
ρ
0
∂u
1
∂t
=−∇ P
1
+ ρ
1
g +
1
µ
0
(∇ × B
1
) × B
0
+
1
µ
0
(∇ × B
0
) × B
1
(5.13)

5.3 Resistive instabilities 151
and the linearized continuity equation
∂ρ
1
∂t
=−u
1
· ∇ρ
0
(5.14)
where, since incompressibility is assumed, we have used ∇ · u
1
= 0. Integrating
(5.14) gives
ρ
1
=−ξ
y
ρ
0
and substituting this in (5.13) the y and z components are
ρ
0
γ
2
ξ
y
=−
dP
1
dy
+ ξ
y
ρ
0
g −
1
µ
0
[B
x
B
0x
+ B
z
B
0z
+ B
0x
B
x
+ B
0z
(B
z
− ikB
y
)] (5.15)
ρ
0
γ
2
ξ
z
=−ikP
1
+
1
µ
0
(B
y
B
0z
− ikB
x
B
0x
) (5.16)
Now we use (4.107) for ξ
z
in (5.16) and substitute the resulting expression for
P
1
in (5.15) to get
µ
0
γ
2
[(ρ
0
ξ
y
)
− k
2
ρ
0
ξ
y
] + µ
0
k
2
gρ
0
ξ
y
=
k
2
(B
x
B
0x
+ B
z
B
0z
+ B
0x
B
x
+ B
0z
B
z
− ikB
y
B
0z
) − ik(B
y
B
0z
− ikB
x
B
0x
)
Also, since
∇ · B
1
= B
y
+ ikB
z
= 0
we may eliminate B
z
to obtain
µ
0
γ
2
[(ρ
0
ξ
y
)
− k
2
ρ
0
ξ
y
] + µ
0
k
2
gρ
0
ξ
y
= ikB
0z
B
y
− k
2
B
y
−
B
0
B
y
B
0z
= i(k · B
0
)
B
y
− k
2
B
y
−
(k · B
0
)
(k · B
0
)
B
y
(5.17)
which is the second basic equation relating ξ
y
and B
y
. It is easily verified that
putting η
0
≡ 0 in (5.12) and substituting B
y
= i (k · B
0
)ξ
y
in (5.17) reproduces
(4.111) with γ replacing iω.
5.3.1 Tearing instability
We begin our heuristic analysis of (5.12) and (5.17) by concentrating on the insta-
bility which arises by spontaneous reconnection of antiparallel field lines. The role
of the η
0
term in (5.12), like that of the g term in (5.17), is to provide a driving force
for a resistive instability. For the moment let us drop both of these terms. Now, in
152 Resistive magnetohydrodynamics
the limit of vanishing resistivity we may ignore the diffusion term in (5.12) except
near the resonant surfaces where k · B
0
≈ 0 and we define the width L of the
resistive boundary layer by equating the magnitudes of the convection and diffusion
terms in (5.12)
(k · B
0
)ξ
y
∼
η
0
µ
0
γ
∇
2
B
y
∼
η
0
γρ
0
(k · B
0
)
ξ
y
where the second approximation arises from (5.17). In this we have ignored the
variation (on scale length L) of equilibrium variables compared with the variation
(on scale length L) of first-order variables and we have assumed kL 1, i.e.
the wavelength of the perturbation is much greater than the width of the boundary
layer. Then replacing ξ
y
by ξ
y
/(L)
2
and (k·B
0
) by Lk ·B
0
(0), since (k ·B
0
) = 0
at y = 0but(k ·B
0
)
= 0, we get
L ∼
γη
0
ρ
0
k
2
(B
0
)
2
1/4
(5.18)
Using (5.12) to eliminate ξ
y
from (5.17) it is clear that in the boundary layer we
have a fourth-order differential equation for B
y
whereas outside this region, where
the diffusion term is negligible, the equation reduces to second order. This is an
eigenvalue problem in which the eigenvalues, some of which lead to positive γ
and hence instability, are determined by matching the solutions at the boundaries
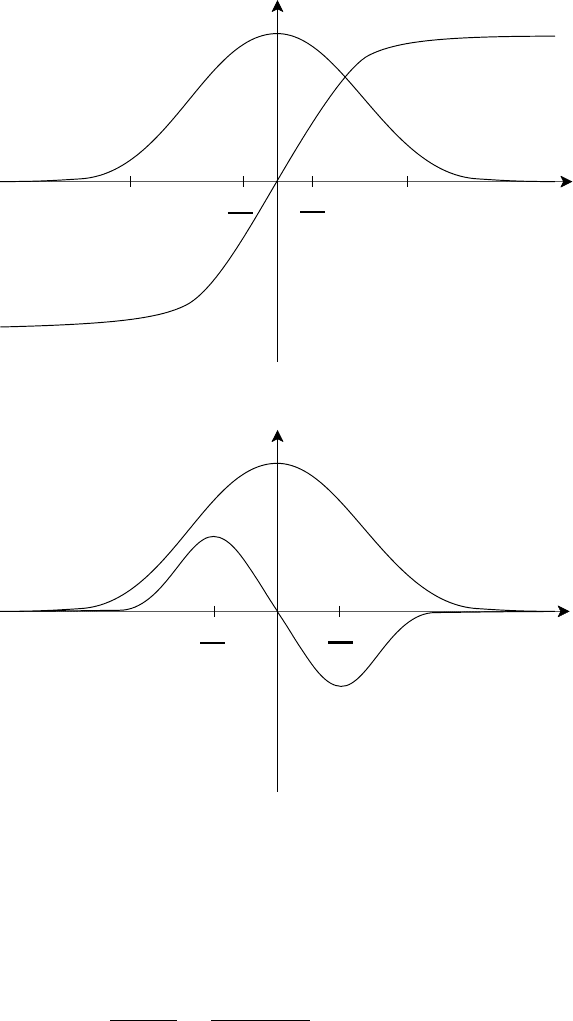
of the resistive region. Figure 5.6 shows schematically the variation of the key
quantities k · B
0
, (k · B
0
)
, B
y
and B
y
. The point to note is the rapid change in B
y
across the boundary layer which, on the scale L of the whole plasma, appears as
a discontinous change at the resonant surface (y = 0) and hence a very large B
y
.
The actual change is determined by the eigenvalue but we shall take it as given in
terms of a dimensionless quantity, usually denoted by
, and defined by
L
= lim
→0
B
y
( L/2) − B
y
(− L/2)
B
y
(0)
(5.19)
The same is true for ξ
y
so the dominant terms in (5.17) are those in ξ
y
and B
y
and
we may write
µ
0
γ
2
ρ
0
ξ
y
( L)
2
∼ (k ·B
0
)B
y
∼ (k ·B
0
)
[B
y
(L/2) − B
y
(− L/2)]
L
∼ (k ·B
0
)
B
y
(0)
L
2
(5.20)
5.3 Resistive instabilities 153
y
y
(k
.
B
0
)
k
.
B
0
-
-
ε
ε
ε
ε
L
L
L
L
2
2
2
2
-1
1
B
y
B
y
′
′
Fig. 5.6. Characteristic variation in tearing instability.
in the limit → 0 on using (5.19). Also, from (5.12), B
y
∼ (k ·B
0
)ξ
y
so that (5.20)
becomes
µ
0
γ
2
ρ
0
( L)
∼
(k · B
0
)
2
L
∼ k
2
(B
0
)
2
2
L
and, substituting for L from (5.18), we find the parametric dependence of the
154 Resistive magnetohydrodynamics
j
x
> 0
j
x
< 0
B
z
B
z
k B
0
> 0
k B
0
< 0
z
y
u
u
.
.
Fig. 5.7. Directions of current, field and velocity variations in the tearing instability.
growth rate γ to be
γ ∼
k
2
(B
0
)
2
(
)
4
η
3
0
µ
4
0
ρ
0
L
4
1/5
(5.21)
In terms of the resistive diffusion and convection times τ
R
and τ
A
, this may be
written as
γ ∼
(kL)
2
(
)
4
τ
2
A
τ
3
R
1/5
∼ (kL)
2/5
S
2/5
(
)
4/5
τ
−1
R
(5.22)
showing that the time scale for the development of this instability is two-fifths
Alfv
´
enic (S/τ
R
)
2/5
and three-fifths diffusive (1/τ
R
)
3/5
and thus intermediate be-
tween these widely differing time scales. In a tokamak τ
R
would be measured
typically in seconds, τ
A
in tens of nanoseconds and γ
−1
in milliseconds. This wide
separation of time scales justifies the simple order of magnitude analysis we have
used.
The growth rate increases with resistivity, η, and with shear, (k ·B
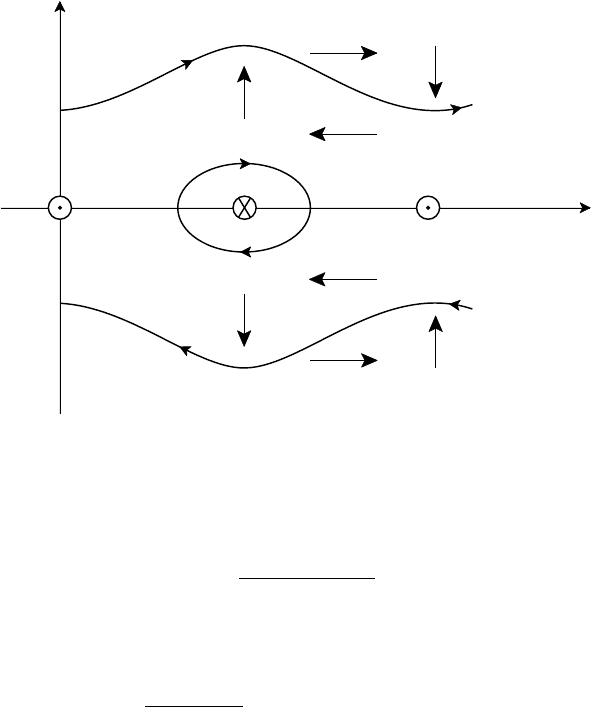
0
)
. It is driven
by the Lorentz force which acts towards the resonant surface. Finite resistivity
allows field lines to break and reconnect at the nodal X points and then contract
towards the axes of the magnetic islands passing through the O points. Figure 5.7
shows the directions of current, field and velocity perturbations. Note that the
equilibrium current j
0
, maintaining the variation in B
0
(y), is in the x direction so
the growth of the instability increases the current at the O points and decreases it at
5.3 Resistive instabilities 155
the X points causing the current to break up into filaments. The characteristics of
this instability, namely the breaking of field lines and filamentation of the current,
are reflected in the name given to it, i.e. the tearing mode.
Finally, an important feature of this instability is its dependence on
, the
discontinuity in B
y
at the resonant surface. This means that the growth rate γ
is determined by the global state of the plasma and not by the local equilibrium
conditions at the resonant surface.
5.3.2 Driven resistive instabilities
Reintroduction of the η
0
term in (5.12) and the g term in (5.17) allows additional
possibilities for instability since these terms appear in the eigenvalues. To find the
parametric dependence of the growth rates of these driven instabilities we need
only replace (5.20) by the corresponding approximations when the gravitational or
variable resistivity terms dominate the dynamics in the boundary layer.
In the case that the g term dominates we have
γ
2
ρ
0
ξ
y
( L)
2
∼ k
2
gρ
0
ξ
y
which, on substitution from (5.18), leads to
γ ∼
η
0
ρ
0
1/3
kgρ
0
B
0
2/3
∼
(kL)
2
τ
2
A
τ
R
τ
4
G
1/3
∼ (kL)
2/3
S
2/3
τ
A
τ
G
4/3
τ
−1
R
(5.23)
where τ
G
= (ρ
0
/gρ
0
)
1/2
is a gravitational time scale. Keeping only the dominant
inertial and gravitational terms in (5.17), it is easily seen that ξ
y
grows when ρ
0
> 0
or g ·∇ρ
0
< 0, i.e. when there is an inverted density gradient as for the Rayleigh–
Taylor instability. This is the gravitational interchange mode. From (5.23) we see
that, unlike the tearing mode, this instability growth rate is reduced by increased
magnetic shear, and, since it depends on ρ
0
(0), it is a local instability. A similar
resistive interchange instability may occur in a curved magnetic field when there is
a pressure gradient aligned with the curvature (see the discussion in Section 4.7.2).
Turning now to the third resistive instability, we first substitute for (B
y
− k
2
B
y
)
from (5.12) in (5.17) to bring the η
0
term into the equation of motion and then
balance this term with the inertia term to obtain
µ
0
γ
2
ρ
0
(L)
2
ξ
y
∼
(k · B
0
)(k · B
0
)
η
0
η
0
ξ
y

156 Resistive magnetohydrodynamics
Table 5.2. Resistive instability characteristics
Mode Range γτ
R
Tearing kL < 1 (kL)
2/5
S
2/5
(
)
4/5
(kL)
−2/5
S
−2/5
(
)
1/5
Gravitational g · ∇ρ
0
< 0 (kL)
2/3
S
2/3
(τ
A
/τ
G
)
4/3
(kL)
−1/3
S
−1/3
(τ
A
/τ
G
)
1/3
interchange
Rippling η
0
= 0 (kL)
2/5
S
2/5
(Lη
0
/η
0
)
4/5
(kL)
−2/5
S
−2/5
(Lη
0
/η
0
)
1/5
Hence,
γ ∼
k
2
(B
0
)
2
(η
0
)
4
µ
4
0
ρ
0
η
0
1/5
∼
Lη
0
η
0
4
(kL)
2
τ
3
R
τ
2
A
1/5
∼ (kL)
2/5
S
2/5
(Lη
0
/η
0
)
4/5
τ
−1
R
(5.24)
As for the other driven instability, this is local. To understand its physical origin
we linearize Ohm’s law, assuming E = 0, to get
η
0
j
1
+ η
1
j
0
= u
1
× B
0
and substitute for η
1
from (5.11) so that
η
0
j
1
= ξ
y
η
0
j
0
+ u
1
× B
0
Thus, there is an additional (driving) force F
d
due to the η
0
term in j
1
,givenby
F
d
= ξ
y
η
0
η
0
j
0
× B
0
= (ξ · ∇η
0
)(j
0
× B
0
/η
0
)
Assuming the variation of η
0
(y) is monotonic across the resonant surface whilst B
0
changes sign, it follows that F
d
is stabilizing on one side and destabilizing on the
other. Physically, it is clear that F
d
is destabilizing on the side of lower resistivity
since this is where the current is increased. Thus, the fluid motion is amplified only
on the lower resistivity side and this creates a rippling effect which gives the mode
its name. The motion of the plasma in relation to the field lines for both driven
instabilities is illustrated in Fig. 5.8.
Table 5.2 lists the main parametric properties of the three resistive instabilities.
The tearing mode, because it is endemic and not dependent on an imposed driving
force and since its growth rate increases with magnetic shear, is usually the most
dangerous. Also, the other two are local instabilities with the resistive interchange
mode stabilized by shear and the rippling mode stabilized by high temperature
which increases the heat conductivity and invalidates the ‘adiabatic’ assumption
(5.8).
