Blum W., Riegler W., Rolandi L. Particle Detection with Drift Chambers

Подождите немного. Документ загружается.

6 1 Gas Ionization by Charged Particles and by Laser Rays

Table 1.3 Energy W spent, on the average, for the creation of one ionization electron in various

gases and gas mixtures [CHR 71]; W

and W

are from measurements using

sources,

respectively. The lowest ionization potential is also indicated

Gas W

(eV) W

(eV) I(eV) Gas mixture

(eV)

36.4 36.3 15.43 Ar (96.5%)+C

(3.5%) 24.4

He 46.0 42.3 24.58 Ar (99.6%)+C

(0.4%) 20.4

Ne 36.6 36.4 21.56 Ar (97%)+CH

(3%) 26.0

Ar 26.4 26.3 15.76 Ar (98%)+C

(2%) 23.5

Kr 24.0 24.05 14.00 Ar (99.9%)+C

(0.1%) 22.4

Xe 21.7 21.9 12.13 Ar (98.8%)+C

(1.2%) 23.8

34.3 32.8 13.81 Kr (99.5%)+C

-2 (0.5%) 22.5

29.1 27.1 12.99 Kr (93.2%)+C

(6.8%) 23.2

26.6 24.4 11.65 Kr (99%)+C

(1%) 22.8

27.5 25.8 11.40

Air 35.0 33.8 12.15

O 30.5 29.9 12.60

The quoted concentration is the one that gave the smallest W.

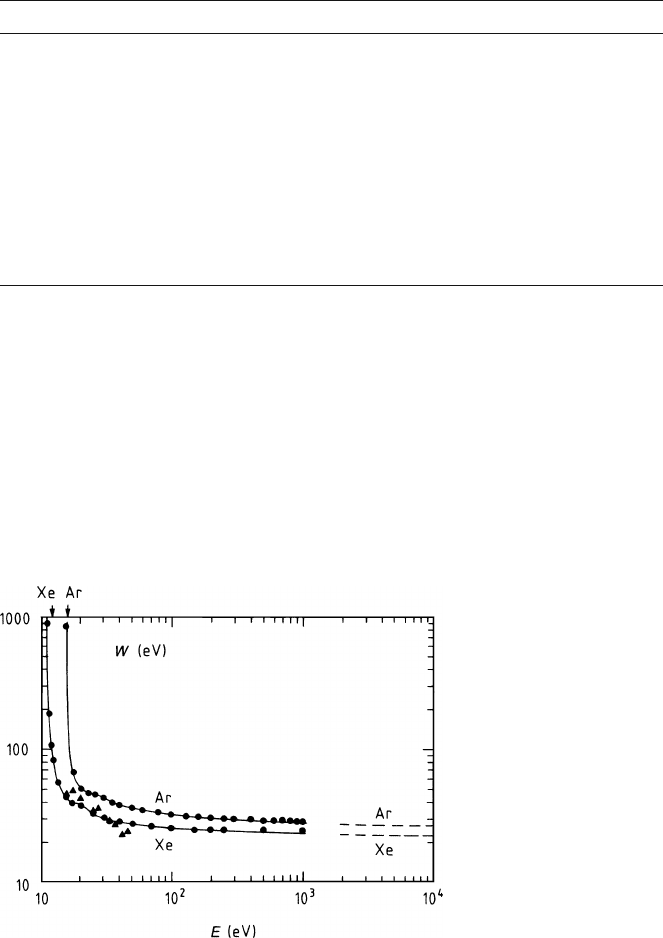
argon, the value of W for very slow electrons such as the ones emitted in primary

ionization processes (1.5) is greater than for fast electrons. Figure 1.2 contains mea-

surements reported by Combecher [COM 77].

From Table 1.3 it is apparent that the total ionization in a noble gas can be in-

creased by adding a small concentration of molecules with low ionization potential.

The extra ionization comes later, depending on the de-excitation rate of the A

∗

in-

volved. For example, a contamination of 3 ×10

−4

nitrogen in neon–helium gas has

Fig. 1.2 Average energy W spent for the creation of one ionization electron in pure argon and in

pure xenon as a function of the energy E of the ionizing particle, which is an electron fully stopped

[COM 77]. The dashed lines represent the values W

from Table 1.2 measured at larger E

1.1 Gas Ionization by Fast Charged Particles 7

caused secondary retarded ionization that amounted to 60% of the primary, with

mean retardation times around 1μs [BLU 74].

Whether the number of primary encounters that lead to ionization can be in-

creased in the same manner is not so clear; the fast particle would have to excite the

state A

∗

in a primary collision (reaction (1.6a)). A metastable state does not have a

dipole transition to the ground state and is therefore not easily excited by the fast

particle.

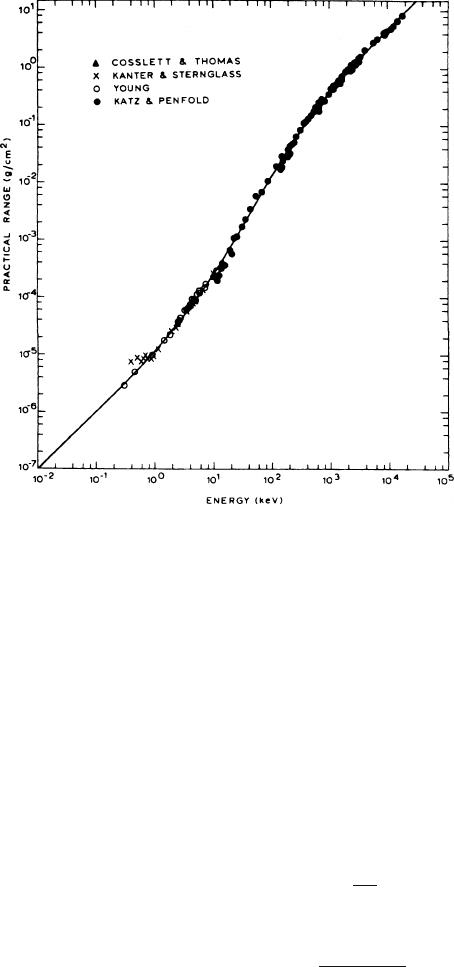
1.1.4 The Range of Primary Electrons

Primary electrons are emitted almost perpendicular to the track as their momentum

remains very small compared to the one of the track. They lose their kinetic energy

E in collisions with the gas molecules, scattering almost randomly and producing

secondary electrons, until they have lost their kinetic energy. A practical range R can

be deﬁned as the thickness of the layer of material they cross before being stopped.

The empirical relation

R(E)=AE



1−

1+CE



(1.10)

with A = 5.37 ×10

−4

gcm

−2

keV

−1

, B = 0.9815, and C = 3.1230 ×10

−3

keV

−1

shown in Fig. 1.3 and compared with experimental data in the range between 300 eV

and 20 MeV. It is shown in [KOB 68] that the parametrization (1.10) is applicable

to all materials with low and intermediate atomic number Z. In the absence of low-

energy data this curve may be taken as a basis for an extrapolation to lower E.Below

1 keV the relation reads

R(E)=9.93



μg

keV



In argon N.T.P. an electron of 1 keV is stopped in about 30μm, and one of 10 keV

in about 1.5 mm. Only 0.05% of the collisions between the particle and the gas

molecules produce primary electrons with kinetic energy larger than 10 keV. We

will compute these probabilities in Sect. 1.2.4.

1.1.5 The Differential Cross-section d

/dE

Every act of ionization is a quantum mechanical transition initiated by the ﬁeld

of the fast particle and the ﬁeld created indirectly by the neighbouring polarizable

atoms. A complete calculation would involve the transition amplitudes of all the

atomic states and does not exist.

For the detection of particles, what we have to know in the ﬁrst place is the

amount of ionization along the track and the associated ﬂuctuation phenomena. For

8 1 Gas Ionization by Charged Particles and by Laser Rays

Fig. 1.3 Practical range versus kinetic energy for electrons in aluminium. The data points are

from various authors, which can be traced back consulting [KOB 68]. The curve is a

parametrization according to (1.10)

this it is sufﬁcient to determine for an act of primary ionization with what probabil-

ities it will result in a total ionization of 1, 2,...,orn electrons. The total ionization

over a piece of track and its frequency distribution can then be determined by sum-

ming over all the primary encounters in that piece.

Using the concept of the average energy W required to produce one ion pair –

be it a constant as in (1.9) or a function of the energy of the primary electron as

in Fig. 1.2 – the problem is reduced to ﬁnding the energy spectrum F(E)dE of

the primary electrons, or, equivalently, the corresponding differential cross-section

/dE. Once this is known, we obtain λ and F(E) with the relations

1/λ =



and

F(E)=

N(d

/dE)

1/λ

where N is the electron number density in the gas and d

/dE the differential cross-

section per electron to produce a primary electron with an energy between E and

E + dE.

1.2 Calculation of Energy Loss 9

1.2 Calculation of Energy Loss

In order to calculate d

/dE we will begin by investigating the average total en-

ergy loss per unit distance, dE/dx, of a moving charged particle in a polarizable

medium. Here a classical calculation is appropriate in which the medium is treated

as a continuum characterized by a complex dielectric constant

. Later on

we will interpret the resulting integral over the lost energy in a quantum mechani-

cal sense.

1.2.1 Force on a Charge Travelling Through a Polarizable Medium

It is the ﬁeld E

long

opposite to its direction of motion, created by the moving particle

in the medium at its own space point, which produces the force equal to the energy

loss per unit distance

dE/dx = eE

long

where e is the charge of the moving particle. We follow the method of Landau and

Lifshitz [LAN 60] in the form used by Allison and Cobb [ALL 80].

Maxwell’s equations in an isotropic, homogeneous, non-magnetic medium are

∇·H = 0,

∇×E = −

∂

∇·(

E)=4

–,

∇×H =

∂

(

∂

(1.11)

Since there will be no confusion between the electric ﬁeld vector E and the energy

lost, E, we will use the customary symbols.

The charge density and the ﬂux are given by the particle moving with velocity βc:

– = e

(r −βct), j = βc–. (1.12)

We work in the Coulomb gauge and introduce the potentials

and A:

H = ∇×A,

∇·A = 0,

E = −

∂

−∇

(1.13)

Equations (1.11), expressed in terms of the potentials, are

∇·(

∇

)=−4

(r −βct),

−∇

A = −

∂



∂



∂

(

∇

)+4

eβ

(r −βct).

(1.14)

10 1 Gas Ionization by Charged Particles and by Laser Rays

The solutions can be found in terms of the Fourier transforms

(k,

) and A(k,

)

of the potentials.

The Fourier transform F(k,

) of a vector ﬁeld F(r, t) is given by

F(k,

)



r dtF(r,t) exp(ik ·r −i

t),

F(r,t)=

)



k d

F(k,

)exp(ik ·r −i

t).

(1.15)

The solutions of (1.14) are

(k,

)=2e

(

−k ·βc)/k

A(k,

)=2e

k/k

c −β

(−k

εω

)

(

−k ·βc).

(1.16)

Using the third of (1.13), the electric ﬁeld for every point is calculated according to

E(r,t)=

)



{A(k,

) −ik

(k,

)exp[i(k ·r −

t)]}d

k d

, (1.17)

and the energy loss per unit length is

dE/dx = eE(βct,t) ·β/

, (1.18)

which is independent of t because the ﬁeld created in the medium is travelling with

the particle. This may be seen by inserting (1.16) into (1.17).

In the evaluation of (1.17) with the help of (1.16) we integrate over the two

directions of k using the fact that the isotropic medium has a scalar

. We further

use

(−

∗

(

) and obtain ﬁnally





∞



∞







−



×Im

−k

εω

−





. (1.19)

The lower limit of the integral over k depends on the particle velocity

c and is

explained later.

The energy loss is determined by the manner in which the complex dielectric

constant

depends on the wave number k and the frequency

. Once

(k,

) is

speciﬁed, dE/dx can be calculated for every

;

(k,

) is, in principle, given

by the structure of the atoms of the medium. In practice, a simplifying model is

sufﬁcient.

1.2 Calculation of Energy Loss 11

1.2.2 The Photo-Absorption Ionization Model

Allison and Cobb [ALL 80] have made a model of

(k,

) based on the measured

photo-absorption cross-section

(

). A plane light-wave travelling along x is at-

tenuated in the medium if the imaginary part of the dielectric constant is larger than

zero. The wave number k is related to the frequency

k =

√

εω

/c (

+ i

). (1.20)

It causes a damping factor e

−

x/2

in the propagation function and e

−

in the inten-

sity with

= 2(

/c)Im

√

≈ (

/c)

. (1.21)

The second equality holds if

−1,

 1 such as for gases.

In terms of free photons traversing a medium that has electron density N and

atomic charge Z, the attenuation is given by the photo-absorption cross-section

(

≈

(

). (1.22)

The cross-section

(

), and therefore

(

), is known, for many gases, from mea-

surements using synchrotron radiation. The real part of

is then derived from the

dispersion relation

(

) −1 =

∞



(x)

−

dx (1.23)

(P = principal value). Figures 1.4 and 1.5 show graphs of

and

for argon.

In the quantum picture, the (

,k) plane appears as the kinematic domain of en-

ergy E =

and momentum p =

hk exchanged between the moving particle and

the atoms and electrons of the medium. The exchanged photons are not free, not

‘on the mass shell’, i.e. they have a relation between E and p that is different from

E = pc/

√

implied by (1.20). Their relationship may be understood in terms of the

kinematic constraints: for example, the photons exchanged with free electrons at

rest have E = p

/2m, the photons exchanged with bound electrons (binding energy

, approximate momentum q)haveE ≈ E

+(p + q)

/2m. The minimum momen-

tum transfer at each energy E depends on the velocity

of the moving particle and

is equal to p

min

= E/

c. It delimits the kinematic domain and is the lower limit of

integration in the integral (1.19).

Figure 1.5 presents a picture of the (E, p) plane. The solution of the integral

(1.19) requires a knowledge of

(k,

) over the kinematic domain. From (1.22) and

(1.23) it is known only for free photons, i.e. outside, along the line p

in Fig. 1.6.

The model of Allison and Cobb (photo-absorption ionization, or PAI model) con-

sists in extending the knowledge of

into the kinematic domain. For small k in the

resonance region below the free-electron line, they take

(k,

(

), (1.24)

12 1 Gas Ionization by Charged Particles and by Laser Rays

Fig. 1.4 Total photo-ionization cross-section of Ar as a function of the photon energy, as

compiled by Marr and West [MAR 76]. The imaginary part of the dielectric constant is calculated

from this curve using (1.22)

independent of k and equal to the value derived by (1.22) and (1.23). On the

free-electron line, they take a

function to represent point-like scattering in the

absorption:

(k,

)=C

(

−k

/2m). (1.25)

The normalizing constant C is determined in such a way that the total coupling

strength satisﬁes the Bethe sum rule for each k:



ωε

(k,

) d

. (1.26)

For a justiﬁcation of this procedure and a more detailed discussion, the reader is

referred to the original article [ALL 80].

Fig. 1.5 The real part of

as a function of E, calculated from Fig. 1.4 using (1.23) [LAP 80]

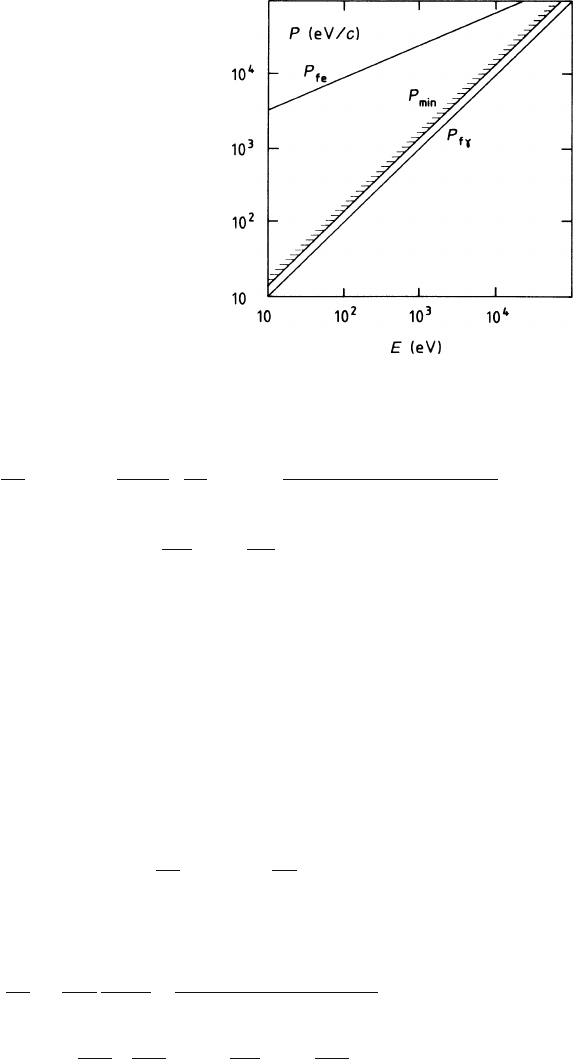
1.2 Calculation of Energy Loss 13

Fig. 1.6 Kinematic domain of

(

,k) or (E, p) of the electro-

magnetic radiation exchanged

between the fast particle (

)

and the medium. The mini-

mum momentum exchanged

is p = E/

c; the momentum

exchanged to a free electron

is p

√

2mE/c

. The mo-

menta exchanged with bound

electrons are smeared out

around the free-electron line;

min

delimits the physical

domain, p

is the free pho-

ton line

Using (1.22–1.25), we are now able to integrate expression (1.19) for dE/dx

over k. What remains is an integral over





∞





(

)ln

2mc

[(1−

)

]

1/2



−





(



. (1.27)

Here we have obtained the energy loss per unit path length in the framework of the

electrodynamics of a continuous medium, although our knowledge of

(k,

) was

actually inspired by a picture of photon absorption and collision.

At this point we leave the frame given by the classical theory and recognize the

energy loss as being caused by a number of discrete collisions per unit length, each

with an energy transfer E =

. Therefore, we reinterpret the integrand of (1.27)

to mean E times a probability of energy transfer per unit path per unit interval of

E. Now a collision probability per unit path is a cross-section times the density N.

Therefore, we write the integral (1.27) in the form





∞



h d

. (1.28)

This gives us the differential energy transfer cross-section per electron:

(E)

2mc

E[(1 −

)

]

1/2

⎡

⎣



−







)dE



⎤

⎦

. (1.29)

14 1 Gas Ionization by Charged Particles and by Laser Rays

The spectrum of energy transfer is determined by expression (1.29). The normalized

differential probability per unit energy is

F(E)=

N(d

/dE)



N(d

/dE)dE

, (1.30)

where N is the number of electrons per unit volume;

+ i

is to be obtained

using (1.22) and (1.23);

= arg(1 −

+ i

); and

is the ﬁne-structure

constant.

The number of primary encounters per unit length is given by

1/λ =



dE. (1.31)

In the gas of a drift chamber the largest possible energy transfers cause secondary

electron tracks which do not belong to the primary track. Depending on the exact

method of observation, there is always an effective cut-off E

max

for the observable

energy transfer, which is independent of

. For simplicity, we keep the ‘∞’asthe

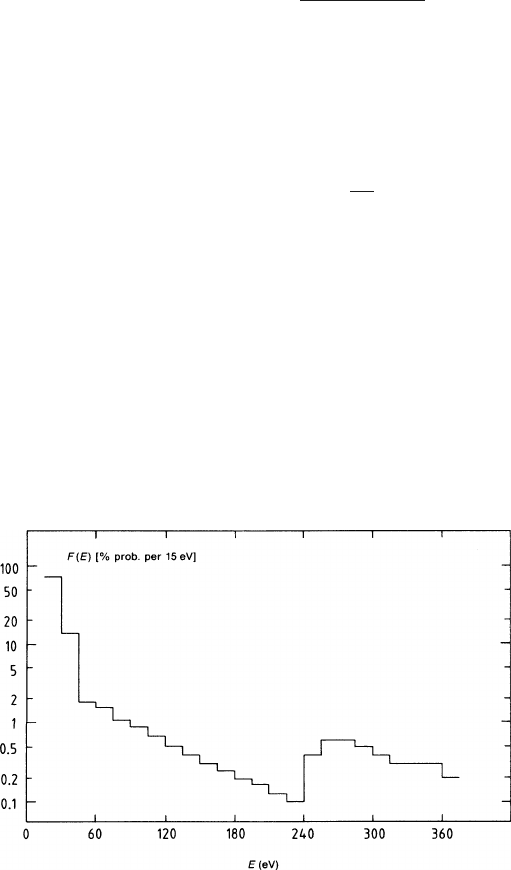
upper limit of integration. Figure 1.7 shows the energy spectrum for argon accord-

ing to a numerical calculation along this line by Lapique and Piuz [LAP 80]; they

have evaluated the model of Chechin et al. [CHE 72, ERM 77], which is very sim-

ilar to the PAI model. The second peak beyond E = 240eV, which is due to the

contribution of the L shell, is easily visible.

Fig. 1.7 Distribution of energy transfer calculated by Lapique and Piuz for argon at

= 1000,

based on the formula by Chechin et al. [CHE 76], similar to our (1.29). Adapted from Table 1.1 of

[LAP 80]

1.2 Calculation of Energy Loss 15

1.2.3 Behaviour for Large E

For energies above the highest atomic binding energy E

, the fast particle undergoes

elastic scattering on the atomic electrons as if they were free, and (1.29) becomes

the differential cross-section for Rutherford scattering on one electron. Using (1.26)

and (1.27) we ﬁnd

→

(E  E

), (1.32)

where r

is the classical electron radius, equal to e

/mc

= 2.82 ×10

−13

cm. This

happens because the third term in (1.29) is the only one surviving at large E, where

(E) vanishes quickly so that the sum rule (1.26) applies, together with (1.22).

This behaviour at large E means that the energy spectrum F(E) has an extremely

long tail. Although



F(E)dE converges, the mean transferred energy per collision,

E, has a logarithmic divergence,

E =



EF(E)dE =



dE/E ∝ logE. (1.33)

This requires a careful interpretation of the mean energy transfer, as shown in the

following sections. There is no danger that the mean transferred energy E diverges

in the practical application because there is always an upper cut-off for E at work in

the integral (1.33), which depends on the situation. This is discussed in Sec. 1.2.8.

1.2.4 Cluster-Size Distribution

An effective description of the ionization left by the particle along its trajectory is

provided by a probability distribution of the number of electrons liberated directly

or indirectly with each primary encounter. It is known under the name cluster-size

distribution, because the secondary electrons are usually created in the immediate

vicinity of the primary encounter and, together with the primary electrons, form

clusters of one or several – sometimes many – electrons. Although the secondary

electrons are not always so well localized, we will use this name.

In order to calculate the cluster-size distribution P(k) we need to know the spec-

trum of energy loss, F(E)dE, and, for each E, the probability p(E, k) of producing

exactly k ionization electrons. The cluster-size distribution is obtained by integration

over the energy:

P(k)=



F(E)p(E,k)dE. (1.34)

We may also form the integrated probability Q( j) that a cluster has more than j

electrons:

Q( j)=1 −

∑

k=1

P(k). (1.35)