Blank B.E., Krantz S.G. Calculus: Single Variable
Подождите немного. Документ загружается.


Estimating the
Remainder Term
(Section 8.8)
The remainder term R
N
satisfies the estimate
jR
N
ðxÞj# M
jx 2 cj
N11
ðN 1 1Þ!
where M is the maximum value of jf
(N 1 1)
(t)j for t between c and x. The Taylor
series T(x)off converges to f (x) if and only if R
N
(x) - 0asN-N.
Taylor’s theorem together with the error estimate may be used to approximate
the values of many transcendental functions to any desired degree of accuracy.
Taylor Series
Representations of
Common
Transcendental
Functions
(Section 8.8)
Many familiar functions have convergent power series representations. For
example,
sinðxÞ 5
X
N
n50
ð21Þ
n
ð2n 1 1Þ!
x
2n11
5 x 2
1
3!
x
3
1
1
5!
x
5
2
1
7!
x
7
1
;
2N , x , N
cosðxÞ 5
X
N
n50
ð21Þ
n
ð2nÞ!
x
2n
5 1 2
1
2!
x
2
1
1
4!
x
4
2
1
6!
x
6
1
;
2N , x , N
e
x
5
X
N
n50
1
n!
x
n
5 1 1 x 1
1
2!
x
2
1
1
3!
x
3
1
1
4!
x
4
1
1
5!
x
5
1
1
6!
x
6
1
;
2N , x , N:
The function f (x) 5 (1 1 x)
α
has a particularly interesting power series repre-
sentation called the binomial series:
ð1 1 xÞ
α
5
X
N
n50
α
n
x
n
for jxj, 1
where
α
n
5
α ðα 2 1Þðα 2 n 1 1Þ
n!
:
Review Exercises for Chapter 8
1. Calculate the first five partial sums S
1
, ..., S
5
of the series
P
N
n51
6n
ðn 1 1Þ
:
2. Calculate the first five partial sums S
1
,..., S
5
of the series
P
N
n51
n 2 2
n!
:
3. Evaluate
P
N
n50
ð3=5Þ
n
:
4. Evaluate
P
N
n51
4ð2=3Þ
n
:
5. Evaluate
P
N
n52
3
n11
=7
n21
:
6. Evaluate
P
N
n52
ð21=4Þ
n11
:
7. Express 0.6313636363 . . . as the ratio of two integers.
8. Express 0.183181818 . . . as the ratio of two integers.
c In Exercises 9244, determine if the given series converges
conditionally,
converges absolutely, or diverges. b
9.
X
N
n50
ð21Þ
n
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 1
ffiffiffi
n
p
p
ð1 1
ffiffiffi
n
p
Þ
2
10.
X
N
n50
ð21Þ
n
2
n
lnð2 1 nÞ
11.
X
N
n51
ð21Þ
n
1
1 1 ð1=nÞ
2
12.
X
N
n50
ð21Þ
n
1
2 1 sinðnÞ
13.
X
N
n50
ð21Þ
n
ffiffiffiffiffiffiffiffiffiffiffiffiffi
n
2
1 1
3
p
14.
X
N
n50
ð21Þ
n
n
2
2
n
Review Exercises 715
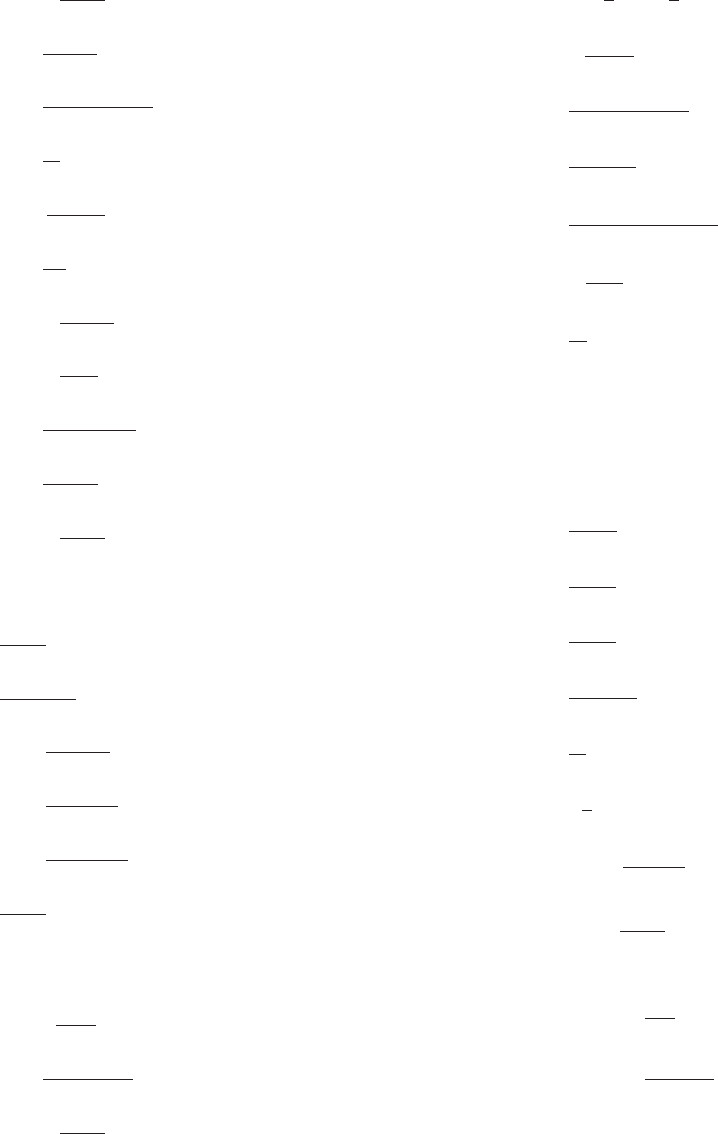
15.
X
N
n52
ð21Þ
n11
n
2
ln
4
ðnÞ
16.
X
N
n50
ð21Þ
n
1
1 1
ffiffiffi
n
p
17.
X
N
n50
ð21Þ
n
1
1 1
ffiffiffi
n
p
1 n
ffiffiffi
n
p
18.
X
N
n50
ð21Þ
n
5
n
n!
19.
X
N
n510
ð21Þ
n
1
nln
2
ðnÞ
20.
X
N
n50
ð21Þ
n
7
n
2
3n
21.
X
N
n50
ð21Þ
n11
ffiffiffi
n
p
n 2
ffiffiffi
2
p
22.
X
N
n51
ð21Þ
n11
lnðnÞ
n
23.
X
N
n51
ð21Þ
n
n
5
ð1 1 n
6
Þ
14=13
24.
X
N
n50
ð21Þ
n
2
n
n
2
1 2
n
25.
X
N
n52
ð21Þ
n11
ln
2
ðnÞ
n
3=2
26.
P
N
n51
ð21Þ
n11
sin
2
ð1=nÞ
27.
P
N
n51
ð21Þ
n11
n sin
2
ð1=nÞ
28.
X
N
n51
ð21Þ
n
n
n
29.
X
N
n50
ð21Þ
n
n 1 ð1:3Þ
n
30.
X
N
n50
ð21Þ
n
n
2=3
1 1 n
ffiffiffi
n
p
31.
X
N
n51
ð21Þ
n
arctanðnÞ
n
ffiffiffi
n
p
32.
X
N
n50
ð21Þ
n
ð1:4Þ
n
n
3
1 ð1: 2Þ
n
33.
X
N
n51
ð21Þ
n
n
1=n
34.
X
N
n51
ð21Þ
n11
ð
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
n
2
1 3n
p
2 nÞ
35.
X
N
n51
ð21Þ
n
n
n 1 1
n
2
36.
X
N
n51
ð21Þ
n
arccosð1=nÞ
n
37.
X
N
n52
ð21Þ
n11
1
lnðn
2
Þ
38.
X
N
n51
ð21Þ
n
sin
1
n
csc
2
n
39.
X
N
n50
ð21Þ
n
n13
2n 1 1
n
40.
X
N
n50
ð21Þ
n
2 4 6 ð2nÞ
ðn!Þ
2
41.
X
N
n51
ð21Þ
n
n! 2
2n21
ð2n 2 1Þ!
42.
X
N
n50
ð21Þ
n
1 3 5 ð2n 1 1Þ
2 5 8 ð3n 1 2Þ
43.
X
N
n50
ð21Þ
n11
ðn!Þ
3
ð3nÞ!
44.
X
N
n51
ð21Þ
n
n!
n
n
c In each of Exercises 45250, examine the given series and
use either the word conclusive or the word inconclusive to
complete each of the following two sentences. (a) The
Divergence Test applied to this series is __. (b) The Ratio Test
applied to this series is __. b
45.
X
N
n51
ð21Þ
n
n
2
1 1
n
2
1 n
46.
X
N
n51
ð21Þ
n
n
n
2
1 1
47.
X
N
n51
ð21Þ
n
n
n
3
1 1
48.
X
N
n51
ð21Þ
n
1
lnðn 1 1Þ
49.
X
N
n51
ð21Þ
n
5
n
n
2
50.
X
N
n51
ð21Þ
n
2
n
n
51. Estimate
P
N
n51
ð21Þ
n11
4n
3
with an error no greater than
0.002.
52. Estimate
P
N
n50
ð22Þ
n
ð2nÞ!
with an error no greater than
0.0005.
53. Use the Integral Test to determine lower and upper
estimates for
P
N
n51
1
n
3=2
:
54. Use the Integral Test to determine lower and upper
estimates for
P
N
n51
2n 1 1
ðn
2
1 nÞ
2
.
c In Exercises 55266, determine the interval of convergence
of
the given power series. b
716 Chapter
8 Infinite Series
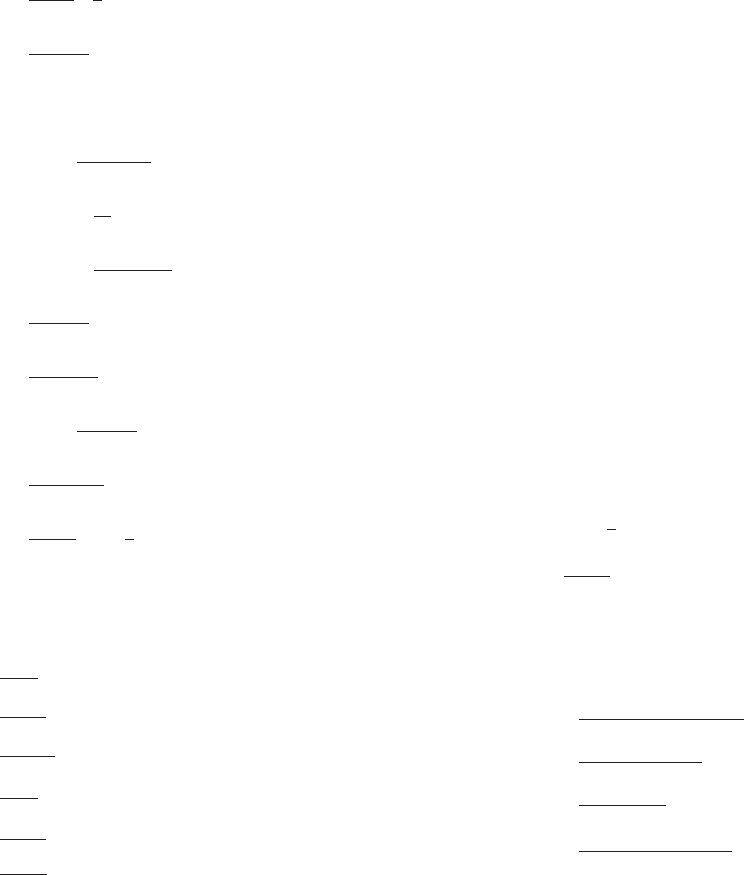
55.
P
N
n50
ð3x 1 4Þ
n
56.
X
N
n51
ð21Þ
n
ffiffiffi
n
p
x
3
n
57.
X
N
n50
3n
ðn 1 1Þ
3
x
n
58.
X
N
n51
ð1 1 1=nÞ
n
x
n
59.
X
N
n50
ð21Þ
n
x
n
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
n
4=3
1 1
p
60.
X
N
n51
ð21Þ
n11
3
n
n
3
x
n
61.
X
N
n51
ð21Þ
n11
ðx 1 1=2Þ
n
ffiffiffi
n
p
62.
X
N
n50
ð2 2 xÞ
n
2n 1 3
63.
X
N
n51
ð2x 1 3Þ
n
3
n
n
64.
X
N
n50
ð21Þ
n
ðx 1 1Þ
n
3n 1 1
65.
X
N
n52
ð21Þ
n
3
n
ln
2
ðnÞ
ð3x 2 1Þ
n
66.
X
N
n50
2
n
1 n
3
n
1 n
x 1
1
2
n
c In Exercises 67272, use the Maclaurin series for 1/(1 2 x)
together with algebra to find the Maclaurin series of the given
function. b
67.
2
3 1 x
68.
1
4 2 x
2
69.
27
81 2 x
4
70.
x
3
1 2 x
71.
x
2
1 1 x
2
72.
x
1 1 2x
c In each of Exercises 73276, approximate f (x)
at the
indicated value of x by using a Taylor polynomial of order 2
and given base point c. Use formula (8.8.3) of Theorem 1 to
express the error resulting from this approximation in terms
of a number s between c and x. b
73.
f ðxÞ5 x 2
12x
x 5 5=4 c 5 1
74.
f ðxÞ5 xe
2x
x 5 1=2 c 5 0
75. f ðxÞ5 3 2 cosðxÞ x 5 π=2 c 5 π=3
76.
f ðxÞ5
ffiffiffi
2
p
sinðxÞ x 5 π=8 c 5 π=4
c In each of Exercises 77280,
a function f, a base point c,
and a point x
0
are given.
a. Calculate
the approximation T
3
(x
0
)off (x
0
).
b. Use inequality (8.8.7) to find an upper bound for the
absolute error jR
3
(x
0
)j5 jf (x
0
)2T
3
(x
0
)j that results from
the approximation of part (a). b
77. f ðxÞ5
ffiffiffiffiffiffiffiffiffiffi
ffi
4 1 x
p
½c; x
0
5 ½0; 0:41
78. f ðxÞ5 1=x ½x
0
; c5 ½1=4; 1=2
79. f ðxÞ5 x 1 expð2xÞ½cx
0
5 ½0; 1=2
80. f ðxÞ5 lnðxÞ½x
0
; c5 ½e 2 1=2; e
c In Exercises 81284, use a Taylor polynomial to calculate
the
given integral with an error less than 10
23
. b
81.
R
0:2
0
sinðx
2
Þ dx
82.
R
0:2
0
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 1
1
2
x
3
r
dx
83.
Z
0:2
0
1
1 1 x
3
dx
84.
R
0:1
0
e
22x
2
dx
c In Exercises 85287,
use power series to calculate the
requested limit. b
85. lim
x-0
expðxÞ1 expð2xÞ2 2
x
2
:
86. lim
x-0
2 2 2cosðxÞ2 x
2
x
4
:
87. lim
x-0
xarctanðx
2
Þ
x 2 sinðxÞ
:
88. lim
x-0
expð2xÞ2 expð22xÞ
expð3xÞ2 expð23xÞ
:
Review Exercises 717
GENESIS
&
DEVELOPMENT8
Infinite Series in Ancient Greece
The concept of infinite series, like many of the mathe-
matical ideas that we study today, originated in ancient
Greece. Zeno’s Arrow paradox, which was posed in the
5th century B.C.E., arises from decomposing the num-
ber 1 into infinitely many summands by means of the
geometric series, 1 5 1/2 1 1/4 1 1/8 1 1/16 1
...
.Two
hundred years later, Archimedes, who worked precisely
and confidently with infinite processes, used the formula
1 1 1/4 1 1/16 1 1/64 1 1/256 1 5 4/3 to calculate the
area of a parabolic sector.
Nicole Oresme
With the decline of Greek mathematics, the study of
infinite series was put on hold. Progress resumed in the
Middle Ages. Nicole Oresme (ca. 13201382) was one
of the greatest of the medieval philosophers. Trained in
theology, Oresme abandoned an academic career to rise
through the ranks of the clergy, obtaining a Bishop’s
chair in 1377. Along the way, he served as financial
advisor to France’s King Charles V. In response to a
request from Charles, Oresme translated severa l of
Aristotle’s works from Latin into French. These
translations are considered to have had an important
influence on the development of the French language.
Oresme’s own writings were both extensive and
varied. He is commonly held to be the leading econo-
mist of the Middle Ages—one can make a case for a
similar status in physics. But it is Oresme’ s work in
mathematics that is best known today. It was Oresme
who first demonstrated that an infinite series can be
divergent even though its terms converge to 0. (The
proof of the divergence of the harmonic series that is
found in Section 8.1 is that of Oresme.) Oresme also
found a clever way to show that
P
N
n51
n
2
n21
5 4.
Oresme turned out to be something of an isolated
genius in medieval mathematics. He had no immediate
followers to develop his theories and extend his
mathematical work. Not all of Oresme’s work sank into
oblivion, but his work on infinite series certainly did.
M
¯
adhavan
The Indian mathematician M¯adhavan (13501425), a
near-contemporary of Oresme, also discovered
remarkable facts about infinite series. M¯adhavan’s
work was even more overlooked than that of Oresme
and remained largely unknown until its recognition
midway through the 20th century. Two examples will
suffice to show M ¯adhavan’s depth and originality:
π
ffiffiffiffiffi
12
p
5
X
N
n50
ð21Þ
n
1
ð2n 1 1Þ3
n
5 1 2
1
3 3 3
1
1
5 3 3
2
2
1
7 3 3
3
1
:::
and
π
4
5
X
N
n50
ð21Þ
n
1
ð2n 1 1Þ
5 1 2
1
3
1
1
5
2
1
7
1
::::
The simplicity of this second formula, which relates the
circular ratio π to the odd integers, is strik ing. With the
methods learned in Chapter 8, we know how to derive
such formulas, but historians do not know how
M¯adhavan himself obtained them—mathematicians
who followed M¯a dhavan recorded only the statements
of his main theorems. Nothing of M ¯adhavan’s own
mathematical writing has survived.
Infinite Series in the 17th Century
The study of infinite series began to flourish in the 17th
century. Pietro Mengoli (16261686) found the value
of the alternating harmonic series,
lnð2Þ5
X
N
n51
ð21Þ
n11
1
n
5 1 2
1
2
1
1
3
1
1
4
2
1
5
1
:::;
as did several other mathematicians. Mengoli also
reproved Oresme’s forgotten theorem concerning the
divergence of the harmonic series. M¯adhavan’s work
did not become known in the West until modern times,
but his infinite series for π/4 was rediscovered in the
second half of the 17th century. In a 1676 letter to
Newton, Leibniz communicated the formula π/4 5
1 2 1/3 1 1/5 2 1/7 1 . . . , the right side of which is now
called Leibniz’s series. Newton, who had previously
obtained similar results, responded with an astonishing
example of scientific one-upmanship. Providing only
the sketchiest of hints, Newton sent Leibniz an analo-
gous but much less transparent series involving π and
the odd natural numbers:
718

π
2
ffiffiffi
2
p
5
1
1
1
1
3
2
1
5
2
1
7
1
1
9
1
1
11
2
1
13
2
1
15
1
1
17
1
1
19
2
1
21
2
1
23
1 ::: :
Euler
A contemporary of the 18th century mathematician,
Leonhard Euler, once remarked that Euler calculated as
effortlessly as “men breathe, as eagles sustain themselves
in the air.” Euler was a master of both convergent and
divergent infinite series. One of his earliest successes was
the evaluation of a series that eluded his predecessors:
π
2
6
5
X
N
n51
1
n
2
5 1 1
1
4
1
1
9
1
1
16
1
1
25
1
::::
In fact, Euler discovered a method to evaluate all p-series
ζðpÞ5
P
N
n51
n
2p
for which p is a positive even integer.
More precisely, Euler was able to determine rational
numbers q
k
such that ζð2kÞ5 q
k
π
2k
for each positive
integer k. For example, q
1
5 1/6, ζ(2) 5 π
2
/6, q
2
5 1/90,
ζ(4) 5 π
4
/90, and q
3
5 1/945, ζ(6) 5 π
6
/945. The numera-
tors of q
1
through q
5
are all 1, but that pattern ends with
q
6
. Euler calculated explicitly as far as q
13
5 1315862/
11094481976 0305 78125.
The function ζ( p) is called the zeta function, after
the Greek letter by which it is usually denoted. Given
our knowledge of ζ(2), ζ(4), ζ(6), and so on, it is natural
to wonder about ζ(3). To this day, the p-series
P
N
n51
1=n
2k11
with odd exponents remain mysterious.
In 1978, Roger Ap
´
ery (19161994) created a sensation
when he proved that ζ(3) is irrational. Despite a great
deal of effort since then, we do not know the exact
value of ζ(3) or of any other odd power p-series.
An Infinite Series of Ramanujan
The formula of M¯adhavan and Leibniz can be used to
calculate the digits of π, but it is a very inefficient
procedure. Sum 1,000 terms of Leibniz’s series, and you
do not even get the third decimal place of π for your
effort. The grade school approximation 22/7 is very
nearly as accurate. Compare that with the formula
1
π
5
ffiffiffi
8
p
9801
X
N
n50
ð4nÞ!ð1103 1 26390nÞ
ðn!Þ
4
ð396Þ
4n
that the Indian mathematician, Srinivasa Ramanujan
(18871920), found early in the 20th century. Sum just
the first two terms of this series, and you obtain
0.3183098861837906, every digit of which agrees with
that of 1/π. Ever since Ramanujan’s untimely death,
mathematicians have been at work analyzing and
proving the mysterious equations that filled his
notebooks.
The Early History of Power Series
Before the general theory of Taylor series was devel-
oped, many particular power series expansions were
discovered and rediscovered. For example, in 1624,
Henry Briggs (15611631) derived the power series
formula
ð1 1 xÞ
1=2
5 1 1
1
2
x 2
1
8
x
2
1
1
16
x
3
2
5
128
x
4
1
:::
in the course of calculating logarithms. This formula of
Briggs is a special case of the binomial series. The
Maclaurin series of ln(1 1 x)
lnð1 1 xÞ5 x 2
1
2
x
2
1
1
3
x
3
2
1
4
x
4
1
1
5
x
5
2
1
6
x
6
1
1
7
x
7
2
:::;
was independently discovered by Johann Hudde
(16281704) in 1656, by Sir Isaac Newton in 1665, and
by Nicolaus Mercator (c. 16191687) in 1668.
James Gregory and Sir Isaac Newton
Newton derived the binomial series as well as several
other power series in 1665. These series played an
important role in his first approach to calculus. Early in
the 1670s, Newton began to correspond with the Scot-
tish mathematician, James Gregory. By 1671, Gregory
had obtained the Maclaurin series for arcsin(x),
arctan(x), tan(x), and sec(x). Until then, the series that
had been discovered—Newton’s binomial series and
the power series for the logarithm, the arcsine, and the
arctangent, in particular—had all been derived by
special techniques. An analysis of Gregory’s private
papers, however, reveal that Gregory hit upon the idea
of Taylor series in the early 1670s and used the con-
struction to derive the power series expansions of
tan(x) and sec(x). It is likely that Gregory, thinking that
he had rediscovered a method already known to
Newton, withhel d publication of his discovery of Taylor
series in order to cede the privilege of first publication
Genesis & Developement 719

to Newton. In fact, Newton did not discover Taylor
series until 1691 and that discovery, hidden in his pri-
vate papers, did not come to light until 1976!
Brook Taylor (16851731)
Brook Taylor was born into a well-to-do family with
connections to the nobility. Although he studied law at
Cambridge, his scientific career was already under way
by the time he graduated. Encouraged by contacts at
the Royal Society to develop and communicate his
discoveries, Tay lor privately disclosed the series that
now bears his name in a letter of 1712. He first publicly
communicated his work on series in his book, The
Method of Increments, which appeared in 1715. At the
time, the unpublished work of Gregory and Newton
remained unknown. Johann Bernoulli and Abraham
De Moivre had published anticipations of Taylor series
in 1694 and 1708, but they did not deny Tay lor’s claim
to priority. Moreover, Taylor’s immediate successors,
including Maclaurin and Euler, credited him not only
for introducing Taylor series, but also for demonstrat-
ing the uses to which they can be put. The term Taylor
series has been traced to 1786, after which it quickly
became established. By the mid-1800s, one writer
commented, “A single analytical formula in the
Method of Increments has conferred a celebrity on its
author, which the most voluminous works have not
often been able to bestow.” It is ironic, then, that
Taylor series were known to Gregory fourteen years
before Taylor was born and the error term, which is a
crucial compo nent of Taylor’s Theorem in practice, was
introduced by Lagrange several years after Taylor’s
death.
Colin Maclaurin (16981746)
Colin Maclaurin was born a minister’s son. Orphaned
at the age of nine, he became the ward of an uncle, who
was also a minister. The precocious boy entered the
University of Glasgow just two years later. The plan
was that he too would become a minister. At the age of
twelve, however, Maclaurin discovered the Elements of
Euclid and was led astray. Within a few days he mas-
tered the first six books of Euclid. His interest in
mathematics did not wane thereafter. Three years later,
at the age of fifteen, he defended his thesis on Gravity
and graduated from university.
The young Maclaurin had to bide his time for a few
years, but he was still only nineteen when, in 1717, he
was appointed Professor of Mathematics at the Uni-
versity of Aberdeen. In the next two years, he forged a
warm friendship with Sir Isaac Newton, a bond that
Maclaurin described as his “greatest honour and hap-
piness.” On Newton’s recommendation, the University
of Edinburgh offered Maclaurin a professorship in
1725. It came in the nick of time—Aberdeen was about
to dismiss Maclaurin for being absent without leave for
nearly three years. His teaching at Edinburgh had
happier results. One student wrote that “He made
mathematics a fashionable study.”
Maclaurin’s research also received the highest
praise. Lagrange described Maclaurin’s study of tides
as “a masterpiece of geometry, comparable to the most
beautiful and ingenious results that Archimedes left to
us.” Maclaurin’s Treatise of Fluxions, which appeared
in 1742, is considered his major work. He conceived it
as an answer to the criticisms of Bishop Berkeley that
have been discussed in Genesis and Development 2 and
3. Although Maclaurin series are to be found in the
Treatise of Fluxions, Maclaurin himself attributed them
to Taylor. Thus the terminology Maclaurin series is not
historically justified. Nonetheless, it is convenient—it
saves us from having to say “Taylor series expanded
about 0.” Furthermore, it is appropriate to remember
this great mathematician in some way. Ideas such as the
Integral Test that Maclaurin did introduce in his
Treatise do not be ar his name.
720 Chapter 8 Infinite Series

Table of Integrals
Integrals Involving x
p
1.
Z
1
x
dx 5 lnð x
jjÞ
1 C
2.
Z
x
p
dx 5
1
p 1 1
x
p11
1 C; ðp 6¼ 21Þ
3.
Z
1
x
2
dx 52
1
x
1 C
4.
Z
1
ffiffiffi
x
p
dx 5 2
ffiffiffi
x
p
1 C
Integrals Involving a 1 bx
5.
Z
1
a 1 bx
dx 5
1
b
lnð a 1 bx
jjÞ
1 C
6.
Z
ða 1 bxÞ
p
dx 5
1
ðp 1 1Þb
ða 1 bxÞ
p11
1 C; ðp 6¼ 2 1Þ
7.
Z
x
a 1 bx
dx 5
1
b
2
ða 1 bxÞ2 a lnð a 1 bxjjÞ
1 C
8.
Z
x
ða 1 bxÞ
2
dx 5
1
b
2
lnð a 1 bx
jjÞ
1
a
ða 1 bxÞ
1 C
9.
Z
x
ða 1 bxÞ
p
dx 5
1
b
2
a
ðp 2 1Þða 1 bxÞ
p21
2
1
ðp 2 2Þða 1 bxÞ
p22
1 C; ðp 6¼ 1; 2Þ
10.
Z
x
2
a 1 bx
dx 5
1
b
3
1
2
ða 1 bxÞ
2
2 2aða 1 bxÞ1 a
2
lnð a 1 bxjjÞ
1 C
11.
Z
x
2
ða 1 bxÞ
2
dx 5
1
b
3
ða 1 bxÞ2 2a lnð a 1 bx
jjÞ
2
a
2
a 1 bx
1 C
12.
Z
x
2
ða 1 bxÞ
3
dx 5
1
b
3
lnð a 1 bx
jjÞ
1
2a
a 1 bx
2
a
2
2ða 1 bxÞ
2
1 C
13.
Z
x
2
ða 1 bxÞ
p
dx 5
1
b
3
2
a
2
ðp 2 1Þða 1 bxÞ
p21
1
2a
ðp 2 2Þða 1 bxÞ
p22
2
1
ðp 2 3Þða 1 bxÞ
p23
1 C; ðp 6¼ 1; 2; 3Þ
14.
Z
x
3
a 1 bx
dx 5
1
b
4
1
3
ða 1 bxÞ
3
2
3a
2
ða 1 bxÞ
2
1 3a
2
ða 1 bxÞ2 a
3
ln
a 1 bxjj
1 C
T-1
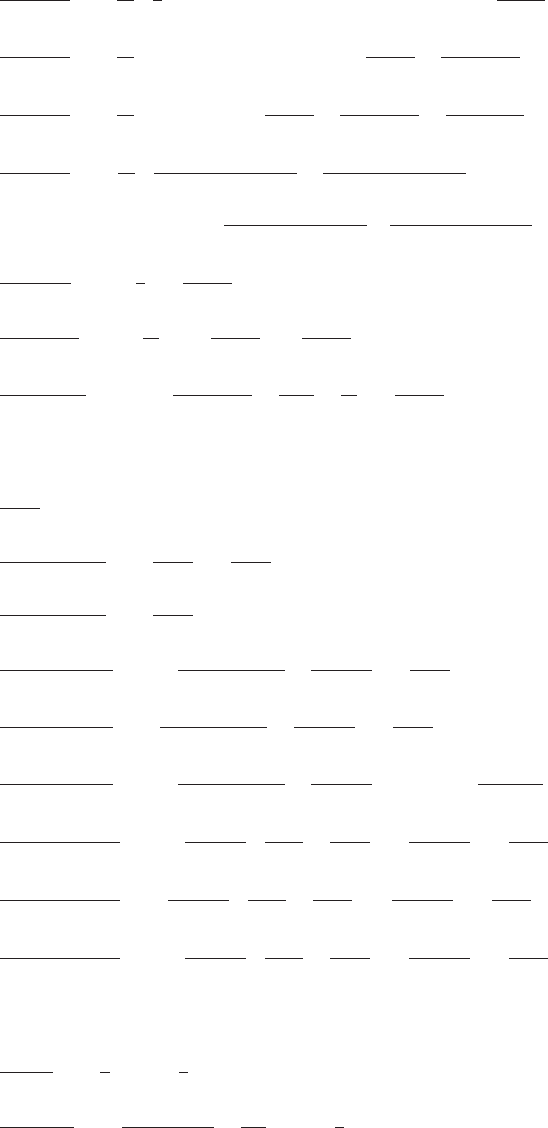
15.
Z
x
3
ða 1 bxÞ
2
dx 5
1
b
4
1
2
ða 1 bxÞ
2
2 3aða 1 bxÞ1 3a
2
ln
a 1 bxjj
1
a
3
a 1 bx
1 C
16.
Z
x
3
ða 1 bxÞ
3
dx 5
1
b
4
ða 1 bxÞ2 3a ln
a 1 bx
jj
2
3a
2
a 1 bx
1
a
3
2ða 1 bxÞ
2
1 C
17.
Z
x
3
ða 1 bxÞ
4
dx 5
1
b
4
ln
a 1 bx
jj
1
3a
a 1 bx
2
3a
2
2ða 1 bxÞ
2
1
a
3
3ða 1 bxÞ
3
1 C
18.
Z
x
3
ða 1 bxÞ
p
dx 5
1
b
4
a
3
ðp 2 1Þða 1 bxÞ
p21
2
3a
2
ðp 2 2Þða 1 bxÞ
p22
1
3a
ðp 2 3Þða 1 bxÞ
p23
2
1
ðp 2 4Þða 1 bxÞ
p24
1 C; ðp 6¼ 1; 2; 3; 4Þ
19.
Z
1
xða 1 bxÞ
dx 52
1
a
ln
a 1 bx
x
1 C
20.
Z
1
xða 1 bxÞ
2
dx 52
1
a
2
ln
a 1 bx
x
1
bx
a 1 bx
1 C
21.
Z
1
x
2
ða 1 bxÞ
2
dx 52b
1
a
2
ða 1 bxÞ
1
1
a
2
bx
2
2
a
3
ln
a 1 bx
x
1 C
Integrals Involving x 1 a and x 1 b, a 6¼b
22.
Z
x 1 a
x 1 b
dx 5 x 1 ða 2 bÞln ð x 1 bÞ1 C
23.
Z
1
ðx 1 aÞðx 1 bÞ
dx 5
1
a 2 b
ln
x 1 b
x 1 a
1 C
24.
Z
x
ðx 1 aÞðx 1 bÞ
dx 5
1
a 2 b
aln
x 1 a
jj
2 b ln
x 1 b
jj
1 C
25.
Z
1
ðx 1 aÞðx 1 bÞ
2
dx 52
1
ða 2 bÞðx 1 bÞ
1
1
ða 2 bÞ
2
ln
x 1 a
x 1 b
1 C
26.
Z
x
ðx 1 aÞðx 1 bÞ
2
dx 5
b
ða 2 bÞðx 1 bÞ
2
a
ða 2 bÞ
2
ln
x 1 a
x 1 b
1 C
27.
Z
x
2
ðx 1 aÞðx 1 bÞ
2
dx 52
b
2
ða 2 bÞðx 1 bÞ
1
a
2
ða 2 bÞ
2
lnð x 1 a
jjÞ
1
b
2
2 2ab
ða 2 bÞ
2
lnð x 1 b
jjÞ
1 C
28.
Z
1
ðx 1 aÞ
2
ðx 1 bÞ
2
dx 52
1
ða 2 bÞ
2
1
x 1 a
1
1
x 1 b
1
2
ða 2 bÞ
3
ln
x 1 a
x 1 b
1 C
29.
Z
x
ðx 1 aÞ
2
ðx 1 bÞ
2
dx 5
1
ða 2 bÞ
2
a
x 1 a
1
b
x 1 b
2
ða 1 bÞ
ða 2 bÞ
3
ln
x 1 a
x 1 b
1 C
30.
Z
x
2
ðx 1 aÞ
2
ðx 1 bÞ
2
dx 52
1
ða 2 bÞ
2
a
2
x 1 a
1
b
2
x 1 b
1
ð2abÞ
ða 2 bÞ
3
ln
x 1 a
x 1 b
1 C
Integrals Involving a
2
1 x
2
31.
Z
1
a
2
1 x
2
dx 5
1
a
arctan
x
a
1 C
32.
Z
1
ða
2
1 x
2
Þ
2
dx 5
x
2a
2
ða
2
1 x
2
Þ
1
1
2a
3
arctan
x
a
1 C
T-2 Table of Integrals
33.
Z
1
ða
2
1 x
2
Þ
3
dx 5
x
4a
2
ða
2
1 x
2
Þ
2
1
3x
8a
4
ða
2
1 x
2
Þ
1
3
8a
5
arctan
x
a
1 C
34.
Z
1
ða
2
1 x
2
Þ
4
dx 5
x
6a
2
ða
2
1 x
2
Þ
3
1
5x
24a
4
ða
2
1 x
2
Þ
2
1
5x
16a
6
ða
2
1 x
2
Þ
1
5
16a
7
arctan
x
a
1 C
35.
Z
x
2
a
2
1 x
2
dx 5 x 2 a arctan
x
a
1 C
36.
Z
x
2
ða
2
1 x
2
Þ
2
dx 52
x
2ða
2
1 x
2
Þ
1
1
2a
arctan
x
a
1 C
37.
Z
x
2
ða
2
1 x
2
Þ
3
dx 52
x
4ða
2
1 x
2
Þ
2
1
x
8a
2
ða
2
1 x
2
Þ
1
1
8a
3
arctan
x
a
1 C
38.
Z
x
2
ða
2
1 x
2
Þ
4
dx 52
x
6ða
2
1 x
2
Þ
3
1
x
24a
2
ða
2
1 x
2
Þ
2
1
x
16a
4
ða
2
1 x
2
Þ
1
1
16a
5
arctan
x
a
1 C
39.
Z
1
xða
2
1 x
2
Þ
dx 5
1
2a
2
ln
x
2
a
2
1 x
2
1 C
40.
Z
1
xða
2
1 x
2
Þ
2
dx 5
1
2a
2
ða
2
1 x
2
Þ
1
1
2a
4
ln
x
2
a
2
1 x
2
1 C
41.
Z
1
x
2
ða
2
1 x
2
Þ
dx 52
1
a
2
x
2
1
a
3
arctan
x
a
1 C
42.
Z
1
x
2
ða
2
1 x
2
Þ
2
dx 52
1
a
4
x
2
x
2a
4
ða
2
1 x
2
Þ
2
3
2a
5
arctan
x
a
1 C
Integrals Involving a
2
2 x
2
43.
Z
1
a
2
2 x
2
dx 5
1
2a
ln
x 1 a
x 2 a
1 C
44.
Z
1
a
2
2 x
2
dx 5
1
a
tanh
21
x
a
1 C
45.
Z
1
ða
2
2 x
2
Þ
2
dx 5
x
2a
2
ða
2
2 x
2
Þ
1
1
4a
3
ln
a 1 x
a 2 x
1 C
46.
Z
1
ða
2
2 x
2
Þ
3
dx 5
x
4a
2
ða
2
2 x
2
Þ
2
1
3x
8a
4
ða
2
2 x
2
Þ
1
3
16a
5
ln
a 1 x
a 2 x
1 C
47.
Z
1
ða
2
2 x
2
Þ
4
dx 5
x
6a
2
ða
2
2 x
2
Þ
3
1
5x
24a
4
ða
2
2 x
2
Þ
2
1
5x
16a
6
ða
2
2 x
2
Þ
1
5
32a
7
ln
a 1 x
a 2 x
1 C
48.
Z
x
2
a
2
2 x
2
dx 52x 1
a
2
ln
a 1 x
a 2 x
1 C
49.
Z
x
2
ða
2
2 x
2
Þ
2
dx 5
x
2ða
2
2 x
2
Þ
2
1
4a
ln
a 1 x
a 2 x
1 C
50.
Z
x
2
ða
2
2 x
2
Þ
3
dx 5
x
4ða
2
2 x
2
Þ
2
2
x
8a
2
ða
2
2 x
2
Þ
2
1
16a
3
ln
a 1 x
a 2 x
1 C
51.
Z
x
2
ða
2
2 x
2
Þ
4
dx 5
x
6ða
2
2 x
2
Þ
3
2
x
24a
2
ða
2
2 x
2
Þ
2
2
x
16a
4
ða
2
2 x
2
Þ
2
1
32a
5
ln
a 1 x
a 2 x
1 C
52.
Z
1
xða
2
2 x
2
Þ
dx 5
1
2a
2
ln
x
2
a
2
2 x
2
1 C
Integrals Involving a
2
2 x
2
T-3
53.
Z
1
xða
2
2 x
2
Þ
2
dx 5
1
2a
2
ða
2
2 x
2
Þ
1
1
2a
4
ln
x
2
a
2
2 x
2
1 C
54.
Z
1
x
2
ða
2
2 x
2
Þ
dx 52
1
a
2
x
1
1
2a
3
ln
a 1 x
a 2 x
1 C
55.
Z
1
x
2
ða
2
2 x
2
Þ
2
dx 52
1
a
4
x
1
x
2a
4
ða
2
2 x
2
Þ
1
3
4a
5
ln
a 1 x
a 2 x
1 C
Integrals Involving a
4
6 x
4
56.
Z
1
a
4
1 x
4
dx 5
1
4a
3
ffiffiffi
2
p
ln
x
2
1 ax
ffiffiffi
2
p
1 a
2
x
2
2 ax
ffiffiffi
2
p
1 a
2
1
1
2a
3
ffiffiffi
2
p
arctan
ax
ffiffiffi
2
p
a
2
2 x
2
1 C
57.
Z
x
a
4
1 x
4
dx 5
1
2a
2
arctan
x
2
a
2
1 C
58.
Z
x
2
a
4
1 x
4
dx 52
1
4a
ffiffiffi
2
p
ln
x
2
1 ax
ffiffiffi
2
p
1 a
2
x
2
2 ax
ffiffiffi
2
p
1 a
2
1
1
2a
ffiffiffi
2
p
arctan
ax
ffiffiffi
2
p
a
2
2 x
2
1 C
59.
Z
1
a
4
2 x
4
dx 5
1
4a
3
ln
a 1 x
a 2 x
1
1
2a
3
arctan
x
a
1 C
60.
Z
x
a
4
2 x
4
dx 5
1
4a
2
ln
a
2
1 x
2
a
2
2 x
2
1 C
61.
Z
x
2
a
4
2 x
4
dx 5
1
4a
ln
a 1 x
a 2 x
2
1
2a
arctan
x
a
1 C
Integrals Involving
ffiffiffi
x
p
and a
2
6 b
2
x
62.
Z
1
ða
2
1 b
2
xÞ
ffiffiffi
x
p
dx 5
2
ab
arctan
b
ffiffiffi
x
p
a
1 C
63.
Z
1
ða
2
1 b
2
xÞ
2
ffiffiffi
x
p
dx 5
ffiffiffi
x
p
a
2
ða
2
1 b
2
xÞ
1
1
a
3
b
arctan
b
ffiffiffi
x
p
a
1 C
64.
Z
ffiffiffi
x
p
a
2
1 b
2
x
dx 5
2
ffiffiffi
x
p
b
2
2
2a
b
3
arctan
b
ffiffiffi
x
p
a
1 C
65.
Z
ffiffiffi
x
p
ða
2
1 b
2
xÞ
2
dx 52
ffiffiffi
x
p
b
2
ða
2
1 b
2
xÞ
1
1
ab
3
arctan
b
ffiffiffi
x
p
a
1 C
66.
Z
x
3=2
a
2
1 b
2
x
dx 5
2x
3=2
3b
2
2
2a
2
ffiffiffi
x
p
b
4
1
2a
3
b
5
arctan
b
ffiffiffi
x
p
a
1 C
67.
Z
x
3=2
ða
2
1 b
2
xÞ
2
dx 5
2x
3=2
b
2
ða
2
1 b
2
xÞ
1
3a
2
ffiffiffi
x
p
b
4
ða
2
1 b
2
xÞ
2
3a
b
5
arctan
b
ffiffiffi
x
p
a
1 C
68.
Z
1
ða
2
2 b
2
xÞ
ffiffiffi
x
p
dx 5
1
ab
ln
a 1 b
ffiffiffi
x
p
a 2 b
ffiffiffi
x
p
1 C
69.
Z
1
ða
2
2 b
2
xÞ
2
ffiffiffi
x
p
dx 5
ffiffiffi
x
p
a
2
ða
2
2 b
2
xÞ
1
1
2a
3
b
ln
a 1 b
ffiffiffi
x
p
a 2 b
ffiffiffi
x
p
1 C
70.
Z
ffiffiffi
x
p
a
2
2 b
2
x
dx 52
2
ffiffiffi
x
p
b
2
1
a
b
3
ln
a 1 b
ffiffiffi
x
p
a 2 b
ffiffiffi
x
p
1 C
71.
Z
ffiffiffi
x
p
ða
2
2 b
2
xÞ
2
dx 5
ffiffiffi
x
p
b
2
ða
2
2 b
2
xÞ
2
1
2ab
3
ln
a 1 b
ffiffiffi
x
p
a 2 b
ffiffiffi
x
p
1 C
T-4 Table of Integrals
