Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena
Подождите немного. Документ загружается.

Problems
67
(a)
Write down the expression for R as a function of
z.
(b)
Change the independent variable in the above equation to R, so that the equation becomes
(c)
Integrate the equation, and then show that the solution can be rearranged to give
Interpret the result. The approximation used here that a flow between nonparallel surfaces
can be regarded locally as flow between parallel surfaces is sometimes referred to as the lubri-
cation approximation and is widely used in the theory of lubrication. By making a careful
order-of-magnitude analysis, it can be shown that, for this problem, the lubrication approxi-
mation is valid as long as4
2B.11
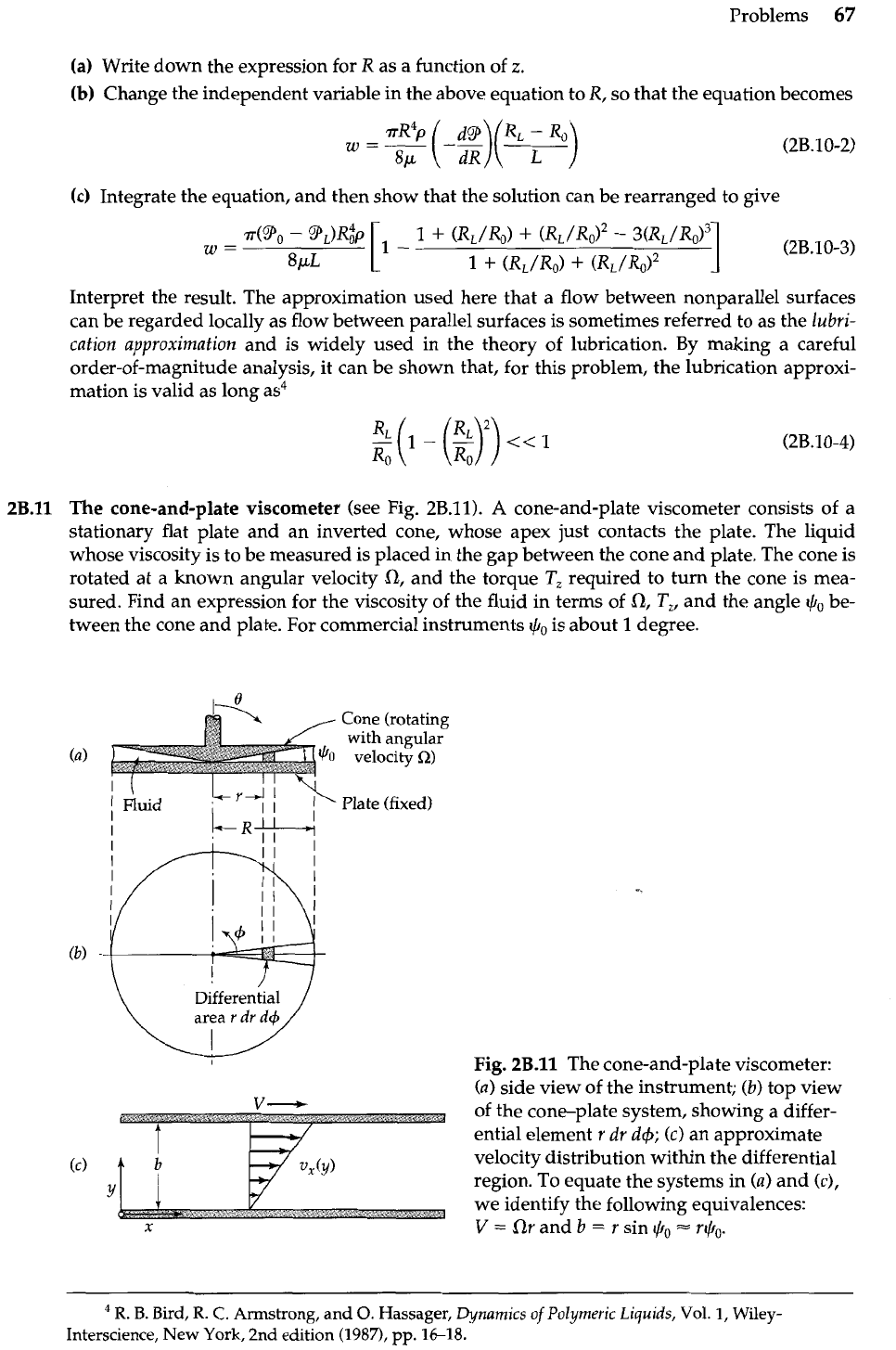
The cone-and-plate viscometer (see Fig. 2B.11).
A
cone-and-plate viscometer consists of a
stationary flat plate and an inverted cone, whose apex just contacts the plate. The liquid
whose viscosity is to be measured is placed in the gap between the cone and plate. The cone is
rotated at a known angular velocity
a,
and the torque
T,
required to turn the cone is mea-
sured. Find an expression for the viscosity of the fluid in terms of
a,
T,,
and the angle
+0
be-
tween the cone and plate. For commercial instruments
is about
1
degree.
~ifferential
,,/
/
area
r dr
Fig.
2B.11
The cone-and-plate viscometer:
(a) side view of the instrument;
(b)
top view
v-
of the cone-plate system, showing a differ-
ential element r dr d+;
(c)
an approximate
(c)
velocity distribution within the differential
Y
region. To equate the systems in (a) and
(c),
we identify the following equivalences:
V
=
Or and
b
=
r
sin
i/io
=
ri/io.
R.
B.
Bird,
R.
C.
Armstrong, and
0.
Hassager,
Dynamics
of
Polymeric Liquids,
Vol.
1,
Wiley-
Interscience, New
York,
2nd edition (19871,
pp.
16-18.
68
Chapter 2
Shell Momentum Balances and Velocity Distributions in Laminar Flow
(a)
Assume that locally the velocity distribution in the gap can be very closely approximated
by that for flow between parallel plates, the upper one moving with a constant speed. Verify
that this leads to the
approximate
velocity distribution (in spherical coordinates)
This approximation should be rather good, because
I++,
is so small.
(b)
From the velocity distribution in Eq. 28.11-1 and Appendix B.l, show that a reasonable
expression for the shear stress is
This result shows that the shear stress is uniform throughout the gap. It is this fact that makes
the cone-and-plate viscometer quite attractive. The instrument is widely used, particularly in
the polymer industry.
(c)
Show that the torque required to turn the cone is given by
This is the standard formula for calculating the viscosity from measurements of the torque
and angular velocity for a cone-plate assembly with known
R
and
rCr,.
(d)
For a cone-and-plate instrument with radius 10 cm and angle
Go
equal to 0.5 degree, what
torque
(in
dyn
.
cm) is required to turn the cone at an angular velocity of 10 radians per
minute
if
the fluid viscosity is 100 cp?
Answer:
(d)
40,000 dyn
.
cm
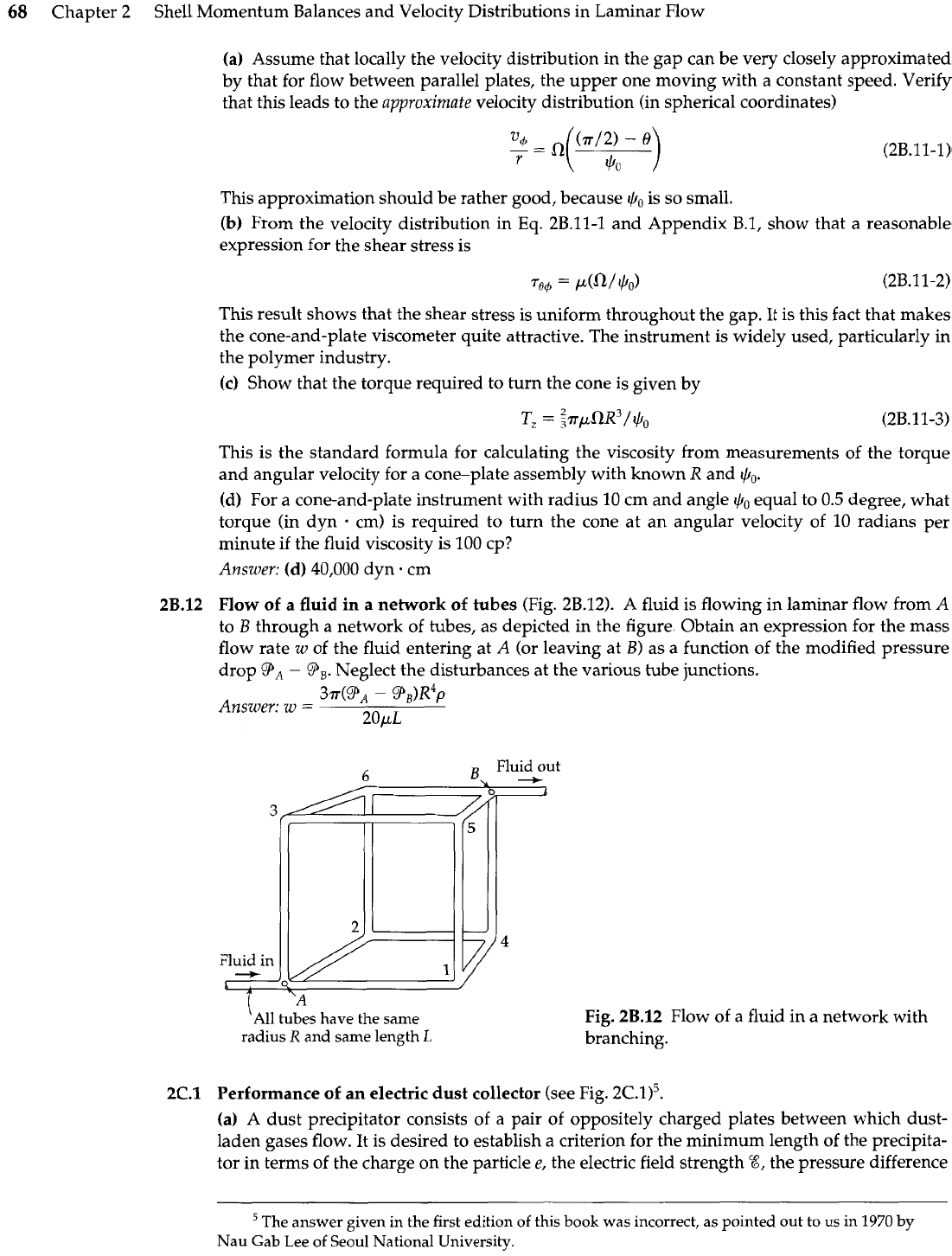
2B.12
Flow of a fluid in a network of tubes
(Fig. 2B.12). A fluid is flowing in laminar flow from
A
to
B
through a network of tubes, as depicted in the figure. Obtain an expression for the mass
flow rate
w
of the fluid entering at
A
(or leaving at
B)
as a function of the modified pressure
drop
gA
-
9,. Neglect the disturbances at the various tube junctions.
3?7(pA
-
9B)R4p
Answer: w
=
20pL
3
Fluid in
6
Fluid out
\
-
d
4
"A
All tubes have the same
radius
X
and same length
L
Fig.
2B.12
Flow of a fluid in a network with
branching.
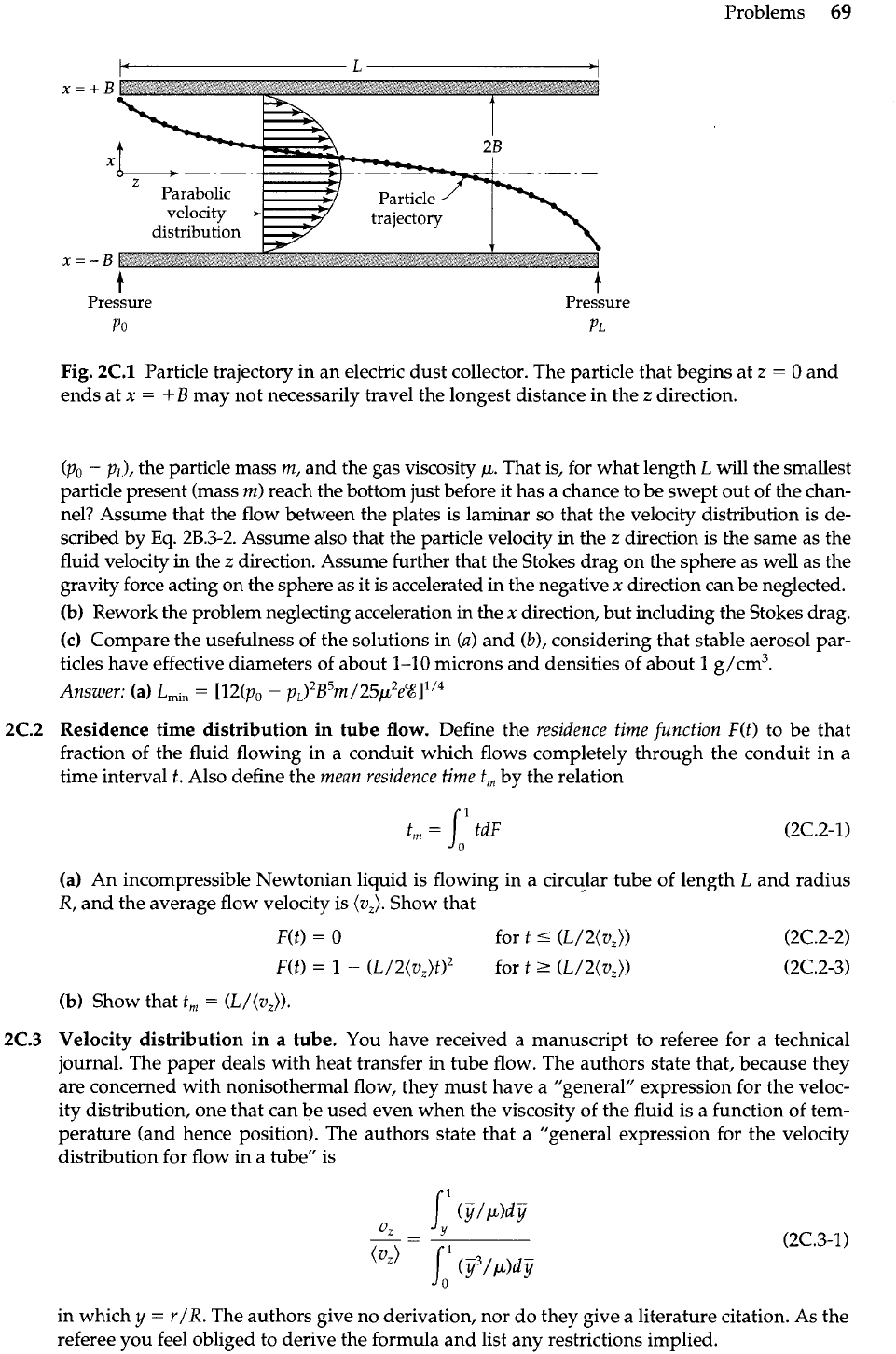
2C.1
Performance of an electric dust collector
(see Fig. 2C.U5.
(a)
A
dust precipitator consists of a pair of oppositely charged plates between which dust-
laden gases flow. It is desired to establish a criterion for the minimum length of the precipita-
tor in terms of the charge on the particle
e,
the electric field strength
%,
the pressure difference
--
The answer given in the first edition
of
this book was incorrect, as pointed out to us in
1970
by
Nau Gab Lee
of
Seoul National University.
Problems
69
X
z
Parabolic
velocity
-
trajectory
distribution
B-:,.''
'
;,
;
..;,
,.-'
,
*-:.
"
",'.
,,
,*-.',~'-(
1
+
"'
%,
f
Pressure
Po
4
Pressure
PL
Fig.
2C.1
Particle trajectory in an electric dust collector. The particle that begins at
z
=
0 and
ends at
x
=
+
B
may not necessarily travel the longest distance in the
z
direction.
(po
-
pJ,
the particle mass m, and the gas viscosity
p.
That is, for what length
L
will the smallest
particle present (mass m) reach the bottom just before it has a chance to be swept out of the chan-
nel? Assume that the flow between the plates is laminar so that the velocity distribution is de-
scribed
by
Eq.
2B.3-2. Assume also that the particle velocity in the
z
direction is the same as the
fluid velocity
in
the
z
direction. Assume further that the Stokes drag on the sphere as well as the
gravity force acting on the sphere as it is accelerated in the negative
x
direction can be neglected.
(b) Rework the problem neglecting acceleration in the
x
direction, but including the Stokes drag.
(c)
Compare the usefulness of the solutions in (a) and
(b),
considering that stable aerosol par-
ticles have effective diameters of about 1-10 microns and densities of about
1
g/cm3.
Answer: (a)
Lmi,
=
[12(po
-
pL)2~5m/25p2eCe11'4
2C.2
Residence time distribution in tube flow. Define the residence time function
F(t)
to be that
fraction of the fluid flowing in a conduit which flows completely through the conduit in
a
time interval t. Also define the mean residence time t, by the relation
(a) An incompressible Newtonian liquid is flowing in a circular tube of length
L
and radius
R,
and the average flow velocity is (v,). Show that
F(t)
=
0
for t
5
(L/2(vZ))
F(t)
=
1
-
(L/2(~,)t)~
fort
2
(L/2(vZ))
(b) Show that t,
=
(L/(v,)).
2C.3
Velocity distribution in a tube. You have received a manuscript to referee for a technical
journal. The paper deals with heat transfer in tube flow. The authors state that, because they
are concerned with nonisothermal flow, they must have a "general" expression for the veloc-
ity distribution, one that can be used even when the viscosity of the fluid is a function of tem-
perature (and hence position). The authors state that a "general expression for the velocity
distribution for flow in a tube" is
in which
y
=
r/R. The authors give no derivation, nor do they give a literature citation. As the
referee you feel obliged to derive the formula and list any restrictions implied.
70
Chapter 2
Shell Momentum Balances and Velocity Distributions in Laminar Flow
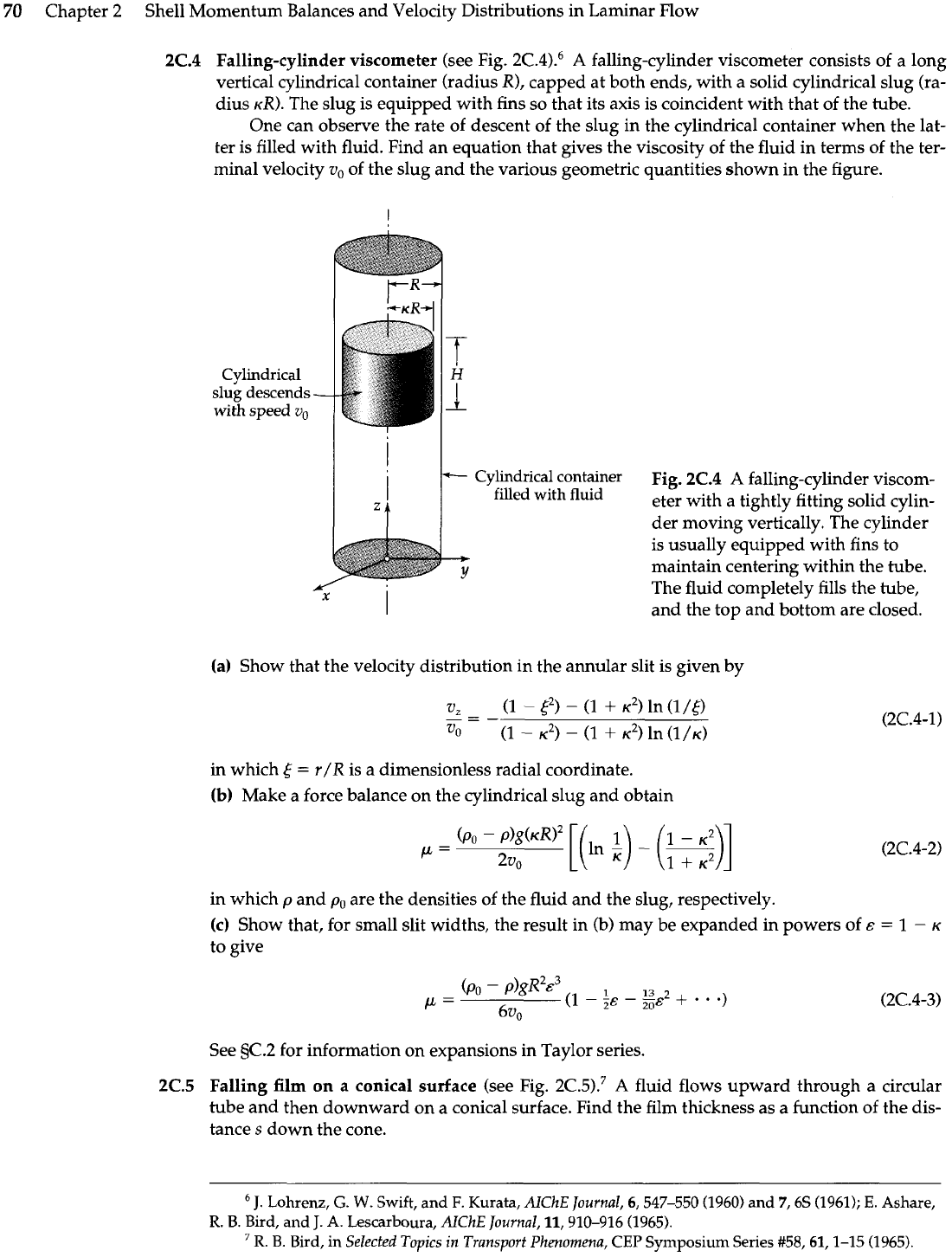
2C.4
Falling-cylinder viscometer (see Fig. 2C.4).6
A
falling-cylinder viscometer consists of a long
vertical cylindrical container (radius R), capped at both ends, with a solid cylindrical slug (ra-
dius
KR).
The slug is equipped with fins so that its axis is coincident with that of the tube.
One can observe the rate of descent of the slug in the cylindrical container when the lat-
ter is filled with fluid. Find an equation that gives the viscosity of the fluid in terms of the ter-
minal velocity
v,
of the slug and the various geometric quantities shown in the figure.
Cylindrical
slug descends
-
with speed
v,
-
Cylindrical container
Fig.
2C.4
A
falling-cylinder viscom-
with
fluid
eter with a tightly fitting solid cylin-
der moving vertically. The cylinder
is usually equipped with fins to
----t
Y
maintain centering within the tube.
The fluid completely fills the tube,
and the top and bottom are closed.
(a) Show that the velocity distribution in the annular slit is given
by
in which
5
=
r/R is a dimensionless radial coordinate.
(b)
Make a force balance on the cylindrical slug and obtain
in which
p
and
p,
are the densities of the fluid and the slug, respectively.
(c)
Show that, for small slit widths, the result in (b) may be expanded in powers of
E
=
1
-
K
to give
See sC.2 for information on expansions
in
Taylor series.
2C.5
Falling film on a conical surface (see Fig. 2C.5).7
A
fluid flows upward through a circular
tube and then downward on a conical surface. Find the film thickness as a function of the dis-
tance s down the cone.
-
--
J.
Lohrenz,
G.
W.
Swift, and
F.
Kurata,
AIChE Journal,
6,547-550 (1960) and 7,6S (1961);
E.
Ashare,
R.
B. Bird, and
J.
A. Lescarboura,
AIChE
Journal,
11,910-916 (1965).
R.
B. Bird, in
Selected Topics in Transport Phenomena,
CEP
Symposium Series #58,61,1-15 (1965).
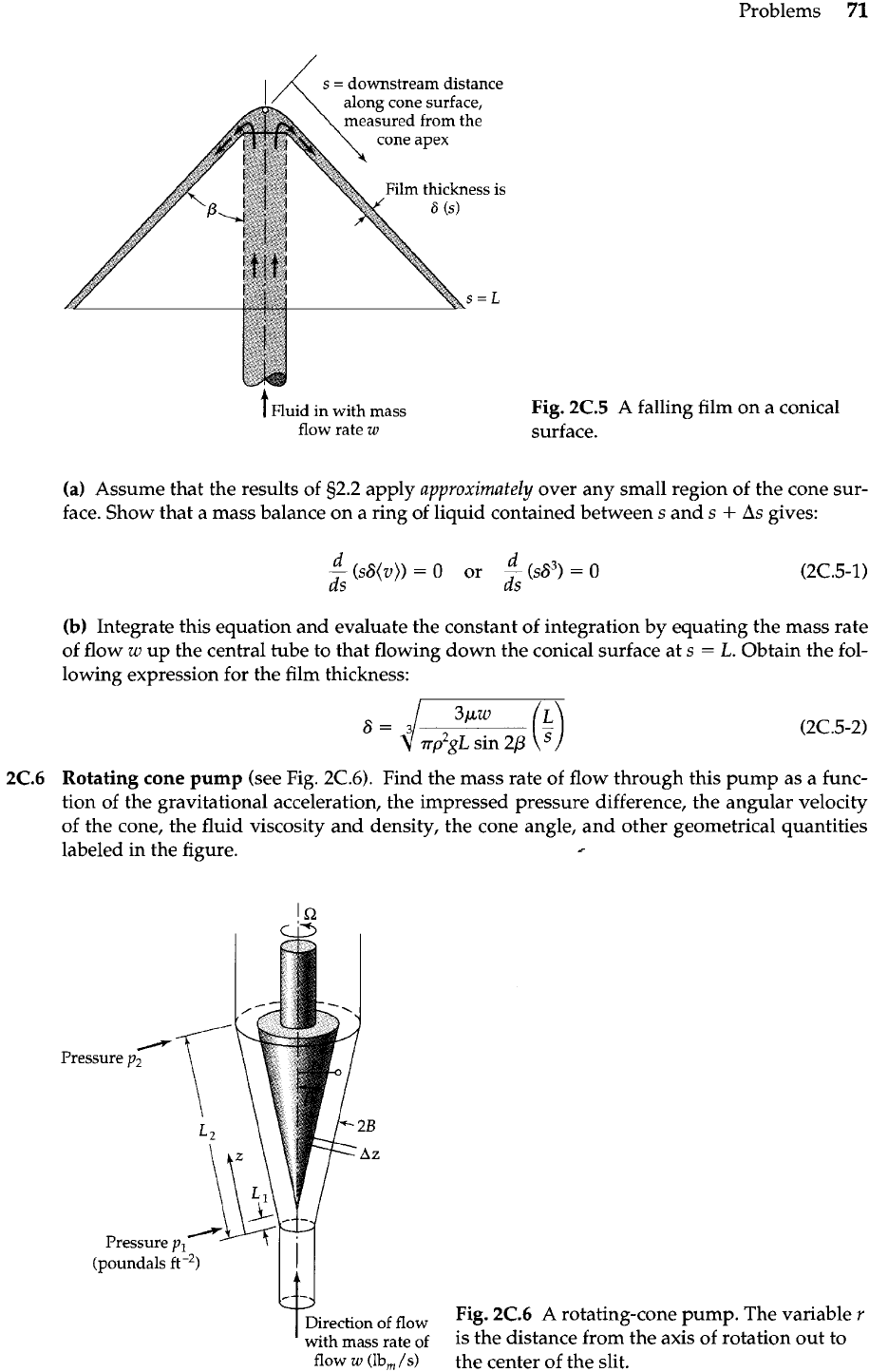
Problems
71
I
s
=
downstream distance
sured from the
ilm thickness is
t
Fluid in with mass
flow rate
w
Fig. 2C.5
A
falling film on a conical
surface.
(a)
Assume that the results of 92.2 apply
approximately
over any small region of the cone sur-
face. Show that a mass balance on a ring of liquid contained between
s
and
s
+
As
gives:
(b)
Integrate this equation and evaluate the constant of integration by equating the mass rate
of flow
w
up the central tube to that flowing down the conical surface at
s
=
L.
Obtain the fol-
lowing expression for the film thickness:
s=d
3pw
(;)
rp2gL
sin 2/3
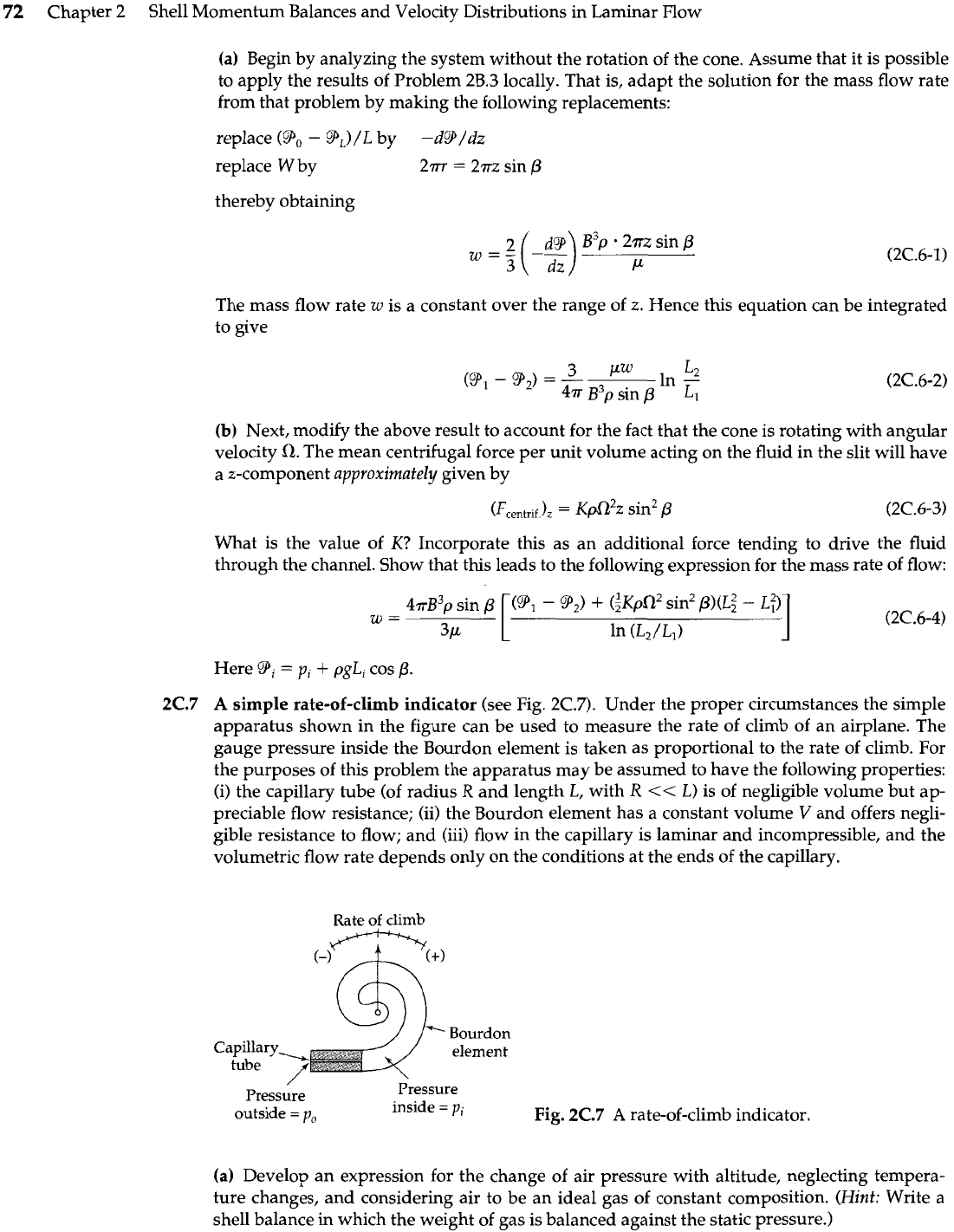
2C.6
Rotating cone
pump
(see Fig. 2C.6). Find the mass rate of flow through this pump as a func-
tion of the gravitational acceleration, the impressed pressure difference, the angular velocity
of the cone, the fluid viscosity and density, the cone angle, and other geometrical quantities
labeled in the figure.
iredion of flow
Fig. 2C.6 A rotating-cone pump. The variable
r
with mass rate of
is the distance from the axis of rotation out to
flow
w
(Ib,
/s)
the center of the slit.
72
Chapter 2
Shell Momentum Balances and Velocity Distributions in Laminar Flow
(a) Begin by analyzing the system without the rotation of the cone. Assume that it is possible
to apply the results of Problem 2B.3 locally. That is, adapt the solution for the mass flow rate
from that problem by making the following replacements:
replace
(9,
-
BJ/L
by
-dP/dz
replace
W
by 2717
=
27rz sin
/3
thereby obtaining
dp
B3p
.2.rrz
sin
p
-=5(-z)
P
The mass flow rate
w
is a constant over the range of z. Hence this equation can be integrated
to give
(b)
Next, modify the above result to account for the fact that the cone is rotating with angular
velocity
fl.
The mean centrifugal force per unit volume acting on the fluid in the slit will have
a z-component
approximately
given by
What is the value of
K?
Incorporate this
as
an additional force tending to drive the fluid
through the channel. Show that this leads to the following expression for the mass rate of flow:
Here
Pi
=
pi
+
pgLi
cos
P.
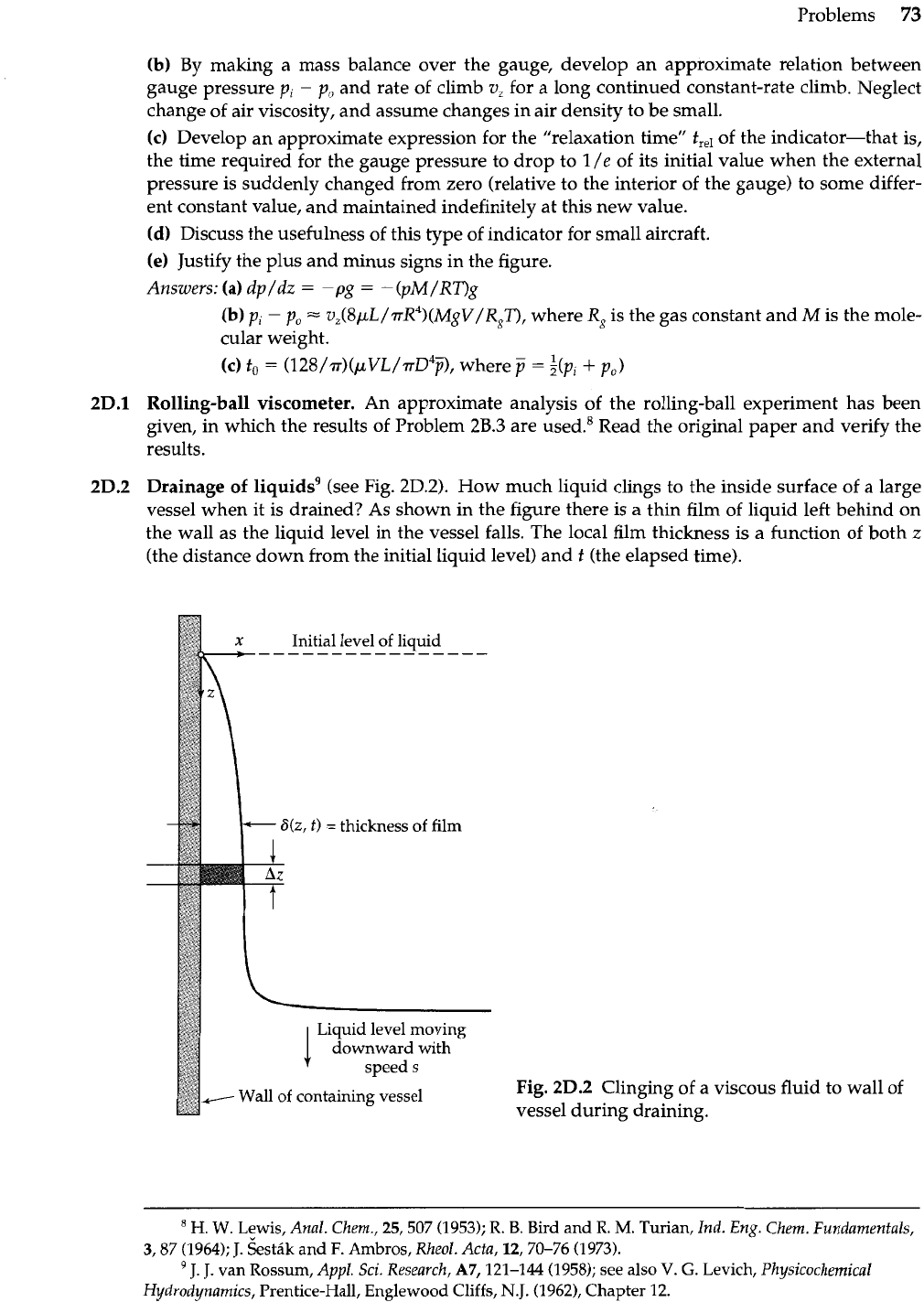
2C.7
A
simple rate-of-climb indicator (see Fig. 2C.7). Under the proper circumstances the simple
apparatus shown in the figure can be used to measure the rate of climb of an airplane. The
gauge pressure inside the Bourdon element is taken as proportional to the rate of climb. For
the purposes of this problem the apparatus may be assumed to have the following properties:
(i) the capillary tube (of radius
R
and length
L,
with
R
<<
L)
is of negligible volume but ap-
preciable flow resistance; (ii) the Bourdon element has a constant volume
V
and offers negli-
gible resistance to flow; and (iii) flow in the capillary
is
laminar and incompressible, and the
volumetric flow rate depends only on the conditions at the ends of the capillary.
Rate
of
climb
Capillary-
tube
Bourdon
element
Pressure
Pressure
outside
=
p,
inside
=
pi
Fig.
2C.7
A
rate-of-climb indicator.
(a)
Develop an expression for the change of air pressure with altitude, neglecting tempera-
ture changes, and considering air to be an ideal gas of constant composition.
(Hint:
Write a
shell balance in which the weight of gas is balanced against the static pressure.)
Problems
73
(b)
By making a mass balance over the gauge, develop an approximate relation between
gauge pressure p,
-
p, and rate of climb v, for a long continued constant-rate climb. Neglect
change of air viscosity, and assume changes in air density to be small.
(c)
Develop an approximate expression for the "relaxation time"
trel
of the indicator-that is,
the time required for the gauge pressure to drop to
l/e
of its initial value when the external
pressure is suddenly changed from zero (relative to the interior of the gauge) to some differ-
ent constant value, and maintained indefinitely at this new value.
(d)
Discuss the usefulness of this type of indicator for small aircraft.
(e)
Justify the plus and minus signs in the figure.
Answers:
(a)
dp/dz
=
pg
=
-(pM/RT)g
(b)
pi
-
p,
=
vZ(8p~/~4)(~g~/~,T), where
R,,
is the gas constant and M is the mole-
cular weight.
(c)
to
=
(128/.ir)(pVL/.rrD4@, where
p
=
$(p,
+
p,)
2D.1
Rolling-ball viscometer.
An approximate analysis of the rolling-ball experiment has been
given, in which the results of Problem 28.3 are used.8 Read the original paper and verify the
results.
2D.2
Drainage of liquids9
(see Fig. 2D.2). How much liquid clings to the inside surface of a large
vessel when it is drained? As shown in the figure there is a thin film of liquid left behind on
the wall as the liquid level in the vessel falls. The local film thickness is a function of both z
(the distance down from the initial liquid level) and
t
(the elapsed time).
Initial level of liquid
----------------
6(z,
t)
=
thickness of film
-
Wall
of
containing vessel
Fig.
2D.2
Clinging of a viscous fluid to wall of
vessel during draining.
H.
W. Lewis,
Anal. Chem.,
25,507 (1953); R.
B.
Bird and
R.
M.
Turian,
Ind. Eng. Chem. Fundamentals,
3,87 (1964);
J.
&stkk and
F.
Arnbros,
Rheol. Acta,
12,70-76 (1973).
J. J.
van Rossum,
Appl. Sci. Research,
A7,121-144 (1958); see also
V.
G.
Levich,
Physicochemical
Hydrodynamics,
Prentice-Hall, Englewood Cliffs,
N.J.
(1962), Chapter 12.

74
Chapter
2
Shell Momentum Balances and Velocity Distributions in Laminar Flow
(a)
Make an unsteady-state mass balance on a portion of the
film
between
z
and
z
+
Az
to get
(b)
Use
Eq.
2.2-18 and a quasi-steady-assumption to obtain the following first-order partial
differential equation for
6(z,
t):
(e)
Solve this equation to get
What restrictions have to be placed on this result?

Chapter
3
The Equations
of
Change
for
Isothermal Systems
3.1
The equation of continuity
93.2
The equation of motion
93.3
The equation of mechanical energy
93.4'
The equation of angular momentum
93.5
The equations of change in terms of the substantial derivative
93.6
Use of the equations of change to solve flow problems
93.7
Dimensional analysis of the equations of change
In Chapter
2,
velocity distributions were determined for several simple flow systems by
the shell momentum balance method. The resulting velocity distributions were then
used to get other quantities, such as the average velocity and drag force. The shell bal-
ance approach was used to acquaint the novice with the notion of a momentum balance.
Even though we made no mention of it in Chapter
2,
at several points we tacitly made
use of the idea of a mass balance.
It is tedious to set up a shell balance for each problem that one encounters. What we
need is a general mass balance and a general momentum balance that can be applied to
any problem, including problems with nonrectilinear motion. That is the main point of
this chapter. The two equations that we derive are called the
equation of continuity
(for the
mass balance) and the
equation of motion
(for the momentum balance). These equations
can be used as the starting point for studying all problems involving the isothermal flow
of a pure fluid.
In Chapter
11
we enlarge our problem-solving capability by developing the equa-
tions needed for nonisothermal pure fluids by adding an equation for the temperature.
In Chapter
19
we go even further and add equations of continuity for the concentra-
tions of the individual species. Thus as we go from Chapter
3
to Chapter
11
and on to
Chapter
19
we are able to analyze systems of increasing complexity, using the com-
plete set of
equations of change.
It should be evident that Chapter
3
is a very important
chapter-perhaps the most important chapter in the book-and it should be mastered
thoroughly.
In
53.1
the equation of continuity is developed by making a mass balance over a
small element of volume through which the fluid is flowing. Then the size of this ele-
ment is allowed to go to zero (thereby treating the fluid as a continuum), and the desired
partial differential equation is generated.

76
Chapter
3
The Equations of Change for Isothermal Systems
In 53.2 the equation of motion is developed by making a momentum balance over a
small element of volume and letting the volume element become infinitesimally small.
Here again a partial differential equation is generated. This equation of motion can be
used, along with some help from the equation of continuity, to set up and solve all the
problems given in Chapter
2
and many more complicated ones. It is thus a key equation
in transport phenomena.
In 53.3 and 53.4 we digress briefly to introduce the equations of change for mechani-
cal energy and angular momentum. These equations are obtained from the equation of
motion and hence contain no new physical information. However, they provide a conve-
nient starting point for several applications in this book-particularly the macroscopic
balances in Chapter
7.
In 53.5 we introduce the "substantial derivative." This is the time derivative follow-
ing the motion of the substance (i.e., the fluid). Because it is widely used in books on
fluid dynamics and transport phenomena, we then show how the various equations of
change can be rewritten in terms of the substantial derivatives.
In 53.6 we discuss the solution of flow problems by use of the equations of continu-
ity and motion. Although these are partial differential equations, we can solve many
problems by postulating the form of the solution and then discarding many terms in
these equations. In this way one ends up with a simpler set of equations to solve. In this
chapter we solve only problems in which the general equations reduce to one or more
ordinary differential equations. In Chapter
4
we examine problems of greater complexity
that require some ability to solve partial differential equations. Then in Chapter
5
the
equations of continuity and motion are used as the starting point for discussing turbu-
lent flow. Later, in Chapter
8,
these same equations are applied to flows of polymeric liq-
uids, which are non-Newtonian fluids.
Finally, 53.7 is devoted to writing the equations of continuity and motion in di-
mensionless form. This makes clear the origin of the Reynolds number, Re, often men-
tioned in Chapter 2, and why it plays a key role in fluid dynamics. This discussion lays
the groundwork for scale-up and model studies. In Chapter
6
dimensionless numbers
arise again in connection with experimental correlations of the drag force in complex
systems.
At the end of 52.2, we emphasized the importance of experiments in fluid dynamics.
We repeat those words of caution here and point out that photographs and other types
of flow visualization have provided us with a much deeper understanding of flow prob-
lems than would be possible by theory alone.' Keep in mind that when one derives a
flow field from the equations of change, it is not necessarily the only physically admissi-
ble solution.
Vector and tensor notations are occasionally used in this chapter, primarily for the
purpose of abbreviating otherwise lengthy expressions. The beginning student will find
that only an elementary knowledge of vector and tensor notation is needed for reading
this chapter and for solving flow problems. The advanced student will find Appendix A
helpful in getting a better understanding of vector and tensor manipulations. With re-
gard to the notation, it should be kept in mind that we use
lightface italic
symbols for
scalars,
boldface
Roman
symbols for vectors, and
boldface
Greek
symbols for tensors.
Also dot-product operations enclosed in
(
)
are scalars, and those enclosed in
I I
are
vectors.
--
'
We recommend particularly
M.
Van
Dyke,
An
Album of Fluid Motion,
Parabolic Press, Stanford
(1982);
H.
Werlk,
Ann. Rev. Fluid Mech.,
5,361-382 (1973);
D.
V.
Boger and
K.
Walters,
Rheological
Phenomena
in
Focus,
Elsevier, Amsterdam
(1993).
