Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

3.1

The Equation of Continuity

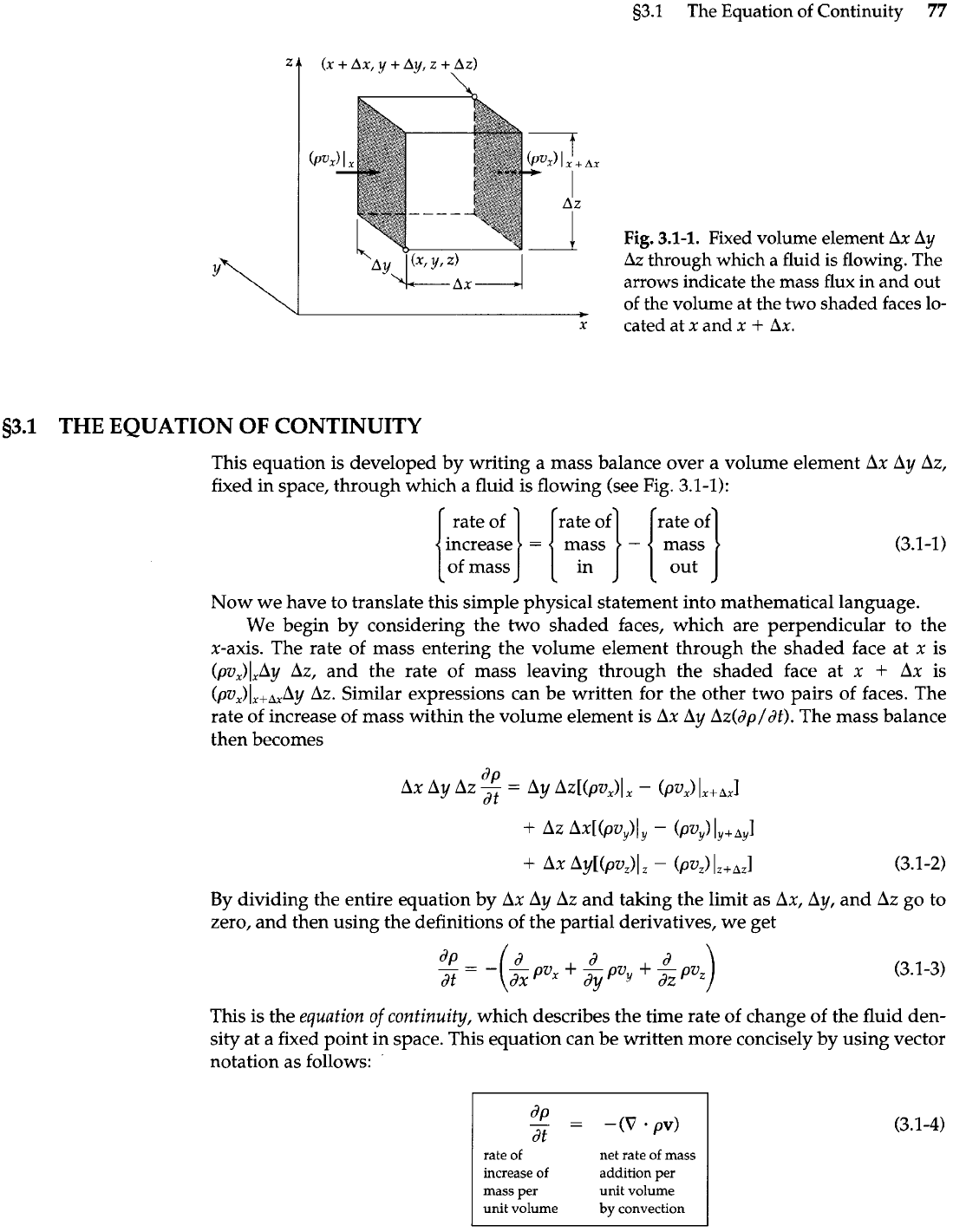
Fig.

3.1-1.

Fixed volume element Ax Ay

Az through which a fluid is flowing. The

arrows indicate the mass flux in and out

of the volume at the two shaded faces lo-

cated at

and

Ax.

3.1

THE EQUATION OF CONTINUITY

This equation is developed by writing a mass balance over a volume element Ax

Az,

fixed in space, through which

fluid is flowing (see Fig. 3.1-1):

rate of rate of rate of

]

[y]

(3.1-1)

Now we have to translate this simple physical statement into mathematical language.

We begin by considering the two shaded faces, which are perpendicular to the

x-axis. The rate of mass entering the volume element through the shaded face at x is

(pvx)lxAy Az, and the rate of mass leaving through the shaded face at x

Ax is

(p~x)lx+AxAy

Az. Similar expressions can be written for the other two pairs of faces. The

rate of increase of mass within the volume element is Ax

Az(dp/dt). The mass balance

then becomes

By dividing the entire equation by Ax

Az and taking the limit as Ax,

Ay,

and Az go to

zero, and then using the definitions of the partial derivatives, we get

This is the equation of continuity, which describes the time rate of change of the fluid den-

sity at a fixed point in space. This equation can be written more concisely by using vector

notation as follows:

rate of net rate of mass

increase of addition per

mass per unit volume

unit volume

convection

Chapter 3 The Equations of Change for Isothermal Systems

Here

pv) is called the "divergence of pv," sometimes written as "div pv." The vector

pv is the mass flux, and its divergence has a simple meaning: it is the net rate of mass ef-

flux per unit volume. The derivation in Problem 3D.1 uses a volume element of arbitrary

shape; it is not necessary to use a rectangular volume element as we have done here.

very important special form of the equation of continuity is that for a fluid of con-

stant density, for which Eq. 3.1-4 assumes the particularly simple form

(incompressible fluid)

(3.1-5)

Of course, no fluid is truly incompressible, but frequently in engineering and biological

applications, the assumption of constant density results in considerable simplification

and very little error.'r2

Show that for any kind of flow pattern, the normal stresses are zero at fluid-solid boundaries,

for Newtonian fluids with constant density. This is an important result that we shall use

Normal Stresses at

often.

Solid Surfaces for

~ncom~r~ssibl~ SOLUTION

Newtonian Fluids

We visualize the flow of a fluid near some solid surface, which may or may not be flat. The

flow may be quite general, with all three velocity components being functions of all three co-

ordinates and time. At some point

on the surface we erect a Cartesian coordinate system

with the origin at

We now ask what the normal stress

r,,

is at

According to Table B.l or Eq. 1.2-6,

T,,

-2p(dv,/dz), because

for incompress-

ible fluids. Then at point

on the surface of the solid

First we replaced the derivative dv,/dz by using Eq. 3.1-3 with

constant. However, on the

solid surface at z

the velocity v, is zero by the no-slip condition (see §2.1), and therefore

the derivative dv,/dx on the surface must be zero. The same is true of dv,/dy on the surface.

Therefore

T,,

is zero. It is also true that

T,,

and ry, are zero at the surface because of the vanish-

ing of the derivatives at z

(Note:

The vanishing of the normal stresses on solid surfaces

does not apply to polymeric fluids, which are viscoelastic. For compressible fluids, the nor-

mal stresses at solid surfaces are zero if the density is not changing with time, as is shown in

Problem 3C.2.)

53.2

THE EQUATION OF MOTION

To get the equation of motion we write a momentum balance over the volume element

in Fig. 3.2-1 of the form

rate of rate of rate of external

momentum momentum

force on

[of

2:E:Eud

[

]

[

out

]

[the

flUij (3'2-1)

Landau and E.

Lifshitz,

Fluid

Mechanics,

Pergamon Press, Oxford (1987), p.

21,

point out

that, for steady, isentropic flows, commonly encountered in aerodynamics, the incompressibility

assumption is valid when the fluid velocity is small compared to the velocity of sound (i.e., low Mach

number).

Equation

3.1-5

is the basis for Chapter

Batchelor,

An Introduction to

Fluid

Dynamics,

Cambridge University Press (1967), which is a lengthy discussion of the kinematical consequences of the

equation of continuity.

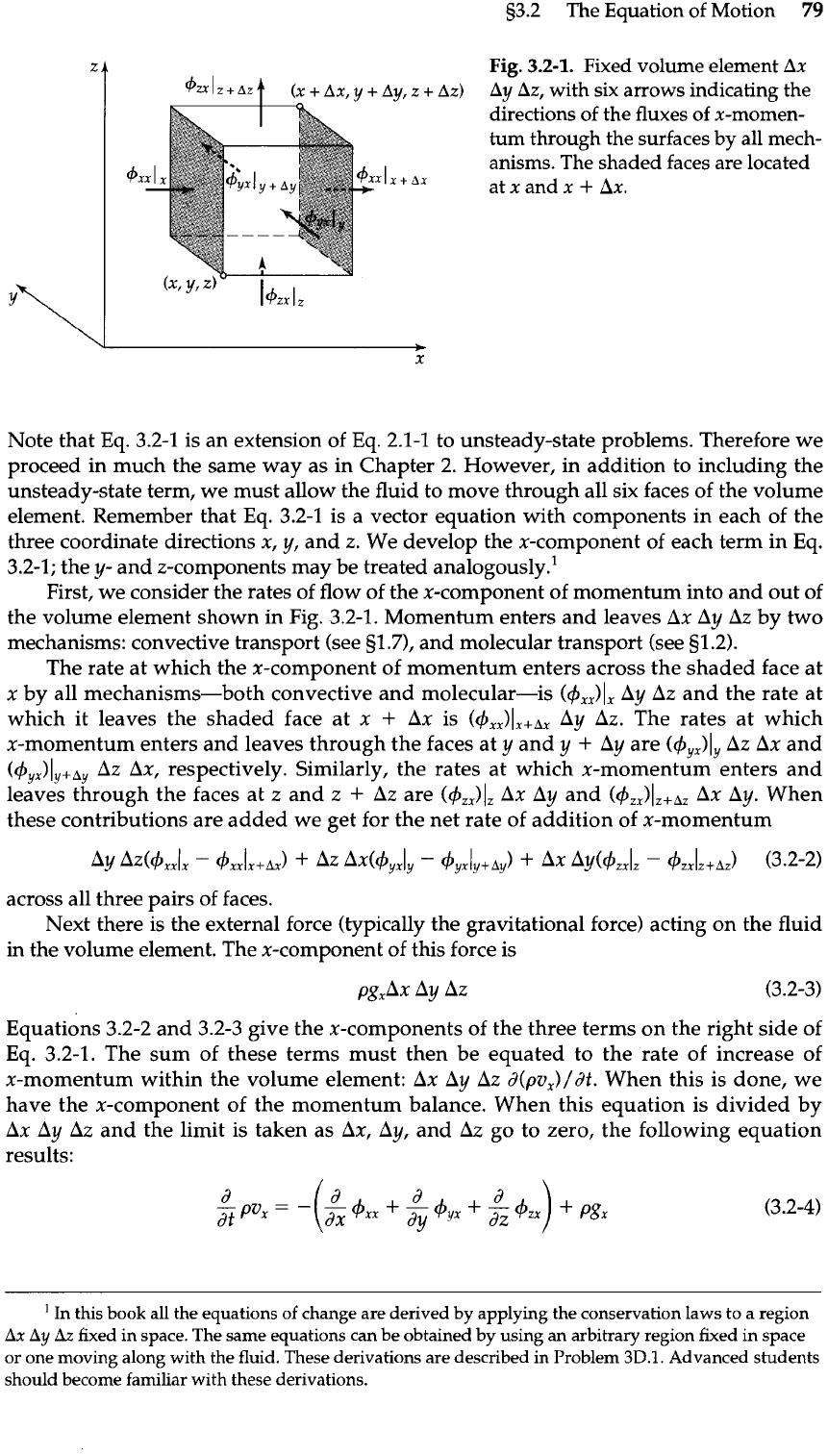
53.2

The Equation of Motion

Fig.

3.2-1.

Fixed volume element

Ax,

Ay,

Az)

Az,

with six arrows indicating the

directions of the fluxes of x-momen-

turn

through the surfaces

all mech-

anisms. The shaded faces are located

X~X+AX

and x

Ax.

Note that Eq. 3.2-1 is an extension of

Eq.

2.1-1 to unsteady-state problems. Therefore we

proceed in much the same way as in Chapter 2. However, in addition to including the

unsteady-state term, we must allow the fluid to move through all six faces of the volume

element. Remember that Eq. 3.2-1 is a vector equation with components in each of the

three coordinate directions x,

and z. We develop the x-component of each term in

Eq.

3.2-1; the

and z-components may be treated analogously.1

First, we consider the rates of flow of the x-component of momentum into and out of

the volume element shown in Fig. 3.2-1. Momentum enters and leaves Ax

Az by two

mechanisms: convective transport (see §1.7), and molecular transport (see 51.2).

The rate at which the x-component of momentum enters across the shaded face at

by all mechanisms-both convective and molecular-is

(4,,)IX

Az and the rate at

which it leaves the shaded face at x

Ax is

(t$xx)lx+Ax

Az.

The rates at which

x-momentum enters and leaves through the faces at

and

are

($,,)I,

Az Ax and

(~yx)Iy+Ay

Az Ax, respectively. Similarly, the rates at which x-momentum enters and

leaves through the faces at z and z

Az are

(+,,)Iz

and

(+Zx)lz+Az

Ay. When

these contributions are added we get for the net rate of addition of x-momentum

across all three pairs of faces.

Next there is the external force (typically the gravitational force) acting on the fluid

in the volume element. The x-component of this force is

Equations 3.2-2 and 3.2-3 give the x-components of the three terms on the right side of

Eq. 3.2-1. The sum of these terms must then be equated to the rate of increase of

x-momentum within the volume element: Ax

Az d(pvx)/dt. When this is done, we

have the x-component of the momentum balance. When this equation is divided by

Az and the limit is taken as Ax, Ay, and Az go to zero, the following equation

results:

In this book all the equations of change are derived by applying the conservation laws to a region

Ax Ay

fixed in space. The same equations can be obtained by using an arbitrary region fixed in space

or one moving along with the fluid. These derivations are described in Problem

3D.1.

Advanced students

should become familiar with these derivations.

Chapter

The Equations of Change for Isothermal Systems

Here we have made use of the definitions of the partial derivatives. Similar equations

can be developed for the

and z-components of the momentum balance:

By using vector-tensor notation, these three equations can be written as follows:

That is, by letting

be successively

and z, Eqs. 3.2-4,5, and

can be reproduced. The

quantities

pvi

are the Cartesian components of the vector

pv,

which is the momentum per

unit volume at a point in the fluid. Similarly, the quantities

pgi

are the components of the

vector

pg,

which is the external force per unit volume. The term

$Ii

is the ith com-

ponent of the vector

-[V

$1.

When the ith component of Eq. 3.2-7 is multiplied by the unit vector in the ith direc-

tion and the three components are added together vectorially, we get

which is the differential statement of the law of conservation of momentum. It is the

translation of Eq. 3.2-1 into mathematical symbols.

In Eq.

1.7-1

it was shown that the combined momentum flux tensor

is the sum of

the convective momentum flux tensor

and the molecular momentum flux tensor

and that the latter can be written as the sum of

and

When we insert

into Eq. 3.2-8, we get the following

equation

rnoti~n:~

zpv

-[V-pvv] -vp -[V.s] +pg

rate of rate of momentum rate of momentum addition external force

increase of addition

by by

molecular transport on fluid

momentum convection per unit volume per unit

per unit per unit volume

volume volume

In this equation

is a vector called the "gradient of (the scalar)

sometimes written as

"grad

p."

The symbol

is a vector called the "divergence of (the tensor)

and

pwl

is a vector called the "divergence of (the dyadic product)

pw."

In the next two sections we give some formal results that are based on the equation

of motion. The equations of change for mechanical energy and angular momentum are

not used for problem solving in this chapter, but will be referred to in Chapter

Begin-

ners are advised to skim these sections on first reading and to refer to them later as the

need arises.

This equation is attributed to

A.-L.

Cauchy,

Ex.

math.,

2,108-111 (1827). (Baron) Augustin-Louis

Cauchy

(1789-1857)

(pronounced "Koh-shee" with the accent on the second syllable), originally trained

as an engineer, made great contributions to theoretical physics and mathematics, including the calculus

of complex variables.

53.3

The Equation of Mechanical Energy

53.3

THE EQUATION OF MECHANICAL ENERGY

Mechanical energy is not conserved in a flow system, but that does not prevent us from

developing an equation of change for this quantity. In fact, during the course of this

book, we will obtain equations of change for a number of nonconserved quantities, such

as internal energy, enthalpy, and entropy. The equation of change for mechanical en-

ergy, which involves only mechanical terms, may be derived from the equation of mo-

tion of g3.2. The resulting equation is referred to in many places in the text that follows.

We take the dot product of the velocity vector v with the equation of motion in Eq.

3.2-9 and then do some rather lengthy rearranging, making use of the equation of conti-

nuity in Eq. 3.1-4. We also split up each of the terms containing

and

into two parts.

The final result is the equation of change for kinetic energy:

($pu2)

;pv%)

pv)

-v

rate of rate of addition

rate of work rate of

reversible

increase of

of kinetic energy

done by pressure conversion of

kinetic energy

by convection

of surroundings kinetic energy into

per unit volume

on the fluid internal energy

v])

(-T:VV)

p(v g) (3.34)'

rate of work done

rate of rate of work

by viscous forces

irreversible by external force

on the fluid conversion on the fluid

from kinetic to

internal energy

At this point it is not clear why we have attributed the indicated physical significance to

the terms

p(V

v) and (7:Vv). Their meaning cannot be properly appreciated until one

has studied the energy balance in Chapter 11. There it will be seen how these same two

terms appear with opposite sign in the equation of change f9r internal energy.

We now introduce the potential energy2 (per unit m%ss)

defined-by g

-V@. Then

the last term in

Eq.

3.3-1 may be rewritten as -p(v

V@)

-(V

pv@)

@(V

-_pv).

The

equation of continuity in

Eq.

3.1-4 m3y now be used to replace

@(V

pv) by -@(dp/dt).

The latter may be written as -d(p@)/dt,

the potential energy is independent of the

time. This is tru: for the gravitational field for systems that are located on the surface of

the earth; then

gh, where g is the (constant) gravitational acceleration and h is the el-

evation coordinate in the gravitational field.

With the introduction of the potential energy,

Eq.

3.3-1 assumes the following form:

This is an equation of change for kinetic-plus-potential energy. Since Eqs. 3.3-1 and 3.3-2 con-

tain only mechanical terms, they are both referred to as the equation of change for mechani-

cal energy.

The term

p(V

v) may be either positive or negative depending on whether the fluid

is undergoing expansion or compression. The resulting temperature changes can be rather

large for gases in compressors, turbines, and shock tubes.

The interpretation under the

(T:VV)

term is correct only for Newtonian fluids; for viscoelastic

fluids, such as polymers, this term may include reversible conversion to elastic energy.

_S,g

is a vector of magnitude

in the negative

direction, then the potential energy per

unit mass is

gz,

where

is the elevation in the gravitational field.

Chapter

The Equations of Change for Isothermal Systems

The term (-T:VV) is always positive for Newtonian fluids: because it may be written

as a sum of squared terms:

which serves to define the two quantities

and

9,.

When the index

takes on the val-

ues

2, 3, the velocity components vi become v,, vy, vz and the Cartesian coordinates xi

become x,

The symbol

is the Kronecker delta, which is

if i

and

if i

The quantity (-T:VV) describes the degradation of mechanical energy into thermal

energy that occurs in all flow systems (sometimes called the viscous dissipation heati~g).~

This heating can produce considerable temperature rises in systems with large viscosity

and large velocity gradients, as in lubrication, rapid extrusion, and high-speed flight.

(Another example of conversion of mechanical energy into heat is the rubbing of two

sticks together to start a fire, which scouts are presumably able to do.)

When we speak of "isothermal systems," we mean systems in which there are no ex-

ternally imposed temperature gradients and no appreciable temperature change result-

ing from expansion, contraction, or viscous dissipation.

The most important use of Eq. 3.3-2 is for the development of the macroscopic me-

chanical energy balance (or engineering Bernoulli equation) in Section

7.8.

53.4

THE EQUATION

ANGULAR MOMENTUM

Another equation can be obtained from the equation of motion by forming the cross

product of the position vector r (which has Cartesian components x,

with Eq. 3.2-9.

The equation of motion as derived in $3.2 does not contain the assumption that the stress

(or momentum-flux) tensor

is symmetric. (Of course, the expressions given in $2.3 for

the Newtonian fluid are symmetric; that is,

rii

T~~.)

When the cross product is formed, we get-after some vector-tensor manipula-

tions-the following equation of change for angular momentum:

Here

is a third-order tensor with components

sijk

(the permutation symbol defined in

sA.2).

the stress tensor

is symmetric, as for Newtonian fluids, the last term is zero.

According to the kinetic theories of dilute gases, monatomic liquids, and polymers, the

tensor

is symmetric, in the absence of electric and magnetic torques.' If, on the other

hand,

is asymmetric, then the last term describes the rate of conversion of bulk angular

momentum to internal angular momentum.

The assumption of

symmetric stress tensor, then, is equivalent to an assertion that

there is no interconversion between bulk angular momentum and internal angular mo-

mentum and that the two forms of angular momentum are conserved separately. This

%n amusing consequence of the viscous dissipation for air is the study

K. Moffatt

[Nature,

404,833434

(2000)l

of the way in which a

spinning

coin comes to rest on a table.

G. G.

Stokes,

Trans. Camb. Phil.

Soc., 9,&106 (1851), see pp. 57-59.

S. Dahler and

Scriven,

Nature,

192,3637 (1961); S. de Groot and

Mazur,

Nonequilibrium

Thermodynamics,

North Holland, Amsterdam (19621, Chapter

XII.

A literature review can be found in

D. C. Kuiken,

Ind. Eng. Chem. Res.,

34,3568-3572 (1995).

53.5

The Equations of Change in Terms of the Substantial Derivative

corresponds, in

Eq.

0.3-8, to equating the cross-product terms and the internal angular

momentum terms separately.

Eq.

3.4-1 will be referred to only in Chapter

where we indicate that the macro-

scopic angular momentum balance can be obtained from it.

53.5

THE EQUATIONS OF CHANGE IN TERMS

THE SUBSTANTIAL DERIVATIVE

Before proceeding we point out that several different time derivatives may be encoun-

tered in transport phenomena. We illustrate these by a homely example--namely, the ob-

servation of the concentration of fish in the Mississippi River. Because fish swim around,

the fish concentration will in general be a function of position (x,

and time (t).

The Partial Time Derivative

dldt

Suppose we stand on a bridge and observe the concentration of fish just below us as a

function of time. We can then record the time rate of change of the fish concentration at a

fixed location. The result is (d~/dt)l,,~,,, the partial derivative of c with respect to t, at con-

stant

and

The Total Time Derivative

dldt

Now suppose that we jump into a motor boat and speed around on the river, sometimes

going upstream, sometimes downstream, and sometimes across the current. All the time

we are observing fish concentration. At any instant, the time rate of change of the ob-

served fish concentration is

in which dx/dt, dy/dt, and dz/dt are the components of the velocity of the boat.

The Substantial Time Derivative

DIDt

Next we climb into a canoe, and not feeling energetic, we just float along with the cur-

rent, observing the fish concentration. In this situation the velocity of the observer is the

same as the velocity

of the stream, which has components v,, vy, and v,. If at any instant

we report the time rate of change of fish concentration, we are then giving

The special operator D/Dt

d/dt

is called the substantial derivative (meaning that

the time rate of change is reported as one moves with the "substance"). The terms mater-

ial derivative, hydrodynamic derivative, and derivative following the motion are also used.

Now we need to know how to convert equations expressed in terms of d/dt into

equations written with D/Dt. For any scalar function

(x,

t) we can do the following

manipulations:

Chapter

The Equations of Change for Isothermal Systems

Table

3.5-1

The Equations of Change for Isothermal Systems in the D/Dt-Forma

Note: At the left are given the equation numbers for the d/dt forms.

(3.4-1)

-[V

p~J']

T)']

pg]

(DY

Equations

(A)

through

(C)

are obtained from Eqs. 3.14,3.2-9, and

3.3-1

with

assumptions.

Equation

(D)

is written for symmetrical

only.

The quantity in the second parentheses in the second line is zero according to the equa-

tion of continuity. Consequently Eq. 3.5-3 can be written in vector form as

Similarly, for any vector function f(x,

t),

(pf)

pvf]

dt Dt

These equations can be used to rewrite the equations of change given in 553.1 to 3.4 in

terms of the substantial derivative as shown in Table 3.5-1.

Equation

in Table 3.5-1 tells how the density is decreasing or increasing as one

moves along with the fluid, because of the compression [(V

01 or expansion of the

fluid

[(V

01. Equation

can be interpreted as (mass)

(acceleration)

the sum of

the pressure forces, viscous forces, and the external force. In other words, Eq. 3.2-9 is

equivalent to Newton's second law of motion applied to a small blob of fluid whose en-

velope moves locally with the fluid velocity

(see Problem 3D.1).

We now discuss briefly the three most common simplifications of the equation of

motion.'

(i)

For constant

and

insertion of the Newtonian expression for

from Eq. 1.2-7

into the equation of motion leads to the very famous Navier-Stokes equation, first de-

veloped from molecular arguments by Navier and from continuum arguments by

Stokes:'

In the second form we have used the "modified pressure"

pgh

introduced in

Chapter 2, where

is the elevation in the gravitational field and

is the gravitational

For discussions of the history of these and other famous fluid dynamics relations, see

Rouse

and

Ince,

History of Hydraulics,

Iowa Institute of Hydraulics, Iowa City (1959).

H. Navier,

Mkmoires de I'Acadkmie Royale des Sciences,

6,389-440

(1827);

Stokes,

Proc.

Cambridge Phil. Soc,

8,287-319 (1845). The name Navier is pronounced "Nah-vyay."

$3.5

The Equations of Change in Terms of the Substantial Derivative

Fig.

3.5-1.

The equation of state for a slightly com-

slight?;;mp;sible

pressible fluid and an incompressible fluid when

~-po=K(p-Po)

is constant.

where

constant

- - -

potential energy per unit mass. Equation 3.5-6 is a standard starting point for describing

isothermal flows of gases and liquids.

It must be kept in mind that, when constant

is assumed, the equation of state (at

constant

vertical line on a plot of

vs.

(see Fig. 3.5-1). Thus, the absolute pres-

sure is no longer determinable from p and

although pressure gradients and instanta-

neous differences remain determinate by Eq. 3.5-6 or Eq. 3.5-7. Absolute pressures are

also obtainable if

is known at some point in the system.

(ii) When the acceleration terms in the Navier-Stokes equation are neglected-that is,

when p(Dv/Dt)

0-we get

which is called the Stokes flow equation. It is sometimes called the creeping flow equation, be-

cause the term p[v

Vvl, which is quadratic in the velocity, can be discarded when the

flow is extremely slow. For some flows, such as the Hagen-Poiseuille tube flow, the term

p[v

Vvl drops out, and a restriction to slow flow is not implied. The Stokes flow equation

is important in lubrication theory, the study of particle motions in suspension, flow

through porous media, and swimming of microbes. There is a vast literature on this

~ubject.~

(iii) When viscous forces are neglected-that is,

0-the equation of motion

becomes

which is known as the Euler equation for "inviscid"

fluid^.^

Of course, there are no truly

"inviscid" fluids, but there are many flows in which the viscous forces are relatively

unimportant. Examples are the flow around airplane wings (except near the solid

boundary), flow of rivers around the upstream surfaces of bridge abutments, some prob-

lems in compressible gas dynamics, and flow of ocean

current^.^

Happel and H. Brenner,

Low Reynolds Number Hydrodynaniics,

Martinus Nijhoff, The Hague

(1983);

Kim and S.

Karrila,

Microkydrodynamics: Principles and Selected Applications,

Butterworth-

Heinemann, Boston (1991).

Euler,

Mim. Acad. Sci. Berlin,

11,217-273,274-315,316-361

(1755). The Swiss-born

mathematician

Leonhard

Euler

(1707-1783) (pronounced "Oiler") taught in

St.

Petersburg, Basel, and

Berlin and published extensively in many fields of mathematics and physics.

See, for example,

Acheson,

Elementary Fluid Mechanics,

Clarendon Press, Oxford (1990),

Chapters

3-5;

and

Batchelor,

An Introduction to Fluid Dynamics,

Cambridge University Press (19671,

Chapter 6.

Chapter 3 The Equations of Change for Isothermal Systems

The Bernoulli equation for steady flow of inviscid fluids is one of the most famous equations

in classical fluid dynami~s.~ Show how it is obtained from the Euler equation of motion.

The Bernoulli Equation

for

the Steady Flow of

SOLUTION

Inviscid

Fluids

Omit the time-derivative term in Eq. 3.5-9, and then use the vector identity

Vvl

iV(v V)

v]]

(Eq.

A.4-23)

to rewrite the equation as

pV;v2

p[v

v]]

-Vp

pgVh

(3.5-10)

In writing the last term, we have expressed

-V@

-gVh,

where

is the elevation in the

gravitational field.

Next we divide Eq. 3.5-10 by

and then form the dot product with the unit vector

v//vl

in the flow direction. When this is done the term involving the curl of the velocity

field can be shown to vanish (a nice exercise in vector analysis), and

can be replaced by

d/ds, where s is the distance along

streamline. Thus we get

When this is integrated along a streamline from point 1 to point

we get

which is called the

Bernoulli equafion.

It relates the velocity, pressure, and elevation of two

points along a streamline in a fluid in steady-state flow. It is used in situations where it

can

assumed that viscosity plays a rather minor role.

53.6

USE OF

THE

EQUATIONS OF CHANGE

TO SOLVE FLOW PROBLEMS

For most applications of the equation of motion, we have to insert the expression for

from Eq. 1.2-7 into Eq. 3.2-9 (or, equivalently, the components of

from Eq. 1.2-6 or Ap-

pendix B.l into Eqs. 3.2-5, 3.2-6, and 3.2-7). Then to describe the flow of a Newtonian

fluid at constant temperature, we need in general

The equation of continuity Eq. 3.1-4

The equation of motion

Eq. 3.2-9

The components of

Eq. 1.2-6

The equation of state

P(P)

The equations for the viscosities

~(p)

These equations, along with the necessary boundary and initial conditions, determine

completely the pressure, density, and velocity distributions in the fluid. They are seldom

used in their complete form to solve fluid dynamics problems. Usually restricted forms

are used for convenience,

in this chapter.

If it is appropriate to assume constant density and viscosity, then we use

The equation of continuity Eq. 3.1-4 and Table

B.4

The Navier-Stokes equation Eq. 3.5-6 and Tables B.5,6,7

along with initial and boundary conditions. From these one determines the pressure and

velocity distributions.

Daniel Bernoulli

(1700-1782)

was one of the early researchers in fluid dynamics and also the

kinetic theory of gases. His hydrodynamical ideas were summarized

Bernoulli,

Hydrodynarnica sive

de uiribus et motibus fluidovum commentarii,

Argentorati

(1738),

however he did not actually give

Eq.

3.5-12.

The credit for the derivation of

Eq.

3.5-12

goes to

Euler,

Histoires de l'Acad6mie de Berlin

(1755).