Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

Problems

577

Surface

Fig.

18B.14.

Side view of a disk-shaped

catalyst particle.

Surface

18B.14.

Effectiveness factors for thin disks (Fig. 18B.14). Consider porous catalyst particles in the

shape of thin disks, such that the surface area of the edge of the disk is small in comparison

with that of the two circular faces. Apply the method of 518.7 to show that the steady-state

concentration profile is

where

and

are described in the figure. Show that the total mass transfer rate at the surfaces

+bis

WAI

21rR*c,,91~h tanh hb

(18B.14-2)

in which h

Show that, if the disk

sliced parallel to the xy-plane into

slices, the

total mass transfer rate becomes

Obtain the expression for the effectiveness factor by taking the limit

Express this result in terms of the parameter

defined in 518.6.

18B.15.

Diffusion and heterogeneous reaction in a slender cylindrical tube with a closed end (Fig.

18B.15).

slender cylindrical pore of length

cross-sectional area

and perimeter

is in

contact at its open end with

large body of well-mixed fluid, consisting of species A and

Species A, a minor constituent of this fluid, disappears into the pore, diffuses in the

direc-

tion and reacts on its walls. The rate of this reaction may be expressed as (n

n,)l,,,,,,

f(oA,);

that is, at the wall the mass flux normal to the surface is some function of the mass fraction,

WA~,

of A in the fluid adjacent to the solid surface. The mass fraction

depends on

the dis-

tance from the inlet. Because

is present in low concentration, the fluid temperature and density

may be considered constant, and the diffusion flux is adequately described by

(a)

Side View

End

View

Fig.

18B.15.

(a)

Diffu-

sion and heterogeneous

reaction in a long, non-

circular cylinder.

(b)

Re-

gion of thickness

over which the mass

balance is made.

578

Chapter 18

Concentration Distributions in Solids and in Laminar Flow

where the diffusivity may be regarded as a constant. Because the pore is long compared to its

lateral dimension, concentration gradients in the lateral directions may be neglected. Note the

similarity with the problem discussed in 510.7.

(a) Show by means of a shell balance that, at steady state,

(b)

Show that the steady-state mass average velocity

is zero for this system.

(c)

Substitute the appropriate form of Fick's law into

Eq.

18.15-1, and integrate the resulting

differential equation for the special case that

(w,,)

k','wAo.

To obtain a boundary condition

neglect the rate of reaction on the closed end of the cylinder; why is this a reasonable

approximation?

(dl Develop an expression for the total rate

of disappearance of

in the cylinder.

(el Compare the results of parts (c) and (d) with those of s10.7 both from the standpoint

the mathematical development and the nature of the assumptions made.

188.16.

Effect of temperature and pressure on evaporation rate.

(a)

518.2 what is the effect of a change of temperature and pressure on the quantity x,,?

(b) If the pressure is doubled, how is the evaporation rate in

Eq.

18.2-14 affected?

(c)

How does the evaporation rate change when the system temperature is raised from

T'?

18B.17.

Reaction rates in large

and

small particles.

(a) Obtain the following limits for

Eq.

18.7-11:

Interpret these results physically.

(b) Obtain the corresponding asymptotes for the system discussed in Problem 18B.14. Com-

pare them with the results in (a).

18B.18.

Evaporation rate for small mole fraction of the volatile liquid. In

Eq.

18.2-15, expand

in a Taylor series appropriate for small mole fractions of

First rewrite the logarithm of the

quotient as the difference of the logarithms. Then expand ln(1

x,,) and ln(1

xA2)

Taylor

series about

XAl

and

XA2

respectively. Verify that

Eq.

18.2-16 is correct.

18B.19.

Oxygen uptake by a bacterial aggregate. Under suitable circumstances the rate of oxygen

metabolism by bacterial cells is very nearly zero order with respect to oxygen concentration.

We examine such a case here and focus our attention on a spherical aggregate of cells, which

has

radius

We wish to determine the total rate of oxygen uptake by the aggregate as

function of aggregate size, oxygen mass concentration po at the aggregate surface, the meta-

bolic activity of the cells, and the diffusional behavior of the oxygen. For simplicity we con-

sider the aggregate to be homogeneous. We then approximate the metabolic rate by an

effective volumetric reaction rate

rO2

-k!

and the diffusional behavior by Fick's law, with

an effective pseudobinary diffusivity

BO2,.

Because the solubility of oxygen is very low in this

system, both convective oxygen transport and transient effects may be neglected.12

Mueller,

Boyle,

and

Lightfoot,

Biotechnol.

and

Bioengr.,

10,331-358 (1968).

Problems

579

(a)

Show by means of a shell mass balance that the quasi-steady-state oxygen concentration

profile is described by the differential equation

where

po2/po,

v/R, and

(b)

There may be an oxygen-free core in the aggregate, if

is sufficiently large, such that

for

to.

Write sufficient boundary conditions to integrate

Eq.

18B.19-1 for this situation. To

do this, it must be recognized that both

and

dx/d&

are zero at

6,.

What is the physical

significance of this last statement?

(c)

Perform the integration of

Eq.

18B.19-1 and show how

may be determined.

(d)

Sketch the total oxygen uptake rate and

as functions of

and discuss the possibility

that no oxygen-free core exists.

Answer:

(c)

for

where

is determined as a func-

tion of

from

18C.1.

Diffusion from a point source in a moving stream

(Fig. 18C.1).

stream of fluid

in lami-

nar motion has a uniform velocity v,.

some point in the stream (taken to be the origin of

coordinates) species

is injected at a small rate

g-moles/s. This rate is assumed to be suf-

ficiently small that the mass average velocity will not deviate appreciably from

v,.

Species

is swept downstream (in the

direction), and at the same time it diffuses both axially and

radially.

(a)

Show that

steady-state mass balance on species

over the indicated ring-shaped ele-

ment leads to the following partial differential equation if

'?JAB

is assumed to be constant:

(b)

Show that

Eq.

18C.1-1 can also be written as

in which s2

z2.

Uniform

stream

velocity

Origin of coordinates placed at

point of iniection;

moles

are iijected per second

LAZ

Fig.

18C.1.

Diffusion of

from a point source into

stream of

that moves with

a uniform velocity.

580

Chapter 18

Concentration Distributions in Solids and in Laminar Flow

(c)

Verify (lengthy!) that the solution

satisfies the differential equation above.

(d) Show further that the following boundary conditions are also satisfied by Eq. 18C.1-3:

B.C.

(18C.1-4)

B.C.

~CA

(18C.1-6)

Explain the physical meaning of each of these boundary conditions.

(e)

Show how data on cA(r,

for given vo and %,, may be plotted, when the preceding solu-

tion applies, to give a straight line with slope v,/29JA, and intercept In

9,,.

18C.2.

Diffusion and reaction in a partially impregnated catalyst. Consider a catalytic sphere like

that in g18.7, except that the active ingredient of the catalyst is present only in the annular re-

gion between

and r

In region I (0

KR),

In region I1

(KR

R),

k;'a

constant

Such a situation may arise when the active ingredient is put on the particles after pelleting, as

is done for many commercial catalysts.

(a) Integrate Eq. 18.7-6 separately for the active and inactive regions. Then apply the appro-

priate boundary conditions to evaluate the integration constants, and solve for the concentra-

tion profile in each region. Give qualitative sketches to illustrate the forms of the profiles.

(b) Evaluate WAR, the total molar rate of conversion of

in a single particle.

18C.3.

Absorption rate

a falling

film.

The result in Eq. 18.5-18 may be obtained by an alternative

procedure.

(a) According to an overall mass balance on the film, the total moles of

transferred per unit

time across the gas-liquid interface must be the same as the total molar rate of flow of

across the plane

The latter rate is calculated as follows:

Explain this procedure carefully.

(b)

Insert the solution for cA in Eq. 18.5-15 into the result of (a) to obtain:

In the second line, the new variable

X/~~%,,L/V~,~ has been introduced.

(c)

Change the order of integration in the double integral, to get

Explain by means of a carefully drawn sketch how the limits are chosen for the integrals The

integrals may now be done analytically to get Eq. 18.5-18.

Problems

581

18C.4.

Estimation of the required length of an isothermal reactor (Fig. 18.3-1). Let

be the area of

catalyst surface per unit volume of a packed-bed catalytic reactor and

be the cross-sectional

area of the reactor. Suppose that the rate of mass flow through the reactor is w (in lb,/hr, for

example).

(a) Show that a steady-state mass balance on substance

over a length

of the reactor leads to

(b)

Use the result of (a) and Eq. 18.3-9, with the assumptions of constant

and

%,,,

to obtain

an expression for the reactor length

needed to convert an inlet stream of composition xA(0)

to an outlet stream of composition x,(L).

(Hint: Equation (P) of Table 17.8-1 may be useful.)

18C.5.

Steady-state evaporation. In a study of the evaporation of a mixture of methanol

(1)

and ace-

tone

(2)

through air (31, the concentration profiles of the three species in the tube were mea-

suredl%fter attainment of steady state. In this situation, species

is not moving, and species

and

are diffusing upward, with the molar fluxes N,, and Nz2, measured in the experi-

ments. The interfacial concentrations of these two species, x,, and

x~~~

were also measured. In

addition, the three binary diffusion coefficients were known. The interface was located at

and the upper end of the diffusion tube was at z

(a) Show that the Maxwell-Stefan equation for species 3 can be solved to get

in which A

Vl13

"223,

with

vmpy

N,L/c~~, and

z/L.

(b)

Next verify that the equation for species

can be solved to get

"212

Cx30

x2,eB[

---

eB5)

(eA5

eB5)

A-B

where

vlI2

y,,

and

yl,

"223.

(c)

Compare the above equations with the published results.

(d)

How well do Eqs. 18C.5-1 and

fit the experimental data?

18D.1.

Effectiveness factors for long cylinders. Derive the expression for

77~

for long cylinders anal-

ogous to Eq. 18.7-16. Neglect the diffusion through the ends of the cylinders.

Zl(2N

Answer:

---

No(2N'

where

I,,

and

are "modified Bessel functions"

18D.2.

Gas absorption

a falling

film

with chemical reaction. Rework the problem discussed in

518.5 and described in Fig. 18.5-1, when gas A reacts with liquid

by a first-order irreversible

chemical reaction in the liquid phase, with rate constant

k;'.

Specifically, find the expression

for the total absorption rate analogous to that given in Eq. 18.5-18. Show that the result for ab-

sorption with reaction properly simplifies to that for absorption without reaction.

Answer:

W~~ZJ,,~

[(;

era

in which u

k;'~/o,,,,.

Wilson,

Proc.

Camb.

Phil.

Soc.,

12,406423 (1904).

Carty and

Schrodt,

Ind.

Eng.

Chem.,

14,276-278 (1975).

Chapter

Equations

Change

for

Multicomponent Systems

519.1 The equations of continuity for a multicomponent mixture

519.2 Summary of the multicomponent equations of change

s19.3

Summary of the multicomponent fluxes

519.4 Use of the equations of change for mixtures

919.5

Dimensional analysis of the equations of change for binary mixtures

In Chapter 18, problems in diffusion were formulated by making shell mass balances

on one or more of the diffusing species. In this chapter we start by making a mass bal-

ance over an arbitrary differential fluid element to establish the equation of continuity

for the various species in a multicomponent mixture. Then insertion of mass flux ex-

pressions gives the diffusion equations in a variety of forms. These diffusion equations

can be used to set up any of the problems in Chapter 18 and more complicated ones as

well.

Then we summarize all of the equations of change for mixtures: the equations of

continuity, the equation of motion, and the equation of energy. These include the equa-

tions of change that were given in Chapters 3 and 11. Next we summarize the flux ex-

pressions for mixtures. All these equations are given in general form, although for

problem solving we generally use simplified versions of them.

The remainder of the chapter is devoted to analytical solutions and dimensional

analyses of mass transfer systems.

519.1

THE

EQUATIONS OF CONTINUITY

FOR

MULTICOMPONENT MIXTURE

In this section we apply the law of conservation of mass to each species

in a mixture,

where

1,2,3,

. .

The system we consider is a volume element

fixed in

space, through which the fluid mixture is flowing (see Fig. 3.1-1). Within this mixture, re-

actions among the various chemical species may be occurring, and we use the symbol

to indicate the rate at which species

is being produced, with dimensions of mass/vol-

ume time.

The various contributions to the mass balance are

rate of increase of mass of

(dp,/Jt)~x

in the volume element

rate of addition of mass of

nffrlx

across face at

519.1 The Equations of Continuity for a Multicomponent Mixture

583

rate of removal of mass of

n,,l,+~,

across face at x

rate of production of mass of r,Ax Ay Az

by chemical reactions

The combined mass flux

n,,

includes both the molecular flux and the convective flux.

There are also addition and removal terms in the y and

directions. When the entire

mass balance is written down and divided by Ax Ay Az, one obtains, after letting the size

of the volume element decrease to zero,

This is the equation of continuity for species

in a multicomponent reacting mixture. It de-

scribes the change in mass concentration of species

with time at a fixed point in space

by the diffusion and convection of

as well as by chemical reactions that produce or

consume a. The quantities n,,, n,,, n,, are the Cartesian components of the mass flux vec-

tor

p,v, given in Eq.

(D)

of Table 17.8-1.

Equation 19.1-5 may be rewritten in vector notation as

Alternatively we can use Eq.

(S)

of Table 17.8-1 to write

rate of net rate of net rate of

rate of

increase addition addition

production

of mass of mass of of mass of

of mass of

per

unit

per

unit

per unit

unit volume

volume

volume convection

diffusion reaction

Addition of all

equations in either Eq. 19.1-6 or 7 gives

which is the equation of continuity for the mixture. This equation is identical to the equation

of continuity for a pure fluid given in

Eq.

3.1-4.

obtaining Eq. 19.1-8 we had to use Eq.

(J)

of Table 17.8-1 and also the fact that the law of conservation of total mass gives Car,

Finally we note that Eq. 19.1-8 becomes

for a fluid mixture of constant mass density p.

the preceding discussion we used mass units. However, a corresponding deriva-

tion

also possible in molar units. The equation of continuity for species

in molar

quantities is

Crank,

The Mathematics of Diffusion,

2nd edition, Oxford University Press

(1975).

584

Chapter

Equations of Change for Multicomponent Systems

where

is the molar rate of production of

per unit volume. This equation can be

rewritten by use of Eq.

(V)

of Table 17.8-1 to give

rate of net rate of rate of

rate of

increase addition

addition production

in moles

moles of of moles of of moles of

per

per unit

unit volume

volume

volume convection diffusion

reaction

When all

equations in Eq. 19.1-10 or

are added we get

for the equation of continuity for the mixture. To get this we used Eq.

(M)

of Table 17.8-1.

We also note that the chemical reaction term does not drop out because the number of

moles is not necessarily conserved in a chemical reaction. Finally we note that

for a fluid mixture of constant

molar density

We have thus seen that the equation of continuity for species

may be written in

two forms,

Eq.

19.1-7 and Eq. 19.1-11. Using the continuity relations in Eqs. 19.1-8 and

19.1-12 the reader may verify that the equation of continuity for species

can be put into

two additional, equivalent forms:

These two equations express exactly the same physical content, but they are written in

two different sets of notation-the first in mass quantities and the second in molar quan-

tities. To use these equations we have to insert the appropriate expressions for the fluxes

and the chemical reaction terms. In this chapter we give only the results for

binary sys-

tems

with constant

p%,,,

with constant

or with zero velocity.

Binary Systems with Constant

p9lAB

For this assumption, Eq. 19.1-14 becomes, after inserting Fick's law from Eq.

(A)

of Table

17.8-2,

with a corresponding equation for species

This equation is appropriate for describing

the diffusion in

dilute liquid solutions

at constant temperature and pressure. The left side

can be written as

pDoA/Dt.

Equation 9.1-16 without the

term is of the same form

Eq. 11.2-8 or

This similarity is quite important, since it is the basis for the analogies

that are frequently drawn between heat and mass transport in flowing fluids with con-

stant physical properties.

s19.1 The Equations of Continuity for a Multicomponent Mixture

585

Binary Systems with Constant

&,,

For this assumption, Eq. 19.1-15 becomes, after inserting Fick's law from Eq.

(B)

of Table

17.8-2,

with a corresponding equation for species

This equation is useful for low-density gases

at constant temperature and pressure. The left side can not be written as cDx,/Dt be-

cause of the appearance of

rather than

Binary Systems with Zero Velocity

If there are no chemical reactions occurring, then the chemical production terms are all

zero. If, in addition

is zero and

constant in Eq. 19.1-16, or

is zero and c constant in

Eq. 19.1-17, then we get

which is called Fick's second law of diffusion, or sometimes simply the diffusion equation.

This equation is usually used for diffusion in solids or stationary liquids (that is,

0 in

Eq. 19.1-16) and for equimolar counter-diffusion in gases (that is,

0 in Eq. 19.1-17). By

equimolar counter-diffusion we mean that the net molar flux with respect to stationary

coordinates is zero; in other words, that for every mole of

that moves, say, in the posi-

tive

direction, there is a mole of

that moves in the negative

direction.

Note that Eq. 19.1-18 has the same form as the heat conduction equation in Eq. 11.2-10.

This similarity is the basis for analogies between many heat conduction and diffusion

problems in solids. Keep in mind that many hundreds of problems described by Fick's

second law have been solved. Solutions are tabulated in the monographs of Crank1 and

of Carslaw and Jaeger.'

In Tables B-10 and 11 we give Eq. 19.1-14 (multicomponent equation of continuity in

terms of

j,)

and Eq. 19.1-16 (binary diffusion equation for constant

and '?JAB) in the

three standard coordinate systems. Other forms of the equation of continuity can be pat-

terned after these.

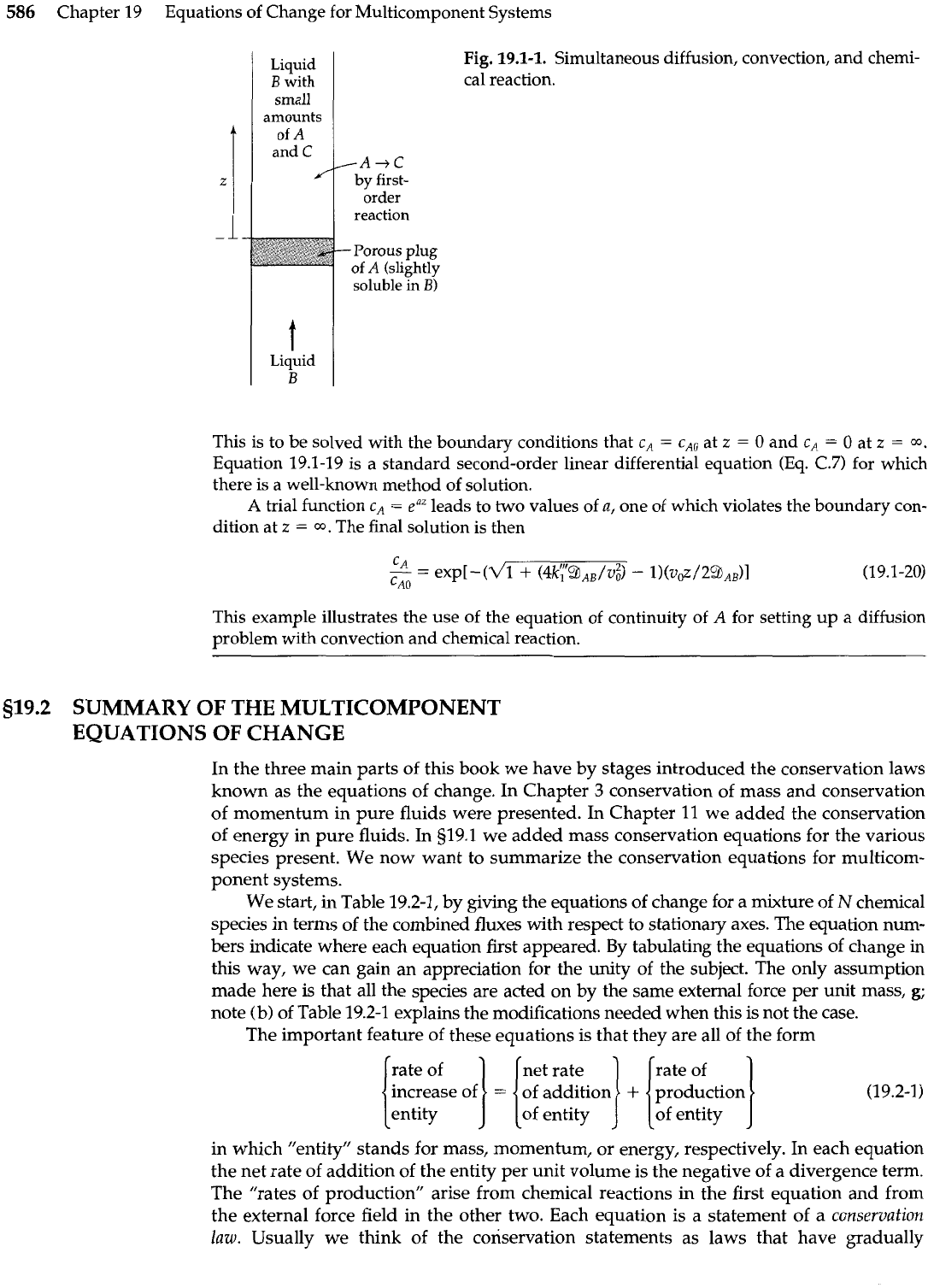
In Fig. 19.1-1 we show a system in which a liquid,

moves slowly upward through a slightly

soluble porous plug of

Then

slowly disappears by a first-order reaction after it has dis-

solved. Find the steady-state concentration profile c,(~), where

is the coordinate upward

and

Chemical Reaction3

from the plug. Assume that the velocity profile is approximately flat across the tube. Assume

further that

CAO

is the solubility of unreacted

in B. Neglect temperature effects associated

with the heat of reaction.

SOLUTION

Equation 19.1-16 is appropriate for dilute liquid solutions. Dividing this equation by the mol-

ecular weight

and specializing for the one-dimensional steady-state problem at hand, we

get for constant

Carslaw and

C. Jaeger,

Conducfion ofHeaf in Solids,

2nd edition, Oxford University Press (1959).

Jost,

Diffusion,

Academic Press, New York (1952),

pp.

58-59.

586

Chapter 19 Equations of Change for Multicomponent Systems

Liquid

Fig.

19.1-1.

Simultaneous diffusion, convection, and chemi-

with

cal reaction.

small

amounts

and

A+C

order

reaction

Porous plug

(slightly

soluble

Liquid

This is to be solved with the boundary conditions that

c,,

and

Equation 19.1-19 is a standard second-order linear differential equation (Eq. C.7) for which

there is a well-known method of solution.

trial function

eaz

leads to two values of

one of which violates the boundary con-

dition at

The final solution is then

This example illustrates the use of the equation of continuity of

for setting up a diffusion

problem with convection and chemical reaction.

519.2

SUMMARY OF

THE

MULTICOMPONENT

EQUATIONS

CHANGE

In the three main parts of this book we have by stages introduced the conservation laws

known as the equations of change. In Chapter

conservation of mass and conservation

of momentum in pure fluids were presented. In Chapter 11 we added the conservation

of energy in pure fluids. In 519.1 we added mass conservation equations for the various

species present. We now want to summarize the conservation equations for multicom-

ponent systems.

We start,

Table 19.2-1, by giving the equations of change for a mixture of

chemical

species in terms of the combined fluxes with respect to stationary axes. The equation num-

bers indicate where each equation first appeared. By tabulating the equations of change in

this way, we can gain an appreciation for the unity of the subject. The only assumption

made here

that all the species are acted on by the same external force per unit mass,

note (b) of Table 19.2-1 explains the modifications needed when this is not the case.

The important feature of these equations is that they are all of the form

rate of

net rate

rate of

increase of

of addition

production (19.2-1)

{entity

]

[ofentity

]

jOf

entity

]

in which "entity" stands for mass, momentum, or energy, respectively. In each equation

the net rate of addition of the entity per unit volume is the negative of a divergence term.

The "rates of production" arise from chemical reactions in the first equation and from

the external force field in the other two. Each equation is a statement of a

conservation

law.

Usually we think of the conservation statements as laws that have gradually