Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

Problems

607

Fig.

198.4.

Oxidation of silicon.

19B.4.

Oxidation of silicon (Fig. 19B.4).'

slab of silicon is exposed to gaseous oxygen (species

at pressure p, producing a layer of silicon dioxide (species

B).

The layer extends from the sur-

face

0, where the oxygen dissolves with concentration

CAO

Kp, to the surface at

SW,

where the oxygen and silicon undergo a first-order reaction with rate coefficient

k;(.

The thick-

ness 6(t) of the growing oxide layer is to be predicted. A quasi-steady-state method is useful

here, inasmuch as the advancement of the reaction front is very slow.

(a)

First solve the diffusion equation of Eq. 19.1-18, with the term dc,/dt neglected, and apply

the boundary conditions to obtain

in which the concentration

CAS

at the reaction plane is as yet unknown.

(b)

Next use an unsteady-state molar

balance on the region 0

6(t) to obtain, with the

aid of the Leibniz formula of gC.3,

(c)

Now write an unsteady-state molar balance on SiO, in the same region to obtain

(d)

In Eq. 19B.4-2, evaluate dS/dt from Eq. 19B.4-3 and dc,/dz from

Eq.

19B.4-I. This will

yield an equation for

CA,:

Inserting numerical values into Eq. 19B.4-4 shows that the quadratic term can safely be

neglected.

(e)

Combine Eqs. 19B.4-3 and 19B.4-4 (without the quadratic term) to get a differential equa-

tion for S(t). Show that this leads to

which agrees with experimental data.' Interpret the result.

19B.5.

The Maxwell-Stefan equations

for

multicomponent gas mixtures. In Eq. 17.9-1 the

Maxwell-Stefan equations for the mass fluxes in a multicomponent gas system are given. Show

that these equations simplify for a binary system to Fick's first law, as given in Eq. 17.1-5.

19B.6.

Diffusion and chemical reaction in a liquid.

(a) A solid sphere of substance

is suspended in

liquid

in which it is slightly soluble,

and with which

undergoes a first-order chemical reaction with rate constant

ky.

At steady

Ghez,

Primer

Diffusion

Problems,

Wiley-Interscience,

New

York

(1988),

pp.

46-55;

this book

discusses

number of problems that arise in the microelectronics field.

608

Chapter 19 Equations of Change for Multicomponent Systems

state the diffusion is exactly balanced by the chemical reaction. Show that the concentration

profile is

in which

is the radius of the sphere,

cAo

is the molar solubility of

and

kyR2/9,,.

(b) Show by quasi-steady-state arguments how to calculate the gradual decrease in diameter

of the sphere as

dissolves and reacts. Show that the radius of the sphere is given by

in which

is the sphere radius at time to, and

p,,,

is the density of the sphere.

19B.7.

Various forms of the species continuity equation.

(a) In this chapter the species equation of continuity is given in three different forms:

Eq.

19.1-7, Eq.

(A)

of Table 19.2-1, and

Eq.

(B) in Table 19.2-3. Show that these three equations are

equivalent.

(b) Show hdv to get Eq. 19.1-15 from Eq. 19.1-11.

19C.1. Alternate form of the binary diffusion equation. In the absence of chemical reactions, Eq.

19.1-17 can be written in terms of v rather than v* by using a different measure of concentra-

tion-namely, the logarithm of the mean molecular weight:'

in which

xAMA

xBMB.

(Caution:

Solution is lengthy.)

Equation 19C.1-1 is difficult to solve even for the stagnant gas film of 518.2, because

the variable mass density

that appears in the continuity equation (Eq.

of Table 19.2-3).

19D.1. Derivation of the equation of continuity. In

s19.1

the species equation of continuity is

de-

rived by making a mass balance on a small rectangular volume

fixed in space.

(a) Repeat the derivation for an arbitrarily shaped volume element

with a sufficiently

smooth fixed boundary

Show that the species mass balance can be written as

Use the Gauss divergence theorem to convert the surface integral to a volume integral, and

then obtain Eq. 19.1-6.

(b) Repeat the derivation using a region of fluid contained within a surface, each point

which is moving with local mass average velocity.

19D.2. Derivation of the equation of change for temperature for a multicomponent system.

De-

rive Eq.

(F)

in Table 19.2-4 from Eq. (E). We suggest the following sequence of steps:

(a) Since the enthalpy is an extensive thermodynamic property, we can write

in which the

are the masses of the various species, is the sum of the

ma,

and the

m,/m

are the corresponding mass fractions. Both Hand Hare understood to be functions of

and

as well as of composition. Use the chain rule of partial differentiation to show that

Bedingfield,

Jr.,

and

Drew,

Ind.

Eng.

Chem.,

42,1164-1173 (1950).

Problems

609

Subtraction then gives for

The subscript

means "holding all other mass fractions constant."

(b) The left side of

Eq.

(E)

can be expanded

regarding the enthalpy per unit mass to be a

function of

and the first (N

mass fractions:

Next, verify that the coefficients of the substantial derivatives can be identified as

The coefficient of p(Dw,/

Dt)

has already been given in

Eq.

19D.2-4.

(c)

Substitute the coefficients into

Eq.

19D.2-5,

and then use Eq.

19.1-14

to eliminate

p(Dw,/Dt), and verify that (dH/dma)p,T,,y is the same as (HJM,). The summation on

which

goes from

now has to be appropriately rewritten as

summation from

to N, by

using

Eq.

(K)

of Table

17.8-1

and the fact that Z,ra

(d)

Then combine the results of (a),

(b),

and (c) with

Eq.

(E) to get

Eq.

(F).

19D.3.

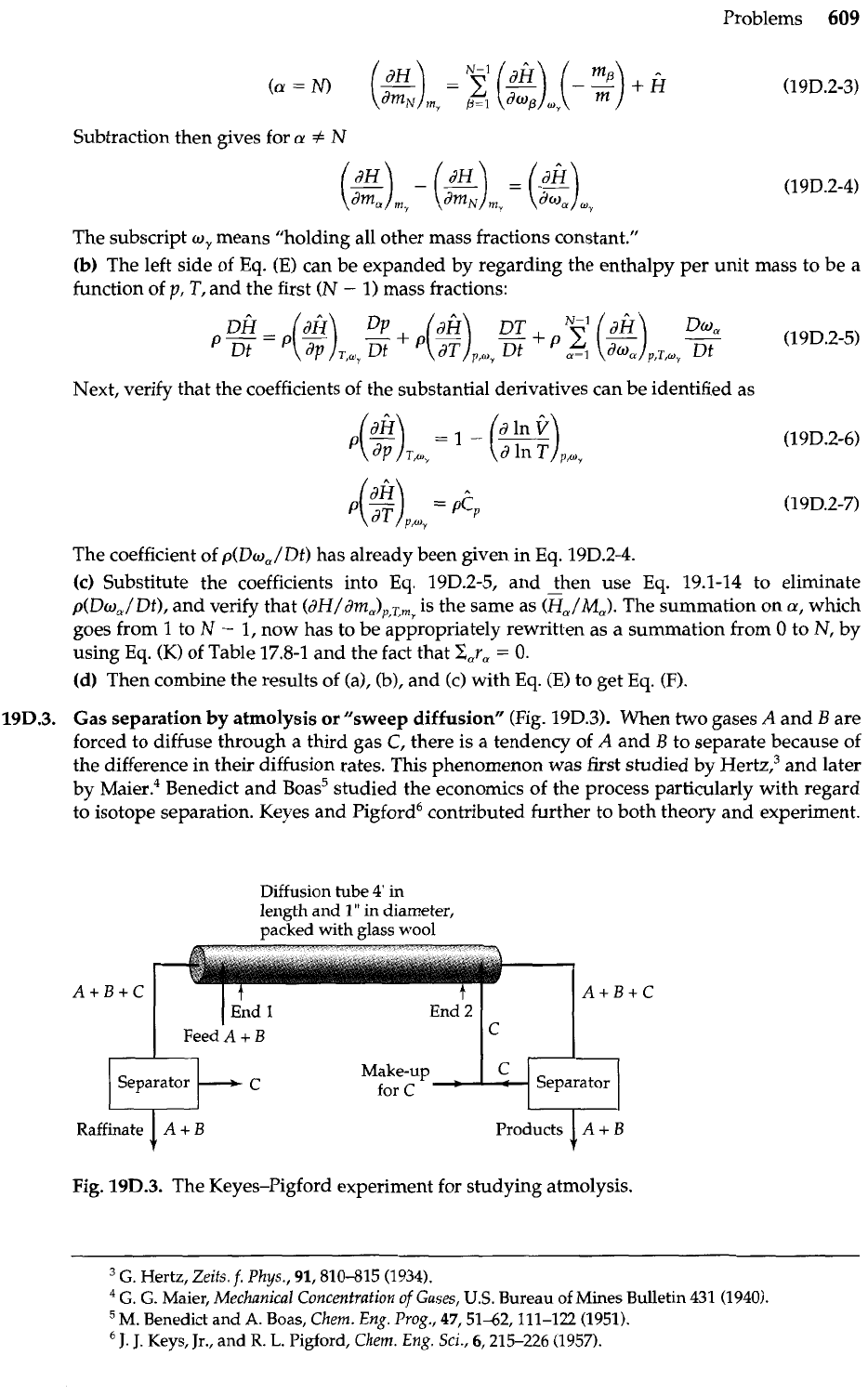
Gas separation by atmolysis or

"sweep

diffusion"

(Fig.

19D.3).

When two gases

and

are

forced to diffuse through a third gas

there is a tendency of

and

to separate because of

the difference in their diffusion rates. This phenomenon was first studied by Hertz? and later

by Maier.* Benedict and Boas5 studied the economics of the process particularly with regard

to isotope separation. Keyes and pigford6 contributed further to both theory and experiment.

Diffusion tube

length and 1

in diameter,

packed with glass

wool

A+B+C A+B+C

1 ~ni'2

Feed

Make-up

Separator for

Separator

Raffinate

Products

Fig.

19D.3.

The Keyes-Pigford experiment for studying atmolysis.

Hertz,

Zeits.

Phys.,

91,810-815 (1934).

G. G.

Maier,

Mechanical Concentration

Gases,

US.

Bureau of Mines Bulletin 431 (1940).

Benedict and

Boas,

Chem. Eng. Prog.,

47,5142,111-122 (1951).

Keys,

Jr.,

and

Pigford,

Chem. Eng.

Sci.,

6,215-226 (1957).

610

Chapter

Equations of Change for Multicomponent Systems

In their experimental arrangement,

was a condensable vapor, which could be separated

from

and

by lowering the temperature so that

would be liquefied.

We want to study the details of the three-component diffusion taking place in the diffu-

sion tube of length

when the apparatus is operated at steady state. Obtain an expression

re-

lating the concentrations x,, and xB1 at the feed end of the tube to the concentrations

X,q

and

xB2 at the product end. This expression will contain the molar fluxes of the three species,

which are controlled by the rates of addition of materials in the two entering streams.

Use the following notation for dimensionless quantities:

z/L

for the distance down

the tube from the feed entrance; rA

9lAB/BAc and

gAB/9BC

for the diffusivity ratios; and

N,,L/c~~~ for the molar fluxes (with

C).

(a) Shows that, in terms of these dimensionless quantities, the Maxwell-Stefan equations for

the diffusion are

where

YAA

rA(vA

vC),

YAS

vA(rA

I),

and

-YAVA, and the remaining quantities

are obtained by interchanging

and

(b)

By using Laplace transforms, solve Eqs.

19D.3-1

and

to get the concentration profiles for

and

in the tube.

(c)

Show that the terminal concentrations are interrelated thus,

XA(~AI, XRI;

XA(~AI, xm;

p+)

exp

XA(xA1,

r,,;

p-)

exp

X~2

P+P-

p+(p+

p-)

(19D.3-3)

P-(P-

p+)

in which

similar expression may be obtained for

xB2.

Keyes and Pigford6 give further results for spe-

cial cases.

19D.4.

Steady-state diffusion from a rotating disk.7

large disk is rotating with an angular veloc-

ity

in an infinite expanse of liquid

The surface is coated with a material A that is slightly

soluble in

Find the rate at which A dissolves in

(The solution to this problem can be ap-

plied to a disk of finite radius

with negligible error.)

The fluid dynamics of this problem was developed by von KBrmBn8 and later corrected by

C~chran.~ It was found that the velocity components can be expressed, except near the edge, as

in which

zmv.

The functions F,

and

have the following expansions:

in which

0.510

and

-0.616.

It is further known that, in the limit as

-0.886,

and

F',

and

all approach zero. Also it is known that the boundary layer thick-

ness is proportional to

except near the edge of the disk.

Levich,

Physicochemical Hydrodynamics,

Prentice-Hall, Englewood

Cliffs,

N.J.

(1962),

§11.

von

Kirmhn,

Zeits.

angew.

Math.

Mech.,l,

244-247

(1921).

Cochran,

Proc.

Camb. Phil.

Soc.,

30,365-375

(1934).

Problems

611

The diffusion equation of Eq. 19.1-16 with the known velocity components is to be solved

under the boundary conditions that:

p,,

and

dp,/dr

Since there can be but one solution to this linear problem, it may be seen that a solution

of the form

p,(z)

can be found that satisfies the differential equation and all the boundary con-

ditions. Thus, the solution for

does not depend on the radial coordinate in the region

considered.

(a)

Show that at steadystate

Eq.

19.1-16 gives

(b)

Solve

Eq.

19D.4-5 to get, for large Schmidt number,

(c)

Show that the mass flux at the surface of the disk is7

for large Schmidt number. Clearly, if desired, one could use higher terms in the series expan-

sion for

and extend the Schmidt-number range.10 This system has been used for studying

the removal of solid behenic acid from stainless-steel surfaces."

Schuhmann,

Physicochemical Hydrodynamics

(V.

Levich Fextschrift),

Vol.

1 (D.

Spalding ed.),

Advance Publications Ltd., London

(1977),

pp.

44.5459;

see also

K.-T.

Liu

and

Stewart,

Intl.

Jnl.

Heat and Mass

Trf.,

15,187-189 (1972).

Grant,

Perka,

Thomas, and

Caton,

AIChE

Journal,

42,1465-1476 (1996).

Chapter

Concentration Distributions

with More Than One

Independent Variable

520.1 Time-dependent diffusion

520.2~ Steady-state transport in binary boundary layers

520.3.

Steady-state boundary layer theory for flow around objects

520.4. Boundary layer mass transport with complex interfacial motion

520.5. Taylor dispersion in laminar tube flow

Most of the diffusion problems discussed in the preceding two chapters led to ordinary

differential equations for the concentration profiles. In this chapter we use the general

equations of Chapter 19 to set up and solve some diffusion problems that lead to partial

differential equations.

large number of diffusion problems can be solved by simply looking up the solu-

tions to the analogous problems in heat conduction. When the differential equations and

the boundary and initial conditions for the diffusion process are of exactly the same form

as those for the heat conduction process, then the heat conduction solution may be taken

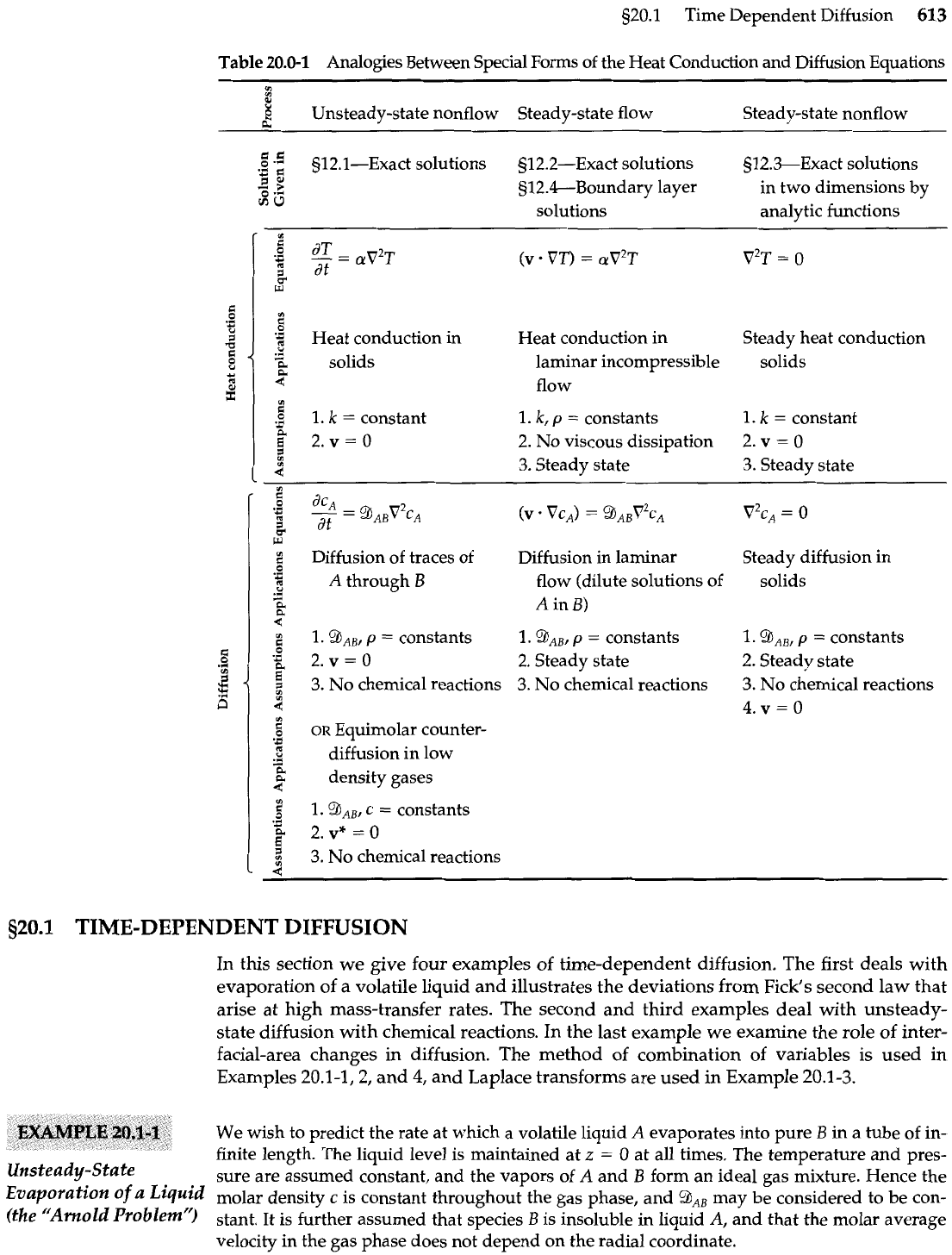
over with appropriate changes in notation. In Table 20.0-1 the three main heat transport

equations used in Chapter 12 are shown along with their mass transport analogs. Many

solutions to the nonflow equations may be found in the monographs of Carslaw and

Jaeger' and of Crank.'

Because the diffusion problems described by the equations in Table 20.0-1 are analo-

gous to the problems of Chapter 12, we do not discuss them extensively here. Instead,

we focus primarily on problems involving diffusion with chemical reactions, diffusion

with a moving interface, and diffusion with rapid mass transfer.

In 920.1 we discuss a variety of time-dependent diffusion problems. In s20.2 we pre-

sent some steady-state boundary layer problems involving binary mixtures. This is fol-

lowed by two boundary layer analyses for more complicated systems: the diffusion in

steady flow around arbitrary objects in 920.3, and the diffusion in flows yvith complex in-

terfacial motion in 920.4. Finally, in

520.5

we explore an asymptotic solution to the "Tay-

lor dispersion" problem.

Carslaw and

C. Jaeger,

Conduction of Heat in Solids,

2nd edition, Oxford University Press

(1959).

Crank,

The Mathematics

Di@sion,

2nd edition, Clarendon Press, Oxford

(1975).

S20.1 Time Dependent Diffusion

613

Table

20.0-1

Analoges Between Special Forms of the Heat Conduction and Diffusion Equations

Unsteady-state nonflow Steady-state flow

Steady-state nonflow

§12.1-Exact solutions 512.2-Exact solutions

s12.3-Exact solutions

512.4--Boundary layer in two dimensions by

solutions

analytic functions

Heat conduction in Heat conduction in Steady heat conduction

solids laminar incompressible solids

flow

constant

constants 1.

constant

2.v=O

No viscous dissipation 2.

Steady state

Diffusion of traces of

through

%ABr

constants

2.v=o

No chemical reactions

Equimolar counter-

diffusion in low

density gases

Diffusion in laminar

Steady diffusion in

flow (dilute solutions of solids

A in

9AB,

constants

%ABI

constants

2. Steady state

Steady state

No chemical reactions

4.v

BAB,

constants

No chemical reactions

$20.1

TIME-DEPENDENT DIFFUSION

In this section we give four examples of time-dependent diffusion. The first deals with

evaporation of a volatile liquid and illustrates the deviations from Fick's second law that

arise at high mass-transfer rates. The second and third examples deal with unsteady-

state diffusion with chemical reactions. In the last example we examine the role of inter-

facial-area changes in diffusion. The method of combination of variables is used in

Examples 20.1-1,2, and

and Laplace transforms are used in Example 20.1-3.

We wish to predict the rate at which a volatile liquid

evaporates into pure

a tube of in-

finite length. The liquid level is maintained at

at all times. The temperature and pres-

Unsteady-State

sure are assumed constant, and the vapors of A and

form an ideal gas mixture. Hence the

Evaporation of

Liquid

molar density

is constant throughout the gas phase, and BAB may be considered to be con-

(the "Amold Problem'')

stant. It is further assumed that species

is insoluble in liquid A, and that the molar average

velocity in the gas phase does not depend on the radial coordinate.

614

Chapter 20 Concentration Distributions with More Than One Independent Variable

SOLUTION

For this system the equation of continuity for the mixture, given in Eq. 19.1-12, becomes

in which

is the z-component of the molar average velocity. Integration with respect to

gives

Here and elsewhere in this problem, the subscript

"0"

indicates a quantity evaluated at

According to

Eq.

(M)

of Table 17.8-1, this velocity can be written in terms of the molar fluxes

and

However,

N,,,

is zero because of the insolubility of species

in liquid

Then use of Eq.

(D)

of Table 17.8-2 gives finally

in which

XAO

is the interfacial gas-phase concentration, evaluated here on the assumption of

interfacial equilibrium. For an ideal gas mixture this is just the vapor pressure of pure

di-

vided by the total pressure.

The equation of continuity of

Eq.

19.1-17 then becomes

This is to be solved with the initial and boundary conditions:

LC.:

B.C. 1:

B.C. 2:

We can try the same kind of combination of variables used in Example 4.1-1; namely,

xA/xA0

and

z/-.

However, since

Eq.

20.1-5 contains the parameter

XA~,

we can an-

ticipate that

will depend not only on

but also parametrically on

xAo.

In terms of these dimensionless variables, Eq. 20.1-5 can be written as

Here the quantity

is a dimensionless molar average velocity,

v:m,

as can be seen by comparing

Eqs,

20.1-10 and 20.1-4. The initial and boundary conditions in Eqs. 20.1-6 to 8 now become

B.C. 1:

B.C. 2 and I.C.:

Equation 20.1-9 can be attacked by first letting

dX/dZ

This gives a first-order differential

equation for

that can be solved to obtain

920.1 Time Dependent Diffusion

615

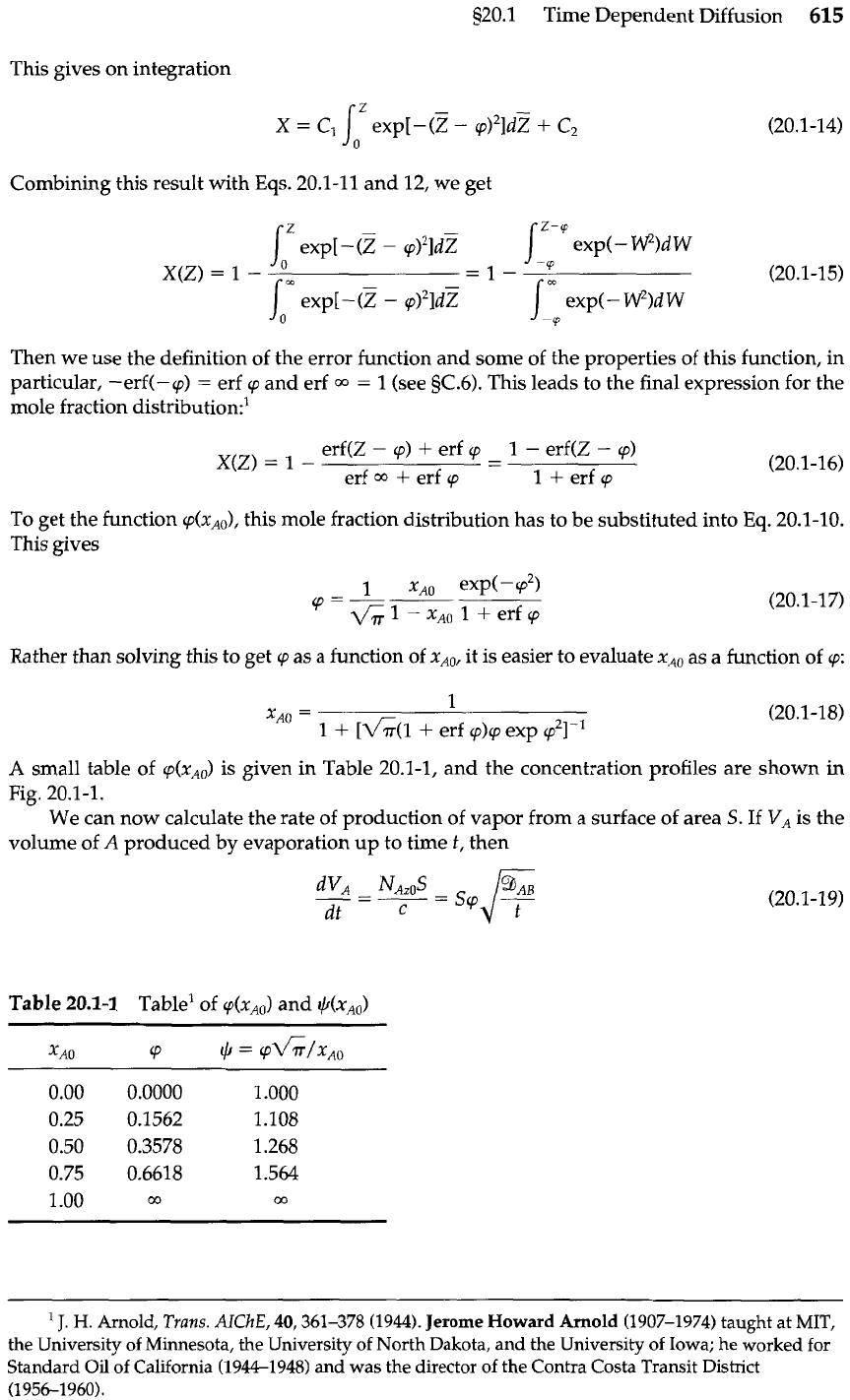
This gives on integration

Combining this result with Eqs. 20.1-11 and 12, we get

Then we use the definition of the error function and some of the properties of this function, in

particular, -erf(-cp)

erf

and erf

(see 5C.6). This leads to the final expression for the

mole fraction distribution:'

erf(Z

cp)

erf

erf(Z

cp)

X(Z)

erf

To get the function cp(xA0), this mole fraction distribution has to be substituted into

Eq.

20.1-10.

This gives

Rather than solving this to get

as a function of xAo, it is easier to evaluate xAo as a function of 9:

X~~

[l/;f(l

erf q)cp exp

cp21-'

small table of p(xAo) is given in Table 20.1-1, and the concentration profiles are shown

Fig. 20.1-1.

We can now calculate the rate of production of vapor from a surface of area

is the

volume of

produced by evaporation up to time

then

Table

20.1-1

able'

of (p(xAo) and $(xA,)

Arnold,

Trans.

AIChE,

40,361-378 (1944).

Jerome

Howard

Arnold

(1907-1974)

taught at

MIT,

the University of Minnesota, the University

North Dakota, and

the

University of Iowa; he worked for

Standard

Oil

of California

(1944-1948)

and was the director of the Contra Costa Transit District

(1956-1960).

616

Chapter 20 Concentration Distributions with More Than One Independent Variable

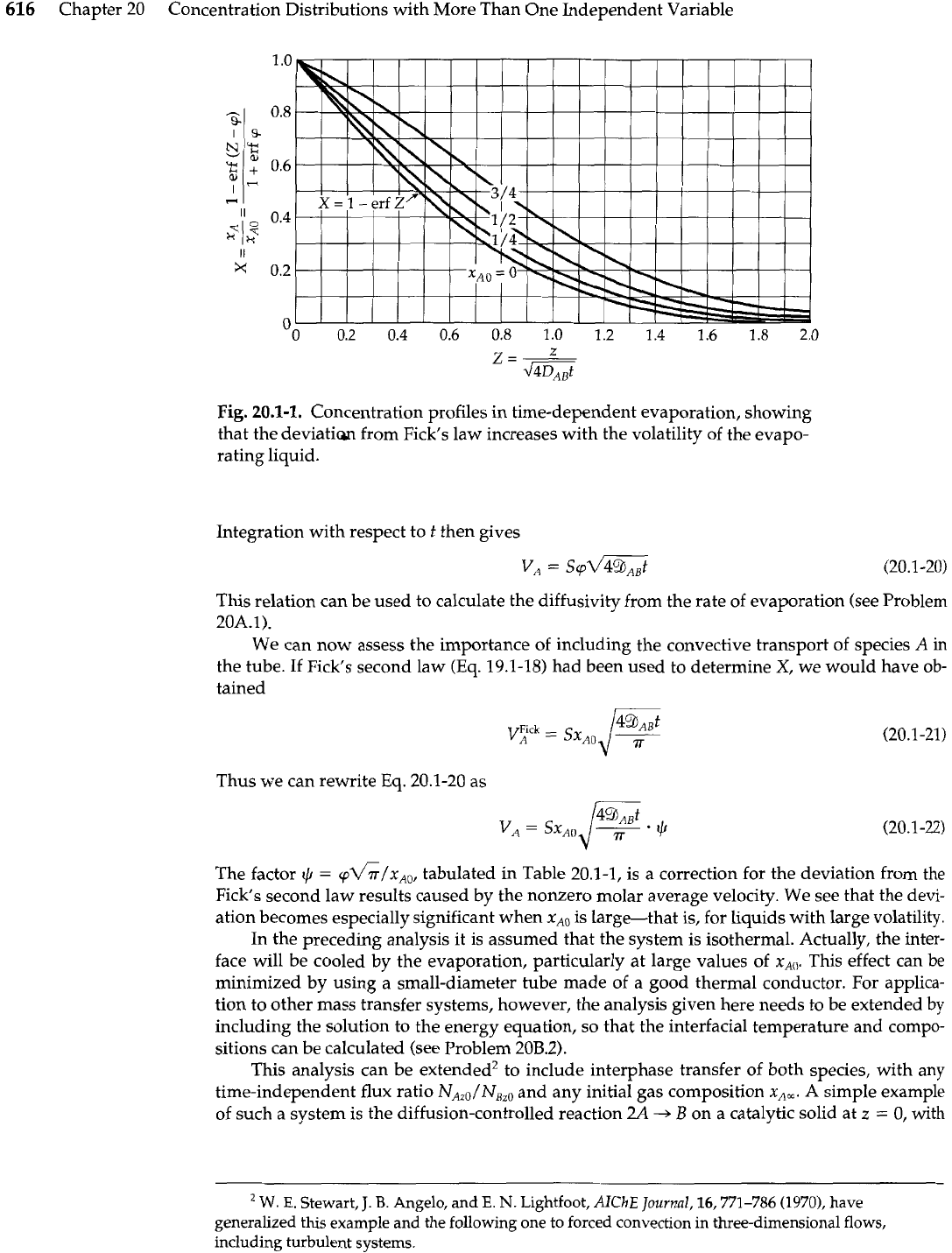
Fig. 20.1-1.

Concentration profiles in time-dependent evaporation, showing

that the deviati~ from Fick's law increases with the volatility of the evapo-

rating liquid.

Integration with respect to

then gives

This relation can be used to calculate the diffusivity from the rate of evaporation (see Problem

20A.1).

We can now assess the importance

including the convective transport of species

the tube. If Fick's second law

(Eq.

19.1-18) had been used to determine

we would have

ob-

tained

Thus we can rewrite Eq.

20.1-20

The factor

(Pfi/~AO,

tabulated in Table 20.1-1, is

correction for the deviation from the

Fick's second law results caused by the nonzero molar average velocity. We see that the devi-

ation becomes especially significant when

xAO

is large-that is, for liquids with large volatility.

In the preceding analysis it is assumed that the system is isothermal. Actually, the inter-

face will be cooled by the evaporation, particularly at large values of

xA0.

This effect can

minimized by using a small-diameter tube made of a good thermal conductor. For applica-

tion to other mass transfer systems, however, the analysis given here needs to be extended

including the solution to the energy equation, so that the interfacial temperature and compo-

sitions can be calculated (see Problem 20B.2).

This analysis can be extended2 to include interphase transfer of both species, with any

time-independent flux ratio NAzO/NBzo and any initial gas composition

x,,.

A simple example

of such a system is the diffusion-controlled reaction

on a catalytic solid at

0, with

Stewart,

Angelo, and

Lightfoot,

AlChE

Journal,

16,771-786

(19701,

have

generalized

this

example and the following one to forced convection in three-dimensional flows,

including turbulent

systems.