Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena
Подождите немного. Документ загружается.


57.5 Estimation of the Viscous Loss
207
Table
7.5-1
Brief Summary of Friction Loss Factors for Use with Eq. 7.5-10
(Approximate Values for Turbulent Flow)"
Disturbances
e,
Sudden changes in cross-sectional areab
Rounded entrance to pipe 0.05
Sudden contraction
Sudden expansionc
Orifice (sharp-edged)
Fittings and valves
90" elbows (rounded) 0.4-0.9
90" elbows (square) 1.3-1.9
45" elbows 0.3-0.4
Globe valve (open) 610
Gate valve (open) 0.2
"
Taken from
H.
Kramers,
Physische
Transportverschijnselen,
Technische Hogeschool Delft, Holland
(19581,
pp.
53-54.
Here
p
=
(smaller cross-sectional area)/(larger cross-sectional area).
See derivation from the macroscopic balances in Example
7.6-1.
If
P
=
0,
then
E,
=
:(v)',
where
(v)
is the
velocity
upstream
from the enlargement.
Most flow systems contain various "obstacles," such as fittings, sudden changes
in
diameter, valves, or flow measuring devices. These also contribute to the friction loss
ED.
Such additional resistances may be written in the form of
Eq.
7.5-4,
with
e,
determined by
one of two methods:
(a)
simultaneous solution of the macroscopic balances, or
(b)
experi-
mental measurement. Some rough values of
e,
are tabulated in Table 7.5-1 for the conven-
tion that
(v)
is the average velocity
downstream
from the disturbance. These
e,
values are
for
turbulent flow
for which the Reynolds number dependence
is
not too important.
Now we are in a position to rewrite
Eq.
7.4-7 in the
approximate
form frequently used
for
turbulent flow
calculations in a system composed of various kinds of piping and addi-
tional resistances:
12L
:(z$
-
v:)
+
~(1~
-
zli
+
/C
$
dp
=
hm
-
Z
jiY
Rif)i
-
(v2e)
i
(7.5-10)
sum over
all
sum over
all
sections of fittings, valves,
straight conduits meters, etc.
Here
Rh
is the mean hydraulic radius defined in
Eq.
6.2-16,
f
is the friction factor defined
in
Eq.
6.1-4, and
e,
is the friction loss factor given in Table 7.5-1. Note that the
v,
and
v2
in
the first term refer to the velocities at planes 1 and
2;
the
v
in the first sum is the average
velocity in the ith pipe segment; and the v in the second sum is the average velocity
downstream
from the ith fitting, valve, or other obstacle.
What is the required power output from the pump at steady state in the system shown
in
Fig.
7.5-l? Water at 68OF
(p
=
62.4 lb,/ft3;
p
=
1.0 cp) is to be delivered to the upper tank at a rate
Requirement
of 12 ft3/min. All of the piping is 4-in. internal diameter smooth circular pipe.
for
Pipeline
Flow

208
Chapter 7 Macroscopic Balances for Isothermal Flow Systems
SOLUTION
Fig.
7.5-1.
Pipeline flow
with friction losses be-
cause of fittings. Planes
1
and
2
are just under
-
-
Plane
2
the surface of the liquid.
The average velocity in the pipe is
and the Reynolds number is
Hence the flow is
turbulent.
The contribution to
i,
from the various lengths of pipe
will
be
The contribution to
k,
from the sudden contraction, the three
90"
elbows, and the sudden ex-
pansion (see Table 7.5-1) will be
2
($v2eJi
=
$(2.30)~(0.45
+
3(;)
+
1)
=
8 ft2/s2
(7.5-14)
I
Then from Eq. 7.5-10 we get
0
+
(32.2)(105
-
20)
+
0
=
wrn
-
85
-
8
(7.5-15)
Solving for
wrn
we get
This is the work (per unit mass of fluid) done
on
the fluid
in
the pump. Hence the pump does
2830 ft2/s2 or 2830/32.2
=
88 ft lbf/lbrn of work on the fluid passing through the system.
The
mass rate of flow is
Consequently
Wrn
=
W&
=
(12.5)(88)
=
1100 ft lbf/s
=
2 hp
=
1.5 kW
(7.5-18)
which is the power delivered by the pump.
s7.6
Use of the Macroscopic Balances for Steady-State Problems
209
Table
7.6-1
Steady-State Macroscopic Balances for Turbulent Flow in Isothermal Systems
Mass:
CW,
-
Zw2
=
O
(A)
Momentum:
X(vlwl
+
plS1)ul
-
C(v2~2
+
p2S2h2
+
mtot!4
=
Ff-s
(B)
Mechanical energy:
C
w2
=
-
W,
+
E,
+
E,
(D)
Notes:
(a) All formulas here assume flat velocity profiles.
(b)
Zwl
=
w,,
+
wlb
+
wlc
+
.
.
.
,
where
w,,
=
p,,v,,S,,,
etc.
(c)
hl
and
h,
are elevations above an arbitrary datum plane.
(d) All equations are written for compressible flow;
for
incompressible flow,
E,
=
0.
57.6
USE OF THE MACROSCOPIC BALANCES
FOR STEADY-STATE PROBLEMS
In
53.6
we saw how to set up the differential equations to calculate the velocity and pres-
sure profiles for isothermal flow systems by simplifying the equations of change. In this
section we show how to use the set of steady-state macroscopic balances to obtain the al-
gebraic equations for describing large systems.
For each problem we start with the four macroscopic balances. By keeping track of
the discarded or approximated terms, we automatically have a complete listing of the as-
sumptions inherent in the final result. All of the examples given here are for isothermal,
incompressible flow. The incompressibility assumption means that the velocity of the
fluid must be less than the velocity of sound in the fluid and the pressure changes must
be small enough that the resulting density changes can be neglected.
The steady-state macroscopic balances may be easily generalized for systems with
multiple inlet streams (called la,
Ib,
lc,
. .
.)
and multiple outlet streams (called 2a, 2b,
2c,
.
.
.).
These balances are summarized in Table 7.6-1 for turbulent flow (where the ve-
locity profiles are regarded as flat).
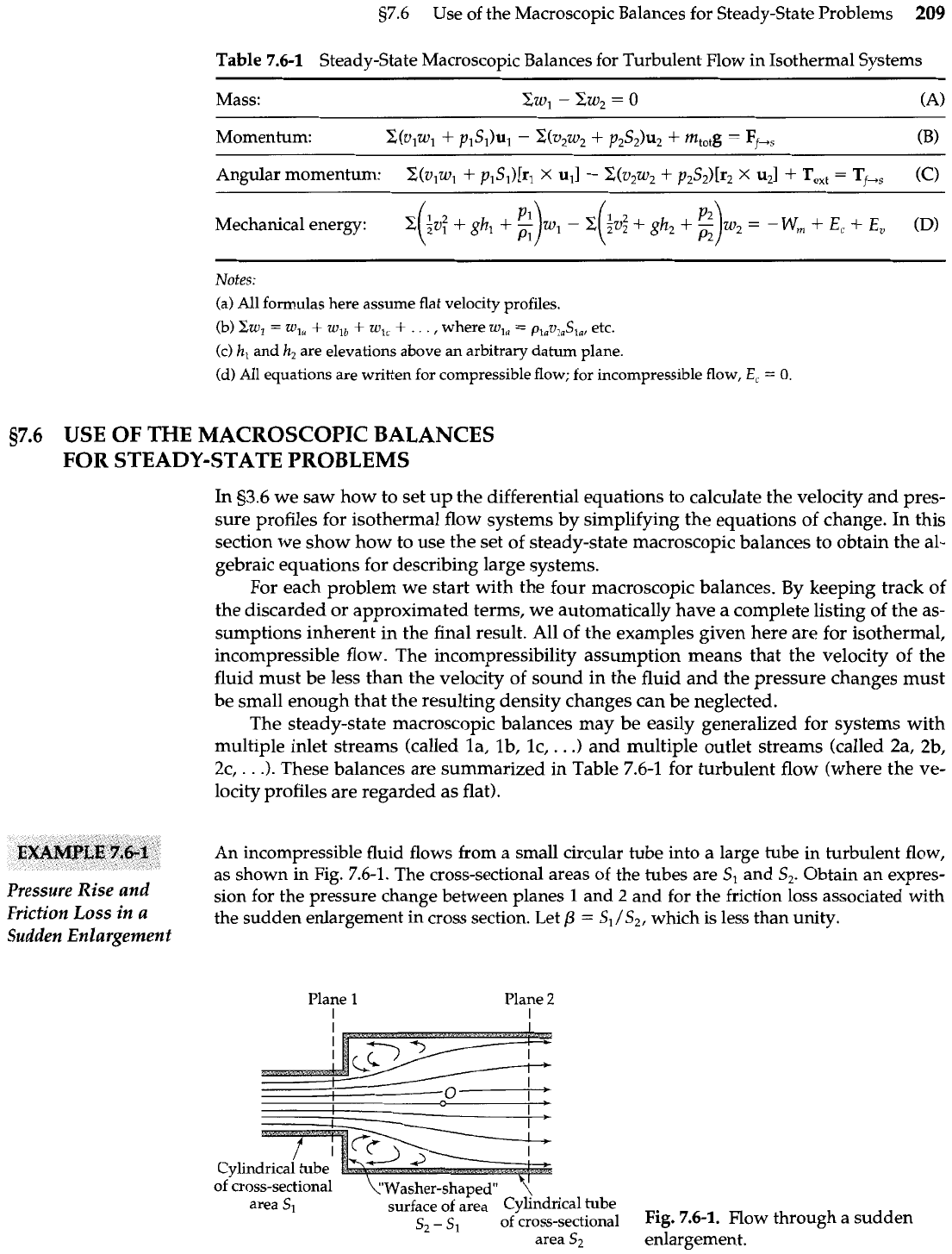
An incompressible fluid flows from a small circular tube into a large tube in turbulent flow,
as shown in
Fig.
7.6-1. The cross-sectional areas of the tubes are
S,
and
S2.
Obtain an expres-
Pressure Rise and
sion for the pressure change between planes
1
and
2
and for the friction loss associated with
Friction Loss in
a
the sudden enlargement in cross section. Let
P
=
S,/S2,
which is less than unity.
Sudden Enlargement
Plane
1
Plane
2
I
I
area
S1
surface of area Cylindrical hibe
s2
-
s1
of
cross-sectiona~
Fig.
7.6-1.
HOW through a sudden
area
S2
enlargement.
210
Chapter 7 Macroscopic Balances for Isothermal Flow Systems
SOLUTION
(a)
Mass balance.
For steady flow the mass balance gives
For a fluid of constant density, this gives
(b)
Momentum balance.
The downstream component of the momentum balance is
The force
Ff,,
is composed of two parts: the viscous force on the cylindrical surfaces parallel
to the direction of flow, and the pressure force on the washer-shaped surface just to the right
of plane
1
and perpendicular to the flow axis. The former contribution we neglect (by intu-
ition) and the latter we take to be
p,(S2
-
S,) by assuming that the pressure on the washer-
shaped surface is the same as that at plane
1.
We then get, by using Eq. 7.6-1,
Solving for the pressure difference gives
or, in terms of the downstream velocity,
Note that the momentum balance predicts (correctly) a
rise
in pressure.
(c)
Angular momentum balance.
This balance is not needed. If we take the origin of coor-
dinates on the axis of the system at the center of gravity of the fluid located between
planes
1
and 2, then [r,
X
u,l
and
[r2
X
u21
are both zero, and there are no torques on the
fluid system.
(dl
Mechanical energy balance.
There is no compressive loss, no work done via moving
parts, and no elevation change, so that
*
I
1
Ev
=
,(v:
-
v:)
+
-
P
(p,
-
p2)
Insertion of Eq. 7.6-6 for the pressure rise then gives, after some rearrangement,
which is an entry in Table 7.5-1.
This example has shown how to use the macroscopic balances to estimate the friction loss
factor for a simple resistance in a flow system. Because of the assumptions mentioned after
Eq. 7.6-3, the results in Eqs. 7.6-6 and
8
are approximate. If great accuracy is needed, a correc-
tion factor based on experimental data should be introduced.
A
diagram of a liquid-liquid ejector is shown in Fig. 7.6-2. It is desired to analyze the mixing
of the two streams, both of the same fluid, by means of the macroscopic balances. At plane
1
Petfowance of
a
the two fluid streams merge. Stream la has a velocity
v,
and a cross-sectional area is,, and
LiPid-LiPid Ejector
stream
lb
has a velocity
iv,
and a cross-sectional area $5,. Plane
2
is chosen far enough down-
stream that the two streams have mixed and the velocity is almost uniform at
v,.
The flow is

57.6 Use of the Macroscopic Balances for Steady-State Problems
211
SOLUTION
Stream lb
Fig.
7.6-2.
Flow in a liquid-liq-
uid ejector pump.
turbulent and the velocity profiles at planes
1
and
2
are assumed to be flat. In the following
analysis
F+,
is neglected, since it is felt to be less important than the other terms in the mo-
mentum balance.
(a)
Mass balance.
At steady state, Eq.
(A)
of Table 7.6-1 gives
Hence, since
S,
=
S2, this equation gives
for the velocity of the exit stream. We also note, for later use, that
w,,
=
wlb
=
$w2.
(b)
Momentum balance.
From Eq. (B) of Table 7.6-1 the component of the momentum bal-
ance in the flow direction is
or using the relation at the end of (a)
from which
2
p2
-
PI
=
$Po
This is the expression for the pressure rise resulting from the mixing of the two streams.
(c)
Angular momentum balance.
This balance is not needed.
(d)
Mechanical energy balance.
Equation
(D)
of Table 7.6-1 gives
or, using the relation at the end of (a), we get
Hence
is the energy dissipation per unit mass. The preceding analysis gives fairly good results for
liquid-liquid ejector pumps. In gas-gas ejectors, however, the density varies significantly and
it is necessary to include the macroscopic total energy balance as well as an equation of state
in the analysis. This is discussed in Example 15.3-2.
212
Chapter 7 Macroscopic Balances for Isothermal Flow Systems
EXAMPLE
7.6-3
Thrust
on
a
Pipe
Bend
SOLUTION
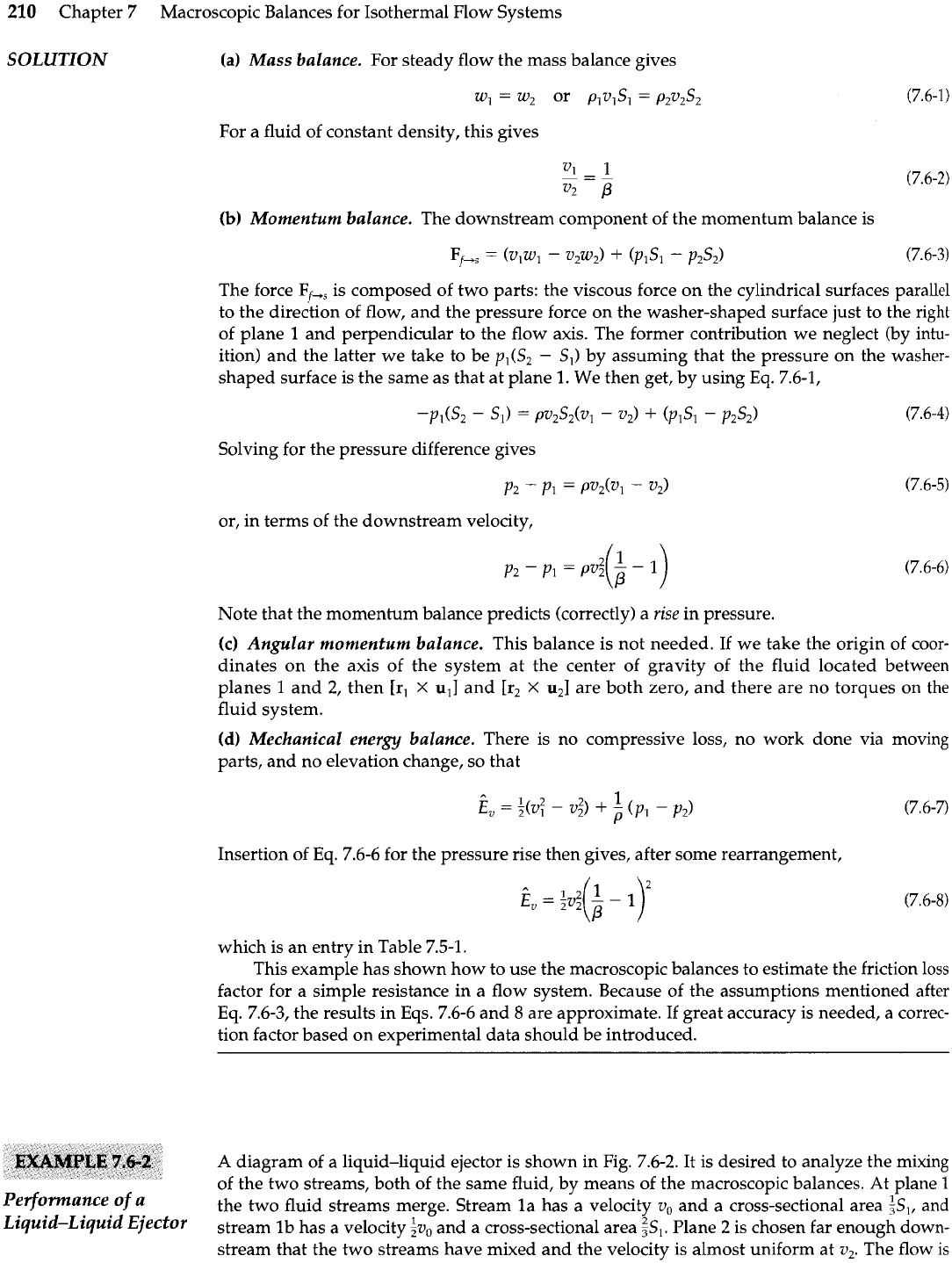
Water at 95°C is flowing at a rate of 2.0 ft3/s through a 60" bend, in which there is a contrac-
tion from 4 to 3 in. internal diameter (see Fig. 7.6-3). Compute the force exerted on the bend if
the pressure at the downstream end is 1.1 atm. The density and viscosity of water at the con-
ditions of the system are 0.962 g/cm3 and 0.299 cp, respectively.
The Reynolds number for the flow in the 3-in. pipe is
At this Reynolds number the flow is highly turbulent, and the assumption of flat velocity pro-
files is reasonable.
(a)
Mass balance.
For steady-state flow,
w1
=
w,.
If the density is constant throughout,
in which
/3
is the ratio of the smaller to the larger cross section.
(b)
Mechanical energy balance.
For steady, incompressible flow,
Eq.
(d) of Table 7.6-1
be-
comes, for this problem,
According to Table 7.5-1 and
Eq.
7.5-4, we can take the friction loss as approximately g(4v:)
=
iv:. Inserting this into
Eq.
7.6-20 and using the mass balance we get
This is the pressure drop through the bend in terms of the known velocity v2 and the known
geometrical factor
P.
(c)
Momentum balance.
We now have to consider both the
x-
and y-components of the mo-
mentum balance. The inlet and outlet unit vectors will have
x-
and y-components given
by
ulw
=
1,
uly
=
0,
u2
=
cos
8, and
u,,
=
sin 8.
Fluid
out
4"
internal
Is
=
520
Fig.
7.6-3.
Reaction force at a reducing
diameter
bend in a pipe.

37.6 Use of the Macroscopic Balances for Steady-State Problems
213
The x-component of the momentum balance then gives
where
F,
is the x-component of
F,+.
Introducing the specific expressions for
w,
and u12, we
get
F,
=
v,(pvlS,)
-
vJPv~SJ
cos
0
+
plS1
-
p2S2
cos
8
=
pv;S2(p
-
cos 0)
+
(pl
-
p2)S1
+
p2(S1
-
S2 cos 6)
(7.6-23)
Substituting into this the expression for p,
-
p2 from Eq. 7.6-21 gives
F,
=
pv;S2(@
-
cos
6)
+
pv;s2pp1(&
-
$p2)
+
pg(h2
-
h,)S2p-'
+
p2S2(p-'
-
cos 0)
=
w2(ps2)-'(p
-
COS
0
+
$1
+
pg(h2
-
h1)S2p-'
+
p2S2(p-'
-
cos
0)
The y-component of the momentum balance is
Fy
=
-(v2w2
+
p2S2) sin
8
-
m,,,g
Fy
=
-w2(pS2)-I sin 0
-
p2S2 sin 0
-
vR2Lpg
in which
R
and
L
are the radius and length of a roughly equivalent cylinder.
We now have the components of the reaction force in terms of known quantities. The nu-
merical values needed are
p
=
60 lb,/ft3
w
=
(2.0)(60)
=
1201b,/s
cos
e
=
;
sin 6
=
+fi
p2
=
16.2 lbf/in.'
With these values we then get
F
=-
(120)2
(d)
I
(16.2)(0.049)(144)
2(0.049)(32.2)
2
Hence the magnitude of the force is
IF
I
=
=
d304'
+
2342
=
384 Ibf
=
1708
N
(7.6-29)
The angle that this force makes with the vertical is
a
=
arctan(F,/Fy)
=
arctan 1.30
=
52"
(7.6-30)
In looking back over the calculation, we see that all the effects we have included are impor-
tant, with the possible exception of the gravity terms of 2.6 Ibf in
F,
and 2.5 Ibi in
F,.

214
Chapter 7 Macroscopic Balances for Isothermal Flow Systems
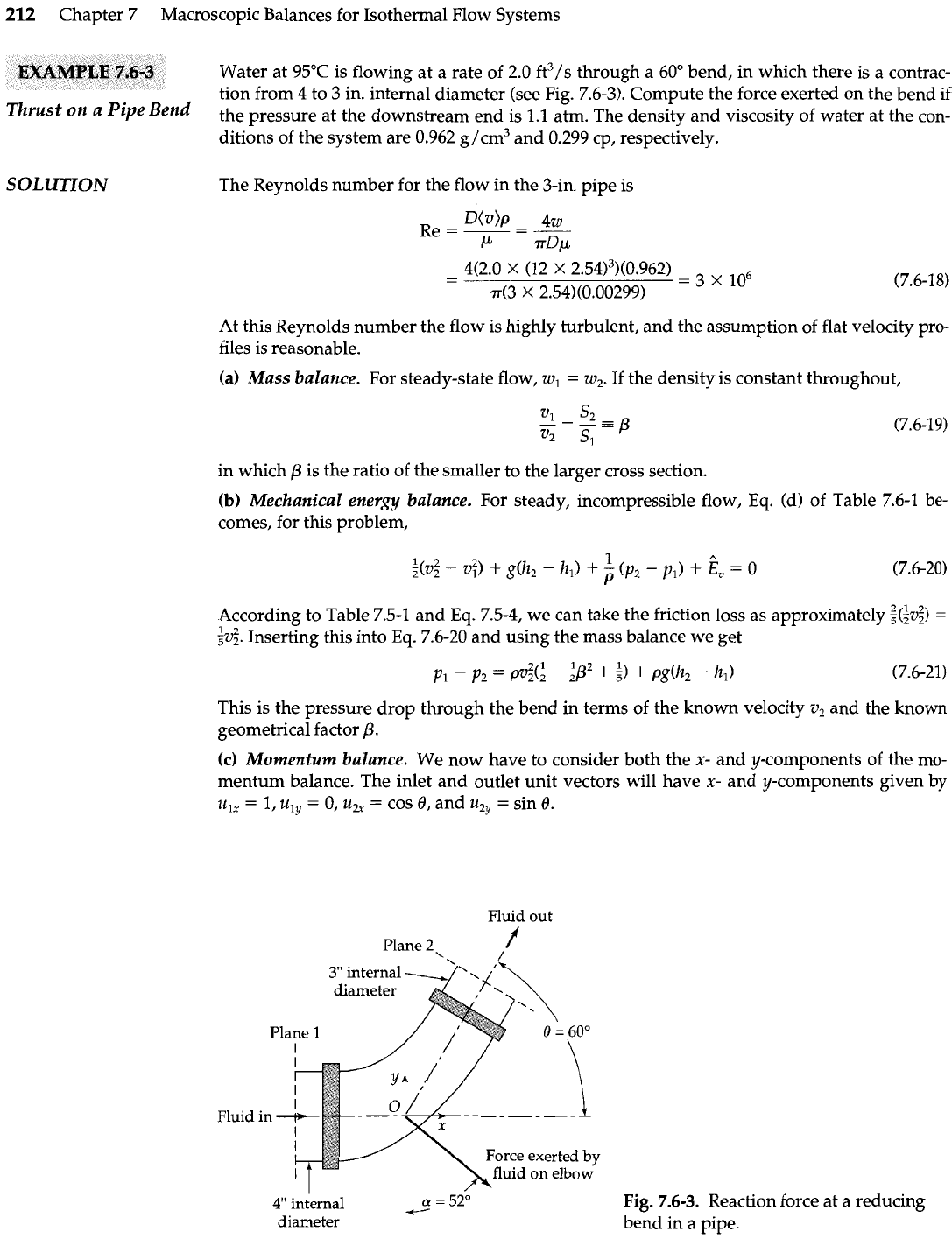
A
rectangular incompressible fluid jet of thickness
b,
emerges from a slot of width c, hits a flat
plate and splits into two streams of thicknesses
bZa
and
bZb
as shown in Fig. 7.6-4. The emerg-
The
Impinging Jet
ing turbulent jet stream has a velocity
v,
and a mass flow rate w,. Find the velocities and mass
rates of flow in the two streams on the plate.'
SOLUTION
We neglect viscous dissipation and gravity, and assume that the velocity profiles of all three
streams are flat and that their pressures are essentially equal. The macroscopic balances then
give
Mass balance
WI
=
W2a
+
W2b
Momentum balance
(in the direction parallel to the plate)
VlWl
COS
6
=
'U2aW2a
-
v2bW2b
(7.6-32)
Mechanical energy balance
iv?wl
=
+
&$b~2b
(7.6-33)
Angular momentum balance
(put the origin of coordinates on the centerline of the jet and
at
an altitude of
gb,;
this is done so that there will be no angular momentum of the incoming jet)
This last equation can be rewritten to eliminate the b's in favor of the w's. Since w1
=
pv,b,c
and w,,
=
pvzab2,c, we can replace
b,
-
b,,
by (w,/pv,c)
-
(w2,/pv2,c) and replace
b,
-
bZb
cor-
respondingly. Then the angular momentum balance becomes
Velocity
ass rate of flow
wl
city
Mass rate of flow
b2b
Plate
b2a
Mass rate of flow
W2b
W2a
Fig.
7.6-4.
Jet impinging on a wall and splitting into two streams. The point
0,
which is the origin of coordinates for the angular momentum balance, is
taken to be the intersection of the centerline of the incoming jet and a plane
that is at an elevation
ib,.
'
For alternative solutions to this problem, see
G.
K.
Batchelor,
An
Introduction
to
Fluid
Dynamics,
Cambridge University Press (1967), pp. 392-394, and
S.
Whitaker,
Introduction to Fluid Dynamics,
Prentice-Hall, Englewood Cliffs,
N.J.
(1968), p. 260. An application of the
compressible
impinging jet
problem has been given by
J.
V.
Foa,
U.S.
Patent 3,361,336 Uan. 2,1968). There, use is made of the fact
that if the slot-shaped nozzle moves to the left in Fig. 7.6-4 (i.e., left with respect to the plate), then, for
a
compressible fluid, the right stream will
be
cooler than the jet and the left stream will
be
warmer.
s7.6 Use of the Macroscopic Balances for Steady-State Problems
215
Now Eqs. 7.6-31
solved we find that
,32,33, and 36 are four equations with four unknowns. When these are
Hence the velocities of all three streams are equal. The same result is obtained by applying
the classical Bernoulli equation for the flow of an inviscid fluid (see Example 3.5-1).
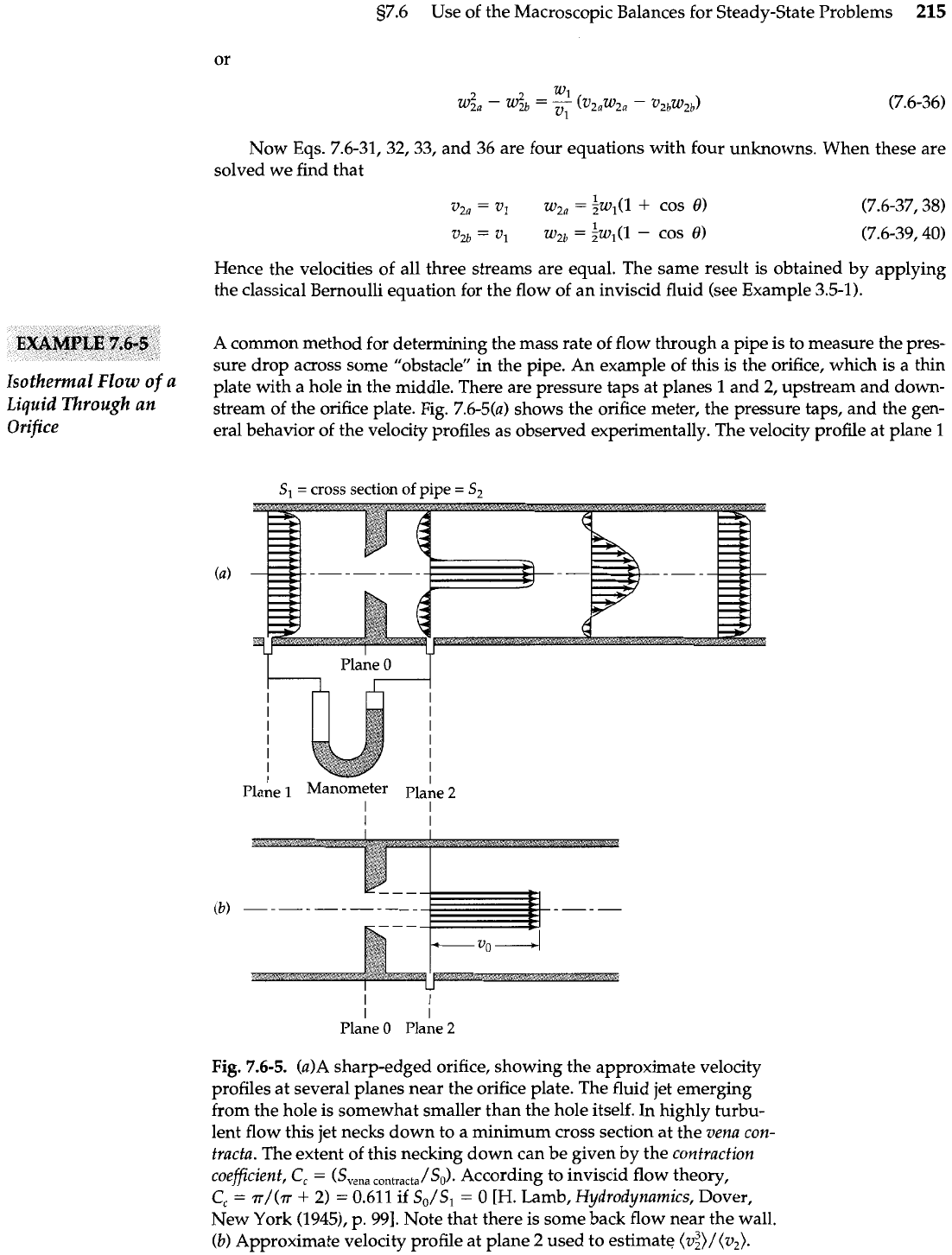
A
common method for determining the mass rate of flow through a pipe is to measure the pres-
sure drop across some "obstacle"
in
the pipe. An example of this
is
the orifice, which is a thin
~so~h~~al Flow
of
a
plate with a hole
in
the middle. There are pressure taps at planes
1
and
2,
upstream and dom-
Liquid
Through
an
stream of the orifice plate. Fig. 7.6-5(a) shows the orifice meter, the pressure taps, and the gen-
Orifice
era1 behavior of the velocity profiles as observed experimentally. The velocity profile at plane
1
S,
=
cross section of pipe
=
S2
I
Plane
0
I
PlaAe
1
hhmneter ~laAe
2
I
I
I I
I
I
I I
Plane
0
Plane
2
Fig.
7.6-5.
(a)A sharp-edged orifice, showing the approximate velocity
profiles at several planes near the orifice plate. The fluid jet emerging
from the hole is somewhat smaller than the hole itself. In highly turbu-
lent flow this jet necks down to a minimum cross section at the vena con-
tracts.
The extent of this necking down can be given by the contraction
coefficient,
C,
=
(S,,,,
c,,,ac,,/S,). According to inviscid flow theory,
C,
=
T/(T
+
2)
=
0.611 if So/Sl
=
0
[H.
Lamb, Hydrodynamics, Dover,
New York (1945), p. 991. Note that there is some back flow near the wall.
(b)
Approximate velocity profile at plane
2
used to estimate (vi)/(v2).

216
Chapter 7 Macroscopic Balances for Isothermal Flow Systems
SOLUTION
will be assumed to be flat. In Fig. 7.6-5(b) we show an approximate velocity profile at plane
2,
which we use in the application of the macroscopic balances. The standard orifice meter equa-
tion is obtained by applying the macroscopic mass and mechanical energy balances.
(a)
Mass balance.
For a fluid of constant density with a system for which
S,
=
S,
=
S,
the
mass balance in Eq. 7.1-1 gives
With the assumed velocity profiles this becomes
and the volume rate of flow is
w
=
pv,S.
(b)
Mechanical energy balance.
For a constant-density fluid in a flow system with no eleva-
tion change and no moving parts,
Eq.
7.4-5 gives
The viscous loss
E,
is neglected, even though it is certainly not equal to zero. With the as-
sumed velocity profiles, Eq. 7.6-43 then becomes
P2
-
P1
;cv;
-
v:,
+
-
=
0
P
(7.6-44)
When Eqs. 7.6-42 and 44 are combined to eliminate
u,,
we can solve for
v,
to get
We can now multiply by pS to get the volume rate of flow. Then to account for the errors
in-
troduced by neglecting
E,
and by the assumptions regarding the velocity profiles we include
a discharge coefficient,
Cd,
and obtain
Experimental discharge coefficients have been correlated as a function of
So/S
and
the
Reynolds n~mber.~ For Reynolds numbers greater than lo4,
Cd
approaches about 0.61 for
all
practical values of
So/S.
This example has illustrated the use of the macroscopic balances to get the general form
of
the result, which is then modified by introducing a multiplicative function of dimensionless
groups to correct for errors introduced by unwarranted assumptions. This combination
of
macroscopic balances and dimensional considerations is often used and can be quite useful.
57.7
USE OF THE MACROSCOPIC BALANCES
FOR UNSTEADY-STATE PROBLEMS
In the preceding section we have illustrated the use of the macroscopic balances for solv-
ing steady-state problems. In this section we turn our attention to unsteady-state prob-
lems. We give two examples to illustrate the use of the time-dependent macroscopic
balance equations.
G.
L.
Tuve and
R.
E.
Sprenkle,
Instruments,
6,202-205,225,232-234 (1935);
see also
R.
H.
Perry and
C. H. Chilton,
Chemical Engineers' Handbook,
McGraw-Hill, New York,
5th
edition
(1973),
Fig.
5-18;
Fluid
Meters: Their Theo
y
and Applications,
6th edition, American Society of Mechanical Engineers, New York
(1971),
pp.
58-65;
Measurement of Fluid Flow Using Small Bore Precision Orifice Meters, American
Society
of
Mechanical Engineers,
MFC-14-M,
New York
(1995).
