Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

Chapter

Interphase Transport

Isothermal

Systems

6.1 Definition of friction factors

56.2

Friction factors for flow in tubes

56.3

Friction factors for flow around spheres

56.4'

Friction factors for packed columns

In Chapters

2-4

we showed how laminar flow problems may be formulated and solved.

In Chapter

we presented some methods for solving turbulent flow problems by dimen-

sional arguments or by semiempirical relations between the momentum flux and the

gradient of the time-smoothed velocity. In this chapter we show how flow problems can

be solved by a combination of dimensional analysis and experimental data. The tech-

nique presented here has been widely used in chemical, mechanical, aeronautical, and

civil engineering, and it is useful for solving many practical problems. It is a topic worth

learning well.

Many engineering flow problems fall into one of two broad categories: flow in chan-

nels and flow around submerged objects. Examples of channel flow are the pumping of

oil through pipes, the flow of water

open channels, and extrusion of plastics through

dies. Examples of flow around submerged objects are the motion of air around an air-

plane wing, motion of fluid around particles undergoing sedimentation, and flow across

tube banks in heat exchangers.

In channel flow the main object is usually to get a relationship between the vol-

ume rate of flow and the pressure drop and/or elevation change. In problems involv-

ing flow around submerged objects the desired information is generally the relation

between the velocity of the approaching fluid and the drag force on the object. We

have seen in the preceding chapters that, if one knows the velocity and pressure dis-

tributions in the system, then the desired relationships for these two cases may be ob-

tained. The derivation of the Hagen-Poiseuille equation in

52.3

and the derivation of

the Stokes equation in

52.6

and

s4.2

illustrate the two categories we are discussing

here.

For many systems the velocity and pressure profiles cannot be easily calculated, par-

ticularly if the flow is turbulent or the geometry is complicated. One such system is the

flow through a packed column; another is the flow in a tube in the shape of a helical coil.

For such systems we can take carefully chosen experimental data and then construct

"correlations" of dimensionless variables that can be used to estimate the flow behavior

in geometrically similar systems. This method is based on

53.7.

178

Chapter

Interphase Transport

Isothermal Systems

We start in 56.1 by defining the "friction factor," and then we show in 556.2 and

6.3

how to construct friction factor charts for flow in circular tubes and flow around spheres.

These are both systems we have already studied and, in fact, several results from earlier

chapters are included in these charts. Finally in 56.4 we examine the flow in packed

columns, to illustrate the treatment of a geometrically complicated system. The more

complex problem of fluidized beds is not included in this chapter.'

6.1

DEFINITION

FRICTION FACTORS

We consider the steadily driven flow of a fluid of constant density in one of two systems:

(a) the fluid flows in a straight conduit of uniform cross section;

(b)

the fluid flows

around a submerged object that has an axis of symmetry (or two planes of symmetry)

parallel to the direction of the approaching fluid. There will be a force F+, exerted by the

fluid on the solid surfaces. It is convenient to split this force into two parts: F,, the force

that would be exerted

the fluid even if it were stationary; and Fk, the additional force

associated with the motion of the fluid (see 52.6 for the discussion of F, and Fk for flow

around spheres). In systems of type

(a),

Fk points in the same direction as the average ve-

locity

(v)

in the conduit, and in systems of type

(b),

Fk points in the same direction as the

approach velocity

v,.

For both types of systems we state that the magnitude of the force Fk is proportional

to a characteristic area

and a characteristic kinetic energy

per unit volume; thus

AKf

(6.1-1)'

in which the proportionality constant

is called the friction factor. Note that

Eq.

6.1-1 is

not a law of fluid dynamics, but only a definition for

This is a useful definition, because

the dimensionless quantity

can be given as a relatively simple function of the Reynolds

number and the system shape.

Clearly, for any given flow system, f is not defined until

and

are specified. Let

now see what the customary definitions are:

(a)

For flow in conduits,

is usually taken to be the wetted surface, and

is taken to

&v)~.

Specifically, for circular tubes of radius

and length

we define

Generally, the quantity measured is not Fk, but rather the pressure difference

and

the elevation difference

hL.

force balance on the fluid between

and

in the direc-

tion of flow gives for fully developed flow

Elimination of Fk between the last two equations then gives

Jackson,

The

Dynamics of Fluidized

Beds,

Cambridge University Press (2000).

For systems lacking symmetry, the

fluid

exerts both a force and a torque on the solid. For

discussions of such systems see

Happel and

Brenner,

Low Reynolds Number Hydrodynamics,

Martinus

Nijhoff, The Hague (1983), Chapter

H. Brenner, in

Adv.

Chem. Engr.,

6,287438

(1966);

Kim

and

Karrila,

Microhydrodynarnics: Principles and Selected Applications,

Butterworth-Heinemann, Boston

(1991), Chapter

Cj6.2

Friction Factors for

Flow

in Tubes

179

in which

is the tube diameter. Equation 6.1-4 shows how to calculate

from ex-

perimental data. The quantity f is sometimes called the

Fanning friction f~ctor.~

(b)

For

flow around submerged objects,

the characteristic area

is usually taken to be

the area obtained by projecting the solid onto a plane perpendicular to the velocity of the

approaching fluid; the quantity

is taken to be

ipv:,

where

is the approach velocity

of the fluid at a large distance from the object. For example, for flow around a sphere of

radius R, we define f by the equation

If it is not possible to measure

then we can measure the terminal velocity of the

sphere when it falls through the fluid (in that case,

has to be interpreted as the termi-

nal velocity of the sphere). For the steady-state fall of a sphere in a fluid, the force

just counterbalanced by the gravitational force on the sphere less the buoyant force (cf.

Eq. 2.6-14):

Elimination of

between Eqs. 6.1-5 and 6.1-6 then gives

This expression can be used to obtain f from terminal velocity data. The friction factor

used in

Eqs.

6.1-5 and

is sometimes called the

drag coefficient

and given the symbol

c,.

We have seen that the "drag coefficient" for submerged objects and the "friction fac-

tor" for channel flow are defined in the same general way. For this reason we prefer to

use the same symbol and name for both of them.

86.2

FRICTION FACTORS FOR FLOW IN

TUBES

We now combine the definition off in

Eq.

6.1-2 with the dimensional analysis of

53.7

show what

must depend on in this kind of system. We consider a "test section" of inner

radius

and length

shown in Fig. 6.2-1, carrying

fluid of constant density and vis-

cosity at a steady mass flow rate. The pressures

and

at the ends of the test section

are known.

This friction factor definition is due to

Fanning,

Practical Treatise on Hydraulic and Watu

Supply Engineering,

Van Nostrand, New York, 1st edition (1877), 16th edition (1906); the name "Fanning"

used to avoid confusion with the "Moody friction factor," which is larger by a factor of 4 than the

used here

[L.

Moody,

Trans.

ASME,

66,671-684 (19441.

If we use the "friction velocity"

d(9,

YL)R/2Lp,

introduced in s5.3, then

Eq.

6.1-4

assumes the form

John

Thomas Fanning

(1837-1911) studied architectural and civil engineering, served as an officer in the

Civil War, and after the war became prominent in hydraulic engineering. The 14th edition of his book

Practical Treatise on Hydraulic and Water-Supply Engineering

appeared in 1899.

For the translational motion of

sphere in three dimensions, one can write

approximately

where

unit vector in the direction of

v,.

See Problem 6C.1.

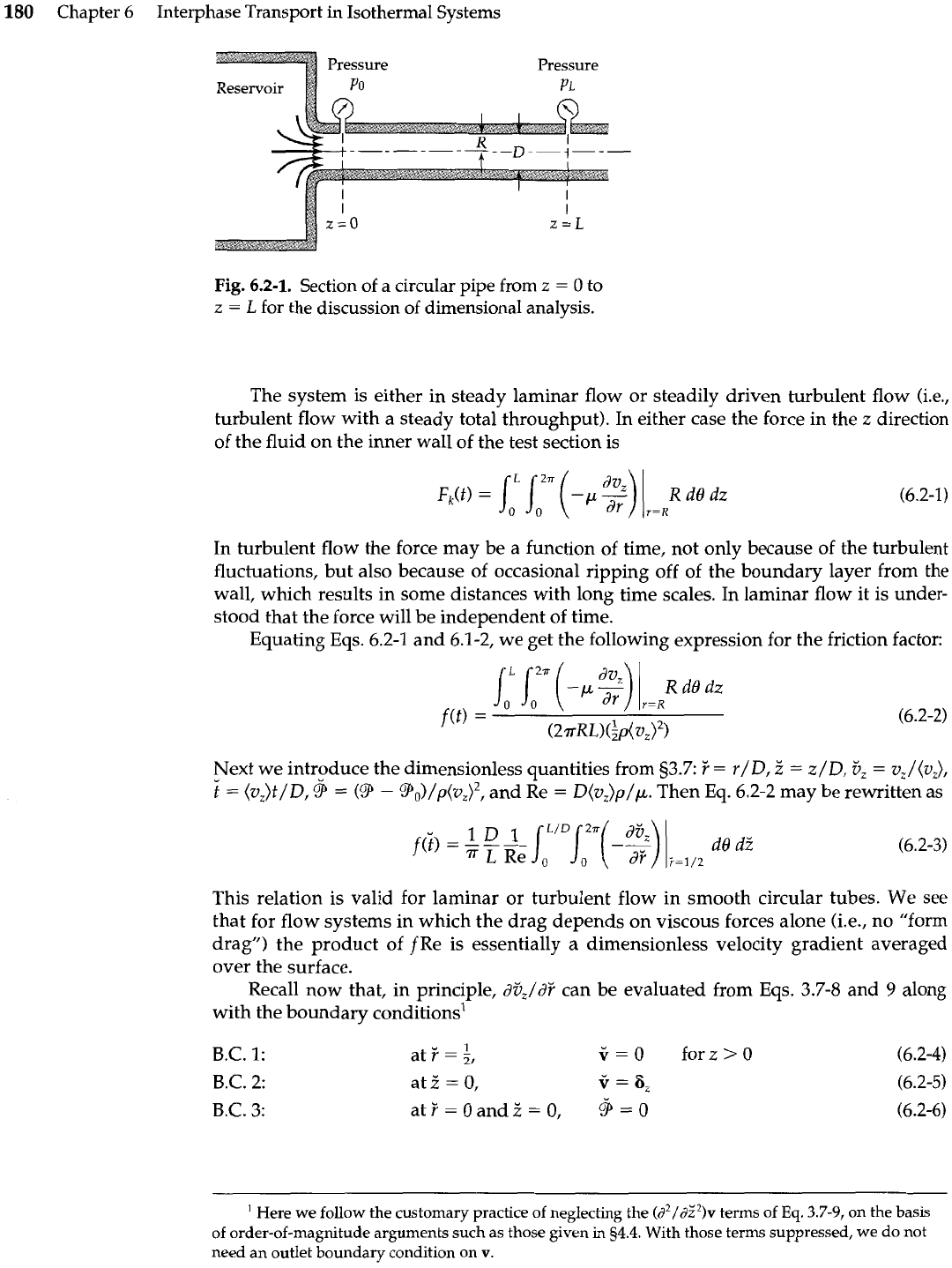
180

Chapter

Interphase Transport in Isothermal Systems

Pressure

Fig.

6.2-1.

Section of a circular pipe from

for the discussion of dimensional analysis.

The system is either in steady laminar flow or steadily driven turbulent flow (i.e.,

turbulent flow with a steady total throughput). In either case the force in the

direction

of the fluid on the inner wall of the test section is

In turbulent flow the force may be a function of time, not only because of the turbulent

fluctuations, but also because of occasional ripping off of the boundary layer from the

wall, which results in some distances with long time scales. In laminar flow it is under-

stood that the force will be independent of time.

Equating Eqs. 6.2-1 and 6.1-2, we get the following expression for the friction factor:

Next we introduce the dimensionless quantities from s3.7:

r/D,

z/D,

ijz

vZ/(v,),

(v,)t/D,

9,)/p(v,)2, and Re

D(v,)p/p. Then Eq. 6.2-2 may be rewritten as

This relation is valid for laminar or turbulent flow in smooth circular tubes.

see

that for flow systems in which the drag depends on viscous forces alone (i.e., no "form

drag") the product of fRe is essentially

dimensionless velocity gradient averaged

over the surface.

Recall now that, in principle,

dCZ/di

can be evaluated from Eqs. 3.7-8 and

along

with the boundary conditions1

B.C.

B.C. 2:

B.C.

Here we follow the customary practice of neglecting the

(d2/di2)v

terms of

Eq.

3.7-9,

on the basis

of order-of-magnitude arguments such as those given in

s4.4.

With those terms suppressed, we do not

need an outlet boundary condition on

s6.2

Friction Factors for Flow in Tubes

181

and appropriate initial conditions. The uniform inlet velocity profile in Eq. 6.2-5 is accu-

rate except very near the wall, for a well-designed nozzle and upstream system. If Eqs.

3.7-8 and 9 could be solved with these boundary and initial conditions to get

and

the

solutions would necessarily be of the form

+(?,

t; Re)

(?,

Re)

That is, the functional dependence of

and

must, in general, include all the dimen-

sionless variables and the one dimensionless group appearing in the differential equa-

tions. No additional dimensionless groups enter via the preceding boundary conditions.

As a consequence,

&?Jd?

must likewise depend on

and Re. When

deZ/d?

is eval-

uated at

and then integrated over

and

in Eq. 6.2-3, the result depends only on

Re, and LID (the latter appearing in the upper limit in the integration over

5).

Therefore

we are led to the conclusion that

f($

(Re,

L/D,

i),

which, when time averaged, becomes

f (Re, L/D)

(6.2-9)

when the time average is performed over an interval long enough to include any long-

time turbulent disturbances. The measured friction factor then depends only on the

Reynolds number and the length-to-diameter ratio.

The dependence off on LID arises from the development of the time-average veloc-

ity distribution from its flat entry shape toward more rounded profiles at downstream

values. This development occurs within an entrance region, of length

0.030 Re for

laminar flow or

60D for turbulent flow, beyond which the shape of the velocity dis-

tribution is "fully developed." In the transportation of fluids, the entrance length is usu-

ally a small fraction of the total; then Eq. 6.2-9 reduces to the long-tube form

and

can be evaluated experimentally from Eq. 6.1-4, which was written for fully devel-

oped flow at the inlet and outlet.

Equations 6.2-9 and 10 are useful results, since they provide a guide for the system-

atic presentation of data on flow rate versus pressure difference for laminar and turbu-

lent flow in circular tubes. For long tubes we need only a single curve off plotted versus

the single combination D(Qp/p. Think how much simpler this is than plotting pressure

drop versus the flow rate for separate values of

L, p, and p, which is what the uniniti-

ated might do.

There is much experimental information for pressure drop versus flow rate

tubes,

and hence

can be calculated from the experimental data by Eq. 6.1-4. Then f can be plot-

ted versus Re for smooth tubes to obtain the solid curves shown in Fig. 6.2-2. These solid

curves describe the laminar and turbulent behavior for fluids flowing in long, smooth, cir-

cular tubes.

Note that the laminar curve on the friction factor chart is merely a plot of the

Hagen-Poiseuille equation in Eq. 2.3-21. This can be seen by substituting the expression

for

(9,

9,)

from Eq. 2.3-21 into Eq. 6.1-4 and using the relation

p(&)~R2; this gives

16 Re

2100 stable

f=-{

Re Re

2100 usually unstable

in which Re

D(&)p/p; this is exactly the laminar line in Fig. 6.2-2.

Analogous turbulent curves have been constructed by using experimental data. Some

analytical curve-fit expressions are also available. For example, Eq. 5.1-6 can be put into

the form

0'0791

2.1

lo3

lo5

f=~e'/'

(6.2-12)

182

Chapter

Interphase Transport in Isothermal Systems

0.5

0.2

0.1

0.05

0.02

cL'

0.01

0.005

0.002

0.001

lo2

103

lo4

lo5

lo7

Reynolds number

D<F>

p/p

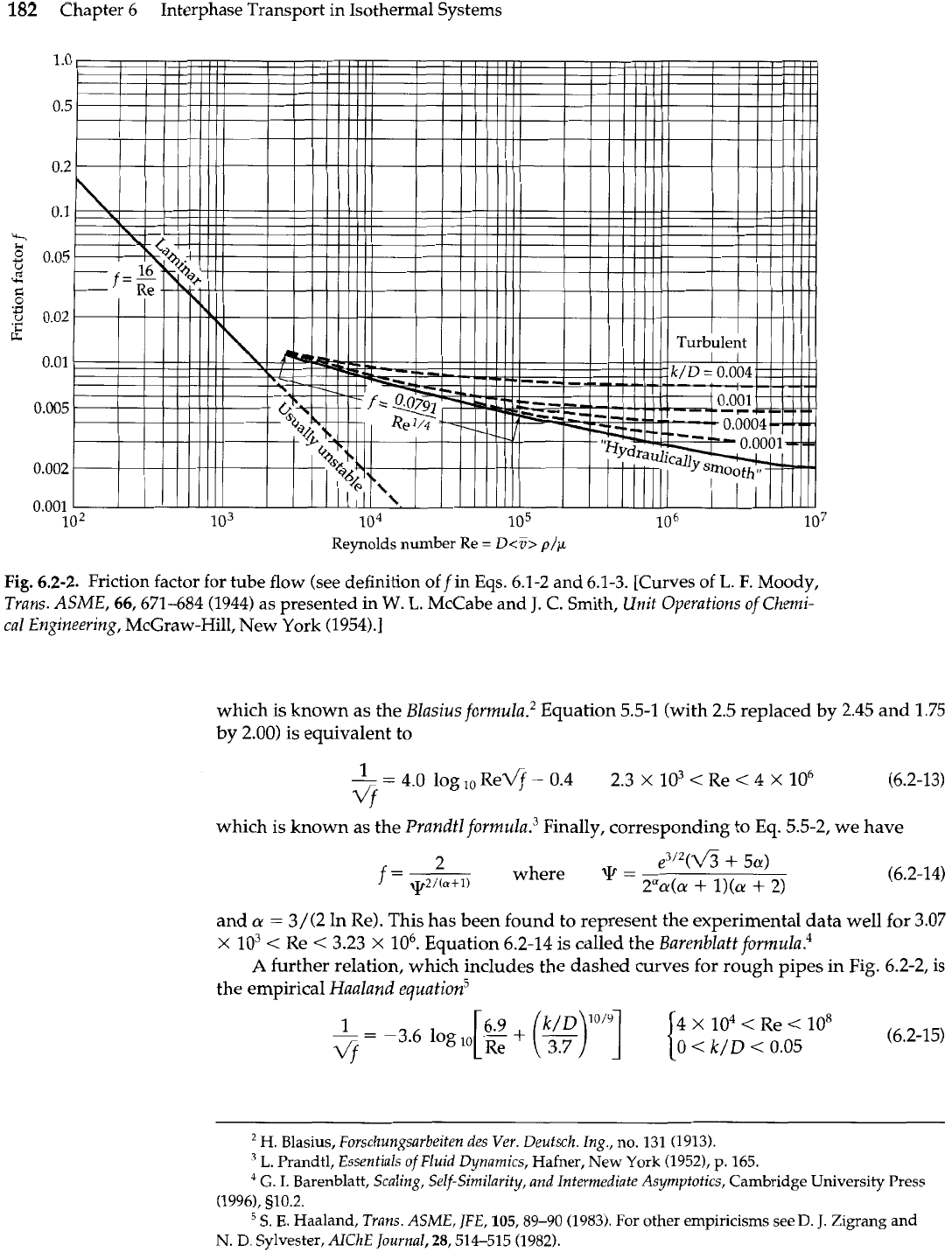
Fig.

6.2-2.

Friction factor for tube flow (see definition off in Eqs. 6.1-2 and 6.1-3. [Curves of

Moody,

Trans.

ASME,

66,671-684 (1944) as presented in

McCabe and

C. Smith,

Unit Operations of Chmi-

cal

Engineering,

McGraw-Hill, New York (1954).]

which is known as the

Blasius forrn~la.~

Equation 5.5-1 (with 2.5 replaced by 2.45 and 1.75

by 2.00) is equivalent to

4.0 log

~eq

0.4

2.3

lo3

10"

(6.2-13)

which is known as the

Prandtl forrn~la.~

Finally, corresponding to Eq. 5.5-2, we have

where

e312(fi

5a)

f=v

2"(u(a

l)(a

and

3/(2 In Re). This has been found to represent the experimental data well for 3.07

lo3

3.23

lo6.

Equation 6.2-14 is called the

Barenblatt

f~rmula.~

further relation, which includes the dashed curves for rough pipes in Fig. 6.2-2,

the empirical

Haaland

equation5

Blasius,

Forschungsarbeiten des Ver. Deutsch. Ing.,

no.

131

(1913).

Prandtl,

Essentials of Fluid Dynamics,

Hafner, New York

(19521,

165.

Barenblatt,

Scaling, Self-Similarity, and Intermediate Asymptofics,

Cambridge University Press

(1996), s10.2.

Haaland,

Trans. ASME, JFE,

105,89-90 (1983).

For other empiricisms see

Zigrang and

Sylvester,

AKhE Journal,

28,514-515 (1982).

s6.2 Friction Factors for Flow in Tubes

183

This equation is stated5 to be accurate within 1.5%. As can be seen in Fig. 6.2-2, the fric-

tional resistance to flow increases with the height,

of the protuberances. Of course,

has to enter into the correlation

a dimensionless fashion and hence appears via the

ratio k/D.

For

turbulent flow

noncircular tubes

it is common to use the following empiricism:

First we define a "mean hydraulic radius"

as follows:

in which

is the cross section of the conduit and

is the wetted perimeter. Then we can

use

Eq.

6.1-4 and Fig. 6.2-2, with the diameter

of the circular pipe replaced by

4Rh.

That

is, we calculate pressure differences by replacing

Eq.

6.1-4 by

and getting

from Fig. 6.2-2 with a Reynolds number defined as

For laminar flows in noncircular passages, this method is less satisfactory.

EXAMPLE

6.2-1

What pressure gradient is required to cause diethylaniline, C,H,N(C,H,),, to flow in a

horizontal, smooth, circular tube of inside diameter

3 cm at a mass rate of 1028 g/s at

Pressure

20°C? At this temperature the density of diethylaniline is p

0.935 g/cm3 and its viscosity is

for

Given Flow

Rate

1.95 cp.

SOLUTION

The Reynolds number for the flow is

From Fig. 6.2-2, we find that for this Reynolds number the friction factor

has a value of

0.0063 for smooth tubes. Hence the pressure gradient required to maintain the flow is (ac-

cording to

Eq.

6.1-4)

Determine the flow rate, in pounds per hour, of water at

68OF

through a 1000-ft length of hori-

zontal 8-in. schedule

steel pipe (internal diameter 7.981

in.)

under a pressure difference of

Rate

for

Given

3.00

psi. For such a pipe use Fig. 6.2-2 and assume that

k/D

2.3

Pressure Drop

SOLUTION

We want to use Eq. 6.1-4 and Fig. 6.2-2 to solve for (v,) when

is known. However, the

quantity

(v,)

appears explicitly on the left side of the equation and implicitly on the right side

which depends on Re

D(v,)p/p. Clearly a trial-and-error solution can be found.

184

Chapter 6 Interphase Transport in Isothermal Systems

However, if one has to make more than a few calculations of

(u,),

it is advantageous to

de-

velop a systematic approach; we suggest two methods here. Because experimental data are

often presented in graphical form, it is important for engineering students to use their origi-

nality in devising special methods such as those described here.

Method

Figure 6.2-2 may be used to construct a plot6 of Re versus the group Re*,

which does not contain

(u,):

The quantity Re* can be computed for this problem, and a value of the Reynolds number

can be read from the Re versus Re* plot. From Re the average velocity and flow rate can

then be calculated.

Method

Figure 6.2-2 may also be used directly without any replotting, by devising

scheme that is equivalent to the graphical solution of two simultaneous equations. The two

equations are

(Re, k/D)

curve given in Fig. 6.2-2 (6.2-22)

The procedure is then to compute ~e* according to Eq. 6.2-21 and then to plot Eq. 6.2-23 on

the log-log plot off versus Re in Fig. 6.2-2. The intersection point gives the Reynolds number

of the flow, from which

(E,)

can then be computed.

For the problem at hand, we have

Then according to Eq. 6.2-21,

1.63

lo4

(dimensionless) (6.2-24)

The line of Eq. 6.2-23 for this value of Re* passes through

1.0 at Re

1.63

lo4

and

through

0.01 at Re

1.63

10".

Extension of the straight line through these points to the

curve of Fig. 6.2-2 for

k/D

0.00023 gives the solution to the two simultaneous equations:

Solving for

then gives

(a/4)Dp Re

(0.7854)(0.665)(6.93

10-4)(36~~)(2.4

lo5)

3.12

105 lb,/hr

39 kg/s

related plot was proposed

von K&rm&n,

Nackr.

Ges.

Wiss.

Gottingen,

Fachgruppen,

5,5&76

(1930).

56.3

Friction Factors for Flow around Spheres

185

56.3

FRICTION FACTORS

FOR

FLOW AROUND SPHERES

In this section we use the definition of the friction factor in Eq. 6.1-5 along with the di-

mensional analysis of 53.7 to determine the behavior off for a stationary sphere in an in-

finite stream of fluid approaching with a uniform, steady velocity

v,.

We have already

studied the flow around a sphere in s2.6 and 54.2 for Re

0.1 (the "creeping flow" re-

gion). At Reynolds numbers above about

there is a significant unsteady eddy motion

in the wake of the sphere. Therefore, it will be necessary to do a time average over a time

interval long with respect to this eddy motion.

Recall from 92.6 that the total force acting in the

direction on the sphere can be

written as the sum of a contribution from the normal stresses (F,) and one from the tan-

gential stresses (F,). One part of the normal-stress contribution is the force that would be

present even if the fluid were stationary,

F,.

Thus the "kinetic force," associated with the

fluid motion, is

(F,

Fform

Ffriction

(6.3-1)

The forces associated with the form drag and the friction drag are then obtained from

Fform(t)

12=

(-91

r=R

cos

B)R2

sin

dB d+

0 0

Since

is zero everywhere on the sphere surface, the term containing

dv,/dB

is zero.

If now we split f into two parts as follows

fform

ffriction

then, from the definition in

Eq.

6.1-5, we get

fform(i)

1'"

(-@

cos

sin

do d+

?To

The friction factor is expressed here in terms of dimensionless variables

and a Reynolds number defined as

To evaluate f

(i)

one would have to know

and 5, as functions of

and

We know that for incompressible flow these distributions can

principle

be ob-

tained from the solution of Eqs. 3.7-8 and

along with the boundary conditions

B.C. 1:

B.C.

atY=l, 5,=O and Ee=O

at?=

co,

5,=1

atY=m, @=O

and some appropriate initial condition on ir. Because no additional dimensionless

groups enter via the boundary and initial conditions, we know that the dimensionless

pressure and velocity profiles will have the following form:

~(i,

HI+,

Re)

+(?,

Re)

(6.3-12)

186

Chapter 6 Interphase Transport in Isothermal Systems

When these expressions are substituted into Eqs. 6.3-5 and 6, it is then evident that the

friction factor in Eq. 6.3-4 must have the form f(i)

f(Re,

i),

which, when time aver-

aged over the turbulent fluctuations, simplifies to

(Re) (6.3-13)

by using arguments similar to those in 56.2. Hence from the definition of the friction fac-

tor and the dimensionless form of the equations of change and the boundary conditions,

we find that

must be a function of Re alone.

Many experimental measurements of the drag force on spheres are available, and

when these are plotted

dimensionless form, Fig. 6.3-1 results. For this system there

no sharp transition from an unstable laminar flow curve to a stable turbulent flow curve

as for long tubes at a Reynolds number of about 2100 (see Fig. 6.2-2). Instead, as the ap-

proach velocity increases, f varies smoothly and moderately up to Reynolds numbers

the order of lo5. The kink in the curve at about Re

lo5

is associated with the shift of

the boundary layer separation zone from in front of the equator to in back of the equator

of the sphere.'

We have juxtaposed the discussions of tube flow and flow around a sphere to

em-

phasize the fact that various flow systems behave quite differently. Several points of dif-

ference between the two systems are:

Flow

Tubes

Rather well defined laminar-turbulent

transition at about Re

2100

The only contribution to

is the friction

drag (if the tubes are smooth)

No boundary layer separation

Flow

Around Spheres

No well defined laminar-turbulent

transition

Contributions to

from both friction

and form drag

There is a kink in the

vs. Re curve

associated with a shift in the separation

zone

The general shape of the curves in Figs. 6.2-2 and 6.3-1 should be carefully remembered.

For the creeping flow region, we already know that the drag force is given by

Stokes'

law,

which is a consequence of solving the continuity equation and the Navier-Stokes

equation of motion without the

pDv/Dt

term. Stokes' law can be rearranged into the

form of Eq. 6.1-5 to get

Hence for creeping flow around a sphere

f=-

for Re

0.1

and this is the straight-line asymptote as Re

0 of the friction factor curve in Fig. 6.3-1.

For higher values of the Reynolds number, Eq. 4.2-21 can describe

accurately up

about Re

1. However, the empirical expression'

0.5409

for Re

6000

Adair,

The Physics

Baseball,

Harper and

Row,

New

York

(1990).

Abraham,

Physics

Fluids,

13,2194 (1970);

Van

Dyke,

Physics

Fluids,

14,103&1039 (1971).