Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

55.2

Time-Smoothed Equations of Change for Incompressible Fluids

157

Time

Fig.

5.2-1.

Sketch showing the velocity component

as well as its time-smoothed value

and its fluctuation

in turbulent flow

(a)

for "steadily driven turbulent flow" in which

does not depend on time, and

(b)

for a

situation in which

does depend on time.

the period to being long enough to give a smooth averaged function. For the system at

hand, the quantity

which we call the time-smoothed velocity, is independent of time,

but of course depends on position. When the time-smoothed velocity does not depend

on time, we speak of steadily driven turbulent pow. The same comments we have made for

velocity can also be made for pressure.

Next we consider turbulent flow

a tube with a time-dependent pressure gradient.

For such a flow one can define time-smoothed quantities as above, but one has to under-

stand that the period

must be small with respect to the changes in the pressure gradi-

ent, but still large with respect to the periods of fluctuations. For such a situation the

time-smoothed velocity and the actual velocity are illustrated in Fig. 5.2-l(b).'

According to the definition in Eq. 5.2-2, it is easy to verify that the following rela-

tions are true:

The quantity

will not, however, be zero, and in fact the ratio

-/(8,)

can be taken to

be a measure of the magnitude of the turbulent fluctuations. This quantity, known as the

intensity of turbulence, may have values from 1 to 10% in the main part of a turbulent

stream and values of 25% or higher in the neighborhood of a solid wall. Hence, it must be

emphasized that we are not necessarily dealing with tiny disturbances; sometimes the fluc-

tuations are actually quite violent and large.

Quantities such as v,:.vi are also nonzero. The reason for this is that the local motions

in the

and y directions are correlated. In other words, the fluctuations in the

direction

are not independent of the fluctuations in the y direction. We shall see presently that

these time-smoothed values of the products of fluctuating properties have an important

role in turbulent momentum transfer. Later we shall find similar correlations arising in

turbulent heat and mass transport.

One can also define the "overbar" quantities in terms of an "ensemble average." For most

purposes the results are equivalent or are assumed to be

so.

See, for example,

Townsend,

The

Structure

Turbulent Shear

Flow,

Cambridge University Press, 2nd edition

(1976).

See also

Kundu,

Fluid

Mechanics,

Academic Press, New York

(1990),

p. 421, regarding the last of the formulas given in

Eq.

5.2-3.

158

Chapter

Velocity Distributions in Turbulent Flow

Having defined the time-smoothed quantities and discussed some of the properties

of the fluctuating quantities, we can now move on to the time-smoothing of the equations

of change. To keep the development as simple as possible, we consider here only the

equations for a fluid of constant density and viscosity. We start by writing the equations

of continuity and motion with

replaced by its equivalent

and p by its equivalent

p'. The equation of continuity is then (V

0, and we write the x-component of the

equation of motion, Eq. 3.5-6, in the d/dt form by using Eq. 3.5-5:

p(vx

v;)

p')

p(v,

v:)(v,

v:)

P(V,

vpv,

v:)

t dx

The y- and z-components of the equation of motion can be similarly written. We next

time-smooth these equations, making use of the relations given in Eq. 5.2-3. This gives

pV2E,

pg, (5.2-7)

with similar relations for the y- and z-components of the equation of motion. These are

then the time-smoothed equations of continuity and motion for a fluid with constant density

and viscosity. By comparing them with the corresponding equations in Eq. 3.1-5 and

Eq. 3.5-6 (the latter rewritten in terms of d/dt), we conclude that

The equation of continuity is the same as we had previously, except that

is now

replaced by

The equation of motion now has

and

where we previously had

and

ad-

dition there appear the dashed-underlined terms, which describe the momentum

transport associated with the turbulent fluctuations.

We may rewrite Eq. 5.2-7 by introducing the turbulent momentum flux tensor

7'')

with

components

7;:)

pvxvx

pvxvy

7:;)

and so on (5.2-8)

These quantities are usually referred to as the Reynolds stresses. We may also introduce

symbol

for the time-smoothed viscous momentum flux. The components of this ten-

sor have the same appearance as the expressions given in Appendices B.l to

8.3,

except

that the time-smoothed velocity components appear in them:

This enables us then to write the equations of change in vector-tensor form as

and (V

v')

0 (5.2-10,ll)

s5.3

The Time-Smoothed Velocity Profile near a Wall

159

Equation 5.2-11 is an extra equation obtained by subtracting Eq. 5.2-10 from the original

equation of continuity.

The principal result of this section is that the equation of motion in terms of the

stress tensor, summarized in Appendix Table B.5, can be adapted for time-smoothed tur-

bulent flow by changing all

and

as well as

Tij

7:'

7;'

in any of the

coordinate systems given.

We have now arrived at the main stumbling block in the theory of turbulence.

The Reynolds stresses

7;'

above are not related to the velocity gradients in a simple

way as are the time-smoothed viscous stresses

in Eq.

5.2-9.

They are, instead, com-

plicated functions of the position and the turbulence intensity. To solve flow prob-

lems we must have experimental information about the Reynolds stresses or else

resort to some empirical expression. In 55.4 we discuss some of the empiricisms that

are available.

Actually one can also obtain equations of change for the Reynolds stresses (see Prob-

111

lem 5D.1). However, these equations contain quantities like vivj vk. Similarly, the equa-

tions of change for the vivjvk contain the next higher-order correlation v,!v!v;v;, and so

on. That is, there is a never-ending hierarchy of equations that must be solved. To solve

flow problems one has to "truncate" this hierarchy by introducing empiricisms. If we

use empiricisms for the Reynolds stresses, we then have

"first-order" theory. If we in-

troduce empiricisms for the vivjv,, we then have a "second-order theory," and so on.

The problem of introducing empiricisms to get a closed set of equations that can be

solved for the velocity and pressure distributions is referred to as the "closure problem."

The discussion in 55.4 deals with closure at the first order. At the second order the

"k-E

empiricism" has been extensively studied and widely used in computational fluid

mechanics.'

55.3

THE TIME-SMOOTHED VELOCITY PROFILE NEAR A WALL

Before we discuss the various empirical expressions used for the Reynolds stresses, we

present here several developments that do not depend on any empiricisms. We are con-

cerned here with the fully developed, time-smoothed velocity distribution in the neigh-

borhood of a wall. We discuss several results: a Taylor expansion of the velocity near the

wall, and the universal logarithmic and power law velocity distributions a little further

out from the wall.

The flow near a flat surface is depicted in Fig. 5.3-1. It

convenient to distinguish

four regions of flow:

the viscous sublayer very near the wall, in which viscosity plays a key role

the buffer layer in which the transition occurs between the viscous and inertial

sublayers

the inertial sublayer at the beginning of the main turbulent stream, in which viscos-

ity plays at most a minor role

the main turbulent stream, in which the time-smoothed velocity distribution is

nearly flat and viscosity is unimportant

It must be emphasized that this classification into regions is somewhat arbitrary.

Lumley,

Adv. Appl.

Mech.,

18,123-176 (1978);

Speziale,

Ann.

Revs.

Fluid

Mech.,

23,

107-157 (1991);

Schlichting and

Gersten,

Bounda

y-Layer

Theoy,

Springer, Berlin, 8th edition (2000),

pp. 560-563.

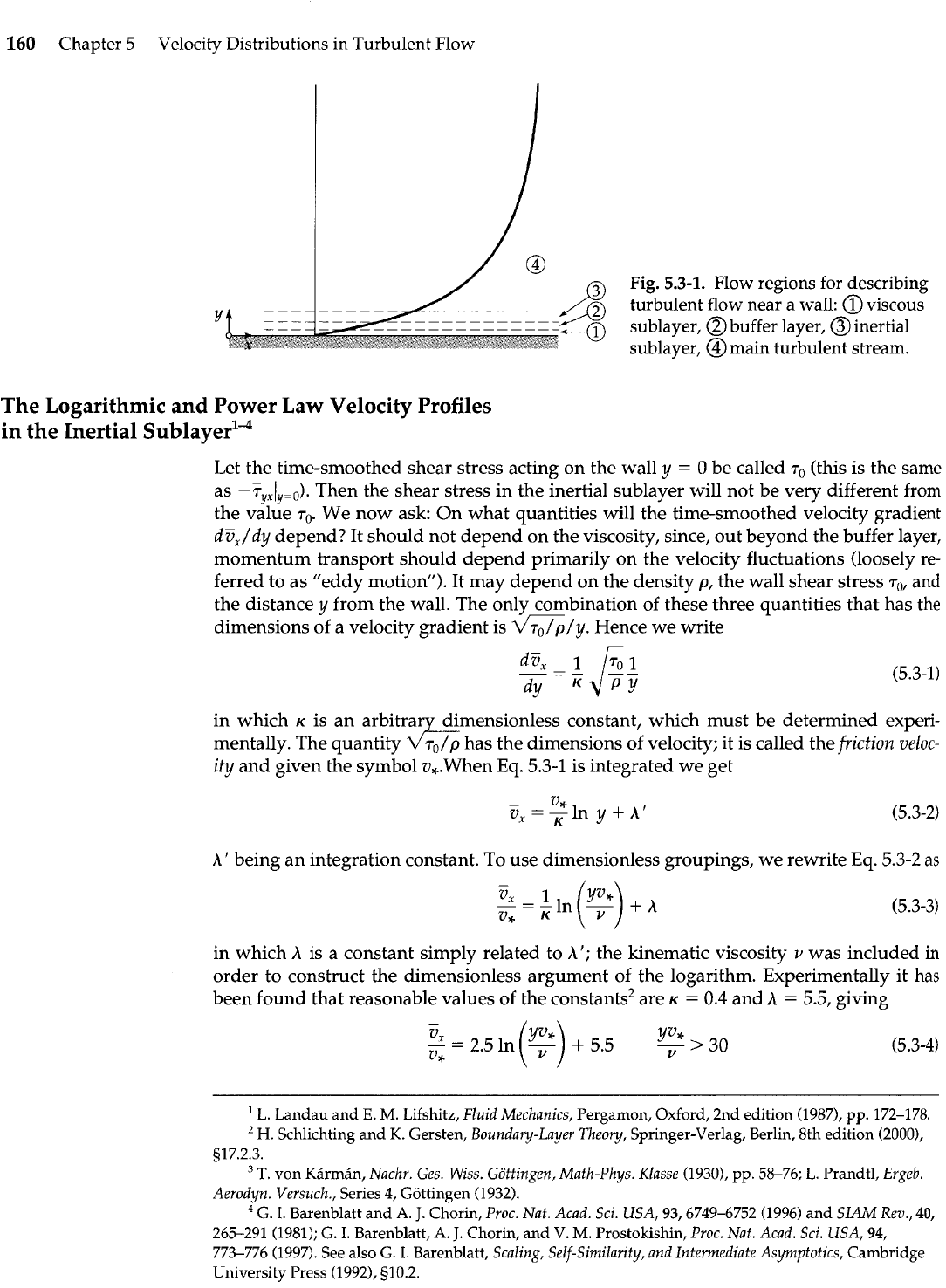
160

Chapter

Velocity Distributions in Turbulent Flow

Fig.

5.3-1.

Flow regions for describing

turbulent flow near a wall: @viscous

sublayer, @buffer layer, @inertial

sublayer,

main turbulent stream.

The Logarithmic and Power Law Velocity Profiles

in the Inertial Sublayer14

Let the time-smoothed shear stress acting on the wall

be called

(this is the same

-7y.u)y=0).

Then the shear stress in the inertial sublayer will not be very different from

the value

7,.

We now ask: On what quantities will the time-smoothed velocity gradient

dv,/dy

depend? It should not depend on the viscosity, since, out beyond the buffer layer,

momentum transport should depend primarily on the velocity fluctuations (loosely re-

ferred to as "eddy motion"). It may depend on the density

the wall shear stress

T,,,

and

the distance

from the wall. The only combination of these three quantities that has the

dimensions of a velocity gradient is

-ly.

Hence we write

in which

is an arbitra

dimensionless constant, which must be determined experi-

mentally. The quantity

3--

~,/p

has the dimensions of velocity; it is called the

friction

veloc-

ity

and given the symbol v,.When Eq. 5.3-1 is integrated we get

being an integration constant. To use dimensionless groupings, we rewrite Eq. 5.3-2

in which

is a constant simply related to

A';

the kinematic viscosity

was included

order to construct the dimensionless argument of the logarithm. Experimentally it

has

been found that reasonable values of the constants2 are

0.4

and

5.5, giving

L. Landau and

Lifshitz,

Fluid Mechanics,

Pergamon, Oxford, 2nd edition (19871, pp. 172-178.

Schlichting and

Gersten,

Boundary-Layer Theory,

Springer-Verlag, Berlin, 8th edition (2000),

g17.2.3.

von KBrmh,

Nachr. Ges. Wiss. Gottingen, Math-Phys. Klasse

(19301,

pp.

58-76;

Prandtl,

Ergeb.

Aerodyn. Versuch.,

Series

Gottingen (1932).

Barenblatt and

Chorin,

Proc. Nat. Acad. Sci. USA,

93,6749-6752 (1996) and

SIAM Rev.,

40,

265-291 (1981); G.

Barenblatt,

Chorin, and V.

Prostokishin,

Proc. Nat. Acad. Sci. USA,

94,

773-776 (1997). See also G.

Barenblatt,

Scaling, Self-Similarity, and Intermediate Asymptotics,

Cambridge

University Press (1992), 510.2.

55.3

The Time-Smoothed Velocity Profile near a Wall

161

This is called the von Urmlin-Prandtl universal logarithmic velocity di~tribution;~ it is intended

to apply only in the inertial sublayer. Later we shall see (in Fig. 5.5-3) that this function

describes moderately well the experimental data somewhat beyond the inertial sublayer.

If Eq. 5.3-1 were correct, then the constants

and

would indeed be "universal con-

stants," applicable at any Reynolds number. However, values of

in the range 0.40 to

0.44 and values of

in the range 5.0 to

6.3

can be found in the literature, depending on

the range of Reynolds numbers. This suggests that the right side of Eq. 5.3-1 should be

multiplied by some function of Reynolds number and that

could be raised to some

power involving the Reynolds number. Theoretical arguments have been advanced4 that

Eq. 5.3-1 should be replaced by

in which

ifi,

and

When Eq. 5.3-5 is integrated with respect to

the

Barenblatt-Chorin universal velocity distribution is obtained:

Equation 5.3-6 describes regions @and

@of

Fig. 5.3-1 better than does Eq. 5.3-4.4 Region

is better described by Eq. 5.3-13.

Taylor-Series Development in the Viscous Sublayer

We start by writing a Taylor series for

as a function of y, thus

To evaluate the terms in this series, we need the expression for the time-smoothed shear

stress in the vicinity of a wall. For the special case of the steadily driven flow in a slit of

thickness

2B,

the shear stress will be of the form

7,,

7::

7:;

~~[l

(y/B)I.

Then

from Eqs. 5.2-8 and

we have

Now we examine one by one the terms that appear in Eq. 5.3-7:5

(i)

The first term is zero by the no-slip condition.

(ii)

The coefficient of the second term can be obtained from Eq. 5.3-8, recognizing

that both v: and

are zero at the wall so that

(iii)

The coefficient of the third term involves the second derivative, which may be

obtained by differentiating Eq. 5.3-8 with respect to

and then setting

0, as follows,

(5.3-10)

y=O

since both

and

are zero at the wall.

A. A.

Townsend,

The Structure

Turbulent Shear

Flow,

Cambridge University Press, 2nd edition

(1976),

163.

162

Chapter

Velocity Distributions in Turbulent Flow

(iv)

The coefficient of the fourth term involves the third derivative, which may be

obtained from Eq. 5.3-8, and this is

Here Eq. 5.2-1

has been used.

There appears to be no reason to set the next coefficient equal to zero, so we find that

the Taylor series, in dimensionless quantities, has the form

The coefficient

has been obtained experimentally,' and therefore we have the final result:

The

term in the brackets will turn out to be very important in connection with turbu-

lent heat and mass transfer correlations in Chapters 13,14,21, and 22.

For the region

yvJv

30 no simple analytical derivations are available, and

empirical curve fits are sometimes used. One of these is shown in Fig. 5.5-3 for circular

tubes.

55.4

EMPIRICAL EXPRESSIONS FOR THE

TURBULENT MOMENTUM FLUX

now return to the problem of using the time-smoothed equations of change in Eqs.

5.2-11 and 12 to obtain the time-smoothed velocity and pressure distributions. As

pointed out in the previous section, some information about the velocity distribution can

be obtained without having a specific expression for the turbulent momentum flux

F"'.

However, it has been popular among engineers to use various empiricisms for

I"'

that

involve velocity gradients. We mention a few of these, and many more can be found in

the turbulence literature.

The Eddy Viscosity of Boussinesq

By analogy with Newton's law of viscosity, Eq. 1.1-1, one may write for a turbulent shear

flow1

Lin,

Moulton, and

L. Putnam,

Ind. Eng.

Chem.,

45,636-640 (1953); the numerical

coefficient was determined from mass transfer experiments in circular tubes. The importance of the

term in heat and mass transfer was recognized earlier by

Murphree,

Ind.

Eng.

Chem.,

24,726-736

(1932). Eger Vaughn Murphree (1898-1962) was captain of the University of Kentucky football team in

1920 and became President of the Standard Oil Development Company.

Boussinesq,

Mkm.

prks. par

diu.

savants

I'acad. sci,

Paris,

23,

#I, 1-680 (1877),24, #2,1-64 (1877).

Joseph Valentin Boussinesq (1842-19291, university professor in Lille, wrote a two-volume treatise on

heat, and is famous for the "Boussinesq approximation" and the idea of

"eddy

viscosity."

55.4

Empirical Expressions for the Turbulent Momentum Flux

163

in which

p"'

is the turbulent viscosity (often called the eddy viscosity, and given the

symbol

E).

As one can see from Table 5.1-1, for at least one of the flows given there,

the circular jet, one might expect

Eq.

5.4-1 to be useful. Usually, however,

p("

is a

strong function of position and the intensity of turbulence. In fact, for some systems2

p"'

may even be negative in some regions. It must be emphasized that the viscosity

is a property of the fluid, whereas the eddy viscosity

p"'

is primarily a property of

the flow.

For two kinds of turbulent flows (i.e., flows along surfaces and flows

jets and

wakes), special expressions for

p't'

are available:

(i)

Wall turbulence:

YV*

0<,<5

14.5~

This expression, derivable from Eq. 5.3-13, is valid only very near the wall. It is of con-

siderable importance in the theory of turbulent heat and mass transfer at fluid-solid

interface^.^

in which

is a dimensionless coefficient to be determined experimentally,

is the

width of the mixing zone at a downstream distance

and the quantity in parentheses

represents the maximum difference in the z-component of the time-smoothed veloci-

ties at that distance

Prandt14 found Eq. 5.4-3 to be a useful empiricism for jets and

wakes.

The

Mixing

Length

Prandtl

By assuming that eddies move around in a fluid very much as molecules move around

in a low-density gas (not a very good analogy) prandt15 developed an expression for mo-

mentum transfer in a turbulent fluid. The "mixing length"

plays roughly the same role

as the mean free path in kinetic theory (see 51.4). This kind of reasoning led Prandtl to

the following relation:

If the mixing length were a universal constant, Eq. 5.4-4 would be very attractive, but in

fact

has been found to be a function of position. Prandtl proposed the following expres-

sions for

(i)

Wall turbulence:

~,y

distance from wall)

(ii)

Free turbulence:

~,b

width of mixing zone)

in which

and

are constants.

result similar to Eq. 5.4-4 was obtained by Taylor6 by

his "vorticity transport theory" some years prior to Prandtl's proposal.

Hinze,

Appl.

Sci. Res.,

22,163-175

(1970);

Kruka

and

Eskinazi,

Fluid. Mech.,

20,555-579

(1964).

Lin,

Moulton,

and

Putnam,

Ind. Eng. Chem.,

45,636-640 (1953).

L. Prandtl,

Zeits.

angew. Math. u. Mech.,

22,241-243 (1942).

Prandtl,

Zeits.

angew. Math. u. Mech.,

5,136-139

(1925).

Taylor,

Phil. Trans.

A215,l-26

(1915),

and

Proc. Roy. Soc.

(London), A135,685-701 (1932).

164

Chapter 5 Velocity Distributions in Turbulent Flow

The

Modified van

Driest

Equation

There have been numerous attempts to devise empirical expressions that can describe

the turbulent shear stress all the way from the wall to the main turbulent stream. Here

we give a modification of the equation of van Drie~t.~ This is a formula for the mixing

length of Eq.

5.4-4:

This relation has been found to be useful for predicting heat and mass transfer rates in

flow in tubes.

In the next two sections and in several problems at the end of the chapter, we illus-

trate the use of the above empiricisms. Keep in mind that these expressions for the

Reynolds stresses are little more than crutches that can be used for the representation of

experimental data or for solving problems that fall into rather special classes.

Obtain an expression for

7:;

pv:vi

function of

in the neighborhood of the wall.

Development of the

SOLUTION

Reyno Ids Stress

Expression in the

(a)

start by making a Taylor series development of the three components of

v':

Vicinity of

the

Wall

The first term in Eqs. 5.4-8 and 10 must be zero because of the no-slip condition; the first term

in Eq.

5.4-9

is zero in the absence of mass transfer. Next we can write Eq. 5.2-11 at

The first and third terms in this equation are zero because of the no-slip condition. Therefore

we have to conclude that the second term is zero as well. Hence all the dashed-underlined

terms in Eqs. 5.4-8 to

are zero, and we may conclude that

$j?

PD:Z'j.

AY3

BY4

. .

(5.4-12)

This suggests-but does not proves-that the lead term

the Reynolds stress near a wall

should be proportional to

y3.

Extensive studies of mass transfer rates in closed channels9 have,

however, established that

R. van Driest,

Aero.

Sci.,

23,1007-1011 and 1036 (1956). Van Driest's original equation did

not have the square root divisor. This modification was made

T. Hanna,

Sandall, and

Mazet,

AIChE Journal,

27,693-697 (1981) so that the turbulent viscosity would be proportional to

in accordance with Eq. 5.4-2.

Reichardt,

Zeits.

angew.

Math.

u. Mech.,

31,20&219 (1951). See also

Hinze,

Turbulence,

McGraw-Hill, New York,

2nd

edition (1975),

pp.

620-621.

H. Notter and

C. A.

Sleicher,

Chem. Eng.

Sci.,

26,161-171 (1971);

Sandal1 and

T. Hanna,

AIChE

Journal,

25,190-192 (1979); D.

Hubbard and

Lightfoot,

Ind. Eng. Chem. Fundamentals,

370-379 (1966).

s5.5

Turbulent Flow in Ducts

165

(b)

For the flow between parallel plates, we can use the expression found in

Eq.

5.3-12 for the

time-smoothed velocity profile to get the turbulent momentum flux:

where

~C(V,/U)~. This is in accord with

Eq.

5.4-12.

55.5

TURBULENT

FLOW

DUCTS

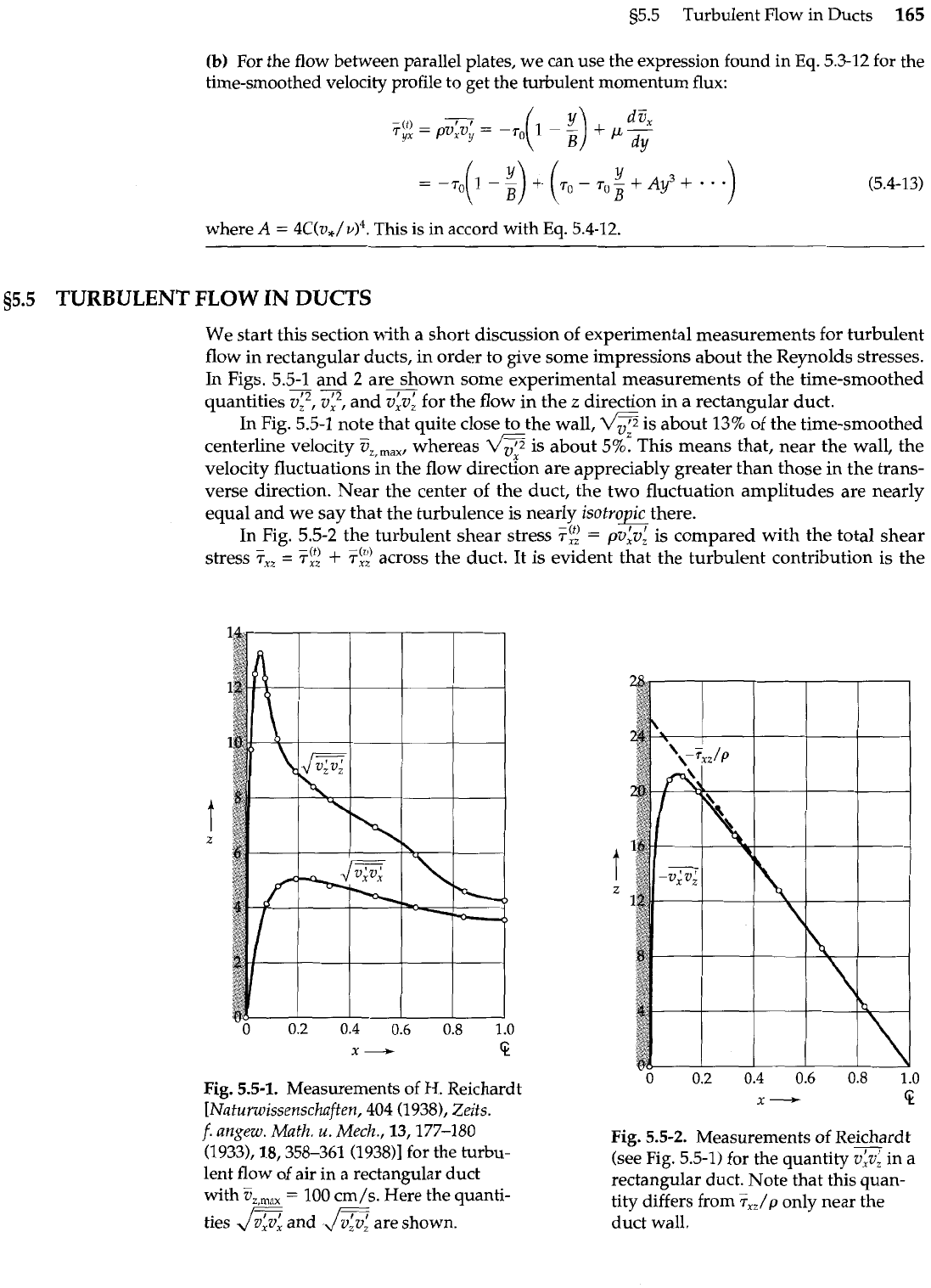
We start this section with a short discussion of experimental measurements for turbulent

flow in rectangular ducts, in order to give some impressions about the Reynolds stresses.

Figs.

5.5-1

and

are

shown some experimental measurements of the time-smoothed

quantities vL2,

v:~,

and v:vi for the flow in the

direction in a rectangular duct.

In Fig. 5.5-1 note that quite close to the wall,

is about

13%

of the time-smoothed

centerline velocity

Z,,,,,,

whereas

is about 5%. This means that, near the wall, the

velocity fluctuations in the flow direction are appreciably greater than those in the trans-

verse direction. Near the center of the duct, the two fluctuation amplitudes are nearly

equal and we say that the turbulence is nearly isotro~there.

Fig. 5.5-2 the turbulent shear stress

7:;

p:v;

is compared with the total shear

stress 7,,

7:;

7:?

across the duct.

is evident that the turbulent contribution is the

Fig.

5.5-1. Measurements

Reichardt

[Naturwissensckaften,

404

(1938),

Zeits.

angew.

Math.

Mech., 13,177-180

(1933), 18,358-361 (1938)l for the turbu-

lent flow of air in a rectangular duct

with

?&,,

100

cm/s. Here the quanti-

ties

and

are shown.

Fig.

5.5-2.

Measurements of Reichardt

(see Fig. 5.5-1) for the quantity in a

rectangular duct. Note that this quan-

tity differs from ?,,/p only near the

duct wall.

166

Chapter

Velocity Distributions in Turbulent Flow

more important over most of the cross section

and

that the viscous contribution

im-

portant only in the vicinity of the wall. This is further illustrated in Example

5.5-3.

Anal-

ogous behavior is observed in tubes of circular cross section.

Apply the results of

95.3

to obtain the average velocity for turbulent flow in a circular tube.

Estimation of

the

Average Velocity

SOLUTION

in a Circular Tube

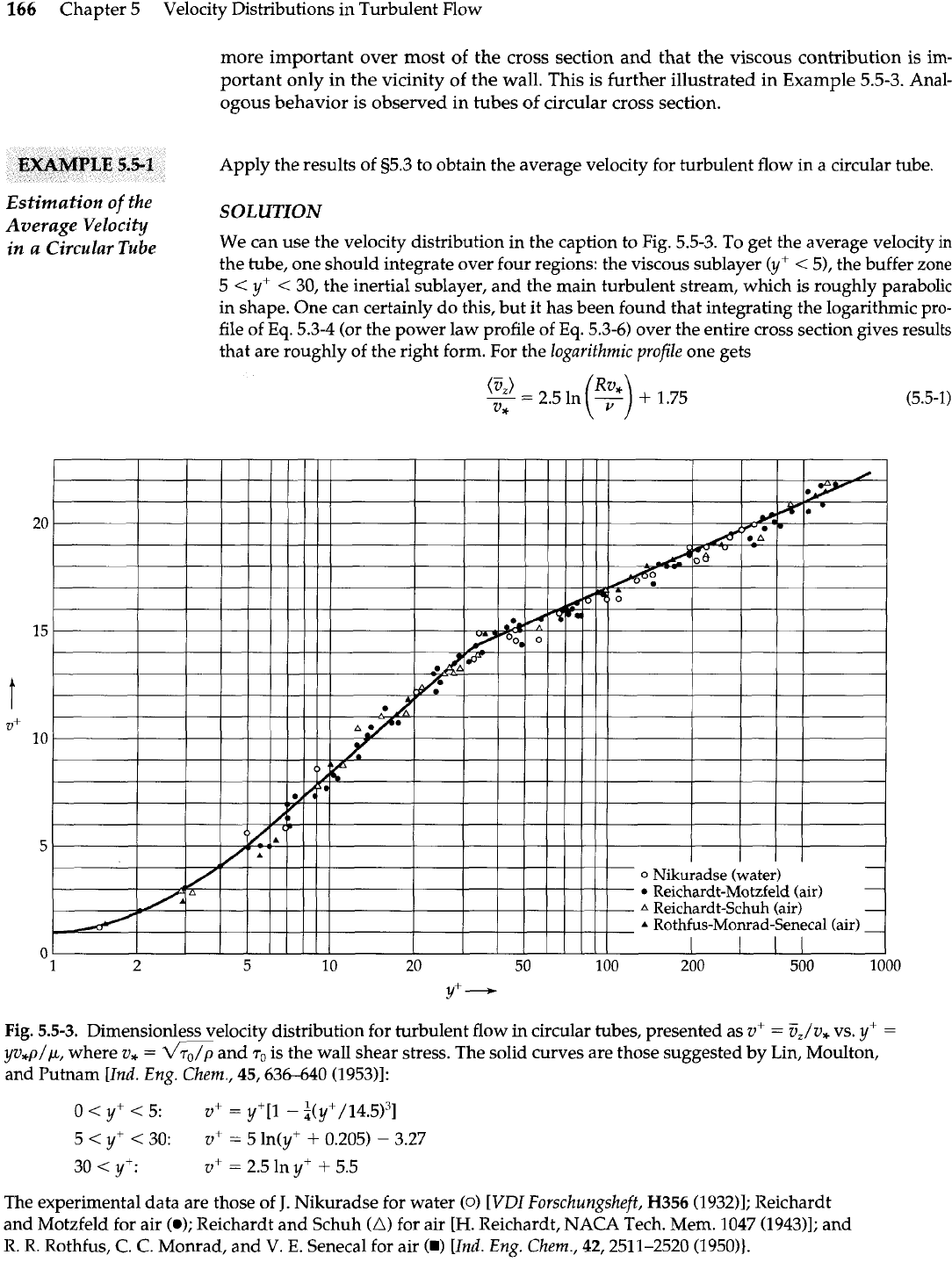
We can use the velocity distribution in the caption to Fig.

5.5-3.

To get the average velocity in

the tube, one should integrate over four regions: the viscous sublayer

(y+

51,

the buffer zone

30,

the inertial sublayer, and themain turbulent stream; which

roughly parabolic

in shape. One can certainly do this, but it has been found that integrating the logarithmic pro-

file of

Eq.

5.3-4

(or the power law profile of

Eq.

5.3-6)

over the entire cross section gives results

that are roughly of the right form. For the logarithmic profile one gets

Fig.

5.5-3.

Dimensionless velocity distribution for turbulent flow

circular tubes, presented as

5,/u,

vs.

yv,p/~,

where

and

.r,

is the wall shear stress. The solid curves are those suggested by Lin, Moulton,

and Putnam [Ind. Eng. Chem.,

45,636-640 (1953)l:

The experimental data are those of

Nikuradse for water

(0)

[VDI Forschungsheft,

H356

(1932)l;

Reichardt

and Motzfeld for air

(0);

Reichardt and Schuh

(A)

for air

[H.

Reichardt,

NACA

Tech. Mem.

1047 (1943)l;

and

Rothfus,

Monrad, and

Seneca1 for air

(H)

[Ind. Eng. Chem.,

42,2511-2520 (1950)).