Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena
Подождите немного. Документ загружается.

s4.3
Flow of Inviscid Fluids by Use of the Velocity Potential
127
We want to solve Eqs. 4.3-3 to
5
to obtain v,,
v,,
and
9
as functions of x and y. We
have already seen in the previous section that the equation of continuity in two-dimen-
sional flows can be satisfied by writing the components of the velocity in terms of a
stream function $(x, y). However, any vector that has a zero curl can also be written as the
gradient of a scalar function (that is, [V
x
vl
=
0 implies that
v
=
-V+).
It is very conve-
nient, then, to introduce a velocity potential $(x, y). Instead of working with the velocity
components
v,
and v,, we choose to work with +(x, y) and +(x,
y).
We then have the fol-
lowing relations:
(stream function)
(velocity potential)
Now Eqs. 4.3-3 and 4.3-4 will automatically be satisfied. By equating the expressions for
the velocity components we get
These are the Cauchy-Riemann equations, which are the relations that must be satisfied by
the real and imaginary parts of any analytic function3 w(z)
=
+(x,
y)
+
i+(x,
y),
where
z
=
x
+
iy. The quantity w(z) is called the complex potential. Differentiation of
Eq.
4.3-10 with
respect to x and Eq. 4.3-11 with respect to y and then adding gives
V2$
=
0.
Differentiat-
ing with respect to the variables in reverse order and then substracting gives
V2+
=
0.
That is, both
+(x,
y) and
+(x,
y) satisfy the two-dimensional Laplace eq~ation.~
As a consequence of the preceding development, it appears that any analytic func-
tion w(z) yields a pair of functions +(x,
y)
and +(x, y) that are the velocity potential and
stream function for some flow problem. Furthermore, the curves $(x, y)
=
constant and
+(x,
y)
=
constant are then the equipotential lines and streamlines for the problem. The ve-
locity components are then obtained from Eqs.
4.3-6
and
7
or
Eqs.
4.3-8 and
9
or from
dw
-
=
-v,
+
ivy
(4.3-12)
dz
in which dw/dz is called the complex velocity. Once the velocity components are known,
the modified pressure can then be found from Eq.
4.3-5.
Alternatively, the equipotential lines and streamlines can be obtained from the in-
verse function z(w)
=
x(+,
$)
+
iy($,
$),
in which z(w) is any analytic function
of
w.
Be-
tween the functions
x($,
$)
and
y($,
+)
we can eliminate
cC/
and get
-
-
-
-
-
-
Some knowledge of the analytic functions of a complex variable is assumed here. Helpful
introductions to the subject can be found in
V.
L.
Streeter,
E.
B.
Wylie, and
K.
W.
Bedford,
Fluid
Mechanics,
McGraw-Hill, New York, 9th ed. (1998), Chapter
8,
and in
M.
D.
Greenberg,
Foundations of
Applied Mathematics,
Prentice-Hall, Englewood Cliffs,
N.J.
(1978), Chapters 11 and 12.
Even for three-dimensional flows the assumption of irrotational flow still permits the definition of
a
velocity potential. When v
=
-V+
is substituted into
(V
.
v)
=
0,
we get the three-dimensional Laplace
equation
V2+
=
0.
The solution of this equation is the subject of "potential theory," for which there is an
enormous literature. See, for example,
P.
M.
Morse and H. Feshbach,
Methods
of
Theoretical Physics,
McGraw-Hill, New York (19531, Chapter
11;
and
J.
M.
Robertson,
Hydrodynamics in Theory and
Application,
Prentice-Hall, Englewood Cliffs,
N.J.
(1965), which emphasizes the engineering applications.
There are many problems in flow through porous media, heat conduction, diffusion, and electrical
conduction that are described by Laplace's equation.

128
Chapter
4
Velocity Distributions with More Than One Independent Variable
EXAMPLE
4.3-1
Potential Flow around
a
Cylinder
SOLUTION
Similar elimination of
4
gives
Setting
4
=
a constant in
Eq.
4.3-13 gives the equations for the equipotential lines for
some
flow problem, and setting
$
=
constant in Eq. 4.3-14 gives equations for the stream-
lines. The velocity components can be obtained from
Thus from any analytic function w(z), or its inverse z(w), we can construct
a
flow net
with streamlines
$
=
constant and equipotential lines
4
=
constant. The task of finding
w(z) or
Z(W)
to satisfy
a
given flow problem is, however, considerably more difficult.
Some special methods are a~ailable"~ but it is frequently more expedient to consult a
table of conformal
mapping^.^
In the next two illustrative examples we show how to use the complex potential w(z)
to describe the potential flow around a cylinder, and the inverse function z(w) to solve
the problem of the potential flow into a channel. In the third example
we
solve the flow
in the neighborhood of a corner, which is treated further in s4.4 by the boundary-layer
method.
A
few general comments should be kept in mind:
The streamlines are everywhere perpendicular to the equipotential lines. This
property, evident from Eqs. 4.3-10,11, is useful for the approximate construction
of flow nets.
Streamlines and equipotential lines can be interchanged to get the solution of
another flow problem. This follows from
(a)
and the fact that both
4
and
$
are
solutions to the two-dimensional Laplace equation.
Any streamline may be replaced by a solid surface. This follows from the
boundary condition that the normal component of the velocity of the fluid is
zero at a solid surface. The tangential component is not restricted, since in po-
tential flow the fluid
is
presumed to be able to slide freely along the surface (the
complete-slip assumption).
(a)
Show that the complex potential
describes the potential flow around a circular cylinder of radius
R,
when the approach veloc-
ity is
v,
in the positive
x
direction.
(b)
Find the components of the velocity vector.
(c)
Find the pressure distribution on the cylinder surface, when the modified pressure far
from the cylinder is
9,.
(a)
To find the stream function and velocity potential, we write the complex potential in the
form
w(z)
=
+(x,
y)
+
i$(x,
y):
J.
Fuka, Chapter
21
in
K.
Rektorys,
Survey
of Applicable Mathematics,
MIT
Press, Cambridge, Mass.
(1969).
H.
Kober,
Dictionary of Conformal Representations,
Dover,
New
York, 2nd edition
(1957).
94.3
Flow of Inviscid Fluids by Use of the Velocity Potential
129
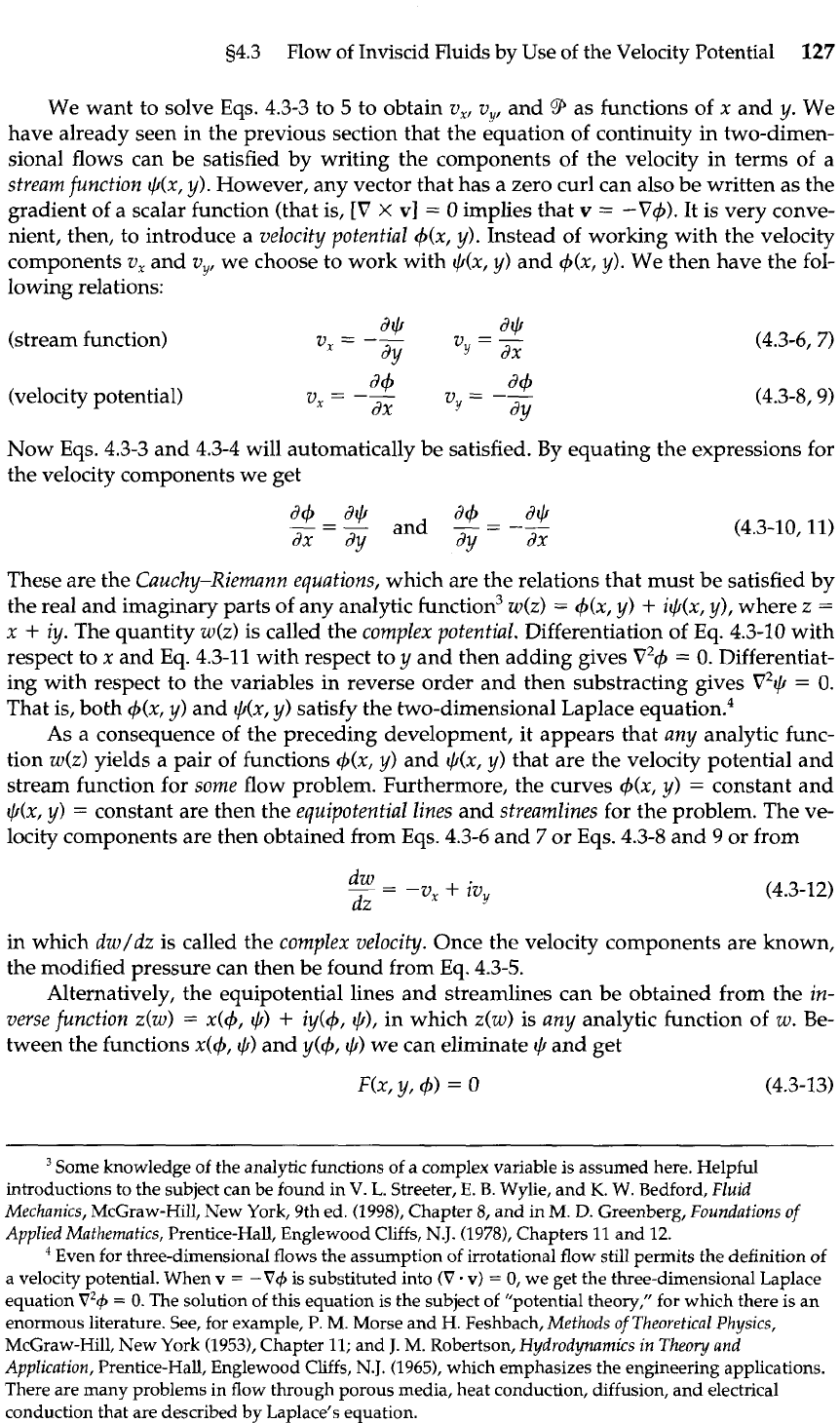
Hence the stream function is
To make a plot of the streamlines it is convenient to rewrite
Eq.
4.3-18 in dimensionless form
in which
q
=
cl//v,R,
X
=
x/R, and
Y
=
y/R.
In
Fig. 4.3-1 the streamlines are plotted as the curves
?
=
constant. The streamline
9
=
0
gives
a
unit circle, which represents the surface of the cylinder. The streamline
=
-:
goes
through the point
X
=
0,
Y
=
2,
and so on.
(b)
The velocity components are obtainable from the stream function by using Eqs. 4.3-6 and
7.
They may also be obtained from the complex velocity according to Eq. 4.3-12, as follows:
(COS
20
-
i
sin
20)
Therefore the velocity components as function of position are
(c)
On the surface of the cylinder,
v
=
R, and
v2
=
7.7;
+
v;
=
v?[(l
-
cos
2012
+
(sin
28)']
=
4v5 sin2
13
When
0
is zero or
T,
the fluid velocity is zero; such points are known as
stagnation points.
From Eq. 4.3-5 we know that
Then from the last two equations we get the pressure distribution on the surface of the cylinder
Fig.
4.3-1.
The streamlines for the potential
flow around
a
cylinder according to
Eq.
4.3-19.

130
Chapter 4 Velocity Distributions with More Than One Independent Variable
Note that the modified pressure distribution is symmetric about the x-axis; that is, for poten-
tial flow there is no form drag on the cylinder (dfAlembert's
para do^).^
Of course, we know
now that this is not really a paradox, but simply the result of the fact that the inviscid fluid
does not permit applying the no-slip boundary condition at the interface.
Show that the inverse function
Flow Into a
Z(W)
=
-
wb
+
+
exp(m/bv,)
11,
(4.3-26)
Rectangular Channel
represents the potential flow into a rectangular channel of half-width b. Here
v,
is the magni-
tude of the velocity far downstream from the entrance
to
the channel.
SOLUTION
First we introduce dimensionless distance variables
and the dimensionless quantities
The inverse function of
Eq.
4.3-26 may now be expressed in terms of dimensionless quantities
and split up into real and imaginary parts
Therefore
We can now set
q
equal to a constant, and the streamline
Y
=
Y(X) is expressed parametrically
in
@.
For example, the streamline
T
=
0
is given by
As
@
goes from
-a,
to
+a,
X
also goes from
-m
to
+
m;
hence the X-axis is a streamline.
Next, the streamline
9
=
n-
is given by
X=@-e'
Y=n-
(4.3-34,35)
As
@
goes from
-
to
+
a,,
X
goes from
-
m
to
-1
and then back to
-a;
that is, the stream-
line doubles back on itself. We select this streamline to be one of the solid walls of the rectan-
gular channel. Similarly, the streamline
?
=
-T
is the other wall.
The
streamlines
q
=
C,
where
-n-
<
C
<
T,
then give the flow pattern for the flow into the rectangular channel
as
shown in Fig.
4.3-2.
Next, from Eq. 4.3-29 the derivative -dz/dw can be found:
Comparison of this expression with
Eq.
4.3-15 gives for the velocity components
vyvm
35
=
-(I
+
e'cos
P)
--
- -
-
(e@ sin
*)
(4.3-37)
v2
v2
These equations have to be used in conjunction with Eqs. 4.3-30 and 31 to eliminate
@
and
?
in order to get the velocity components as functions of position.
Hydrodynamic paradoxes
are
discussed
in
G.
Birkhoff,
Hydrodynamics,
Dover,
New
York
(1955).
94.3
Flow of Inviscid Fluids by Use of the Velocity Potential
131
Flow
Near a Corner8
SOLUTION
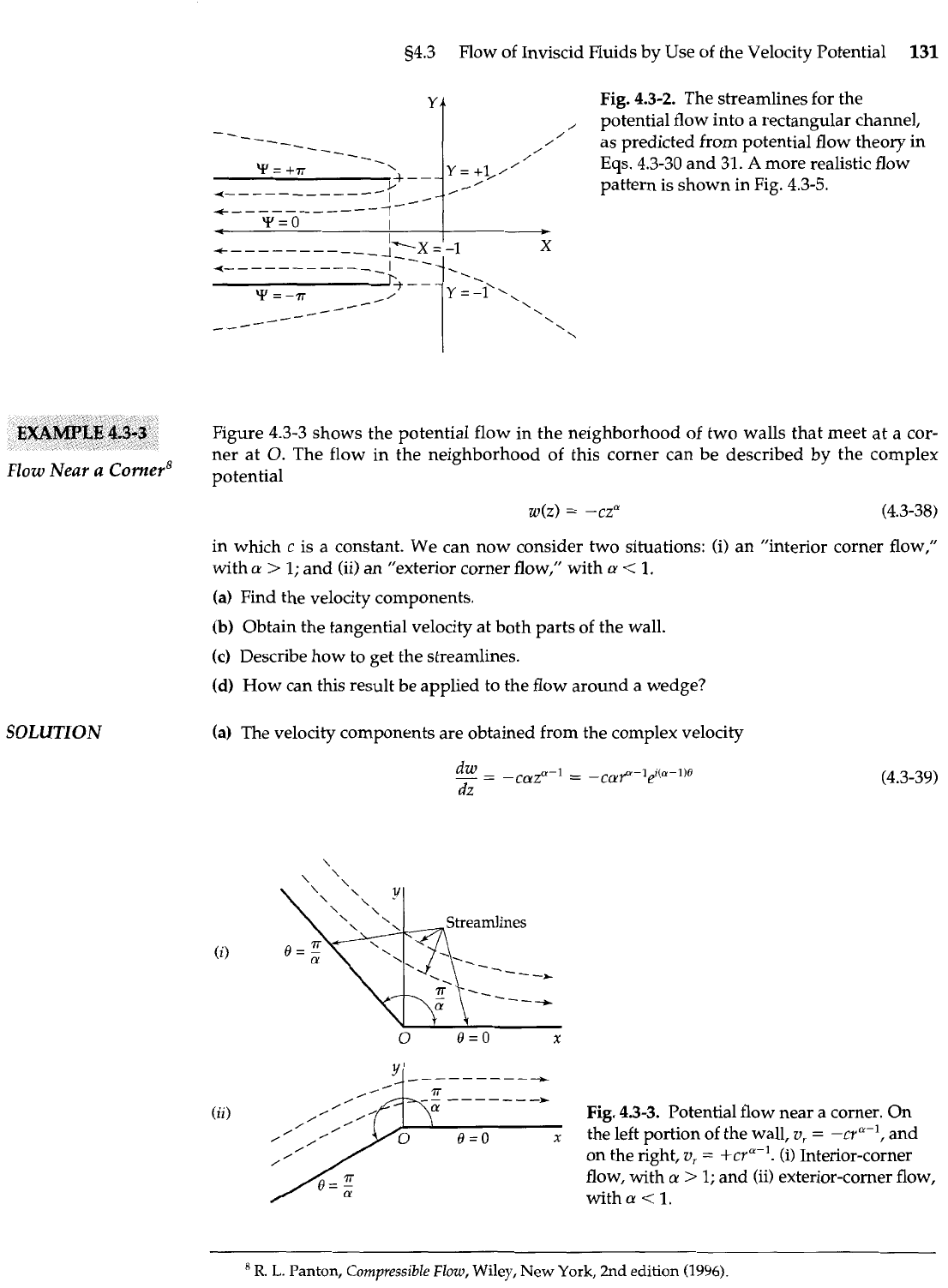
Figure
4.3-3
shows the potential flow in the neighborhood of two walls that meet at a cor-
ner at
0.
The flow in the neighborhood of this corner can be described by the complex
potential
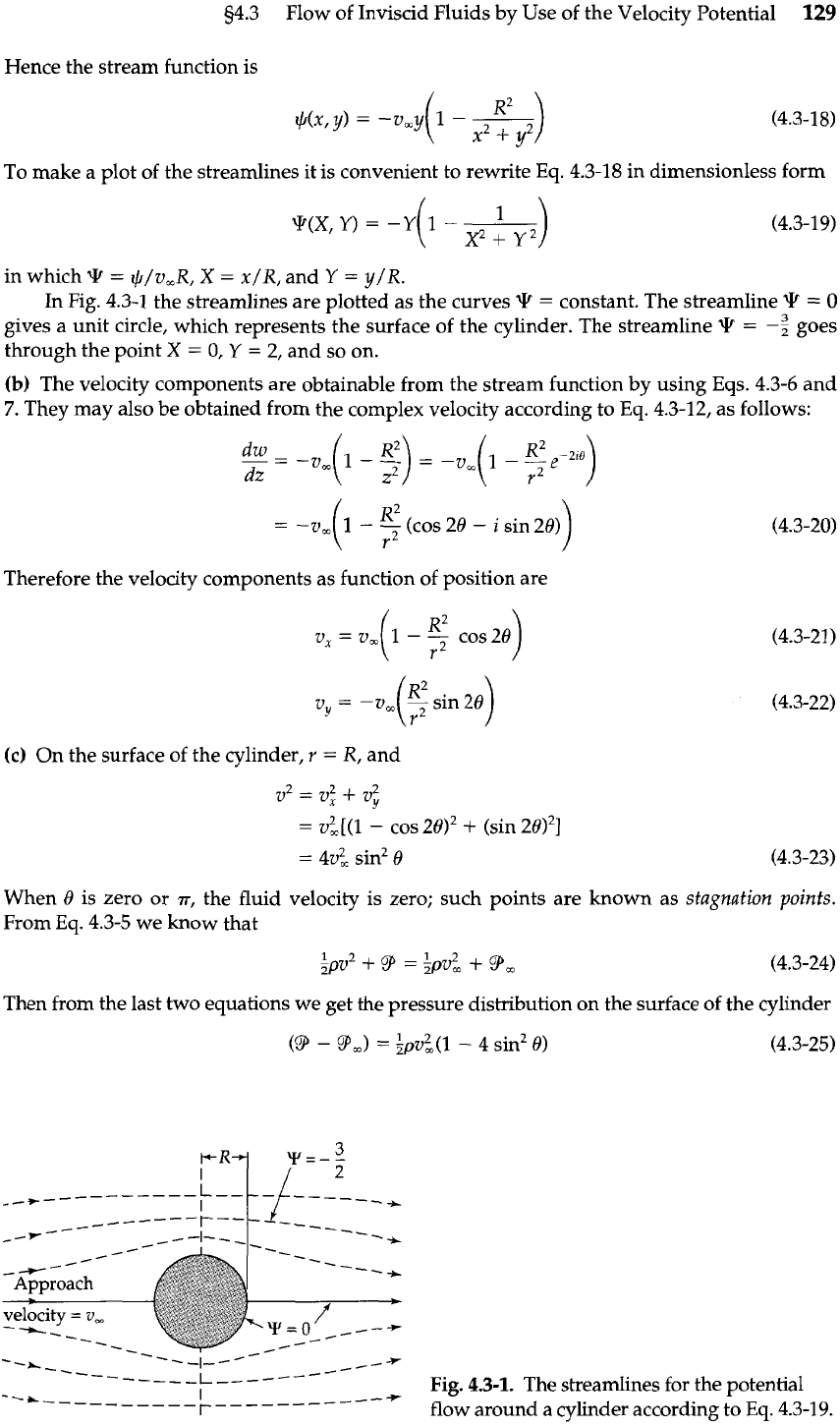
YA
Fig.
4.3-2.
The streamlines for the
in which
c
is a constant. We can now consider two situations: (i) an "interior corner flow,"
with
a
>
1;
and (ii) an "exterior corner flow," with
a
<
1.
(a) Find the velocity components.
(b)
Obtain the tangential velocity at both parts of the wall.
(c)
Describe how to get the streamlines.
(d)
How can this result be applied to the flow around a wedge?
-
-
--
--
-_
---
-
.
Y=+T
\
pG+-Y=
+
----------
--
I
*
--------
---
Y=O
-I---
<
I
(a) The velocity components are obtained from the complex velocity
,
potential flow into a rectangular channel,
/
/ /
as predicted from potential flow theory in
/
/
Eqs.
4.3-30
and
31.
A
more realistic flow
,
I
+I//
.
_
pattern is shown
in
Fig.
4.3-5.
t
Y,
-
- - -
-
-
-
-
-
+
*
(ii)
Fig.
4.3-3.
Potential flow near a corner. On
the left portion of the wall,
v,
=
-cF1,
and
on the right,
v,
=
+cua-'. (i) Interior-corner
flow, with
a
>
1;
and (ii) exterior-corner flow,
with
a
<
1.
+
-
-
-
-
- - - -
-
-
X
.
'.
\
_/---
\
\
----
\
\
'
R.
L.
Panton,
Compressible
Flow,
Wiley,
New
York,
2nd
edition
(1996).

132
Chapter
4
Velocity Distributions with More Than One Independent Variable
Fig.
4.3-4.
Potential flow along a wedge. On
-
the upper surface of the wedge,
v,
=
cxa-'
-
__-__---
cxP"'-P). The quantities
a
and
P
are related by
-------
P
=
(2/a)(a
-
1).
------------
Streamlines
-.
.
Hence from
Eq.
4.3-12 we get
v,
=
+cara-' cos
(a
-
1)6
v,
=
-cara-' sin
(a
-
1)6
(b)
The tangential velocity at the walls is
at 0
=
0:
ZIx
=
vr
=
Carn-l
=
C(yp-l
at 0
=
r/a:
v,
=
v,
cos
0
+
vy
sin 6
=
+cara-' cos
(a
-
1)6 cos 6
-
cara-' sin
(a
-
1)6 sin 0
=
cara-'
COS
a6
- -
-cays-'
(4.3-43)
Hence,
in
Case (i), the incoming fluid at the wall decelerates as it approaches the junction, and
the departing fluid accelerates as it moves away from the junction. In Case (ii) the velocity
components become infinite at the corner as
a
-
1
is then negative.
(c)
The complex potential can be decomposed into its real and imaginary parts
w
=
4
+
it,b
=
-cua(cos a6
+
i
sin a61
(4.3-44)
Hence the stream function is
1C,
=
-cyn sin a0 (4.3-45)
To get the streamlines, one selects various values for the stream function-say,
$,,
$,,
t,b,
.
,
-and then for each value one plots r as a function of 0.
(d)
Since for ideal flow any streamline may be replaced by a wall, and vice versa, the results
found here for
a
>
0
describe the inviscid flow over a wedge (see Fig. 4.3-4). We make use of
this in Example 4.4-3.
A
few words of warning are in order concerning the applicability of potential-flow
theory to real systems:
a.
For the flow around a cylinder, the streamlines shown in Fig. 4.3-1 do not con-
form to any of the flow regimes sketched in Fig. 3.7-2.
b.
For the flow into
a
channel, the predicted flow pattern of Fig. 4.3-2 is unrealistic
inside the channel and just upstream from the channel entrance.
A
much better
approximation to the actual behavior is shown in Fig.
4.3-5.
Both of these failures of the elementary potential theory result from the phenomenon of
separation:
the departure of streamlines from a boundary surface.
Separation tends to occur at sharp corners of solid boundaries, as in channel flow,
and on the downstream sides of bluff objects, as in the flow around a cylinder. Gener-
ally, separation is likely to occur in regions where the pressure increases in the direction
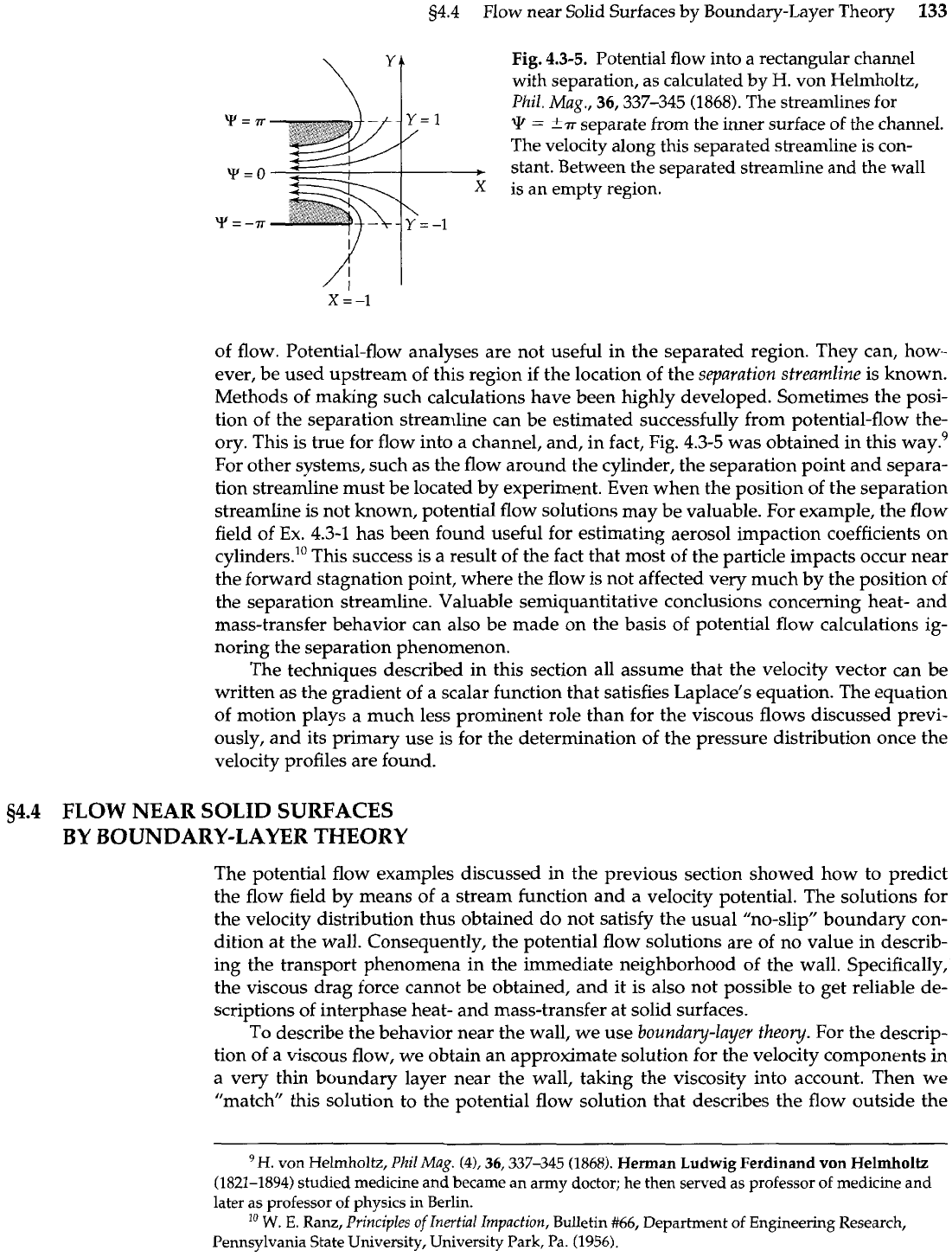
s4.4
Flow near Solid Surfaces by Boundary-Layer Theory
133
Fig.
4.3-5. Potential flow into a rectangular channel
with separation, as calculated by
H.
von Helmholtz,
Phil.
Mag.,
36,337-345
(1868).
The streamlines for
!P
=
-+r
separate from the inner surface of the channel.
The velocity along this separated streamline is con-
stant. Between the separated streamline and the wall
X is an empty region.
Y=-7r
of flow. Potential-flow analyses are not useful in the separated region. They can, how-
ever, be used upstream of this region if the location of the separation streamline is known.
Methods of making such calculations have been highly developed. Sometimes the posi-
tion of the separation streamline can be estimated successfully from potential-flow the-
ory. This is true for flow into a channel, and, in fact, Fig.
4.3-5
was obtained in this way.9
For other systems, such as the flow around the cylinder, the separation point and separa-
tion streamline must be located by experiment. Even when the position of the separation
streamline is not known, potential flow solutions may be valuable. For example, the flow
field of
Ex.
4.3-1 has been found useful for estimating aerosol impaction coefficients on
cylinders.1° This success is a result of the fact that most of the particle impacts occur near
the forward stagnation point, where the flow is not affected very much by the position of
the separation streamline. Valuable semiquantitative conclusions concerning heat- and
mass-transfer behavior can also be made on the basis of potential flow calculations ig-
noring the separation phenomenon.
The techniques described in this section all assume that the velocity vector can be
written as the gradient of a scalar function that satisfies Laplace's equation. The equation
of motion plays a much less prominent role than for the viscous flows discussed previ-
ously, and its primary use is for the determination of the pressure distribution once the
velocity profiles are found.
54.4
FLOW NEAR SOLID SURFACES
BY
BOUNDARY-LAYER
THEORY
The potential flow examples discussed in the previous section showed how to predict
the flow field by means of a stream function and a velocity potential. The solutions for
the velocity distribution thus obtained do not satisfy the usual "no-slip" boundary con-
dition at the wall. Consequently, the potential flow solutions
are
of no value in describ-
ing the transport phenomena in the immediate neighborhood of the wall. Specifically,
the viscous drag force cannot be obtained, and it is also not possible to get reliable de-
scriptions of interphase heat- and mass-transfer at solid surfaces.
To describe the behavior near the wall, we use boundary-layer the0
y.
For the descrip-
tion of a viscous flow, we obtain an approximate solution for the velocity components in
a very thin boundary layer near the wall, taking the viscosity into account. Then we
"match this solution to the potential flow solution that describes the flow outside the
H.
von Helmholtz,
Phil
Mag.
(4),
36,337-345
(1868).
Herman Ludwig Ferdinand von Helmholtz
(1821-1894) studied medicine and became an army doctor; he then served as professor of medicine and
later as professor of physics in Berlin.
lo
W.
E.
Ranz,
Principles
of
Inertial Impaction,
Bulletin
#66,
Department of Engineering Research,
Pennsylvania State University, University Park, Pa. (1956).

134
Chapter
4
Velocity Distributions with More Than One Independent Variable
boundary layer. The success of the method depends on the thinness of the boundary
layer, a condition that is met at high Reynolds number.
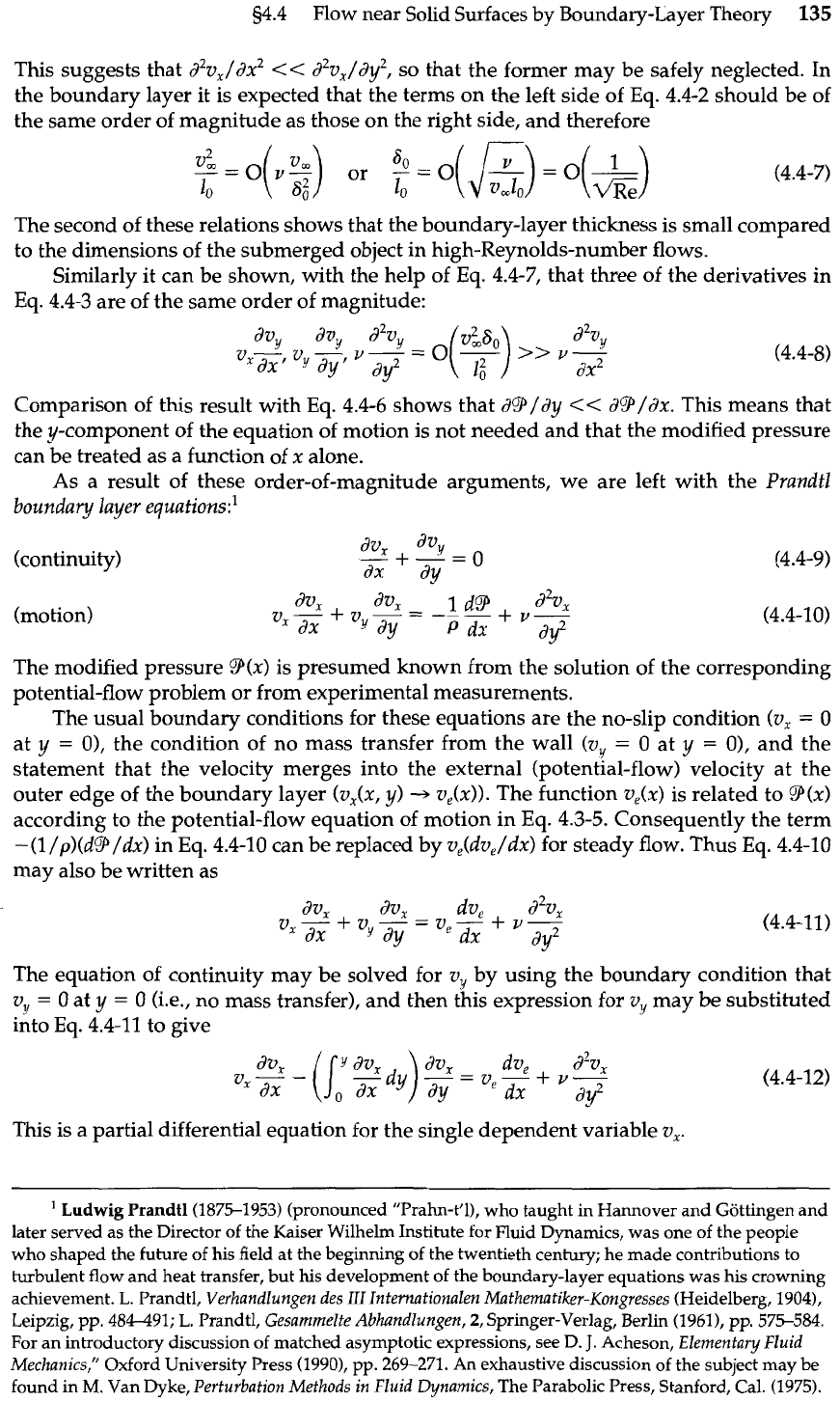
We consider the steady, two-dimensional flow of a fluid with constant
p
and
p
around a submerged object, such as that shown in Fig. 4.4-1. We assert that the main
changes in the velocity take place in a very thin region, the boundary layer, in which the
curvature effects are not important. We can then set up a Cartesian coordinate system
with x pointing downstream, and
y
perpendicular to the solid surface. The continuity
equation and the Navier-Stokes equations then become:
dv,
dvy
-+--=o
dx
dy
Some of the terms in these equations can be discarded by order-of-magnitude argu-
ments. We use three quantities as "yardsticks": the approach velocity v,, some linear di-
mension
1,
of the submerged body, and an average thickness 60 of the boundary layer.
The presumption that So
<<
lo
allows us to make a number of rough calculations of or-
ders of magnitude.
Since
vx
varies from zero at the solid surface to v, at the outer edge of the boundary
layer, we can say that
where
0
means "order of magnitude of." Similarly, the maximum variation in
v,
over
the length
lo
of the surface will be v,, so that
Here we have made use of the equation of continuity to get one more derivative (we are
concerned here only with orders of magnitude and not the signs of the quantities). Inte-
gration of the second relation suggests that
vy
=
0((6,/1,)v,)
<<
v,. The various terms in
Eq. 4.4-2 may now be estimated as
v--=O- v-=O--
d2vx
d2v,
(3;
2
((r)
-
=
O(t)
-
=
O($) (4.4-6)
"
dx dx2
dy2
Approximate outer limit
of
boundary
layer where
V,
+
VJX)
Fig.
4.4-1.
Coordinate system
for the two-dimensional flow
around a submerged object.
The boundary-layer thickness
is greatly exaggerated for pur-
poses of illustration. Because
the boundary layer is in fact
quite thin, it is permissible to
use rectangular coordinates lo-
cally along the curved surface.
54.4
Flow near Solid Surfaces by Boundary-Layer Theory
135
This suggests that d2vX/dx2
<<
d2vx/dy2,
SO
that the former may be safely neglected. In
the boundary layer it is expected that the terms on the left side of Eq. 4.4-2 should be of
the same order of magnitude as those on the right side, and therefore
The second of these relations shows that the boundary-layer thickness is small compared
to the dimensions of the submerged object in high-Reynolds-number flows.
Similarly it can be shown, with the help of Eq. 4.4-7, that three of the derivatives in
Eq. 4.4-3 are of the same order of magnitude:
Comparison of this result with Eq. 4.4-6 shows that
d9/dy
<<
dP/dx. This means that
the y-component of the equation of motion is not needed and that the modified pressure
can be treated as a function of
x
alone.
As a result of these order-of-magnitude arguments, we are left with the Prandtl
boundary layer equations:'
(continuity)
(motion)
The modified pressure 9(x) is presumed known from the solution of the corresponding
potential-flow problem or from experimental measurements.
The usual boundary conditions for these equations are the no-slip condition
(v,
=
0
at
y
=
O),
the condition of no mass transfer from the wall (vy
=
0 at
y
=
O),
and the
statement that the velocity merges into the external (potential-flow) velocity at the
outer edge of the boundary layer (vx(x,
y)
-,
v,(x)). The function v,(x) is related to 9(x)
according to the potential-flow equation of motion in Eq. 4.3-5. Consequently the term
-(1 /p)(dY /dx) in Eq. 4.4-10 can be replaced by v,(dv,/dx) for steady flow. Thus Eq. 4.4-10
may also be written as
dv, dv, dv, d2v,
vx-+v -=v,-+
V-
dx
Y
dy
dx
ay2
The equation of continuity may be solved for v, by using the boundary condition that
v,
=
0 at
y
=
0
(i.e., no mass transfer), and then this expression for v, may
be
substituted
into Eq. 4.4-11 to give
Y
dv, dv, d2v,
vX3- dx
(/,
iildy)%= veZ+
v2
This is a partial differential equation for the single dependent variable vx.
'
Ludwig
Prandtl(18751953) (pronounced "Prahn-t'l), who taught in Hannover and Gottingen and
later served as the Director of the Kaiser Wilhelm Institute for Fluid Dynamics, was one of the people
who shaped the future of his field at the beginning of the twentieth century; he made contributions to
turbulent flow and heat transfer, but his development of the boundary-layer equations was his crowning
achievement.
L.
Prandtl,
Verhandlungen des III Internationalen Mathematiker-Kongresses
(Heidelberg, 19041,
Leipzig,
pp.
484-491;
L.
Prandtl,
Gesammelte Abhandlungen,
2,
Springer-Verlag, Berlin (1961),
pp.
575-584.
For an introductory discussion of matched asymptotic expressions, see
D.
J.
Acheson,
Elementary Fluid
Mechanics,"
Oxford University Press (1990), pp. 269-271. An exhaustive discussion of the subject may be
found in
M.
Van Dyke,
Perturbation Methods in Fluid Dynamics,
The Parabolic Press, Stanford,
Cal.
(1975).
136
Chapter 4
Velocity Distributions with More Than One Independent Variable
This equation may now be multiplied by
p
and integrated from
y
=
0
to
y
=
to
give the
van Ka'rmhn momentum balance2
Here use has been made of the condition that v,(x,
y)
-+
v,(x) as
y
-+
a.
The quantity on
the left side of
Eq.
4.4-13 is the shear stress exerted by the fluid on the wall:
-~,,l~=~.
The original Prandtl boundary-layer equations, Eqs. 4.4-9 and 10, have thus been
transformed into Eq. 4.4-11,
Eq.
4.4-12, and
Eq.
4.4-13, and any of these may be taken as
the starting point for solving two-dimensional boundary-layer problems. Equation 4.4-
13, with assumed expressions for the velocity profile, is the basis of many "approximate
boundary-layer solutions" (see Example 4.4-1). On the other hand, the analytical or nu-
merical solutions of Eqs. 4.4-11 or 12 are called "exact boundary-layer solutions" (see
Ex-
ample 4.4-2).
The discussion here is for steady, laminar, two-dimensional flows of fluids with con-
stant density and viscosity. Corresponding equations are available for unsteady flow,
turbulent flow, variable fluid properties, and three-dimensional boundary
Although many exact and approximate boundary-layer solutions have been ob-
tained and applications of the theory to streamlined objects have been quite successful,
considerable work remains to be done on flows with adverse pressure gradients (i.e.,
positive
dP/dx)
in
Eq.
4.4-10, such as the flow on the downstream side of
a
blunt object.
In such flows the streamlines usually separate from the surface before reaching the rear
of the object (see Fig. 3.7-2). The boundary-layer approach described here is suitable for
such flows only in the region upstream from the separation point.
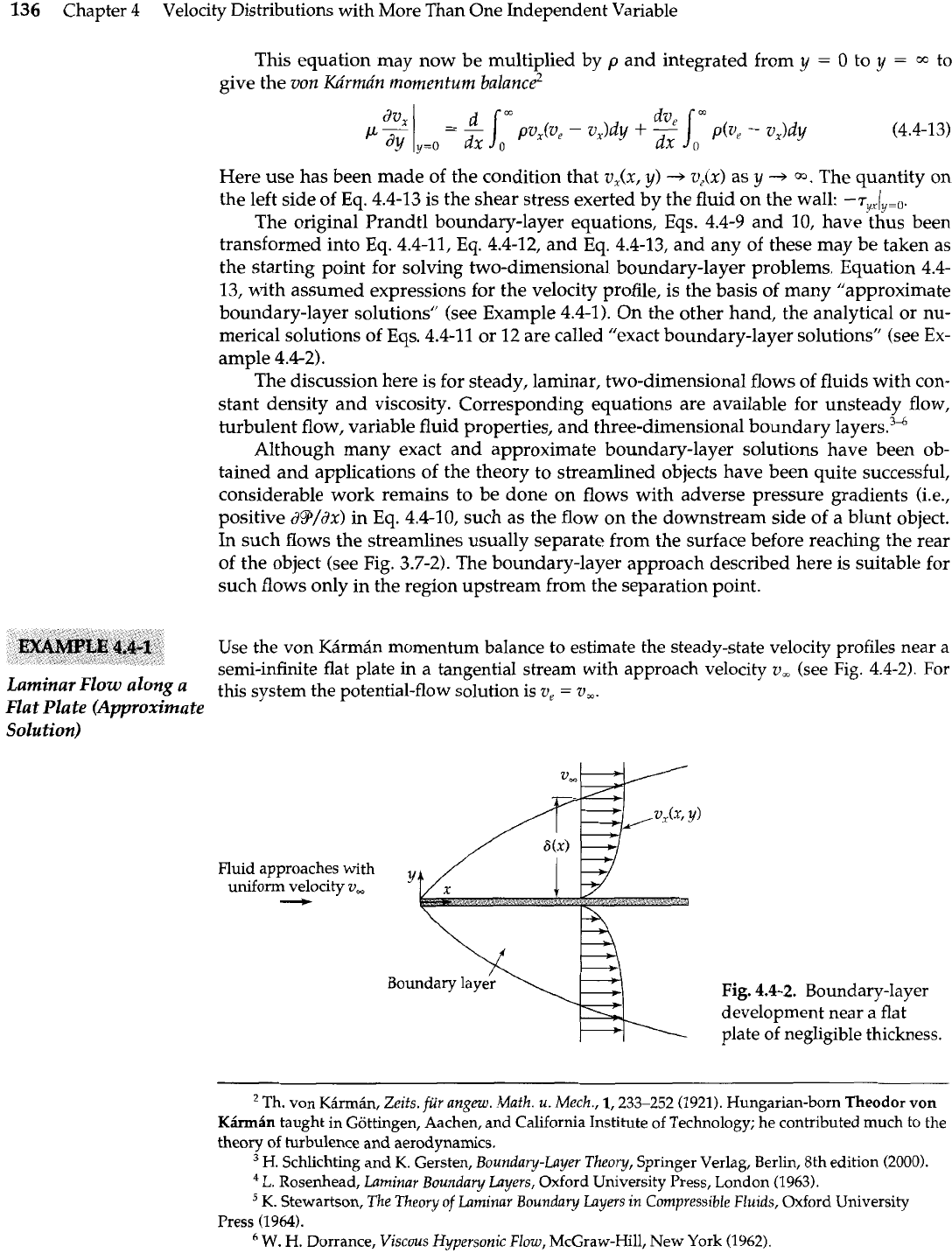
Use the von K5rm6n momentum balance to estimate the steady-state velocity profiles near a
semi-infinite flat plate in a tangential stream with approach velocity
v,
(see Fig. 4.4-2). For
Laminar
a
this system the potential-flow solution is
v,
=
a,.
Flat Plate (Approximate
Solution)
Fluid approaches with
uniform velocity
v,
-
Fig.
4.4-2.
Boundary-layer
development near a flat
plate of negligible thickness.
Th.
von Ksrmin,
Zeits, fur angew. Math. u. Mech.,
1,233-252 (1921). Hungarian-born Theodor von
Kh6n
taught in Gottingen, Aachen, and California Institute of Technology; he contributed much to the
theory
of
turbulence
and aerodynamics.
H.
Schlichting and
K.
Gersten,
Boundary-Layer Theory,
Springer Verlag, Berlin, 8th edition (2000).
L.
Rosenhead,
Laminar Boundary Layers,
Oxford University Press, London (1963).
K.
Stewartson,
The
Theory of Laminar Bounday Layers in Compressible Fluids,
Oxford University
Press
(1964).
'
W.
H.
Dorrance,
Viscous Hypersonic Flow,
McGraw-Hill, New York (1962).
