Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

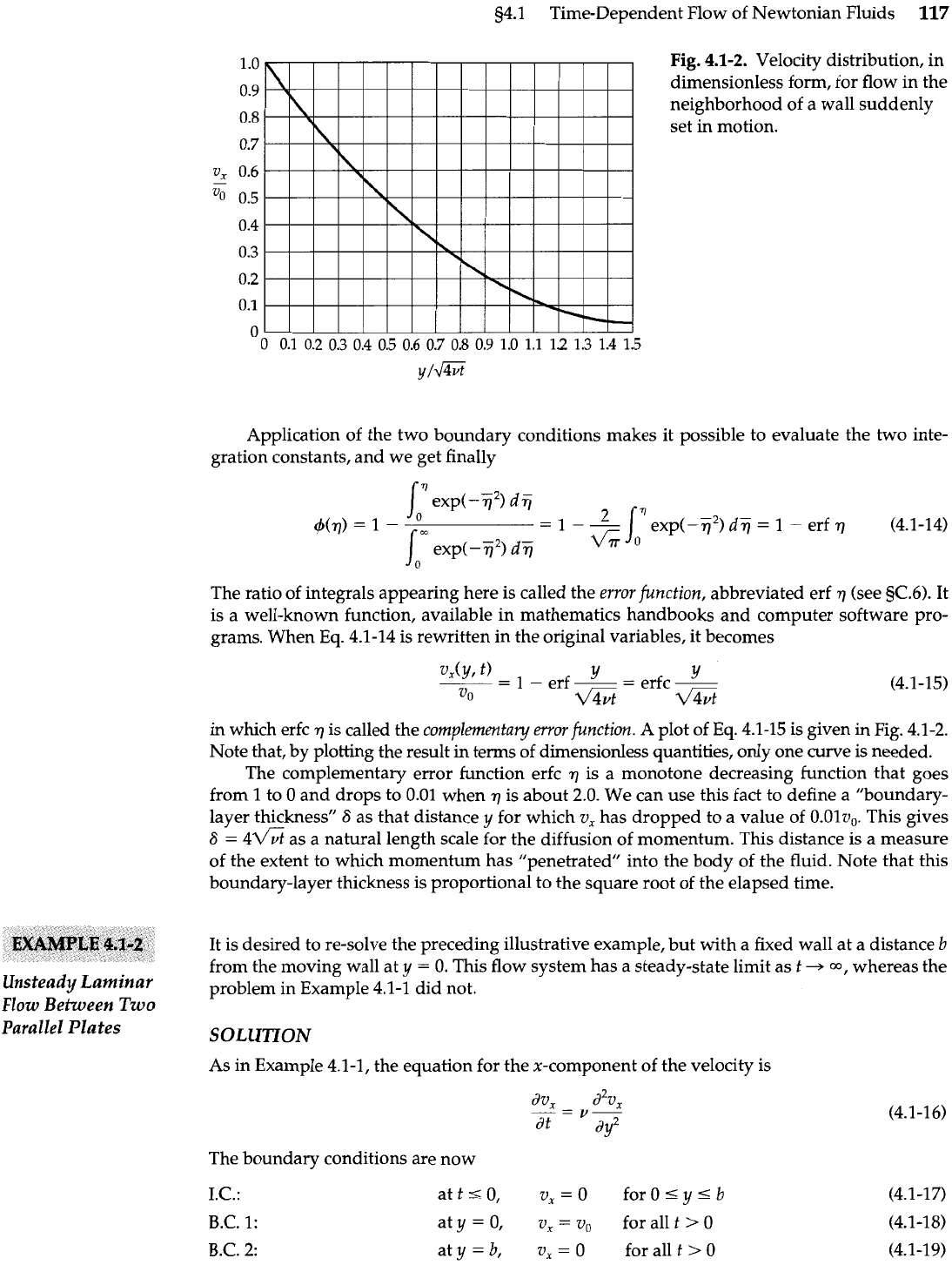
94.1 Time-Dependent Flow of Newtonian Fluids

117

Fig.

4.1-2.

Velocity distribution, in

dimensionless form, for flow in the

neighborhood of a wall suddenly

set in motion.

Application of the two boundary conditions makes it possible to evaluate the two inte-

gration constants, and we get finally

The ratio of integrals appearing here is called the error function, abbreviated erf

(see 9C.6). It

is a well-known function, available in mathematics handbooks and computer software pro-

grams. When Eq. 4.1-14 is rewritten in the original variables, it becomes

which erfc

is called the complementa

mor function. A plot of Eq. 4.1-15 is given in Fig. 4.1-2.

Note that, by plotting the result

terms of dimensionless quantities, only one curve

needed.

The complementary error function erfc

is a monotone decreasing function that goes

from

to 0 and drops to 0.01 when

is about 2.0. We can use this fact to define a "boundary-

layer thickness"

as that distance

for which

has dropped to a value of 0.01~~. This gives

46 as a natural length scale for the diffusion of momentum. This distance is a measure

of the extent to which momentum has "penetrated into the body of the fluid. Note that this

boundary-layer thickness is proportional to the square root of the elapsed time.

It is desired to re-solve the preceding illustrative example, but with a fixed wall at a distance

from the moving wall at

0. This flow system has a steady-state limit as

whereas the

Unsteady Laminar

problem in Example 4.1-1 did not.

Flow

Between

Two

Parallel Plates

SOLUTlON

As in Example 4.1-1, the equation for the x-component of the velocity is

The boundary conditions are now

I.C.:

B.C.

att50, v,=O forOSySb

for all

118

Chapter

Velocity Distributions with More Than One Independent Variable

It is convenient to introduce the following dimensionless variables:

The choices for dimensionless velocity and position ensure that these variables will go from

to 1. The choice of the dimensionless time is made so that there will be no parameters occur-

ring

the transformed partial differential equation:

The initial condition is

0 at

0, and the boundary conditions are

and

+=Oatq=l.

We know that at infinite time the system attains a steady-state velocity profile +,(q) so

that at

Eq. 4.1-21 becomes

for the steady-state limiting profile.

We then can write

$47,

+,(77)

4t(77,

where

is the transient part of the solution, which fades out as time goes to infinity. Substi-

tution of this expression into the original differential equation and boundary conditions then

gives for

with

0, and

at q

0 and

To solve Eq. 4.1-25 we use the "method of separation of (dependent) variables," in which

we assume a solution of the form

Substitution of this trial solution into Eq. 4.1-25 and then division by the product

gives

The left side is a function of

alone, and the right side is a function of

alone. This means that

both sides must equal a constant. We choose to designate the constant as -c2 (we could equally

well use c or +c2, but experience tells us that these choices make the subsequent mathematics

somewhat more complicated). Equation 4.1-27 can then be separated into two equations

These equations have the following solutions (see Eqs. C.l-1 and

3):

A~-~~~

sin cv

cos

in which

and

are constants of integration.

4. Time-Dependent Flow of Newtonian Fluids

119

We now apply the boundary and initial conditions in the following way:

B.C.

Because

0 at q

0, the function

must be zero at

Hence

must be zero.

B.C.

Because

0 at

the function

must be zero at

This will be true if

sin

is zero. The first choice would lead to

0 for all

r],

and that would be physically

unacceptable. Therefore we make the second choice, which leads to the requirement that

?r,

+2r, +3r,

- - - -

We label these various admissible values of

(called "eigenvalues") as

and write

nr,

with n

21,

+2,

+3,

. .

(4.1-32)

There are thus many admissible functions

(called "eigenfunctions") that satisfy Eq. 4.1-29

and the boundary conditions; namely,

sin nrq, with n

0, +I, -C2, k3,

. .

(4.1-33)

The corresponding functions satisfying

Eq.

4.1-28 are called

and are given by

I.C.: The combinations

fng,

satisfy the partial differential equation for

in Eq. 4.1-25, and

so will any superposition of such products. Therefore we write for the solution of Eq.

4.1-25

exp(-n2.rr2d sin nrr]

n=-m

in which the expansion coefficients

AnBn

have yet to be determined. In the sum, the term

0 does not contribute; also since sin(-n)q

-sin(+n)q, we may omit all the terms

with negative values of n. Hence, Eq. 4.1-35 becomes

exp(-n2r2r) sin nrq

n=l

According to the initial condition,

0, so that

,=I

sin nrr]

We now have to determine all the

from this one equation! This is done by multiplying

both sides of the equation by sin mrr], where

is an integer, and then integrating over the

physically pertinent range from

0 to

1, thus:

lo1

r])

sin mrqdr)

sin nrr] sin rnrr]dr]

n=l

The left side gives l/m.rr; the integrals on the right side are zero when n

m and when n

Hence the initial condition leads to

The final expression for the dimensionless velocity profile is obtained from Eqs. 4.1-24, 36,

and 39 as

+(r],

r])

exp(-n2r2r) sin nrr]

n=l

The solution thus consists of a steady-state-limit term minus a transient term, which fades out

with increasing time.

120

Chapter 4

Velocity Distributions with More Than One Independent Variable

Those readers who are encountering the method of separation of variables for the

first time will have found the above sequence of steps rather long and complicated.

However, no single step

the development is particularly difficult. The final solution in

Eq.

4.1-40 looks rather involved because of the infinite sum. Actually, except for very

small values of the time, only the first few terms in the series contribute appreciably.

Although we do not prove it here, the solution to this problem and that of the pre-

ceding problem are closely related.* In the limit of vanishingly small time, Eq. 4.1-40 be-

comes equivalent to Eq. 4.1-15. This is reasonable, since, at very small time, in this

problem the fluid is in motion only very near the wall at

and the fluid cannot

"feel" the presence of the wall at

Since the solution and result in Example 4.1-1 are

far simpler than those of this one, they are often used to represent the system if only

small times are involved. This is, of course, an approximation, but a very useful one. It is

often used in heat- and mass-transport problems as well.

semi-infinite body of liquid is bounded on one side by a plane surface (the xz-plane). Ini-

tially the fluid and solid are at rest. At time t

0 the solid surface is made to oscillate sinu-

Unsteady Laminar

soidally

the

direction with amplitude Xo and (circular) frequency o. That is, the

Flow near an

displacement

of the plane from its rest position is

Oscillating Plate

X(t)

Xo sin ot

(4.1-41)

and the velocity of the fluid at

0 is then

vx(O,

COS

d t

(4.1-42)

We designate the amplitude of the velocity oscillation by

Xow and rewrite Eq. 4.1-42 as

v,(O,

cos wt

vo%{eiUt} (4.1-43)

where

%{z]

means "the real part of

z."

For oscillating systems we are generally not interested in the complete solution, but only

the "periodic steady state" that exists after the initial "transients" have disappeared. In this

state all the fluid particles in the system will be executing sinusoidal oscillations with fre-

quency

but with phase and amplitude that are functions only of position. This "periodic

steady state" solution may be obtained by an elementary technique that is widely used. Math-

ematically it is an asymptotic solution for t

SOLUTION

Once again the equation of motion is given by

and the initial and boundary conditions are given by

I.C.:

B.C. 1:

B.C. 2:

at t

0 for all y

~,%{e'"~) for all

aty=m, v,=O for all t

The initial condition will not be needed, since we are concerned only with the fluid response

after the plate has been oscillating for a long time.

We postulate an oscillatory solution of the form

See

Carslaw and J.

Jaeger,

Conduction of Heat

Solids,

Oxford University Press, 2nd edition

(1959),

pp.

308-310, for a series solution that is particularly good for short times.

s4.2 Solving Flow Problems Using a Stream Function

121

Here vO is chosen to be a complex function of

so that v,(y, t) will differ from

v,(O,

t) both in

amplitude and phase. We substitute this trial solution into Eq. 4.1-44 and obtain

Next we make use of the fact that, if %{z,w)

%{z2w}, where

and

are two complex quan-

tities and w is an arbitrary complex quantity, then

2,.

Then Eq. 4.1-49 becomes

with the following boundary conditions:

B.C.

B.C. 2:

Equation 4.1-50 is of the form of Eq.

C.1-4

and has the solution

CleGy

cze-Gy

Since

/V'?)(l

i),

this equation can be rewritten as

CleGml+dY

-v'iz(l+i)y

The second boundary condition requires that

0, and the first boundary condition gives

v,. Therefore the solution to Eq. 4.1-50 is

From this result and Eq. 4.1-48, we get

or finally

vJy, t)

voe-t/w/2v~

cos

(ot

l/o/2vy)

(4.1-57)

In this expression, the exponential describes the

attenuation

of the oscillatory motion-that is,

the decrease in the amplitude of the fluid oscillations with increasing distance from the plate.

In the argument of the cosine, the quantity

-wy

is called the phase shift; that is, it de-

scribes how much the fluid oscillations at a distance

from the wall are "out-of-step" with the

oscillations of the wall itself.

Keep in mind that Eq. 4.1-57 is not the complete solution to the problem as stated in Eqs.

4.1-44 to 47, but only the "periodic-steady-state" solution. The complete solution is given in

Problem 4D.1.

$4.2

SOLVING FLOW PROBLEMS USING

STREAM FUNCTION

Up to this point the examples and problems have been chosen so that there was only one

nonvanishing component of the fluid velocity. Solutions of the complete Navier-Stokes

equation for flow

two or three dimensions are more difficult to obtain. The basic pro-

cedure is, of course, similar: one solves simultaneously the equations of continuity and

motion, along with the appropriate initial and boundary conditions, to obtain the pres-

sure and velocity profiles.

However, having both velocity and pressure as dependent variables in the equation

of motion presents more difficulty in multidimensional flow problems than in the sim-

pler ones discussed previously. It is therefore frequently convenient to eliminate the

122

Chapter 4

Velocity Distributions with More Than One Independent Variable

pressure by taking the curl of the equation of motion, after making use of the vector

identity [v

Vv]

$V(V

v]], which is given in Eq.

A.4-23.

For fluids of

constant viscosity and density, this operation gives

This is the

equation of change

for

the vorticity

vl; two other ways of writing it are

given in Problem

3D.2.

For viscous flow problems one can then solve the vorticity equation (a third-order

vector equation) together with the equation of continuity and the relevant initial and

boundary conditions to get the velocity distribution. Once that is known, the pressure

distribution can be obtained from the Navier-Stokes equation in Eq. 3.5-6. This method

of solving flow problems is sometimes convenient even for the one-dimensional flows

previously discussed (see, for example, Problem

4B.4).

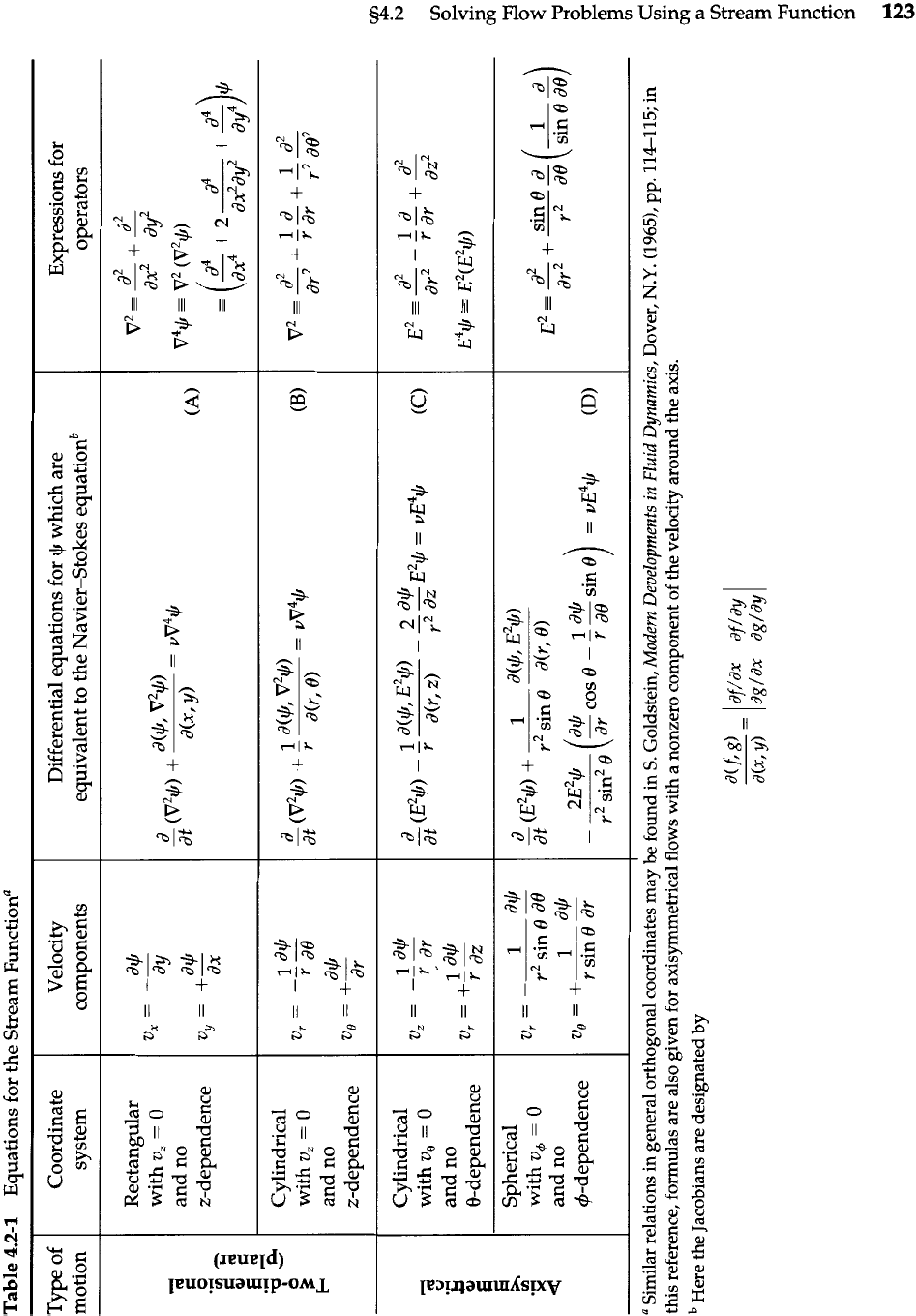
For planar or axisymmetric flows the vorticity equation can be reformulated by in-

troducing the

stream function

To do this, we express the two nonvanishing compo-

nents of the velocity as derivatives of

cC,

in such a way that the equation of continuity is

automatically satisfied (see Table 4.2-1). The component of the vorticity equation corre-

sponding to the direction in which there is no flow then becomes a fourth-order scalar

equation for

The two nonvanishing velocity components can then be obtained after

the equation for the scalar

has been found. The most important problems that can be

treated in this way are given in Table 4.1-1.'

The stream function itself is not without interest. Surfaces of constant

contain the

streamlines:

which in steady-state flow are the paths of fluid elements. The volumetric

rate of flow between the surfaces

and

is proportional to

In this section we consider, as an example, the steady, creeping flow past a station-

ary sphere, which is described by the Stokes equation of Eq. 3.5-8, valid for Re

< <

(see

the discussion right after Eq. 3.7-9). For creeping flow the second term on the left side of

Eq.

4.2-1

is set equal to zero. The equation is then linear, and therefore there are many

methods available for solving the pr~blem.~ We use the stream function method based

on Eq.

4.2-1.

Use Table 4.2-1 to set up the differential equation for the stream function for the flow of a

Newtonian fluid around

stationary sphere of radius

at Re

1. Obtain the velocity and

around

pressure distributions when the fluid approaches the sphere in the positive

direction, as in

Sphere

Fig.

2.6-1.

For a technique applicable to more general flows, see

M. Robertson,

Hydrodynamics in Theory and

Application,

Prentice-Hall, Englewood Cliffs, N.J. (1965), p. 77; for examples of three-dimensional flows

using two stream functions, see Problem 4D.5 and also

Ssrensen and

Stewart,

Chem. Eng. Sci.,

29,8194325 (1974).

Lahbabi and H.-C. Chang,

Chem. Eng. Sci.,

40,434447 (1985) dealt with high-Re

flow through cubic arrays of spheres, including steady-state solutions and transition to turbulence.

Stewart and M.

McClelland,

AICkE Journal,

29,947-956 (1983) gave matched asymptotic

solutions for forced convection in three-dimensional flows with viscous heating.

See, for example, G.

Batchelor,

An Introduction to Fluid Dynamics,

Cambridge University Press

(1967), S2.2. Chapter 2 of this book is an extensive discussion of the kinematics of fluid motion.

The solution given here follows that given by L. M. Milne-Thomson,

Theoretical Hydrodynamics,

Macmillan, New York, 3rd edition (1955), pp. 555-557. For other approaches, see H. Lamb,

Hydrodynamics,

Dover, New York (1945), §§337,338. For a discussion of unsteady flow around a sphere, see

Berker, in

Handbuck der Pkysik,

Volume VIII-2, Springer, Berlin (1963), §69; or

Villat and

Kravtchenko,

Leqons sur les Fluides Visqueux,

Gauthier-Villars, Paris (1943), Chapter VII. The problem of finding the

forces and torques on objects of arbitrary shapes is discussed thoroughly by

Kim and S.

Karrila,

Microkydrodynamics: Principles and Selected Applications,

Butterworth-Heinemann, Boston (1991), Chapter 11.

54.2

Solving Flow Problems Using

Stream Function

123

124

Chapter 4 Velocity Distributions with More Than One Independent Variable

SOLUTION

For steady, creeping flow, the entire left side of Eq.

of Table 4.2-1 may be set equal to zero,

and the equation for axisymmetric flow becomes

or, in spherical coordinates

[a2

-+--

sin

(

)I2,

dr2

sin

0 do

This is to be solved with the following boundary conditions:

B.C.

v,=

+--=o

sin 9

The first two boundary conditions describe the no-slip condition at the sphere surface. The

third implies that v,

v, far from the sphere (this can be seen by recognizing that

cos

and

-v, sin

far from the sphere).

We now postulate a solution of the form

since it will at least satisfy the third boundary condition in Eq. 4.2-6. When it is substituted

into Eq. 4.2-4, we get

The fact that the variable

does not appear in this equation suggests that the postulate in

Eq.

4.2-7 is satisfactory. Equation

4.2-8

is an "equidimensional" fourth-order equation (see

Eq.

C.1-14). When a trial solution of the form f(r)

Crn

is substituted into this equation, we find

that

may have the values -1,1,2, and

Therefore f(r) has the form

To satisfy the third boundary condition,

must be zero, and

has to be -$urn. Hence the

stream function is

The velocity components are then obtained by using Table 4.2-1 as follows:

The first two boundary conditions now give

-$V,X~

and

:V,R,

that

1--

;(:Y)

coso

-V,(l

(:)

(:)')

sin

These are the velocity components given in Eqs. 2.6-1 and 2 without proof.

g4.2 Solving Flow Problems Using a Stream Function

125

To get the pressure distribution, we substitute these velocity components into the r- and

6-components of the Navier-Stokes equation (given in Table B.7). After some tedious manip-

ulations we get

These equations may be integrated (cf. Eqs.

3.6-38

41),

and, when use is made of the bound-

ary condition that as r

the modified pressure

tends to

(the pressure in the plane

far from the sphere), we get

This is the same as the pressure distribution given in Eq. 2.6-4.

In g2.6 we showed how one can integrate the pressure and velocity distributions over

the sphere surface to get the drag force. That method for getting the force of the fluid on

the solid is general. Here we evaluate the "kinetic force"

by equating the rate of doing

work on the sphere (force

velocity) to the rate of viscous dissipation within the fluid,

thus

Fp,

I:T

(~:Vv)&r sin

Od6d+

Insertion of the function

(-~VV)

in spherical coordinates from Table

B.7

gives

Then the velocity profiles from Eqs. 4.2-13 and

are substituted into Eq. 4.2-19. When the in-

dicated differentiations and integrations (lengthy!) are performed, one finally gets

which is

Stokes'

law.

As pointed out in g2.6, Stokes' law is restricted to Re

0.1.

The expression for the drag

force can be improved by going back and including the [v

Vvl term. Then use of the

method

of matched asymptotic expansions

leads to the following result4

where

0.5772

is Euler's constant. This expression is good up to Re of about

Proudman and

Pearson,

Fluid

Mech.

2,237-262

(1957);

Chester and

Breach,

Fluid.

Mech.

37,751-760

(1969).

126

Chapter

Velocity Distributions with More Than One Independent Variable

54.3

FLOW OF

INVISCID FLUIDS BY USE

THE

VELOCITY

POTENTIAL'

Of course, we know that inviscid fluids (i.e., fluids devoid of viscosity) do not actually

exist. However, the Euler equation of motion of Eq. 3.5-9 has been found to be useful

for describing the flows of low-viscosity fluids at Re

around streamlined objects

and gives a reasonably good description of the velocity profile, except very near the

object and beyond the line of separation.

Then the vorticity equation in Eq. 3D.2-1 may be simplified by omitting the term

containing the kinematic viscosity. If, in addition, the flow is steady and two-dimen-

sional, then the terms d/dt and

Vv] vanish. This means that the vorticity

[Vx v]

is constant along a streamline. If the fluid approaching a submerged object has no vortic-

ity far away from the object, then the flow will be such that

vl will be zero

throughout the entire flow field. That is, the flow will be irrotational.

To summarize, if we assume that

constant and [V

0, then we can expect

to get a reasonably good description of the flow of low-viscosity fluids around sub-

merged objects in two-dimensional flows. This type of flow is referred to as potential pow.

Of course we know that this flow description will be inadequate in the neighbor-

hood of solid surfaces. Near these surfaces we make use of a different set of assump-

tions, and these lead to boundary-layer the0

which is discussed in s4.4. By solving the

potential flow equations for the "far field and the boundary-layer equations for the

"near field and then matching the solutions asymptotically for large Re, it is possible to

develop an understanding of the entire flow field around a streamlined ~bject.~

To describe potential flow we start with the equation of continuity for an incom-

pressible fluid and the Euler equation for an inviscid fluid (Eq. 3.5-9):

(continuity) (V-v)

0 (4.3-1)

(motion)

In the equation of motion we have made use of the vector identity [v

Vv]

v;v2

v11 (see Eq. A.4-23).

For the two-dimensional, irrotational flow the statement that

0 is

(irrotational)

and the equation of continuity is

(continuity)

The equation of motion for steady, irrotational flow can be integrated to give

(motion) ip(vz

constant (4.3-5)

That is, the sum of the pressure and the kinetic and potential energy per unit volume is

constant throughout the entire flow field. This is the Bernoulli equation for incompress-

ible, potential flow, and the constant is the same for all streamlines. (This has to be con-

trasted with Eq. 3.5-12, the Bernoulli equation for a compressible fluid in any kind of

flow; there the sum of the three contributions is a different constant on each streamline.)

Kirchhoff, Chapter

Handbook of

Fluid

Dynamics

(R.

Johnson,

ed.),

CRC

Press,

Boca

Raton, Fla.

(1998).

Van Dyke,

Perturbation Methods in Fluid Dynamics,

The Parabolic Press, Stanford,

Cal.

(1975).