Bhushan B. Nanotribology and Nanomechanics: An Introduction
Подождите немного. Документ загружается.

808 Bharat Bhushan and Michael Nosonovsky
In the preceding subsections, it was assumed that the roughness parameters are
scale-dependent for L < L
lwl
, whereas the contact parameters are scale-dependent
for L < L
lc
. The upper limit of scale dependence for the contact parameters, L
lc
,
depends on the normal load, and it is reasonable to assume that L
lc
is a function of
A
r
/A
a
, andthe contactparametersare scale-dependentwhen A
r
/A
a
is belowa certain
critical value. It is convenient to consider the apparent pressure p
a
, which is equal
to the normal load divided by the apparent area of contact [23].
For elastic contact, based on (15.15) and (15.17), this condition can be written
as
A
re
A
a
∝
β
∗
p
a
σ
= p
a
β
∗
0
σ
0
L
L
lwl
m−n
< p
ac
, (15.24)
where p
ac
is a critical apparent pressure, below which the scale dependence oc-
curs [23]. From (15.24) one can find
L < L
lwl
β
∗
0
σ
0
p
a
p
ac
1/(n−m)
. (15.25)
The right-hand expression in (15.24) is defined as L
lc
L
lc
= L
lwl
β
∗
0
σ
0
p
a
p
ac
1/(n−m)
. (15.26)
For plastic contact, based on (15.22)
A
rp
A
a
∝
p
a
√
1+ (L
d
/L)
m
< p
ac
. (15.27)
In a similar manner to the elastic case, (15.27) yields [23]
L
lc
= L
d
⎡
⎢
⎢
⎢
⎢
⎢
⎣
p
a
p
ac
2
−1
⎤
⎥
⎥
⎥
⎥
⎥
⎦
−1/m
. (15.28)
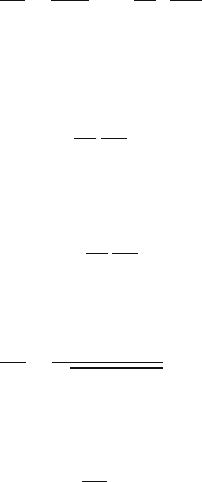
Load dependenceof the long wavelength limit for contactparameters, L
lc
is pre-
sented in Fig. 15.11 for an elastic contact based on (15.28), and for a plastic contact
based on (15.28), for m = 0.5, n = 0.2 [23]. The load (apparent pressure) is normal-
ized by β
∗
0
/(p
ac
σ
0
) for the elastic contact and by p
ac
for the plastic contact. In the
case of elastic contact, it is observed, that the long wavelength limit decreases with
increasing load. For a problem, characterized by a given scale length L, increase
of load will result in decrease of L
lc
and, eventually, the condition L < L
lc
will be
violated;thus the contact parameters, including the coefficient of friction, will reach
the macroscale values. Decrease of L
lc
with increasing load is also observed in the
case of plastic contact, the data presented for p
a
/p
ac
> 1.
15 Scale Effect in Mechanical Properties and Tribology 809
2
1
0
01 2
2
1
0
01 2
Load dependence of wavelength limit
L
lc
/L
lwl
Apparent pressure p
a
β
*
0
/(p
a0
σ
0
)
m = 0.5
n = 0.2
L
lc
/L
d
Apparent pressure p
a
/p
a0
m = 0.5
n = 0.2
Elastic contact
Plastic contact
Fig. 15.11. Dependence of
the normalized long wave-
length limit for contact par-
ameters on load (normalized
apparent pressure) for elastic
and plastic contacts (m = 0.5,
n = 0.2)
15.5 Scale Effect in Friction
According to the adhesion and deformationmodel of friction [16], the coefficient of
dry friction μ can be presented as a sum of adhesion component μ
a
and deformation
(plowing) component μ
d
. The later, in the presence of particles, is a sum of asperity
summits deformation component μ
ds
and particles deformation component μ
dp
,so
that the total coefficient of friction is [21]
μ = μ
a
+ μ
ds
+ μ
dp
=
F
a
+ F
ds
+ F
dp
W
=
A
ra
τ
a
+ A
ds
τ
ds
+ A
dp
τ
dp
W
, (15.29)
where W is the normal load, F is the friction force, A
ra
, A
ds
, A
dp
are the real areas of
contact during adhesion, two body deformationand with particles, respectively, and
τ is the shear strength. The subscripts a, ds, and dp correspond to adhesion, summit
deformation and particle deformation.
In the presence of meniscus, the friction force is given by
F = μ
(
W + F
m
)
, (15.30)
where F
m
is the meniscusforce [16].The coefficient offriction in thepresence of the
meniscus force, μ
wet
, is calculated using only the applied normal load, as normally
measured in the experiments [22]
μ
wet
= μ
1+
F
m
W
=
A
ra
τ
a
+ A
ds
τ
ds
+ A
dp
τ
dp
W
1+
F
m
W
. (15.31)
The (15.31) shows that μ
wet
is greater than μ, because F
m
is not taken into account
for calculation of the normal load in the wet contact.
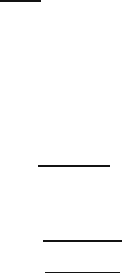
810 Bharat Bhushan and Michael Nosonovsky
It was shown by Greenwood and Williamson [42] and by subsequent modifi-
cations of their model, that for contacting surfaces with common statistical distri-
butions of asperity heights, the real area of contact is almost linearly proportional
to the normal load. This linear dependence, along with (15.29), result in linear de-
pendence of the friction force on the normal load, or coefficient of friction being
independent of the normal load. For a review of the numerical analysis of rough
surface contacts, see Bhushan [43,44] and Bhushan and Peng [45]. The statistical
and numerical theories of contact involve roughness parameters – e.g. the standard
deviation of asperity heights and the correlation length [16]. The roughness par-
ameters are scale dependent. In contrast to this, the theory of self-similar (fractal)
surfaces solid contact developed by Majumdar and Bhushan [46] does not include
length parameters and are scale-invariantin principle. The shear strength of the con-
tacts in (15.29) is also scale dependent. In addition to the adhesional contribution
to friction, elastic and plastic deformation on nano- to macroscale contributes to
friction [16]. The deformations are also scale dependent.
15.5.1 Adhesional Friction
The adhesional component of friction depends on the real area of contact and adhe-
sion shear strength. Here we derive expressions for scale dependence of adhesional
friction during single-asperity and multiple-asperity contacts.
Single-Asperity Contact
The scale length during single-asperity contact is the nominal contact length, which
is equal to the contact diameter 2a. In the case of predominantlyelastic contacts, the
real area of contact A
re
depends on the load according to the Hertz analysis [47]
A
re
= πa
2
, (15.32)
and
a =
3WR
4E
∗
1/3
, (15.33)
where R is effective radius of curvature of summit tips, and E
∗
is the effective elastic
modulusof the twobodies. In the case ofpredominantlyplastic contact,the real area
of contact A
rp
is given by (15.21), whereas the hardness is given by (15.10).
Combining (15.10), (15.13), (15.29), and (15.32), the adhesional component of
the coefficient of friction can be determined for the predominantly elastic contact as
μ
ae
= μ
ae0
1+ (l
s
/a) (15.34)
and for the predominantly plastic contact as
μ
ap
= μ
ap0
1+ (l
s
/a)
1+ (l
d
/a)
, (15.35)
where μ
ae0
and μ
ap0
are corresponding values at the macroscale [20].
15 Scale Effect in Mechanical Properties and Tribology 811
3
2
1
0
0123
3
2
1
0
0123
1
0
2
1
0
0 0.5 1
0 0.5 1
Single asperity elastic contact
Coefficient of friction μ
e
/μ
e0
a/l
s
Single asperity plastic contact
Coefficient of friction μ
p
/μ
p0
a/l
s
l
d
/l
s
= 0.25
l
d
/l
s
= 5
a)
b)
Multiple-asperity elastic contact
Coefficient of friction μ
e
/μ
e0
L/L
lc
L
S
/L
lc
= 1000
L
S
/L
lc
= 1
L
S
/L
lc
= 0
Multiple-asperity plastic contact
Coefficient of friction μ
p
/μ
p0
L/L
lc
L
d
/L
lc
= 1
L
d
/L
lc
= 1000
L
d
/L
s
= 0.25
L
d
/L
s
= 5
Fig. 15.12a,b. Normalized
results for the adhesional
component of the coefficient
of friction, as a function of
scale (a/l
s
for single asperity
contact and L/L
lc
for multi-
asperity contact). (a)Inthe
case of single asperity plastic
contact, data are presented
for two values of l
d
/l
s
.(b)In
the case of multi-asperity
contact, data are presented
for m = 0.5, n = 0.2. For
multi-asperity elastic contact,
data are presented for three
values of L
S
/L
lc
. For multi-
asperity plastic contact, data
are presented for two values
of L
d
/L
s
The scale dependence of adhesional friction in single-asperity contact is pre-
sented in Fig. 15.12a. In the case of single asperity elastic contact, the coefficient of
friction increases with decreasing scale (contact diameter), because of an increase
in the adhesion strength, according to (15.34). In the case of single asperity plastic
contact, the coefficient of friction can increase or decrease with decreasing scale,
because of an increased hardness or increase in adhesional strength. The compe-
tition of these two factors is governed by l
d
/l
s
, according to (15.35). There is no
direct way to measure l
d
and l
s
. We will see later, from experimental data, that the
coefficient of friction tends to decrease with decreasing scale, therefore, it must be
assumed that l
d
/l
s
> 1 for the data reported in the paper [20].
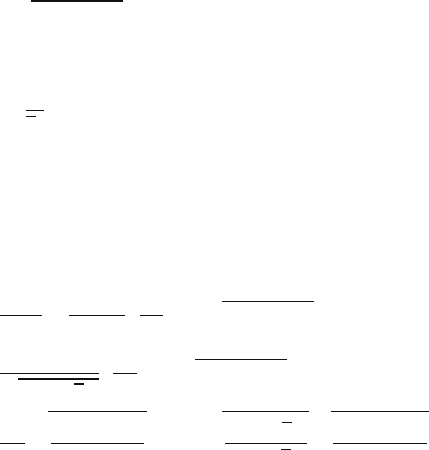
812 Bharat Bhushan and Michael Nosonovsky
Multiple-Asperity Contact
The adhesional component of friction depends on the real area of contact and adhe-
sion shear strength. Scale dependence of the real area of contact was considered in
the preceding section. Here we derive expressionsfor scale-dependence of the shear
strength at the interface during adhesional friction. It is suggested by Bhushan and
Nosonovsky [20] that, for many materials, dislocation-assisted sliding (microslip) is
the main mechanism, which is responsible for the shear strength. They considered
dislocation assisted sliding based on the assumption, that contributing dislocations
are located in a subsurface volume. The thickness of this volume is limited by the
distance which dislocations can climb l
s
(material parameter) and by the radius of
contact a. They showed that τ
a
is scale dependent according to (15.13). Assuming
the contact radii equal to the mean value given by (15.19)
τ
a
= τ
a0
1+
(
L
s
/L
)
m
, L < L
lc
, (15.36)
where
L
s
= L
lc
l
s
a
0
1/m
. (15.37)
In the case of absence of the microslip (e.g., for an amorphous material), it should
be assumed in (15.34–15.36), L
s
= l
s
= 0.
Based on (15.9, 15.17,15.24, 15.29, 15.36,15.37), the adhesional componentof
the coefficient of friction in the case of elastic contact μ
ae
and in the case of plastic
contact μ
ap
, is given as [20]
μ
ae
=
τ
a
A
re
W
=
τ
a0
A
re0
W
L
L
lc
m−n
1+
(
L
s
/L
)
m
=
μ
ae0
1+ (l
s
/a
0
)
L
L
lc
m−n
1+
(
L
s
/L
)
m
, L < L
lc
; (15.38)
μ
ap
=
τ
a0
H
0
1+
(
L
s
/L
)
m
1+
(
L
d
/L
)
m
= μ
ap0
1+ (l
d
/a
0
)
1+ (l
s
/a
0
)
1+
(
L
s
/L
)
m
1+
(
L
d
/L
)
m
, L < L
lc
,
(15.39)
where μ
ae0
and μ
ap0
are values of the coefficient of friction at macroscale (L ≥ L
lc
).
The scale dependence of adhesional friction in multiple-asperity elastic contact
is presented in Fig. 15.12b, which is based on (15.38), for various values of L
s
/L
lc
.
The change of scale length L affects the coefficient of friction in two different ways:
through the change of A
re
(15.17) and τ
a
(15.36) below L
lc
.Further,τ
a
is controlled
by the ratio L
s
/L. Based on (15.36), for small ratio of L
s
/L
lc
, scale effects on τ
a
is insignificant for L/L
lc
> 0. As it is seen from Fig. 15.12b by comparison of the
curve with L
s
/L
lc
= 0(insignificantscale effect on τ
a
), L
s
/L
lc
= 1,and L
s
/L
lc
= 1000
(significant scale effect on τ
a
), the results for the normalized coefficient of friction
are close, thus, the main contribution to the scaling effect is due to change of A
re
.

15 Scale Effect in Mechanical Properties and Tribology 813
Table 15.1. Scaling factors for the coefficient of adhesional friction [20]
Single asperity Single asperity Multiple-asperity Multiple-asperity
elastic contact plastic contact elastic contact plastic contact
μ
e
= μ
e
= μ
e
= μ
p
=
μ
e0
√
1+ (l
s
/a) μ
e0
!
1+(l
s
/a)
1+(l
d
/a)
μ
e0
C
E
L
m−n
μ
p0
C
P
!
1+
(
L
s
/L
)
m
1+
(
L
d
/L
)
m
×
1+
(
L
s
/L
)
m
In the case of multiple-asperity plastic contact, the results, based on (15.39), are
presented in Fig. 15.12b for L
d
/L
s
= 0.25, L
d
/L
s
= 5andL
d
/L
lc
= 1andL
d
/L
lc
=
1000.The changeof scaleaffects the coefficientof friction throughthe changeof A
rp
(15.34), which is controlled by L
d
,andτ
a
(15.36), which is controlled by L
s
. It can
be observed from Fig. 15.12b, that for L
d
> L
s
, the change of A
rp
prevails over
the change of τ
a
, with decreasing scale, and the coefficient of friction decreases.
For L
d
< L
s
, the change of τ
a
prevails, with decreasing scale, and the coefficient
of friction increases [20]. Expressions for the coefficient of adhesional friction are
presented in Table 15.1.
15.5.2 Two-Body Deformation
Based on the assumption that multiple asperities of two rough surfaces in contact
have conical shape, the two-body deformation component of friction can be deter-
mined as
μ
ds
=
2tanθ
r
π
, (15.40)
where θ
r
is theroughnessangle (or attackangle) of aconical asperity[16,48].Mech-
anical properties affect the real area of contact and shear strength and these cancel
out in (15.29).
The roughness angle is scale-dependent and is related to the roughness param-
eters [41]. Based on statistical analysis of a random Gaussian surface,
tanθ
r
∝
σ
β
∗
. (15.41)
From (15.40) it can be interpreted that stretching the rough surface in the vertical
direction (increasing vertical scale parameter σ) increases tanθ
r
, and stretching in
the horizontal direction (increasing vertical scale parameter β
∗
) decreases tanθ
r
.
Using (15.40) and (15.41), the scale dependence of the two-body deformation
component of the coefficient of friction is given as [21]
μ
ds
=
2σ
0
πβ
∗
L
L
lc
n−m
= μ
ds0
L
L
lc
n−m
, L < L
lc
, (15.42)
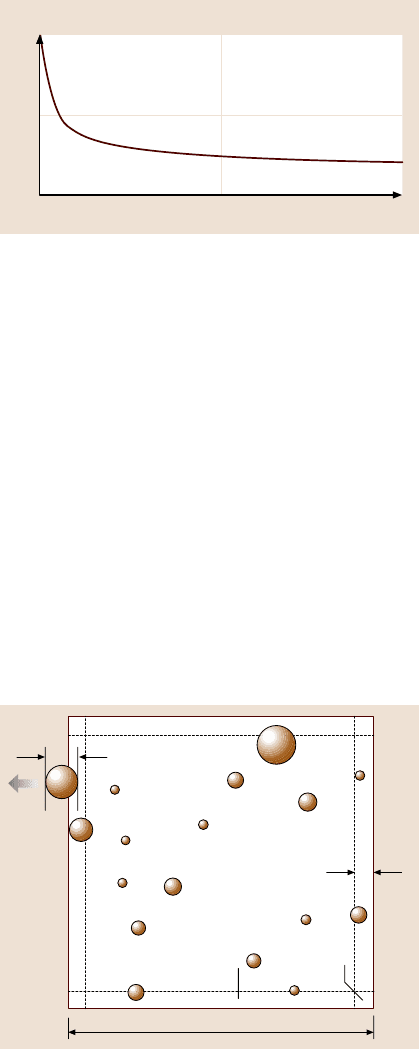
814 Bharat Bhushan and Michael Nosonovsky
5
2.5
0
0 0.5 1
Asperities plowing contribution
L/L
lc
μ
ds
/μ
ds0
m = 0.5
n = 0.2
Fig. 15.13. Normalized re-
sults for the two-body de-
formation component of the
coefficient of friction
where μ
ds0
is the value of the coefficient of summits deformation component of the
coefficient of friction at macroscale (L ≥ L
lc
).
The scale dependence for the two-body deformation component of the coeffi-
cient of friction is presented in Fig. 15.13 for m = 0.5, n = 0.2. The coefficient of
friction increases with decreasing scale, according to (15.42).This effect is a conse-
quence of increasing average slope or roughness angle.
15.5.3 Three-Body Deformation Friction
In this sections of the paper, size distribution of particles will be idealized according
to the exponential, normal, and log normal density functions, since these distribu-
tions are the most common in nature and industrial applications (see Sect. 15.A).
The probability for a particle of a given size to be trapped at the interface depends
on the size of the contact region. Particles at the edge of the region of contact are
likely to leave the contact area, whereas those in the middle are likely to be trapped.
The ratio of the edge region area to the total apparent area of contact increases
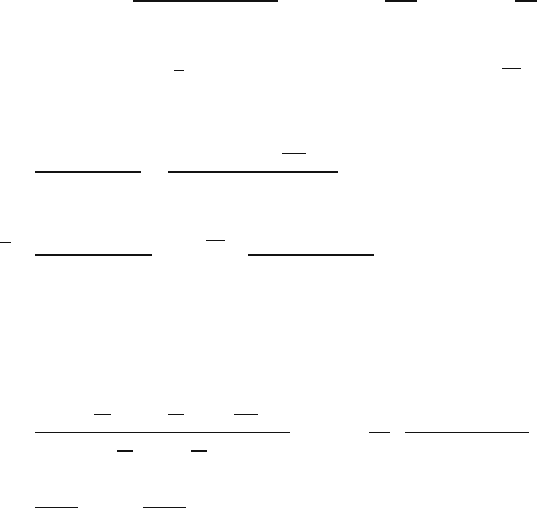
L
Contact region
d
d/2
Border region Corner
Fig. 15.14. Schematics of
debris at the contact zone and
at its border region. A particle
of diameter d in the border
region of d/2islikelytoleave
the contact zone
15 Scale Effect in Mechanical Properties and Tribology 815
with decreasing scale size. Therefore, the probability for a particle to be trapped
decreases, as well as the three-body component of the coefficient of friction [21].
Let us consider a square region of contact of two rough surfaces with a length L
(relevant scale length), with the density of debris of η particles per unit area
(Fig. 15.14). We assume that the particles have the spherical form and that p(d)is
the probability density function of particles size. It is also assumed that, for a given
diameter, particles at the border region of the contact zone of the width d/2are
likely to leave the contact zone, with a certain probability α, whereasparticles at the
center of the contact regionare likely to be trapped. It should be noted, that particles
in the corners of the contact region can leave in two different directions, therefore,
for them the probability to leave is 2α. The total nominal contact area is equal to L
2
,
the area of the border region, without the corners, is equal to 4(L−d)d/2, and the
area of the corners is equal to d
2
.
The probability density of size distribution for the trapped particles p
tr
(d) can
be calculated by multiplying p(d) by one minus the probability of a particle with
diameter d to leave; the later is equal to the ratioof the borderregionarea, multiplied
by a corresponding probability of the particle to leave, divided by the total contact
area [21]
p
tr
(d) = p(d)
1−
2α(L−d)d+ 2αd
2
L
2
= p(d)
1−
2αd
L
, d <
L
2α
. (15.43)
The ratio of the number of trapped particles to the total number of particles, average
radiusof a trapped particle
d,and averagesquareof trappedparticlesd
2
, asfunctions
of L, can be calculated as
n
tr
=
1
L/2
0
p
tr
(d)dd
1
∞
0
p(d)dd
=
1
L/2
0
p(d)
1−
2αd
L
dd
1
∞
0
p(d)dd
,
d =
1
L/2
0
dp
tr
(d)dd
1
L/2
0
p
tr
(d)dd
,
d
2
=
1
L/2
0
d
2
p
tr
(d)dd
1
L/2
0
p
tr
(d)dd
. (15.44)
Let us assume an exponential distribution of particles’ size (15.A7) with d
e
= 0.
Substituting (15.A7) into (15.44) and integrating yields for the ratio of trapped par-
ticles [21]
n
tr
=
1
L/(α2)
0
1
σ
e
exp
−
d
σ
e
1−
2αd
L
dd
1
∞
0
1
σ
e
exp
−
d
σ
e
dd
= exp
−
d
σ
e
σ
e
−L/(2α)+ d
L/(2α)
"
"
"
"
"
"
L/(2α)
0
=
2ασ
e
L
exp
−
L
2ασ
e
−1
+ 1 (15.45)
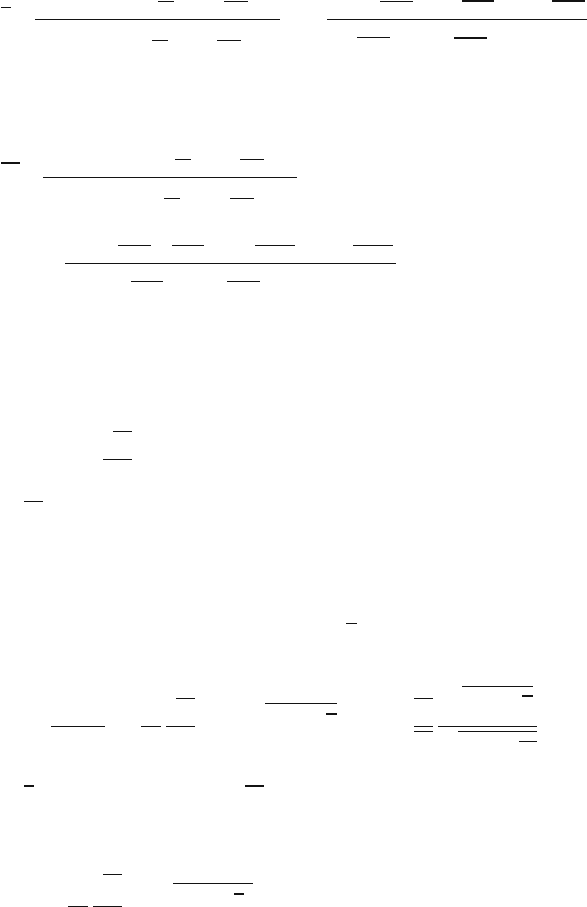
816 Bharat Bhushan and Michael Nosonovsky
whereas the mean diameter of the trapped particles is
d =
1
L/(2α)
0
dexp
−
d
σ
e
1−
2αd
L
dd
1
L/(2α)
0
exp
−
d
σ
e
1−
2αd
L
dd
= σ
e
exp
−
L
2ασ
e
1+
4ασ
e
L
+ 1−
4ασ
e
L
2ασ
e
L
exp
−
L
2ασ
e
−1
+ 1
(15.46)
and the mean square radius of the trapped particles is
d
2
=
1
L/(2α)
0
d
2
exp
−
d
σ
e
1−
2αd
L
dd
1
L/(2α)
0
exp
−
d
σ
e
1−
2αd
L
dd
= σ
2
e
exp
−
L
2ασ
e
L
2ασ
e
+ 4+
12ασ
e
L
+ 2−
12ασ
e
L
2ασ
e
L
exp
−
L
2ασ
e
−1
+ 1
. (15.47)
For the normal and log normal distributions, similar calculations can be conducted
numerically.
The area, supported by particles can be found as the number of trapped particles
ηL
2
n
tr
multiplied by average particle contact area
A
dp
= ηL
2
n
tr
πd
2
4
, (15.48)
where
d
2
is mean square of particle diameter, η is particle density per apparent area
of contact (L
2
)andn
tr
is a number of trapped particles divided by the total number
of particles [21].
The plowing deformation is plastic and, assuming that particles are harder than
the bodies, the shear strength τ
dp
is equal to the shear yield strength of the softer
body τ
Y
which is given by the (15.9) with a = d/2. Combining (15.29) with (15.9)
and (15.48)
μ
dp
=
A
dp
τ
dp
W
= η
L
2
W
π
d
2
4
n
tr
τ
Y0
!
1+ 2l
d
/d = μ
dp0
n
tr
d
2
d
2
0
!
1+ 2l
d
/d
!
1+ 2l
d
/d
0
, (15.49)
where
d is mean particle diameter,d
0
is the macroscale value of mean particle diam-
eter, and μ
dp0
is macroscale (L →∞, n
tr
→ 1) value of the third-body deformation
component of the coefficient of friction given as
μ
dp0
= η
L
2
W
π
d
2
0
4
τ
Y0
!
1+ 2l
d
/d
0
. (15.50)
Scale dependence of the three-body deformation component of the coefficient of
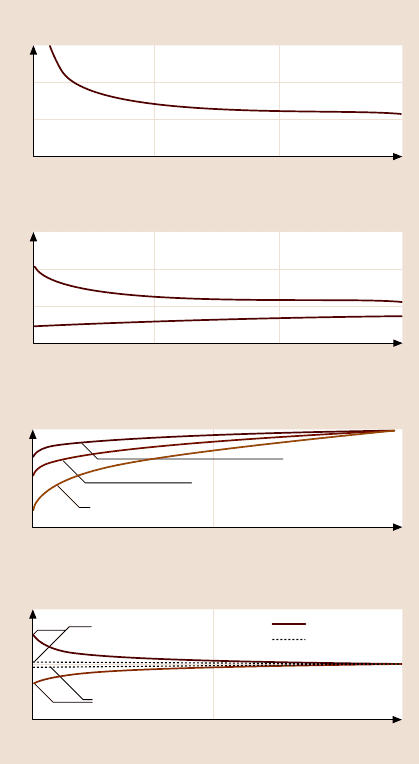
friction is presented in Fig. 15.15, based on (15.49). The number of trapped parti-
cles divided by the total number of particles, as well as the three-body deformation
15 Scale Effect in Mechanical Properties and Tribology 817
1
0.5
0
020406080
1
0.5
0
020406080
1
0.5
0
10
0
10
2
10
4
10
6
Three body plowing contribution
Exponential distribution
Fraction of trapped
particles
Coefficient of friction
L/(ασ)
n
tr
and μ
dp
/μ
dp0
Fraction of trapped
particles
Coefficient of friction
n
tr
and μ
dp
/μ
dp0
Normal distribution
L/(ασ)
d
n
= 2σ
l
d
/σ = 1
d
e
= 0
l
d
/σ
e
= 1
Fraction of trapped particles
Coefficient of friction
n
tr
and μ
dp
/μ
dp0
Log normal distribution
L/α (nm)
ln(d
0
) = 2
σ
ln
= 1
l
d
/σ = 1
Fig. 15.15. The number of
trapped particles divided by
the total number of particles
and three-body deformation
component of the coefficient
of friction, normalized by
the macroscale value, for
three different distributions of
debris size: (a) exponential,
(b) normal, and (c) log-
normal distributions
component of the coefficient of friction, are presented as a function of scale size
divided by α for the exponential, normal, and log normal distributions. The depen-
dence of μ
d
/μ
d0
is shown as a function of L/(ασ
e
) for the exponential distribution
and normal distribution, for d
n
= d
e
= 2σ
e
and l
d
/σ
e
= 1, whereas for the log normal
distribution the results are presented as a function of L/α,for(lnd
ln
) = 2, σ
ln
= 1,
and l
d
/σ
ln
= 1. This component of the coefficient of friction decreases for all of
the three distributions. The results are shown for l
d
/σ
ln
= 1, however, variation of
l
d
/σ
ln
in the range between 0.1 and 10 does not change significantly the shape of
the curve.The decrease of the three-bodydeformationfriction forcewith decreasing
scale results with this componentbeing small at the nanoscale.
15.5.4 Ratchet Mechanism
Surface roughness can have an appreciable influence on friction during adhesion.
If one of the contacting surfaces has asperities of much smaller lateral size, such
