Bhushan B. Nanotribology and Nanomechanics: An Introduction

Подождите немного. Документ загружается.

13 Computer Simulations of Nanometer-Scale Indentation and Friction 667

(nN)

60

20

40

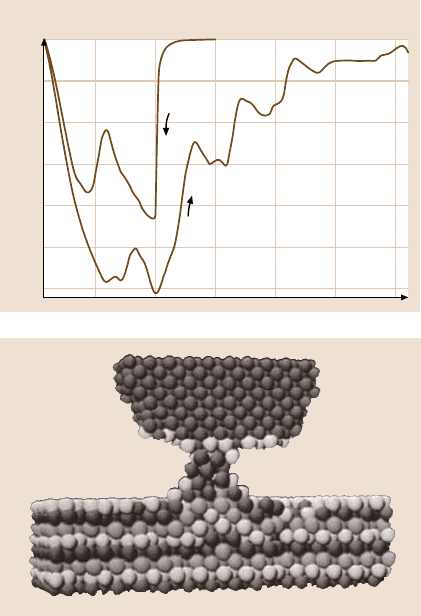
Fig. 13.3. Computationally

derived force F

versus tip-

to-sample distance d

curves

for approach, contact, inden-

tation, then separation using

the same tip–sample system

shown in Fig. 13.4. These

data were calculated from an

MD simulation. After [111]

with the permission of the

AAAS (1990)

Fig. 13.4. Illustration of

atoms in the MD simulat-

ion of a Ni tip being pulled

back from an Au substrate.

This causes the formation of

a connective neck of atoms

between the tip and the sur-

face. After [111] with the

permission of the AAAS

(1990)

occurs increases, as does the contact area between the tip and the surface and the

amount of nanowire elongation prior to breakage.

Simulations by Komvopoulos and Yan [122] using LJ potentials showed how

metallic surfaces respond to single and repeated indentation by metallic, or cova-

lently bound, rigid tips. The simulations predicted that a single indentation event

produces hysteresis in the force curve as a result of surface plastic deformation

and heating. The repulsive force decreases abruptly during surface penetration by

the tip and surface plastic deformation. Repeated indentation results in the continu-

ous decrease of the elastic stiﬀness, surface heating, and mean contact pressures at

maximum penetration depths to produce behaviors that are similar to cyclic work

hardening and softening by annealing observed in metals at the macroscale.

When the tip is much stiﬀer than the surface,pile-up of surface atoms aroundthe

tip occurs to relieve the stresses induced by nanoindentation. In contrast, when the

surface is much stiﬀer than the tip, the tip can be damaged or destroyed. Simulat-

ions by Belak et al. [123] using perfectly rigid tips showed the mechanism by which

the surface yields plastically after its elastic threshold is exceeded. The simulations

showed how nanoindentation causes surface atoms to move on to the surface but

668 Susan B. Sinnott et al.

under the tip and thus cause atomic pile-up. In this study, variations in the inden-

tation rate reveal that point defects created as a result of nanoindentation relax by

moving through the surface if the rate of indentation is slow enough. If the indenta-

tion rate is too high, there is no time for the point defects to relax and move away

from the indentation area and so strain builds up more rapidly. The rigid indenters

considered in these MD simulations are analogous to experiments that use surface

passivation to prevent JC between the tip and the surface [120,124], the results of

which agree with the predicted results of pile-up and crater formation, as shown in

Fig. 13.5 [120].

In short, MD simulations are able to explain the atomic-scale mechanisms be-

hind measured experimentalforce curves producedwhen metal tips indent homoge-

neousmetal surfacesto nanometer-scaledepths. Thispreliminary workhas spawned

much of the current interest in using the JC to produce metal nanowires [126–128].

MD simulations have also been used to examine the relationship between

nanoindentation and surface structure. This is most apparent in a series of com-

putational studies that consider the indentation of a surface with a “virtual” hard-

sphere indenter in a manner that is independent of the rate of indentation, as shown

in Fig. 13.6. The virtual indenter is modeled through the application of a repulsive

force to the surface rather than through the presence of an actual atomic tip. Kelch-

ner et al. [129], rather than use MD, pushed the indenter against the surface a short

distance and then allowed the system to relax using standard energy minimization

methods in combination with EAM potentials. The system is fully relaxed when the

energy of the surface system is minimized. After relaxation,the tip is pushed further

into the surface and the process is repeated. As the tip generates more stresses in the

surface,dislocationsare generated andplastic deformationoccurs. If thetip is pulled

back after indenting less than a speciﬁc critical value, the atoms that were plastically

deformed are healed during the retraction and the surface recoversits original struc-

ture. In contrast, if the tip is indented past the critical depth, additional dislocations

1ìm

100nm

Before

1ìm

100nm

After

1ìm

Fig. 13.5. Images of a gold surface before and after being indented with a pyramidal shaped

diamond tip in air. The indentation created a surface crater. Note the pile-up around the crater

edges. After [120] with the permission of Elsevier (1993)

13 Computer Simulations of Nanometer-Scale Indentation and Friction 669

Free surface

Fixed

B.C.

Fixed

B.C.

Fixed B.C.

Free surface

Indenter

Fig. 13.6. A schematic of a spherical, virtual tip indenting a metal surface. After [125] with

the permission of Elsevier (1993)

are created that interfere with the surface healing process on tip withdrawal. In this

case, a surface crater is left on the surface following nanoindentation.

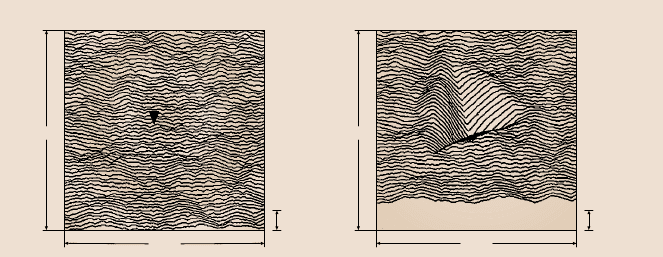
A similar study by Lilleodden et al. [125] considered the generation of disloca-

tions in perfect crystals and near grain boundaries in gold. Analysis of the relation-

ship between the load and the tip displacement in the perfect crystal shows discrete

load drops followed by elastic behavior. These load drops are shown to correspond

to the homogeneous nucleation of dislocations, as illustrated in Fig. 13.7, which

is a snapshot taken just after the load drop. When nanoindentation occurs close to

a grain boundary, similar relationships between the load and tip displacement are

predicted to occur as were seen for the perfect crystal. However, the dislocations

responsible for the load drop are preferentially emitted from the grain boundaries,

as illustrated in Fig. 13.8.

Simulations can also show how atomic structure and stresses are aﬀected by

nanoindentation.For instance, MD simulations with a virtual indenter by Hasnaoui

Fig. 13.7. Snapshot of two

partial dislocations separated

by a stacking fault. The dark

spheres in the center of the

structure indicate atoms in

perfect crystal positions after

both partial dislocations have

passed. After [125] with the

permission of Elsevier (1993)

670 Susan B. Sinnott et al.

Fig. 13.8. Snapshot of the

high-energy atoms only after

a load drop caused by dislo-

cation generation during the

nanoindentation of gold near

a grain boundary. After [125]

with the permission of Else-

vier (1993)

et al. [130] using semi-empirical tight-binding methods showed the interaction be-

tween the grain boundaries under the indenter and the dislocations generated by the

indentation, as illustrated in Fig. 13.9. This study shows that if the size of the inden-

ter is smaller than the grain size, the grain boundaries can emit, absorb, and reﬂect

the dislocations in a manner that depends on atomic structure and the distribution of

stresses.

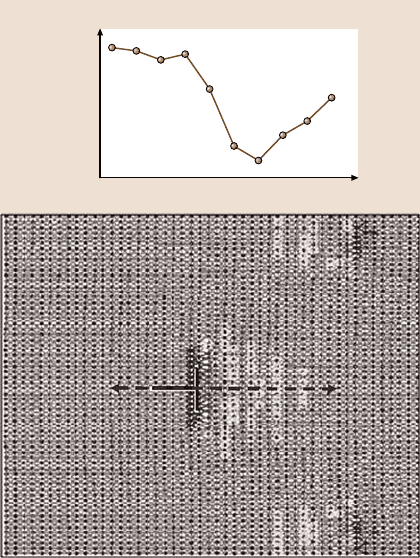
Zimmerman et al. considered the indentation of a single-crystal gold substrate

both near and far from a surface step [131]. The results of these simulations, which

used EAM potentials, showed that the onset of plastic deformation depends to a sig-

niﬁcant degree on the distance of indentation from the step, and whether the in-

dentation is on the plane above or below the step. In a related set of simulations,

Shenderova et al. [132] examined whether ultrashallow elastic nanoindentation can

nondestructivelyprobe surfacestress distributions associated with surface structures

such as a trench and a dislocation intersecting a surface. The simulations carried out

the nanoindentation to a constant depth. They predicted maximum loads that reﬂect

the in-plane stresses at the point of contact between the indenter and the substrate,

as illustrated in Fig. 13.10.

Sincethe 1930s,studies havebeen performedusing hardnessmeasurementtech-

niques [133–136] and indentation methods [137] that suggest that the hardness of

a material depends on applied in-plane uni- and bi-axial strain. In general, tensile

strain appeared to decrease hardness while increases in hardness under compres-

sive in-plane strain were reported. This behavior had traditionally been attributed

to the contribution of stresses from the local strain from the indentation to the re-

solved shear stresses and the in-plane strain [134, 136]. However, in 1996, Pharr

and coworkers determined that changes in elastic modulus determined from un-

loading curves of strained substrates using contact areas estimated via an elastic

model are too large to have physical signiﬁcance, a result that brought into ques-

tion the interpretation of prior hardness data [137, 138]. They hypothesized that

13 Computer Simulations of Nanometer-Scale Indentation and Friction 671

1.7 GPa

a) d)

0.5 GPa

Fig. 13.9a–f. Snapshots showing the atomic stress distribution and atomic structures in a gold

surface. Figures (a)–(c) show the atomic structure at indentation depths of 7.9, 8.6, and 9.6Å,

respectively, with a virtual spherical indenter. A dislocation is represented by the two parallel

{111} planes (two dark lines) that show the stacking fault left behind after the leading par-

tial dislocation has passed. Figures (d)–(f) show the atomic stress distribution of the same

system at the same indentation depths. Here the dark color indicates compressive hydrostatic

pressures of 1.7 GPa and higher while the gray color indicates tensile pressures of −0.5GPa

and lower. The arrow in (d) shows the region of the system where a dislocation interacts with

a grain boundary. After [130] with the permission of Elsevier (2004)

the apparent change in modulus (and hardness) with in-plane strain is mainly due

to changes in contact area that are not typically taken into consideration in elas-

tic half-space models. This hypothesis was based on experimental nanoindentation

studies of a strained polycrystalline aluminium alloy and ﬁnite element calculations

on an isotropic solid [137, 138]. They further suggested that in-plane compression

increases pile-up around the indenter that, when not taken into accountin the analy-

sis of unloading curves, implies a nonphysical increase in modulus. Likewise, they

suggested that in-plane tensile strain reduces the amount of material that is piled up

around an indenter, which leads to a corresponding reduced (nonphysical) modulus

when interpreting unloading curves using elastic models.

To explore in more detail the issue of pile-up and its inﬂuence on the interpre-

tation of loading curves, Schall and Brenner used MD simulations and EAM po-

tentials to model the plastic nanoindentation of a single-crystal gold surface under

an applied in-plane strain [139]. These simulations predicted that the mean pres-

sure, calculated from true contact areas that take into account plastic pile-up around

the indenter, varies only slightly with applied pre-stress. They also predicted that

the higher values occur in compression rather than in tension, and that the modu-

672 Susan B. Sinnott et al.

Compression Tension

Maximum load

Fig. 13.10. Data and system

illustration from a simulation

of a gold surface containing

a dislocation. Top:Maximum

load for simulated shallow

indentation at several points

along the dotted line in the

bottom illustration. Bottom:

Top view of the simulated

surface. The dislocation is

denoted by the solid black

lines

lus calculated from the true contact area is essentially independent of the pre-stress

level in the substrate. In contrast, if the contact area is estimated from approximate

elastic formulae, the contact area is underestimated, which leads to a strong, incor-

rect dependence of apparent modulus on the pre-stress level. The simulations also

showed larger pile-up in compression than in tension, in agreement with the Pharr

model, and both regimesproduced contact areas larger than those typically assumed

in elastic analyses. These ﬁndings are illustrated in Fig. 13.11.

Nanometer-scale indentation of ceramic systems has also been investigatedwith

MD simulations. Ceramics are stiﬀer and more brittle than metals at the macroscale

and examining the nanoindentation of ceramic surfaces provides information about

the nanometer-scale properties. They also reveal the manner by which defects form

in covalent and ionic materials. For example, Landman et al. [110,140] considered

the interaction of a CaF

tip with a CaF

substrate in MD simulations using em-

pirical potentials. As the tip approaches the surface, the attractive force between

them steadily increases. This attractive force increases dramatically at the critical

distance of 2.3Å as the interlayer spacing of the tip increases (the tip is elongated)

in a process that is similar to the JC phenomenon observed in metals. An important

diﬀerence, however, is the amount of elongation, which is 0.35Å in the case of the

ionic ceramics and several angstroms in the case of metals. As the distance between

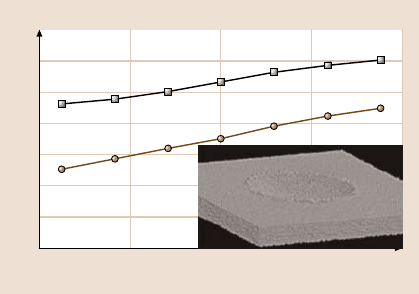
13 Computer Simulations of Nanometer-Scale Indentation and Friction 673

1

Relative stress

Area (nm

)

110

10.5 0 0.5

Fig. 13.11. Contact area projected in the plane at a maximum load for simulated indention

of a gold surface as a function of in-plane biaxial stress. The stress is normalized to the

theoretical yield stress. The top curve is from an atomistic simulation; the bottom curve is

from an elastic model. Inset: Illustration of the region near the indention from the simulation.

The tip is not shown for clarity. Initial formation of pile-up around the edge of the indentation

is apparent

the tip and the surface decreases further, the attractive nature of their interaction

increases until a maximum value is reached. Indentation beyond this point results

in a repulsive tip–substrate interaction, compression of the tip, and ionic bonding

between the tip and substrate. These bonds are responsible for the hysteresis pre-

dicted to occur in the force curve on retraction, which ultimately leads to plastic

deformation of the tip followed by fracture.

The responses of covalently bound ceramics such as diamond and silicon to

nanoindentation have been heavily studied with MD simulations. One of the ﬁrst

of these computational studies was carried out by Kallman et al. who used the

Stillinger–Weber potential to examine the indentation of amorphous and crystalline

silicon [141]. The motivation for this study came from experimental data that indi-

cated a large change in electrical resistivity during indentation of silicon, which led

to the suggestion of a load-inducedphase transition below the indenter. Clarke et al.,

for example, reportedforming an Ohmic contact under load, and using transmission

electron microscopy they observed an amorphousphase at the point of contact after

indentation [142]. Using micro-Raman microscopy, Kailer et al. identiﬁed a metal-

lic β-Sn phase in silicon near the interface of a diamond indenter during hardness

loading [143]. Furthermore, upon rapid unloading they detected amorphous silicon

as in the Clarke et al. [142] experiments, while slow unloading resulted in a mixture

of high-pressure polymorphs near the indent point. At the highest indentation rate

and the lowest temperature, the simulations by Kallman et al. [141] showed that

amorphous and crystalline silicon have similar yield strengths of 138 and 179 kbar,

respectively. In contrast, at temperatures near the melting temperature and at the

slowest indentation rate, both amorphous and crystalline silicon are predicted to

674 Susan B. Sinnott et al.

have lower yield strengths of 30kbar. The simulations thus show how the predicted

yield strength of silicon at the nanometer scale depends on structure, rate of defor-

mation, and surface temperature.

Interestingly, Kallman et al. [141] found that amorphous silicon does not crys-

tallize upon indentation, but indentation of crystalline silicon at temperatures near

the melting point transforms the surface structure near the indenter to the amor-

phous phase. The simulations do not predict transformation to the β-Sn structure

under any of the conditions considered. These results agree with the outcomes of

scratching experiments [144] that showed that amorphous silicon emerges from

room-temperature scratching of crystalline silicon.

Kaxiras and coworkers revisited the silicon nanoindentation issue using a quasi-

continuum model that couples interatomic forces from the Stillinger–Weber poten-

tial to a ﬁnite element grid [145]. They report good agreement between simulated

loading curves and experiment provided that the curves are scaled by the indenter

size. Rather than the β-Sn structure, however, atomic displacements suggest for-

mation of a metallic structure with ﬁvefold coordination below the indenter upon

loading, and a residual simple cubic phase near the indentation site after the load is

released rather than the mix of high-pressure phases characterized experimentally.

Smith et al. attribute this discrepancy to shortcomings of the Stillinger–Weber po-

tential in adequately describing the high-pressure phases of silicon. They also used

a simple model for changes in electrical resistivity with loading involving contribu-

tions from both a Schottky barrier and spreading resistance. Simulated resistance-

versus-loading curves agree well with experiment despite possible discrepancies

between the high-pressure phases under the indenter,suggesting that the salient fea-

tures of the experiment are not dependent on the details of the high-pressure phases

produced.

Additional MD simulations of the indentation of silicon were carried out by

Cheong and Zhang [146]. Their simulations provide further details about the phase

transformations that occur in silicon as a result of nanoindentation. In particular,

they ﬁnd that the diamond cubic silicon is transformed into a body-centered tetrag-

onal structure (β-Si) upon loading of the indenter, as illustrated in Fig. 13.12. Fig-

ure 13.13 shows that the coordination numbers of silicon atoms also coincide with

that of the theoreticalβ-Si structure. The body-centeredtetragonal structure is trans-

formed into amorphous silicon during the unloading stage. A second indentation

simulation again predicted that that this is a reversible process. Atomistic simulat-

ions by Sanz-Navarro et al. [147] shows the relation between the indentation of

silicon and the hydrostatic pressure on surface cells due to the nanoindentation, as

illustrated in Fig. 13.14. These simulations further predict that the transformation

of diamond silicon into the β-Si structure can occur if the hydrostatic pressure is

somewhat over 12GPa.

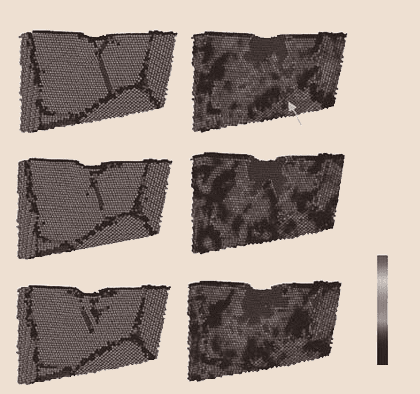
Multimillion atom simulations of the indentation of silicon nitride were recently

carried out by Walsh et al. [148]. The elastic modulus and hardness of the surface

was calculated using load–displacement relationships. Snapshots from the simu-

lations, illustrated in Fig. 13.15, show that pile-up occurs on the surface along the

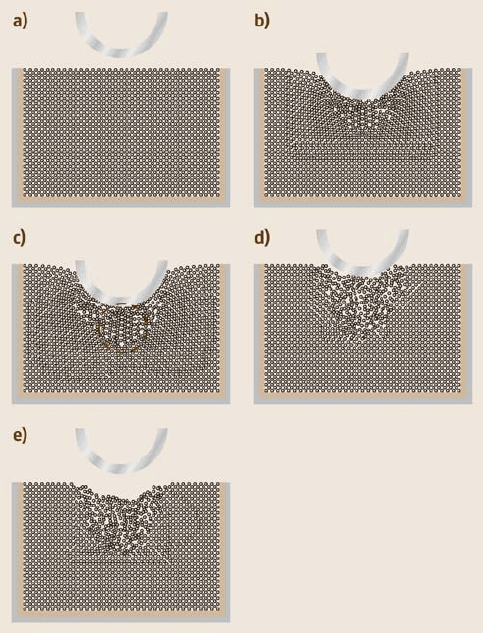
13 Computer Simulations of Nanometer-Scale Indentation and Friction 675

Fig. 13.12a–e. Snapshots of a silicon sample during indentation. The smaller dots are dia-

mond atoms. (a) Crystalline silicon prior to indentation. (b) Atoms beneath the indenter are

displaced as a result of indentation. (c) The system at maximum indentation. Some of the

atoms are in a crystalline arrangement (circled region)thatisdiﬀerent from the diamond

structure. (d) The surface structure is largely amorphous as the tip is withdrawn. (e)The

surface after indentation. Note the amorphous region at the site of the indentation process.

After [130] with the permission of the IOP (2000)

edges of the tip. Plastic deformationof the surface is predicted to extenda signiﬁcant

distance beyond the actual contact area of the indenter, as illustrated in Fig. 13.15.

The indentation of bare and hydrogen-terminated diamond (111) surfaces be-

yond the elastic limit was investigated by Harrison et al. [149] using a hydrogen-

terminated sp

-bonded tip in MD simulations that utilized bond-order potentials.

The simulations identiﬁed the depth and applied force at which the diamond (111)

substrate incurred plastic deformation due to indentation.At low indentationforces,

the tip–surface interaction is purely elastic, as illustrated in Fig. 13.16. This ﬁnd-

ing agrees with the ﬁndings of Cho et al. [150], who examined the atomic-scale

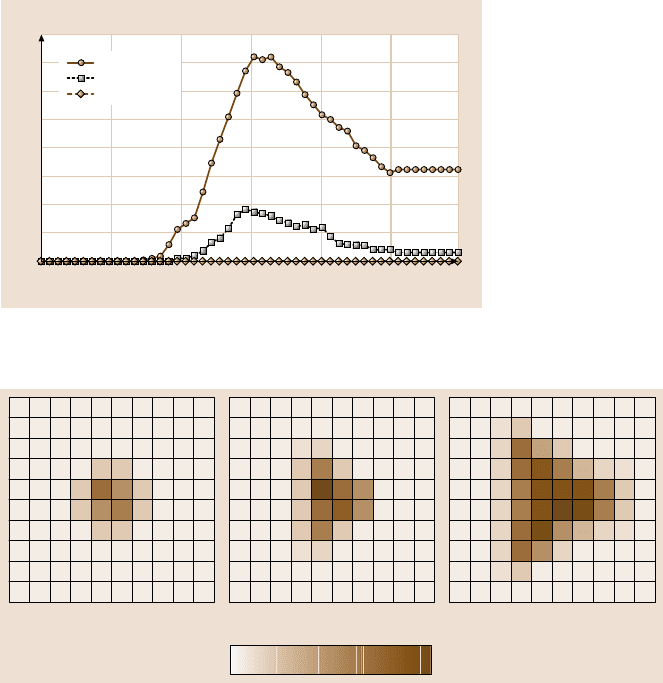
676 Susan B. Sinnott et al.

Time steps (× 100)

Number of atoms

100

200

300

400

500

600

700

800

490

90 170 250 330 410

6-coordinated

7-coordinated

8-coordinated

Fig. 13.13. The coordination of the silicon atoms shown in Fig. 13.12 as a function of time

during nanoindentation. After [146] with the permission of the IOP (2000)

Hydrostatic pressure (GPa)

184.5 9 13.5

a) b) c)

Fig. 13.14. Calculated hydrostatic pressure of surface cells at indentation depths of (a)8.9Å,

(b)15.7Å,and(c)25.3 Å. After [147] with the permission of the IOP (2000)

mechanical hysteresis experienced by an AFM tip indenting Si(100) with density

functional theory. The calculations predicted that at low rates it is possible to cycle

repeatedly between two buckled conﬁgurations of the surface without adhesion.

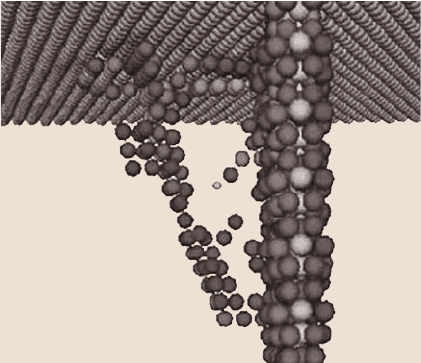
When the nanoindentation process of diamond (111) is plastic, connective

strings of atoms are formed between the tip and the surface, as illustrated in

Fig. 13.17. These strings break as the distance between the tip and crystal increases

and each break is accompanied by a sudden drop in the potential energy at large

positive values of tip–substrate separation. The simulations further predict that the

tip end twists to minimize interatomic repulsive interactions between the hydrogen

atoms on the surface and the tip. This behavior is predicted to lead to new covalent

bond formationbetween the tip and the carbon atoms below the ﬁrst layer of the sur-

face and connective strings of atoms between the tip and the surface when the tip is