Bhattacharjee J.K., Bhattacharyya S. Non-Linear Dynamics Near and Far from Equilibrium
Подождите немного. Документ загружается.


152 6 Systems Far from Equilibrium
It is possible for the above system to show the FDT if certain conditions are satisfied.
At the least these conditions require m
2
⊥
/m
2
=N
⊥
/N
. In general this would not
be valid for our system and so we cannot expect a FDT. For the system in the
absence of driving, m
2
→0 as the phase transition approaches. In the presence of
the drive, we have two mass terms m
2
⊥
and m
2
and the question is whether both
are involved or only one (in this case one needs to specify which one). If there is
a FDT, then both m
2
⊥
and m
2
will tend to zero at the transition. In the presence of
the field, we do not expect this to happen. In the direction of the field, there should
be an additional mass term, i.e. we do not expect the mass term to vanish at the
previous temperature but at a lower temperature. Thus m
2
> 0 at the transition. On
the other hand the transverse direction will be unaffected and we would have m
2
⊥
still vanishing at the transition. These arguments are at best heuristic but prompt
us to consider the situation
m
2
⊥
→0,m
2
> 0atT =T
0
(6.2.10)
Away from the transition, the correlations in our system are short ranged in the
absence of the external field. The signature of the driving field is to make the
correlation functions long ranged and power law like. We will try to show this
explicitly by working in the easiest of regimes T T
0
. In this range the fluctuations
φ are small and we can drop the nonlinear terms in the Langevin equation of
Eq.(6.2.3) which then becomes
∂φ
∂t
=
(m
2
⊥
−α
⊥
∇
2
⊥
)∇
2
⊥
φ +(m
2
−α
δ
2
)δ
2
φ
−2α∇
2
⊥
∂
2
φ
−(
∇
⊥
.
ξ
⊥
−∂ξ
) (6.2.11)
In Fourier space
−iωφ(k,ω) =−
(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
)k
2
+2αk
2
⊥
k
2
φ(k,ω)−i(
k
⊥
.
ξ
⊥
−k
ξ
) (6.2.12)
with the correlation of
ξ appropriately transformed into Fourier space. From
Eq.(6.2.12) we have
φ(k,ω)=
−i(
k
⊥
.
ξ
⊥
−k
ξ
)
−iω + [(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
+2αk
2
⊥
k
2
]
(6.2.13)
The correlation function
C(k,ω) =φ(k,ω)φ(−k, −ω)
=
N
⊥
k
2
⊥
+N
k
2
ω
2
+
2
[(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
+2αk
2
⊥
k
2
]
2
(6.2.14)
6.2 Ginzburg-Landau Model 153
The equal time correlation function is
C(k) =
dω
2π
C(k,ω)
=
N
⊥
k
2
⊥
+N
k
2
2[(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
+2αk
2
⊥
k
2
]
(6.2.15)
The interesting thing is the behaviour for small k. To find this, we can neglect the
k
4
terms in the denominator in Eq.(6.2.15)
C(k)=
N
⊥
k
2
⊥
+N
k
2
2(m
2
⊥
k
2
⊥
+m
2
k
2
)
(6.2.16)
The limit of k →0 can now be attained in two different ways
lim
k
⊥
→0
lim
k
→0
C(k)=
N
⊥
2m
2
⊥
(6.2.17)
and
lim
k
→0
lim
k
⊥
→0
C(k)=
N
2m
2
. (6.2.18)
If FDT holds, then the two limits are equal. In general though the two limits are
not equal and we will define
R =
N
⊥
m
2
N
m
2
⊥
(6.2.19)
as the quantity which is an index of the violation of the FDT. For R =1, FDT is
valid. The biggest violation occurs as T →T
c
.AtT
c
, τ
⊥
→0, while τ
is finite.
Thus R →∞as T →T
c
.
The quantity C(k) is the structure factor which can be probed experimentally
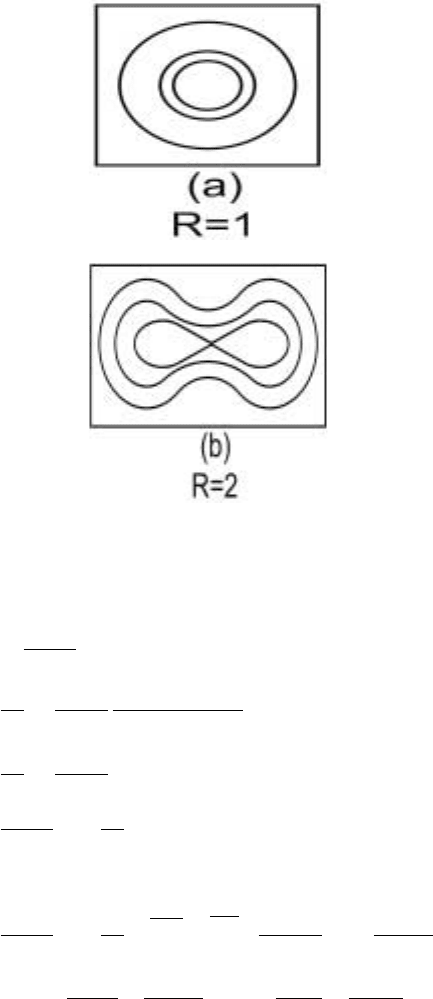
by light scattering. Consequently, it is interesting to look at the effect of R on C(k).
C(k) is a function of two independent variables k
⊥
and k
and it is best exhibited
as a contour plot in the k
⊥
−k
plane. In Fig. 6.2a, we show the contours for
R =1(N
⊥
=2,N
=1,m
2
⊥
=2,m
2
=1,α=1.5) and Fig. 6.2b for R =2(N
⊥
=
2,N
=1,m
2
⊥
=1,m
2
=1,α
⊥
=2,α
=1,α=1.5). For R =1, the contour has a
lobed structure.
154 6 Systems Far from Equilibrium
Figure 6.2. Structure factor as a function of R a) R=1 b) R=2
It is instructive to look at the correlation function in the coordinate space
C(x) =
d
D
k
(2π)
D
e
−i
k.x
C(k)
=
1
2
d
D
k
(2π)
D
N
⊥
k
2
⊥
+N
k
2
(m
2
⊥
k
2
⊥
+m
2
k
2
)
e
−i
k.x
=
1
2
d
D
k
(2π)
D
∞
0
ds(N
⊥
k
2
⊥
+N
k
2
)e
−i
k.x
e
−sm
2
⊥
k
2
⊥
e
−sm
2
k
2
=
1
(2π)
D
∞
0
dS
2
∞
−∞
dk
∞
−∞
D−1
i=1
(dk
⊥
)
i
(N
⊥
k
2
⊥
+N
k
2
)
×e
−i
k
⊥
. r
⊥
e
−ik
.r
e
−sm
2
⊥
k
2
⊥
e
−sm
2
k
2
=
1
(2π)
D
∞
0
dS
2
e
−
r
2
⊥
4Sm
2
⊥
e
−
r
2
4Sm
2
1
m
⊥
S
1/2
D−1
1
m
S
1/2
×
N
⊥
D −1
2m
2
⊥
S
−
r
2
⊥
4m
4
⊥
S
2
+N
1
2m
2
S
−
r
2
4m
4
S
2
6.2 Ginzburg-Landau Model 155
Expanding,
C(x) =
1
(2π)
D
∞
0
dS
2
1
m
D−1
⊥
1
m
1
S
D/2
e
−
1
4S
(
r
2
⊥
m
2
⊥
+
r
2
m
2
)
×
N
⊥
D −1
2m
2
⊥
+
N
2m
2
1
S
−
1
4
N
⊥
r
2
⊥
m
4
⊥
+
N
r
2
m
4
1
S
2
1
(2π)
D
1
2
1
m
D−1
⊥
1
m
4m
2
⊥
m
2
m
2
r
2
⊥
+m
2
⊥
r
2
D
2
+1
D
2
×
N
⊥
D −1
2m
2
⊥
+
N
2m
2
m
2
r
2
⊥
+m
2
⊥
r
2
4m
2
⊥
m
2
−
D
2
×
1
4
N
⊥
r
2
⊥
m
4
⊥
+
N
r
2
m
4
Finally, after simplification,
C(x) =
1
(2π)
D
1
16
1
m
4
⊥
m
4
4m
2
⊥
m
2
m
2
r
2
⊥
+m
2
⊥
r
2
D
2
+1
D
2
N
⊥
(D −1)m
2
+N
m
2
⊥
×
m
2
r
2
⊥
+m
2
⊥
r
2
−D
N
⊥
r
2
⊥
m
4
+N
r
2
m
4
⊥
=
1
(2π)
D
1
16
1
m
4
⊥
m
4
4m
2
⊥
m
2
m
2
r
2
⊥
+m
2
⊥
r
2
D
2
+1
D
2
N
m
2
⊥
(R −1)
×
(D −1)m
2
⊥
r
2
−m
2
r
2
⊥
(6.2.20)
The vital thing about the result is that it is proportional to R −1, i.e. it exists only
if FDT is violated. For R =1, Eq.(6.2.16) leads to C(k)=constant and hence the
Fourier transform would be a delta function at x =0 - including the k
4
term in
C(k), which would yield the usual exponential decay.
For R =1, the typical behaviour of C(r) is
1
r
D
- the power law behaviour that we
said in the beginning can be generated in the presence of a driving force. However,
the correlation function as obtained in Eq.(6.2.20) is anisotropic. If we call θ the
angle between the direction of the applied field
E and the radius vector r, then
r
=r cos θ and r
⊥
=r sin θ . We can now write Eq.(6.2.20) as
C(r,θ) =
1
(2π)
D
1
16
1
m
4
⊥
m
4
4m
2
⊥
m
2
m
2
sin
2
θ +m
2
⊥
cos
2
θ
D
2
+1
1
r
D
D
2
×N
m
2
⊥
(R −1)[(D −1)m
2
⊥
cos
2
θ −m
2
sin
2
θ] (6.2.21)
If we average over θ, then we would get zero and C(r) would decay exponentially.
One of the interesting things about the model of Eq.(6.2.7) is the existence of
the quadratic term E∂φ
2
in the equation of motion. This term will cause the three
point correlation function, which is usually zero, to be nonzero and we will now
156 6 Systems Far from Equilibrium
point out how this comes about. The three point correlation function in the Fourier
space is
C(1, 2, 3) =φ(p,ω
1
)φ(q, ω
2
)φ(r, ω
3
) (6.2.22)
Writing Eq.(6.2.7) in k −ω space and keeping only the nonlinear term involving
E,wehave
G
−1
0
φ(k,ω)=−i(
k
⊥
.
ξ
⊥
+k
ξ
) +iEk
p,ω
φ(p,ω
)φ(k −p, ω −ω
)
(6.2.23)
where
G
−1
0
=−iω +{(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
+2αk
2
⊥
k
2
} (6.2.24)
For E =0, the zeroth order solution φ
(0)
(k, ω) is given by Eq.(6.2.13) i.e.
φ
(0)
(k, ω) =−iG
0
(
k
⊥
.
ξ
⊥
+k
ξ
).
To first order in E, the field φ(k,ω) is
φ(k,ω)=φ
(0)
(k, ω) +iEk
G
0
(k, ω)
p,ω
φ
(0)
(p, ω
)φ
(0)
(k −p, ω −ω
)
(6.2.25)
and to the first order in E, the three point correlation is
C(1, 2, 3) = iEp
G
0
(p, ω
1
)
p
1
,ω
φ
(0)
(p
1
,ω
)φ
(0)
(p −p
1
,ω
1
−ω
)
×φ
(0)
(q, ω
2
)φ
(0)
(r, ω
3
)
+similar terms for q and r (6.2.26)
(Note that φ
(0)
φ
(0)
φ
(0)
=0.) Forming the correlations,
C(1, 2, 3) = iE
p
G
0
(p, ω
1
)C
0
(q, ω
2
)C
0
(r, ω
3
)
+q
G
0
(q, ω
2
)C
0
(p, ω
1
)C
0
(r, ω
3
)
+r
G
0
(r, ω
3
)C
0
(p, ω
1
)C
0
(q, ω
2
)
×δ( p +q +r)δ(ω
1
+ω
2
+ω
3
) (6.2.27)
Integrating over the frequencies, we have

6.3 Phase Ordering Kinetics 157
C(p,q, r) =
dω
1
2π
dω
2
2π
dω
3
2π
C(1, 2, 3)
=
iEδ(p +q +r)
γ(p)+γ(q)γ(r)
p
C
0
(q)c
0
(r) +q
C
0
(r)c
0
(p)
+r
C
0
(q)c
0
(p)
(6.2.28)
where
γ(k)=(m
2
⊥
+α
⊥
k
2
⊥
)k
2
⊥
+(m
2
+α
k
2
)k
2
+2αk
2
⊥
k
2
+2αk
2
⊥
k
2
(6.2.29)
If the FDT holds, for small momenta, C
0
(k) goes to a constant and we have
C(p,q, r) ∝δ( p +q +r)(p
+q
+r
) which is zero. If the FDT does not hold,
C(p,q, r) can be zero if p
=q
=r
=0. On the other hand it can typically be
infinity as momenta tend to zero. This is because C(k) goes to a constant while γ(k)
vanishes as k
2
. In coordinate space C(x
1
,x
2
,x
3
) depends on x
1
−x
2
,x
2
−x
3
and
for |x
1
−x
2
|=|x
2
−x
3
|=r, dimensional counting shows C(r) ∼r
1−2D
, which is
a power law behaviour.
6.3 Phase Ordering Kinetics
In this section we return to the time dependent Ginzburg-landau (TDGL) model
as we discuss the formation of phase ordering. The model, as we have discussed
several times before, is governed by the free energy functional
F =
d
D
x
m
2
2
φ
2
+
1
2
(∇φ)
2
+
λ
4
(φ
2
)
2
(6.3.1)
with m
2
=a
0
(T −T
c
). The high temperature phase (T>T
c
) is symmetric and the
expectation value φ of φ is zero. In this section we imagine the temperature
suddenly being lowered at t =0 from T>T
c
to a temperature T<T
c
.ForT<T
c
,
the ground state of the system governed by the above free energy has a non zero
expectation value. Thus at t =0, the system is in a state with zero expectation
value but is being governed by a free energy whose ground state has a non zero
expectation value. So the system is out of equilibrium at t=0, and as time goes
on it has to gradually move towards equilibrium as t →∞. This means the order
parameter field φ has to acquire expectation value φ - this is the growth of order.
Domains with φ=0 will gradually be formed in the system and this growth is
referred to as phase ordering dynamics. One of the best ways to characterize this
growth is through the typical domain size L(t). A probe of the domain size is the
structure factor C(r,t) defined as
C(r,t) =φ(x,t)φ(x +r,t) (6.3.2)

158 6 Systems Far from Equilibrium
or its Fourier transform
C(k,t) =
C(r,t)e
i
k.r
d
D
r (6.3.3)
The evaluation of φ is governed by the TDGL equation, which reads
˙
φ =−
δF
δφ
+N (6.3.4)
for non conserved order parameter, and
˙
φ =∇
2
δF
δφ
+N (6.3.5)
for conserved order parameter. The existence of a characteristic scale can be seen
easily in the linearized version of Eq.(6.3.5), where for negative values of m
2
(=
−m
2
0
, say), the relaxation rate is
ω =k
2
(k
2
−m
2
0
)
For small k, i.e. k
2
<m
2
0
, the relaxation rate is negative, signalling instability. The
growth rate is a maximum for k =k
c
, where k
2
c
=m
2
0
/2. Thus a characteristic scale
k
c
emerges. The above discussion serves only to emphasize that a characteristic
length scale is not an unusual happening. In reality, the solution of the linearized
equation is never seen. A typical time development after a quench to an ordered
phase is seen in simulations where the dark regions correspond to one phase (pos-
itive φ) and the white regions to the other (negative φ). The interfaces between
the two phases are known as domain walls.
It should be noted that the role of the noise in Eqns.(6.3.2) and (6.3.3) is severely
limited. The quench to the low temperature phase does not in any significant sense
depend on the precise temperature to which the system has been quenched. For
all practical purposes, one would quench to T =0 and the thermal noise would be
absent. By the same token, the precise value of the temperature in the initial state
is irrelevant and we could as well consider the system to be initially at T =∞, i.e.
a totally disordered phase. In this case, one could choose the initial condition to be
specified by the correlation function
φ(x,0 )φ (
x
, 0)=δ(x −
x
) (6.3.6)
and have the evolution equation as completely deterministic, i.e.
∂φ
∂t
=−
δF
δφ
(6.3.7)
6.3 Phase Ordering Kinetics 159
for the non conserved order parameter, and
∂φ
∂t
=∇
2
δF
δφ
(6.3.8)
for the conserved case (we have scaled the time variable by ).
We now bring in the scaling hypothesis. This is the expectation arrived at on the
basis of the pictures of the phase separation process, namely, that for large enough
time, there is a single characteristic scale L(t) such that the domain structure (in
a statistical sense) is independent of time. For the correlation function shown in
Eq.(6.3.2), this implies that
C(r,t) =f
r
L(t)
(6.3.9)
and
C(k,t) =L
D
g(kL) (6.3.10)
where D is the spatial dimensionality (for quenches down to T =0, f(0) =1;
for quenches to arbitrary T<T
c
, f(0) =M(T )
2
) and we can write C(r, t) =
M
2
f
r/L
. For two different times,
C(r,t, t
) =f
r
L
,
r
L
(6.3.11)
where L and L
stand for L(t) and L(t
).ForL L
C(r,t, t
) →
L
L
¯
λ
h
r
L
(6.3.12)
where the exponent
¯
λ is a nontrivial exponent associated with the phase ordering
kinetics. Writing the free energy in Eq.(6.3.1) in general as
F =
d
D
x[
1
2
(∇φ)
2
+V(φ)] (6.3.13)
the equation of motion for non conserved order parameter is
∂φ
∂t
=(∇
2
φ −
∂V
∂φ
) (6.3.14)
In the absence of dynamics, the profile of the order parameter (i.e. the wall profile)
is
∇
2
φ =
∂V
∂φ
(6.3.15)

160 6 Systems Far from Equilibrium
If we consider a domain wall which is flat and z is the direction perpendicular to
the domain wall, then
∂
2
φ
∂z
2
=
∂V
∂φ
(6.3.16)
with φ(±∞) =±1. The wall where φ =0 is taken to have its centre at z =0, so
that φ(0) =0. Integrating Eq.(6.3.16) once
(
∂φ
∂z
)
2
=2V(φ) (6.3.17)
and hence the energy per unit area of the wall which is the surface tension is given
by,
σ =
∞
−∞
dz(
∂φ
∂z
)
2
=
+1
−1
dφ[2V ]
1/2
(6.3.18)
Linearizing Eq.(6.3.17) about φ =±1gives
1 ∓φ ∼e
−[V
(±1)]
1/2
|z|
z →±∞ (6.3.19)
The order parameter exponentially moves away from the walls. The excess energy
is localized in the domain walls (where the order changes rapidly) and the driving
force for domain growth is the wall curvature since the system energy can decrease
only through a reduction in the total wall area. The existence of a surface tension
means a force per unit area proportional to the mean curvature at each point of
the wall. If the force per unit area is F then the work done in reducing the area
by dR is F 4πR
2
dR and this must equal the reduction in surface energy which is
8πσRdR and thus F =2σ/R. For non conserved order parameter this force will
cause the wall to move with a velocity proportional to the local curvature. The
force is expressible as η
dR
dt
where η is a friction coefficient and hence
η
dR
dt
=−2σ/R (6.3.20)
This same result follows from the equation of motion given in Eq.(6.3.14). Con-
sidering a spherical domain of φ =−1 immersed in a sea of φ =+1, we note that
in a D-dimensional space
∂φ
∂t
=
∂
2
φ
∂r
2
+
D −1
r
∂φ
∂r
−v
(φ) (6.3.21)
If the droplet radius R is much greater than the interface width ξ, then we expect
a solution of the form
φ(r,t)=f [r −R(t)] (6.3.22)
6.3 Phase Ordering Kinetics 161
Inserting in Eq.(6.3.21)
f
+(
D −1
r
+
dR
dt
)f
−V
(f ) =0 (6.3.23)
Now f changes from +1to−1 in a small region of width ξ near the interface. Its
derivative is consequently zero away from the surface r =R(t) and highly peaked
near r =R(t). Multiplying Eq.(6.3.23) by f
and integrating from one side of the
surface to the other
D −1
R
+
dR
dt
=0 (6.3.24)
since away from r =R(t), f
=0 on either side and V(φ) being symmetric in
φ also has the same value on either side of r =R(t). Integration of Eq.(6.3.23)
yields
R
2
(t) =R
2
(0) −2(D −1)t (6.3.25)
which shows that the time of collapse, t
0
, of the wrong kind of droplet scales with
the initial size R(0) of the droplet as t
0
∼R
2
(0).Ifη =σ , then Eqns.(6.3.24) and
(6.3.20) give identical results. But why should this equality hold ? Let us consider
the rate of energy dissipation for a plane wall moving under the influence of some
external driving force with speed v. The rate of energy dissipation per unit area is
dE
dt
=
∞
−∞
dz
δF
δφ
∂φ
∂t
=−
∞
−∞
dz(
∂φ
∂t
)
2
(6.3.26)
using Eq.(6.3.7). If the wall profile has the form
φ(z,t)=φ(z−vt),
then
∂φ
∂t
=−v
∂φ
∂z
and Eq.(6.3.26) reduces to
dE
dt
=−v
2
∞
−∞
dz(
∂φ
∂z
)
2
=−σv
2
(6.3.27)
from Eq.(6.3.18). If η is a friction coefficient, then by definition
dE
dt
=−ηv
2
