Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.

90
The first universal bound (independent of initial data) has been established recently
in [16]. More precisely, if (N - l)p < N + 1, r > 0 and u is a global nonnegative solution
then
u{x,t)<C{T,D,p), xeD,t>r. (2)
Even more recently, Quittner has derived a universal bound of this kind in [32] for
(N - 2)p < N + 2 and N < 3.
2.2 Exponential nonlinearity
Consider next the problem:
u
t
= Au + Ae
u
i€Bi(0),<>0,
u = 0 x 6 dfli(O), i>0,
u(x,0) = uo(x) i £ Bi(0),
where Bi(0) = {x e R
N
: |x| < 1}, u
0
is a radial continuous function on B
1
(0) vanishing
on dBi(0) and
A
is a positive parameter.
For JV = 1,2 it was shown in [5] that every global classical solution is uniformly
bounded even for general domains and initial data. On the other hand, for N > 10 and
A
= 2(iV-2), global unbounded classical solutions do exist (cf. [22]). For 3 < N < 9 it was
known that global unbounded L
1
-solutions exist for certain
A
> 0 (see [23]), which makes
the problem of existence of unbounded global classical solutions even more interesting. It
was conjectured (see [23] and [42]) that such solutions might exist for some dimensions
3 < N < 9 and A > 0. A result from [12] rules out this conjecture, as far as radial
solutions are concerned. Namely, the main aim of [12] was to prove that every global
classical solution is uniformly bounded if 3 < N < 9 and
A
> 0.
2.3 Chipot-Weissler equation
In this subsection we discuss the problem
ut = Au - \Wu\
q
+ Xu" xeD,t>0,
u = 0 x € 3D, t > 0,
u(x,0) = u
0
(x) > 0 ieB.
Here p, q > 1 and
A
> 0. Under the assumptions
3<-7T, A>0 or q = ^r,
A
> 2"(p+ l)(p -
l)-*"
1
,
p+
1
p+ 1
and (N
—
2)p < N + 2it was shown in [6] that global increasing solutions are bounded if
D is a bounded domain in R
JV
.
If q > p and A is small enough then all solutions are global, bounded and decay
exponentially to zero provided D C R
N
is a domain (possibly unbounded) where the
Poincare inequality is valid (see [40]). Conversely, if D is such that the Poincare inequality
is not valid then there always exist unbounded solutions (cf. [40]). These results of
91
[40] were slightly improved and given a more complete form in [38]. In particular, the
restriction on smallness of
A
was removed for global existence.
Studies of global solutions of similar problems with more general nonlinearities /
(u,
Vu)
were performed in [24] and [35].
2.4 Nonlocal nonlinearity
The following nonlocal problem is considered in [7]:
x e D, t > 0,
xeT-L,
t > 0,
x
e r
2
, t > o,
x el>,
where D is a bounded domain in R
N
, 3D =
T
1
L)T
2
,
I\ DF
2
= 0,
U
f e C\R), / > 0, J f(u) du < oo, F(u) = J f(v) dv,
— oo —oo
g : (0, oo)
—>
(0, oo) is a locally Lipschitz function.
The assumptions on / and g imposed in [7] guarantee blow-up in finite time if
UQ
is
large. Sufficient conditions for boundedness of global solutions are given in [7] and their
sharpness is discussed there. A particular case of the main result from [7] was proved
independently in [1] where the method from [5] was also used. A priori bounds for global
solutions of nonlocal equations of this type and also of the form
u
t
= Au +
f(x,u)-
—J f(x,u)dx
were established in [33].
2.5 Equation with a localized reaction term
In [36], the following problem was studied:
u
t
= &u + u
p
(x
0
(t),t) xeD,t>0,
u = 0 x
G
dD, t > 0,
u(x,0) = u
0
(x) > 0 ieB.
where p > 1, D is a bounded domain in R
N
and x
0
:
[0,
oo)
—>
D is a Holder continuous
function. The results in [36] say that if either i
0
= 0 6 D or J\f = 1 then every global
solution is uniformly bounded. The former result is in contrast with the fact that for
the problem discussed in Subsection 2.1, boundedness of global solutions holds only for p
which is Sobolev subcritical ((TV - 2)p <
TV
+ 2).
ut
u
du
u(x,Q)
Au + g(J
D
F(u)dx)f(u)
0
0
u
0
(x)
92
2.6 Periodic forcing term
Consider the problem
ih = Au + m(t)f(u) x £ D, t > 0,
u = 0 x e dD, t > 0,
u(x,0) = u
0
(x) x e D,
where D is a bounded domain in Ft", / is a superlinear function and m is positive and
periodic. An a priori bound for global solutions of this problem was derived in [34].
2.7 Abstract equation
In [9] we studied the initial value problem for evolution equations of the form
d
^+Au(t)=F(u(t)),
in a Banach space where A is the infinitesimal generator of an analytic semi-group and F
is a nonlinear function such that the initial value problem possesses non-global solutions
for some initial data. (See [9] for details of the setting which are omitted here). We gave
sufficient conditions (on A, F and the underlying spaces) to ensure that global solutions
are necessarily bounded in a suitable norm. We also gave several examples of problems
to which the abstract result applies. They include some nonlocal equations and equations
of order higher than two.
3 Quasilinear equations with the Dirichlet boundary
condition
Several works have been devoted to the study of boundedness of global solutions of the
degenerate problem
u
t
= A(|u|
m
-
1
u) + /(w) xeD, t>0,
u = 0 xedD, t >0,
u(x,0) = u
0
(x) x € D,
where m > 0. It was shown in [28] that for m > 1, D convex, uo > 0 and f(u) = u
p
, all
global solutions are bounded if m < p < (N + 2)/N, while global unbounded solutions
exist iip/m > (N + 2)/(N
—
2),N> 2. The results from [17] that were mentioned above
also apply to this problem if f(u) = u
p
, D is a ball and u
0
is radial (cf. [17] for details).
In [5], boundedness of global solutions is proved for general D and
UQ
and nonlinearities
/ that include
/ s , ,„-i r ^ f iV-21 p N + 2
/(«) = H
P
»: p>max{l,m}, m > max JO, -^—- j , — < ^3^-
This result was improved in [25] in several ways. Instead of |u|
m_1
u a large class of
continuous increasing functions $(u) was taken there. Non-power growth of $ was allowed

93
for
N
< 2 as well as more general /'s. For
N =
1, Suzuki ([41]) proved boundedness of
global solutions for
m
> 1 for some nonlinearities
/
that are not allowed in [5] and [25].
Arguments and results from [5] were generalized to parabolic p-Laplace equations in [26].
For any global solution, an a priori bound of the form
Hx,t)\
< C(\\u
0
\\
L
«,
(D)
,D,p,rn) ,
x €
D,
i >
0
has been recently established in [39] for f(u)
=
|u|
p_1
w under the assumption
10m+ 2
Km<p
<m +
——
,
if JV
>
1,
and
a
universal bound of the form
u(x,t)<C(T,D,p,m), xeD,t>r>0
has been derived there for global nonnegative solutions provided
N + 2
1 <m<p< —Tz—m, if
N
> 1.
N
4 Cauchy problem for
a
semilinear equation
Consider the problem
u
t
=
Au
-
ii\Vu\i +
vP
ieR",(>0,
u(x,0)
=
u
0
(a;)>0
xeR
N
.
Herep, q > 1 and /u
>
0. If fi = 0 and (N-2)p < N + 2 then all global solutions decay
uniformly to zero (cf. [37]). (An earlier study of global solutions of this problem with
fi = 0 was performed in [21] where sign-changing solutions with fast decay (as
x
—>
oo)
were considered). If
/x
> 0 then there exist global solutions such that (cf. [40])
:
lim u(x,t) = oo for all
x e
R
w
.
5 Stefan problem for
a
semilinear equation
Consider now the following reaction-diffusion problem with free boundary:
u
t
=
u
xx
+ v?
xe
(0, s(i)),
t
> 0,
u(x,
0)
=
u
0
(x)
>
0
x e
(0,
s
0
), s(0)
=
s
0
> 0,
u(s(t),t)
=
u
x
{0,t) = 0
t>0,
s'(t)
=
-
Ua;
(s(t),t)
t>0,
where
p > 1.
This problem can be viewed as
a
simple model of
a
chemically reactive
and heat-diffusive liquid surrounded by ice. Here u > 0 represents the temperature of the
liquid phase, and the ice is assumed to be at temperature 0.
Global existence, stability and blow-up for this problem were studied in [18], for pre-
vious studies see references therein and in [15].
In
[15] we showed that
if u
is
a
global
solution then
u<C(s
0
, ||uo||
c
i([
0
,
so
]).
94
6 Cauchy problem
for a
semilinear equation
in
simi-
larity variables
Consider
a
solution
of the
problem
11,
=
A«
+ «
p
x e R
N
,
0<t<T,
u(x,0)
=
u
0
{x)>0 xeR
N
,
which blows
up at t = T. For a E R" one can
rescale
u
using backward similarity
variables
as
follows
s
=
-log(r-t),
j/
= -^L,
w{y,s)
=
(T-t)^u{x,t).
Then
w
satisfies
w.
= Am y
•
Vw + w
p
-w y € R", s >
—
logT,
2
y
p-1
y
(3)
w(y,-logT)
=
T^u
0
(a
+ VTy)
yeR
N
.
Under some assumptions
on u
0
and p, it has
been shown
in [27]
that
for 6 > 0
there
exists
C =
C(p,
N,
6)
> 0
such that
for any
global solution
of
(3),
it
holds
w<C
for s> -\ogT
+ S.
In
the
original variables this
can be
equivalently formulated
as a
universal bound
(independent
of
«o)
for the
blow-up rate.
7 Nonlinear boundary conditions
7.1 Heat equation
The following problem
is
studied
in [4]:
u
t
= Au x
G
D, t > 0,
—
= f(u)
x£dD,t>0,
u(x,0)
=
UQ(X)
X&D.
Here
I? is a
bounded domain
in R and v is the
outer normal
to dD. The
result from
[4]
as
applied
to the
particular case with
f(u) =
\u\
p
~
1
u says that global solutions
are
bounded
if (N
—
2)p < N.
This means that
in
this case there
are no
global positive
solutions since there
is no
positive steady state
and the
trivial steady state
is
unstable
from above.
The
main result
of
[4]
and
several elements
of its
proof were generalized
in
[24].
95
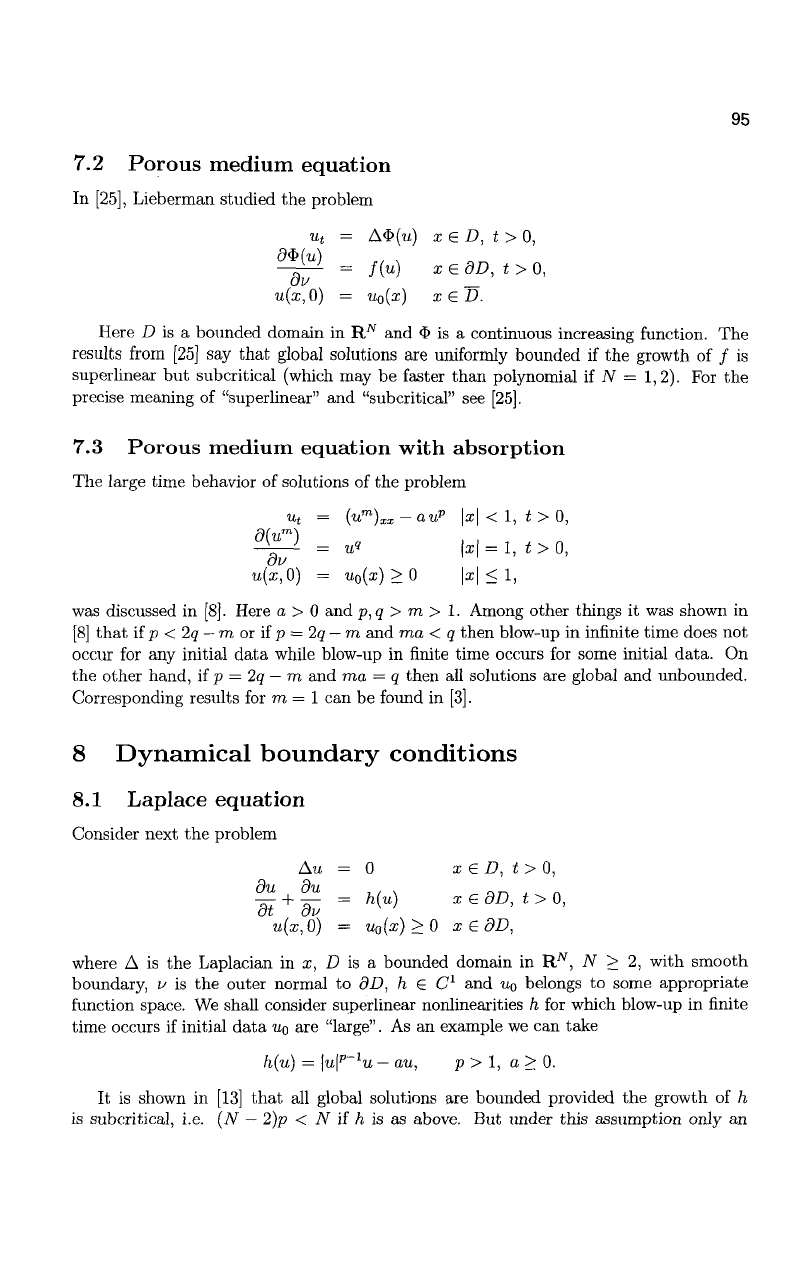
7.2 Porous medium equation
In [25], Lieberman studied the problem
A$(u) x e D, t > 0,
/(«) xedD, t > 0,
«o(^) x £ D.
Here D is a bounded domain in R
N
and $ is a continuous increasing function. The
results from [25] say that global solutions are uniformly bounded if the growth of / is
super linear but subcritical (which may be faster than polynomial if N = 1,2). For the
precise meaning of "superlinear" and "subcritical" see [25].
7.3 Porous medium equation with absorption
The large time behavior of solutions of the problem
u
t
=
(u
m
)
xx
- au
p
u(x,0) =
UQ(X)
> 0
was discussed in [8]. Here a > 0 and p,q> m > 1. Among other things it was shown in
[8] that if p < 2q
—
m or if p =
2q —
m and ma < q then blow-up in infinite time does not
occur for any initial data while blow-up in finite time occurs for some initial data. On
the other hand, if p = 2q
—
m and ma = q then all solutions are global and unbounded.
Corresponding results for m = 1 can be found in [3].
8 Dynamical boundary conditions
8.1 Laplace equation
Consider next the problem
0 x e D, t> 0,
h(u) x&dD, t > 0,
u
0
(a;) > 0 xedD,
where A is the Laplacian in x, D is a bounded domain in R
N
, N > 2, with smooth
boundary, u is the outer normal to dD, h e C
1
and u
0
belongs to some appropriate
function space. We shall consider superlinear nonlinearities h for which blow-up in finite
time occurs if initial data
UQ
are "large". As an example we can take
h{u) = |w|
p_1
u
—
au, p > 1, a > 0.
It is shown in [13] that all global solutions are bounded provided the growth of h
is subcritical, i.e. (N
—
2)p < N if h is as above. But under this assumption only an
dv
u(x,0)
\x\ < 1, t >0,
|x|
= 1, t> 0,
W<1,
Au
du du
u(x,0)
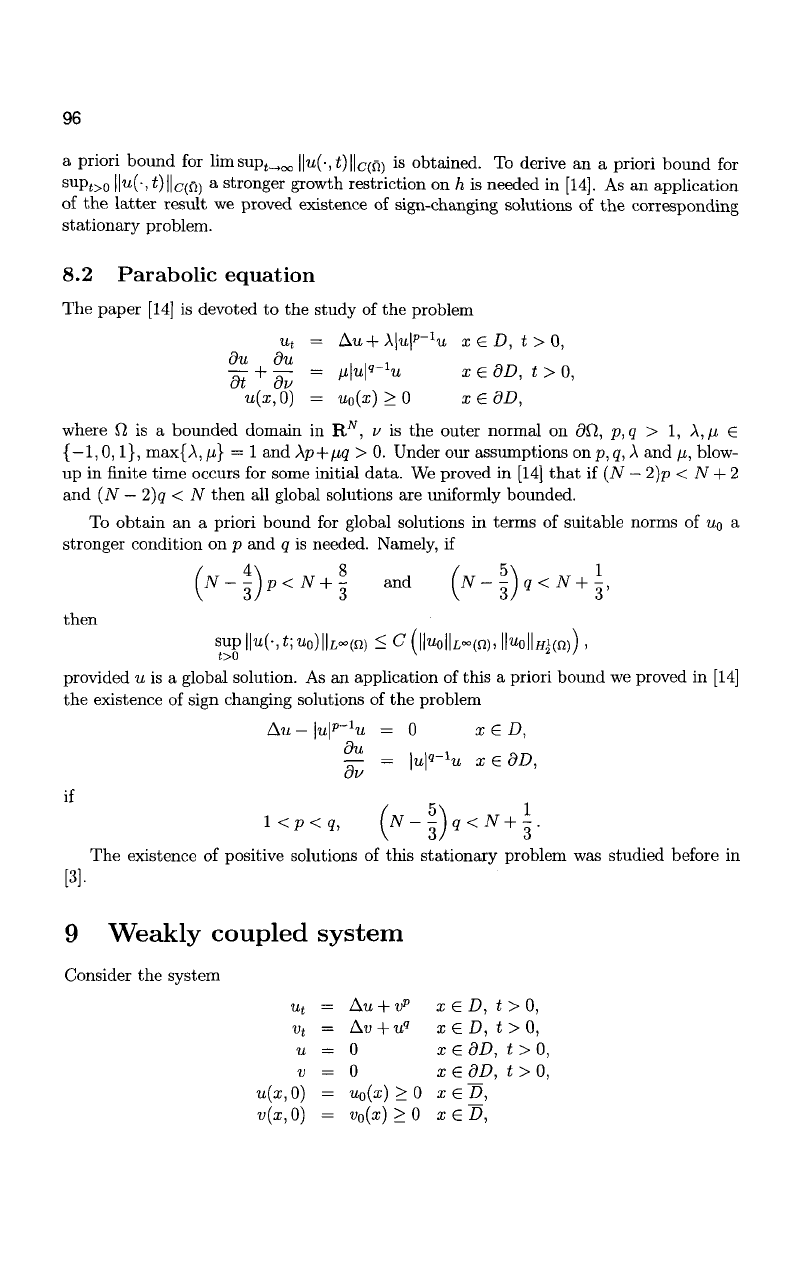
96
a priori bound for limsup^^ ||w(-,t)||
C
(n)
is
obtained. To derive an a priori bound for
su
Poo ll
u
('i*)llc(ft)
a
stronger growth restriction on h is needed in [14]. As an application
of the latter result we proved existence of sign-changing solutions of the corresponding
stationary problem.
8.2 Parabolic equation
The paper
[14] is
devoted
to the
study
of the
problem
tH
= Au + \\u\
p
-
l
u x£D,t>0,
du du , , i „„
-sr + w- = nM
q
u xedD, t > 0,
at dv
u(x,0) = u
o
{x)>0 xedD,
where Q is a bounded domain in R
w
, v is the outer normal on dQ, p, q > 1,
A,
fj,
£
{—1,0,1}, max{A,
fj,}
= 1 and \p+/iq > 0. Under our assumptions on p, q,
X
and n, blow-
up in finite time occurs for some initial data. We proved in [14] that if (JV
—
2)p < N + 2
and (N
—
2)q < N then all global solutions are uniformly bounded.
To obtain an a priori bound for global solutions in terms of suitable norms of
M
0
a
stronger condition on p and q is needed. Namely, if
N--^)p<N + - and (N - -) q < N + -,
then
sup||u(-,£;u
0
)||z,~(n) < C (|KIU~(0), IKII/fi(n))
>
oo
x
'
provided u is a global solution. As an application of this a priori bound we proved in [14]
the existence of sign changing solutions of the problem
Au - lu^u = 0 x £ D,
^ = Wo-^ xedD,
dv
if
/ 5\ 1
Kp<q, (N--)q<N + -.
The existence of positive solutions of this stationary problem was studied before in
[3].
9 Weakly coupled system
Consider the system
«t
v
t
u
V
u(x,0)
v(x,0)
= Aw
+
?)
p
= Av + u
q
= 0
= 0
= u
0
(x) > 0
= v
0
(x)>Q
x e D, t > 0,
x e D, t > 0,
xedD, t > 0.
xedD, t>0,
x
e
ID,
iefl,
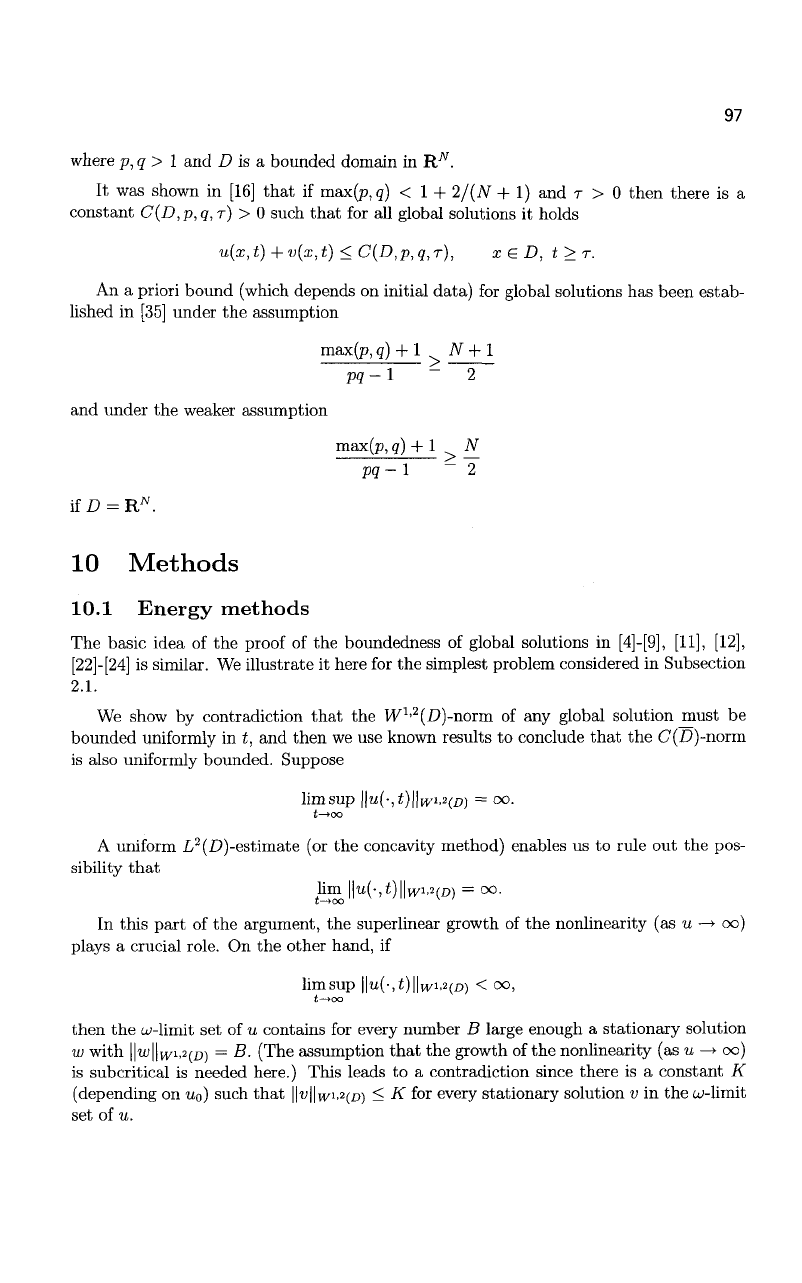
97
where p, q > 1 and D is a bounded domain in R
N
.
It was shown in [16] that if max(p,g) < 1 + 2/(N + 1) and r > 0 then there is a
constant C(D,p,q,r) > 0 such that for all global solutions it holds
u(x,t) +v(x,t) < C(D,p,q,r), x e D, t > T.
An a priori bound (which depends on initial data) for global solutions has been estab-
lished in [35] under the assumption
max(p,g) + l
TV
+ 1
pq - 1 " 2
and under the weaker assumption
max(p,
q)
+ 1 TV
pq-1 ~^
if D = R
N
.
10 Methods
10.1 Energy methods
The basic idea of the proof of the boundedness of global solutions in [4]-[9], [11], [12],
[22]-[24] is similar. We illustrate it here for the simplest problem considered in Subsection
2.1.
We show by contradiction that the W
/1,2
(D)-norm of any global solution must be
bounded uniformly in t, and then we use known results to conclude that the C(Z))-norm
is also uniformly bounded. Suppose
limsup ||u(-,t)||
v
^i,2(£
)
) = oo.
t—*oo
A uniform L
2
(D)-estimate (or the concavity method) enables us to rule out the pos-
sibility that
hm||u(-,t)
11^1,2(0)
= oo.
In this part of the argument, the superlinear growth of the nonlinearity (as u
—>
oo)
plays a crucial role. On the other hand, if
limsup ||u(-,i)||vKi.2(D) < oo,
then the o;-limit set of u contains for every number B large enough a stationary solution
w with
||w||W^
2
(D)
= B. (The assumption that the growth of the nonlinearity (as u
—*
oo)
is subcritical is needed here.) This leads to a contradiction since there is a constant K
(depending on
UQ)
such that ||u||iyi,2(£)) < K for every stationary solution v in the oi-limit
set of u.

98
Both parts of the argument rely on the existence of a suitable Lyapunov functional.
In general, this method does not yield any a priori estimate of ||u(-, i)||iv
1
.
2
(D) in terms of
some norms of
UQ.
Other energy and interpolation arguments were used in [2]. The method from [2] was
further developped in [31, 33] by bootstrapping and employing maximal regularity results.
10.2 Scaling arguments
The variational structure together with scaling invariance are the main tools in [19]. If
we rescale a solution u of the equation
u
t
= AH + u
p
,
as
v(y, s) = \r-
l
u{x + Xy,t + As),
then v satisfies the same equation. This fact, combined with the existence of a Lyapunov
functional, yield a contradiction argument to prove the a priori bound in [19].
A different contradiction argument, relying on scaling, energy and Hardy's inequality,
gives the universal bound in [32]. In order to handle problem (3), this approach was
extended in [27].
For problems without variational structure, a scaling argument was used in [35] in
order to prove a priori estimates for global solutions.
10.3 Zero number techniques
The method of our proof of boundedness of global classical solutions in [12] is based on
the intersection-comparison with a particular self-similar solution. It was inspired by the
proof of Theorem 15.1 in [17] where a similar result was shown for a different problem.
However, unlike the case in [17], the nonincrease of the number of zeros of the difference
of two solutions, by
itself,
is not sufficient. We also employ the more subtle "zero number
diminishing property" which says that the number of zeros drops when a multiple zero
occurs.
The nonincrease of the zero number also plays a crucial role in [41].
10.4 Smoothing effect in weighted Lebesgue spaces
To prove (2.2) in [16], we start from the classical argument of Kaplan which yields that
for any r > 0 there is T\ £ (0, r/2) such that
Ju"(T
1
,x)<pi{x)dx<C{n,p,T), (4)
n
where ipi > 0 is the first eigenfunction of —A in
HQ(SV),
normalized by /
n
ipi
— 1. Since
Ci<5 < ipi < C2<5, <5(a;) = dist(x,dD), the bound (4) suggests that it is rather natural to

99
consider the problem in
L%
= L
q
(D, S(x) dx) spaces. With this motivation, we developped
in [16] both linear and local nonlinear theories in these spaces.
Our main result in the linear theory is an optimal Lj - L
r
s
estimate for the heat
semigroup. In view of this estimate for the heat semigroup, the local existence and
uniqueness theory in L\ is similar to the usual L
9
-theory in dimension
JV+1.
In particular,
we proved local existence and uniqueness for solutions in L\ for q > q
c
= |(JV + l)(p
—
1)
and local nonexistence for q < q
c
. We also established a nonlinear version of the L\
—
L
r
e
estimate whose particular case q = p, r = oo, plays a crucial role in deriving the universal
bound (2) from (4).
A similar idea is used in [27] but weighted Lebesgue spaces are replaced by convolution
Lebesgue spaces there.
11 Applications
11.1 Blow-up
The first application concerns blow-up in finite time. It requires some knowledge of the
corresponding stationary problem. For example, if one can show that there are no steady
states then all solutions of the parabolic problem blow up in finite time (cf. [1, 5]). A
slightly more sophisticated application of this type says that a solution blows up in finite
time if it starts above a maximal steady state provided this steady state is unstable from
above, cf. [3, 4, 6, 8], for instance.
11.2 Existence of stationary solutions
The second application goes in the opposite direction. Some knowledge of the parabolic
problem is used to draw conclusions on the corresponding stationary problem. We describe
roughly a simple illustration of this idea. Assume a stable steady state is known to exist.
If
Mo
belongs to the boundary of its domain of attraction and the solution u starting from
u
0
is global then there must exist another (unstable) steady state which is in the w-limit
set of u. For dynamical proofs of existence of solutions of elliptic problems we refer to
[13,
14, 29, 30, 34].
11.3 Existence of periodic solutions
The a priori bound discussed in Subsection 2.6 was used in [34] to prove the existence of
solutions which are periodic in t.
11.4 .^-connections
The result from [12] mentioned in Subsection 2.2 plays a fundamental role in the study
of connections between equilibria by solutions which blow up but continue to exist in a
weak sense (see [10, 11]).
