Balakumar Balachandran, Magrab E.B. Vibrations

Подождите немного. Документ загружается.

While displacement-mode shapes are important for determining loca-

tions of displacement sensors, velocity sensors, and accelerometer sensors,

strain-mode shapes are important for determining the locations of strain sen-

sors. For design purposes, the information about locations of sensors and

actuators with reference to nodes of a mode is put forth in the form of the fol-

lowing guidelines.

Natural frequency comparisons for different boundary conditions We

now examine the percentage differences  in the lowest natural frequency in

beams with different boundary conditions, namely, clamped at both ends,

hinged at both ends, clamped at one end and hinged at the other, and clamped

at one end and free at the other. We assume that the beam material and geom-

etry are the same in each case and that only the boundary conditions are dif-

ferent from one case to another. The natural frequencies of the beam clamped

at both ends are used as reference values for the calculations. Then, from

Table 9.3, we determine the following

where 

ch

is the measure of how much the ﬁrst natural frequency f

1,ch

of a

clamped-hinged beam is below the ﬁrst natural frequency f

1,cc

of the

clamped-clamped beam, 

hh

is the measure of how much the ﬁrst natural fre-

quency f

1,hh

of a hinged-hinged beam is below the ﬁrst natural frequency

1,cc

of the clamped-clamped beam, and 

cf

is the measure of how much the

ﬁrst natural frequency f

1,cf

of a cantilever beam is below the ﬁrst natural fre-

quency f

1,cc

of the clamped-clamped beam. Thus, the clamped-pinned

beam’s natural frequency is 31% lower than that of a beam clamped at each

end and the cantilever beam’s natural frequency is 84% lower than that of a

beam clamped at each end. These observations lend themselves to the fol-

lowing design guideline.

cf

 100 a



1,cf



1,cc

1b 100 a

10.5969p 2

11.5056p 2

1b84.3%

hh

 100 a



1,hh



1,cc

1b 100 a

1p2

11.5056p2

1b55.9%

ch

 100 a

1n,ch

1n,cc

1b  100 a



1,ch



1,cc

1b 100 a

11.25p2

11.5056p2

1b31.1%

580 CHAPTER 9 Vibrations of Beams

Design Guidelines: For a system modeled as a beam, if a sensor

needs to be located so as to sense the vibrations in a certain mode, then

this sensor should be located away from the nodes of this mode. Simi-

larly, in order to actuate or excite a certain vibration mode, the actuator

should be away from the node of this mode. Alternatively, if a sensor

should not sense a certain vibration mode or an actuator should not

excite a certain vibration mode, then they should be located at a node

of that mode.

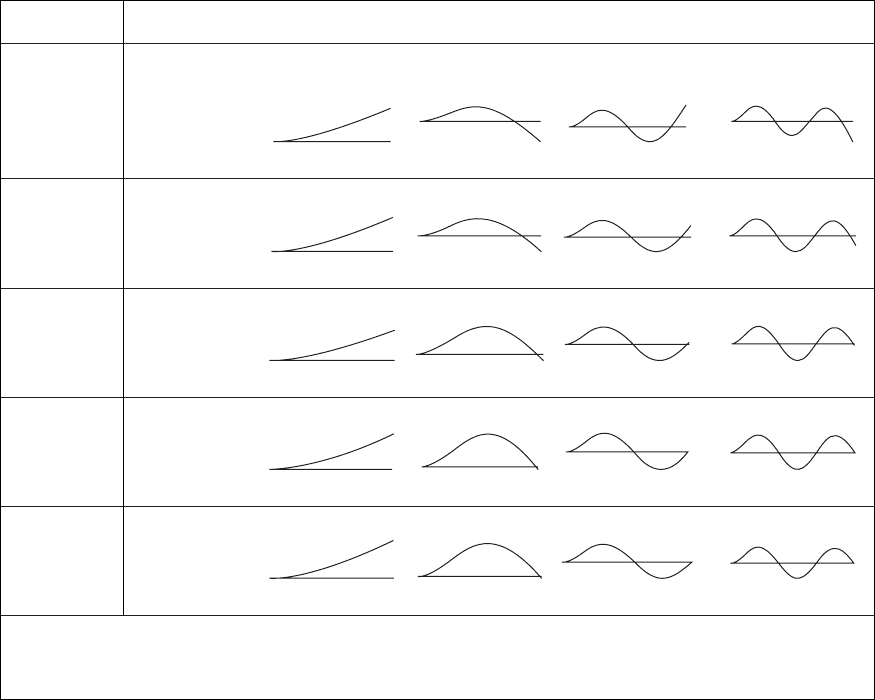
We note from Table 9.3 that as the frequency mode number n becomes

large, the natural frequency coefﬁcients for different boundary conditions

tend towards the same value: np. Thus, for natural frequencies higher than the

tenth (n  10), the beam can be approximated as if it were hinged at both its

ends; in this region the error in the natural frequencies will be less then 5%.

Beam free at both ends We now consider a beam that is free at both ends,

which is given by Case 5 of Tables 9.2 and 9.3. It is seen in Table 9.3 that the

natural frequency coefﬁcients are the same as those for a beam clamped at

both ends. However, the mode shapes are different. The mode shape corre-

sponding to the nth nondimensional frequency coefﬁcient 

has (n  1)

node points; that is, there are two node points in the ﬁrst mode, and so forth.

Correspondingly, for the beam clamped at both ends, there are (n  1) node

points in the interior of the beam. Examples of practical applications that use

models of beams free at both ends are the study of the vibrations of launch ve-

hicles

and ships.

In addition to the mode shapes shown in Table 9.3, a beam free at both

ends also has rigid-body modes whose form is given by

(9.102)

Both modes given in Eq. (9.102) are associated with 

 0; that is, the nat-

ural frequency in each case is zero; thus, the zero eigenvalue corresponds to a

nontrivial mode shape in this case. The ﬁrst of the expressions in Eqs. (9.102)

represents a case where every point on the beam experiences the same trans-

lation, and the second of these expressions represents a case where every

point on the beam experiences a combination of translation and rotation. Un-

like the ﬂexural modes of vibration given in Case 5 of Table 9.2, for the rigid-

body modes given by Eq. (9.102), d

/dh

is zero everywhere on the beam

and hence, the strain associated with vibrations in a rigid-body mode is zero

everywhere on the beam.

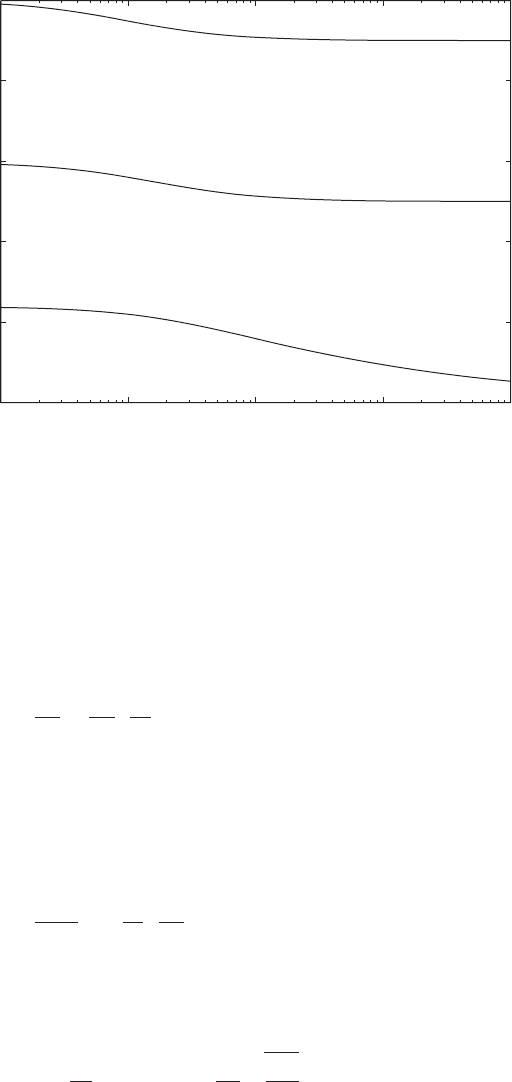
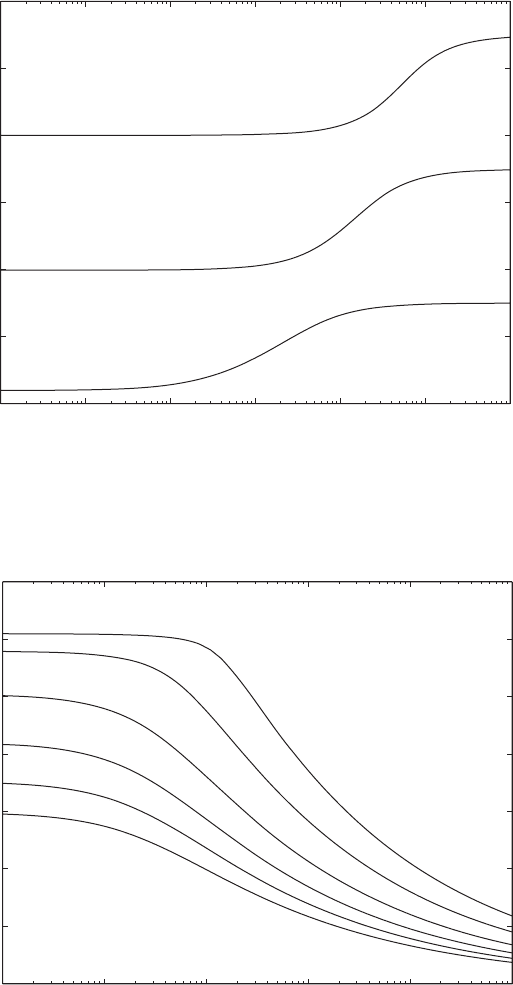
Cantilever beam with a mass at the free end The natural-frequency coefﬁ-

cients and corresponding mode shapes for a cantilever beam with a mass at

the free end are given Table 9.4 and the variations of the lowest three natural

frequency-coefﬁcients as a function M

are given in Figure 9.8. From

Eq. (6a) of Table 9.2, we see that when the ratio of the attached mass to the

mass of the beam M

becomes very large, the characteristic equation and

mode shapes approach the characteristic equation and mode shapes of a beam

1h 2 C

and

1h 2 D

 E

9.3 Free Oscillations 581

A. Joshi, “Prediction of Free-Free Modes from Single Point Support Ground Vibration Test of

Launch Vehicles,” J. Sound Vibration, Vol. 216, No. 4, pp. 739–747 (1998).

Design Guideline: For uniform beams having the same material and

same geometry, the beam clamped at both ends has the highest funda-

mental natural frequency.

clamped at one end and hinged at the other, which are given by Eqs. (3a) and

(3b) of Table 9.2. When M

becomes very small, the characteristic equa-

tion and mode shapes approach the characteristic equation and mode shapes

of a beam clamped at one end and free at the other, which are given by

Eqs. (4a) and (4b), respectively, of Table 9.2. The nondimensional natural-

frequency coefﬁcient 

approaches zero as M

approaches inﬁnity. Thus,

in the limit, the nth mode of a cantilever beam with a mass at the free end be-

comes the (n  1)th mode of a beam clamped at one end and hinged at the

other. An example of a system that is modeled as a cantilever beam with a

mass on its free end is a water tower.

In many instances where a beam carries an end mass, the inertia of

the beam is neglected and the system is represented by an equivalent single

582 CHAPTER 9 Vibrations of Beams

TABLE 9.4

Natural-Frequency Coefﬁcients, Mode Shapes and Interior Node Points for a Cantilever Beam with Mass at Free End:

Case 6 of Table 9.2.

n  1 n  2 n  3 n  4



‡

0.5969 1.4942 2.5002 3.5000

(n  0.5, n  4)

0.0 Mode shapes

Node points

None 0.783 0.504, 0.868 0.358, 0.644, 0.906



‡

0.5484 1.4004 2.3717 3.3492

Mode shapes0.1

Node points

None 0.841 0.530, 0.921 0.375, 0.673, 0.953



‡

0.3972 1.2832 2.2709 3.2648

Mode shapes1.0

Node points

None 0.953 0.55, 0.983 0.384, 0.689, 0.991



‡

0.2769 1.2573

†

2.2544

†

3.2531

†

Mode shapes5.0

Node points

None 0.988 0.55, 0.996 0.386, 0.692, 0.998



‡

0.2342 1.2537

†

2.2522

†

3.2516

†

Mode shapes10.0

Node points

None 0.994 0.557, 0.998 0.386, 0.692, 0.999

‡

The natural frequency f

in Hertz as a function of 

is given by Eq. (9.94).

Values of h not including the boundaries.

†

Approaches (n  1)th natural frequency and mode shape for the clamped-hinged boundary conditions.

9.3 Free Oscillations 583

degree-of-freedom system. This situation is revisited to point out when it is

reasonable to neglect the beam inertia and when it is not. For a beam with an

end mass, the natural frequency of the equivalent single degree-of-freedom

system is determined by making use of the stiffness expressions listed in

Table 2.3. For a cantilever beam with an end mass, we make use of Case 4 of

Table 2.3 and ﬁnd that the natural frequency is

(9.103)

Equation (9.103) is the result obtained by approximating the ﬁrst natural fre-

quency of a cantilever beam with an end mass as the natural frequency of a

single degree-of-freedom system where only the beam stiffness is taken into

account and the beam inertia is neglected. However, when the beam inertia is

not neglected, the ﬁrst natural frequency v

is obtained from Eq. (9.67) as

(9.104)

where 

is the lowest natural-frequency coefﬁcient obtained by solving

Eq. (6a) in Table 9.2. On comparing Eqs. (9.103) and (9.104), the percentage

error one incurs in using Eq. (9.103) is

(9.105)e  100 a

 1b 100 a



1b %



EI

rAL

 a





2

1

0.5

1.5

2.5

Ω

/

Ω

/

Ω

/

Ω

/

FIGURE 9.8

First three natural-frequency coefﬁ-

cients for a cantilever beam carrying

a mass at its free end as a function

of M

Determining 

from Eq. (6a) in Table 9.2 for a given M

, we ﬁnd from

Eq. (9.104) that we have an error e  5% when the mass ratio M

 2.3

and that we have an error e  1% when the mass ratio M

 11.7. For

 1, the error is 11.2%. This leads to the following design guideline.

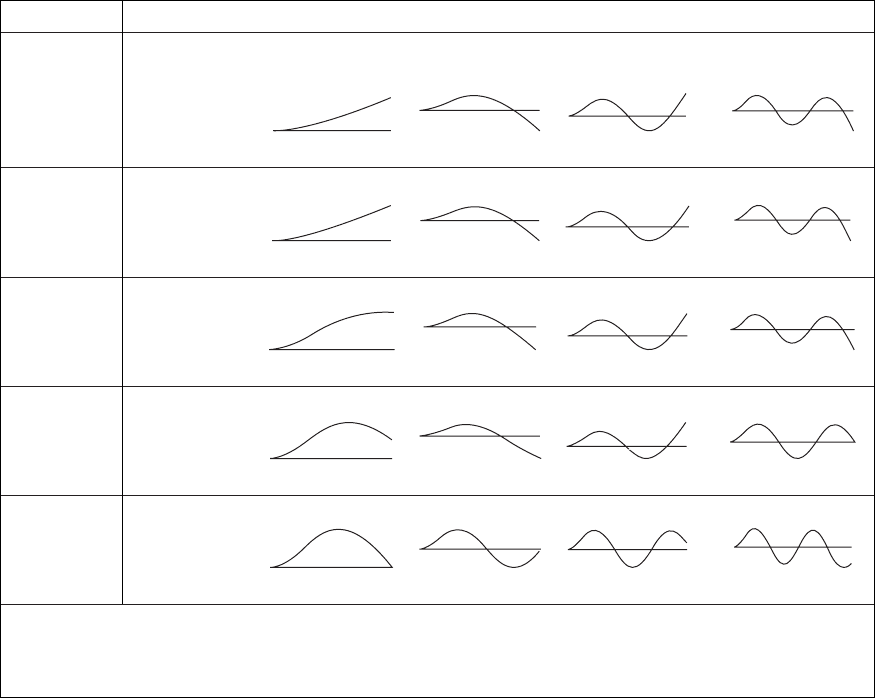
Cantilever beam restrained by a translation spring at the free end The

natural-frequency coefﬁcients and corresponding mode shapes for a cantilever

beam with a translation spring of nondimensional stiffness K

at its free end are

given Table 9.5 and the variations of the lowest three natural-frequency coefﬁ-

cients as a function K

are given in Figure 9.9. From Eqs. (7a) and (7b) in Table

9.2, we see that as K

becomes very large, the characteristic equation and mode

shapes approach the characteristic equation and mode shapes of a beam

clamped at one end and hinged at the other, which are given by Eqs. (3a) and

(3b), respectively, in Table 9.2. On the other hand, as K

becomes very small,

the characteristic equation and mode shapes approach those of a beam clamped

at one end and free at the other, which are given by Eqs. (4a) and (4b), respec-

tively, in Table 9.2. Comparing these limiting cases to the case of a cantilever

carrying a mass at its free end, we see that although the beams have very dif-

ferent physical constraints, they have identical limiting cases. However, there

is one very important difference: when the stiffness of the end spring increases,

the natural frequencies increase whereas for a cantilever beam with a mass, as

the magnitude of the mass increases the natural frequencies decrease. A can-

tilever beam with a translational spring at its free end has been used to model

the vibration characteristics of atomic-force microscope cantilevers.

Beam restrained by translation springs at both ends From Eqs. (8a) and

(8b) in Table 9.2, we see that when K

and K

become very large, the charac-

teristic equation and mode shapes approach the characteristic equation and

mode shapes of a beam pinned at each end, which are given by Eqs. (2a) and

(2b), respectively, in Table 9.2. At the other extreme, as both K

and K

be-

come very small in magnitude, the characteristic equation and mode shapes

approach the characteristic equation and mode shapes of a beam free at both

ends, which are given by Eqs. (5a) and (5b), respectively, in Table 9.2.

584 CHAPTER 9 Vibrations of Beams

U. Rabe, K. Janser, and W. Arnold, “Vibrations of Free and Surface-Coupled Atomic Force

Microscope Cantilevers: Theory and Experiment,” Review Scientiﬁc Instruments, Vol. 67, No. 9,

pp. 3281–3293 (1996).

Design Guideline: When a cantilever beam of mass m

and an end

mass M

is approximated by a single degree-of-freedom system where

the beam stiffness is taken into account and the beam inertia is neg-

lected, the approximation for the ﬁrst natural frequency obtained by us-

ing the single degree-of-freedom model is reasonable to use only when

the ratio of the end mass to the beam mass M

is greater than 2.3.

n  1 n  2 n  3 n  4



‡

0.5969 1.4942 2.5002 3.5000

(n  0.5, n  4)

0 Mode shapes

Node points

None 0.783 0.504, 0.868 0.358, 0.644, 0.906



‡

0.7046 1.5035 2.5022 3.5007

Mode shapes

Node points

None 0.779 0.503, 0.867 0.358, 0.644, 0.905



‡

1.0083 1.5919 2.5207 3.5073

30 Mode shapes

Node points

None 0.739 0.499, 0.861 0.358, 0.643, 0.904



‡

1.1588 1.7876 2.5732 3.5252

100 Mode shapes

Node points

None 0.673 0.489, 0.844 0.356, 0.639, 0.899



*‡

1.2468

†

2.2306

†

3.1869 4.0906

3000 Mode shapes

Node points

None 0.562 0.394, 0.705 0.307, 0.550, 0.792

‡

The natural frequency f

in Hertz as a function of 

is given by Eq. (9.94).

Values of h not including the boundaries.

†

Approaches natural frequency and mode shape for the clamped-hinged boundary conditions.

Beam restrained by torsion springs at both ends From Eqs. (9a) and (9b)

in Table 9.2, it is seen that as both B

and B

become very large, the charac-

teristic equation and mode shapes approach those of a beam clamped at each

end, as given by Eqs. (1a) and (1b), respectively, in Table 9.2. When both B

and B

become very small, the characteristic equation and mode shapes ap-

proach those of a beam pinned at both ends, as given by Eqs. (2a) and (2b),

respectively, in Table 9.2.

Cantilever beam with mass and translation spring at the free end To de-

termine the effects of both a mass and spring attached at the free end of a

cantilever beam, a plot of the ﬁrst natural-frequency coefﬁcient of Case 10 of

Table 9.2 is given in Figure 9.10. It is seen that as K

increases the ﬁrst natu-

ral coefﬁcient increases regardless of the magnitude of the mass ratio M

9.3 Free Oscillations 585

TABLE 9.5

Natural-Frequency Coefﬁcients, Mode Shapes and Interior Node Points for a Cantilever Beam with Spring at Free End:

Case 7 in Table 9.2.

1

0.5

1.5

2.5

3.5

2

Ω

/

Ω

/

Ω

/

Ω

/

FIGURE 9.9

First three natural-frequency coefﬁcients for a cantilever beam with a translation spring at the

free end as a function of the spring stiffness K

FIGURE 9.10

Lowest natural-frequency coefﬁcient for a cantilever beam with a mass and spring attached

to the free end as a function of M

for several values of K

2

1

0.2

0.4

0.6

0.8

1.2

1.4

 0

 3

 10

 30

 100

 300

Ω

/

EXAMPLE 9.2 Beams with attachments: maintaining a constant ﬁrst natural frequency

Consider a cantilever beam whose lowest natural frequency is v

. A mass M

is placed on the free end of the beam, with the requirement that the lowest nat-

ural frequency of this new system be equal to v

. There are two ways in which

this can be done: (1) by changing the cross-sectional properties of the beam

and (2) by adding a translation spring to the mass. To employ the ﬁrst method,

we use Eq. (9.94) and note that

(a)

where is a constant, r is the radius of gyration of the beam

without the mass, r

is the radius of gyration of the beam with the mass,

is the natural frequency of the system with the mass, 

is the natural-

frequency coefﬁcient as determined from Eq. (4a) in Table 9.2, and 

is the

corresponding natural-frequency coefﬁcient as determined from Eq. (6a) in

Table 9.2. Since the requirement is that v

 v

, and the material and length

of both beam conﬁgurations are the same, from Eq. (a) we ﬁnd that

(b)

Thus, we must increase (since 



) the radius of gyration of the beam

with the mass by the ratio (



)

. For example, if the ratio M

 1,

then from Table 9.4 we have that 

 0.3972p and 

 0.5969p. There-

fore, (



)

 (0.5969/0.3972)

 2.26 and r has to be increased by a fac-

tor of 2.26.

The second way to keep the natural frequency constant is to add a trans-

lation spring k

to the mass and adjust the stiffness k

so that natural frequency

remains the same. Since we are implicitly requiring that the beam properties

and geometry be constant for both systems, the task is to ﬁnd for a given value

of M

the value of k

that ensures the ﬁrst natural-frequency coefﬁcient is con-

stant; that is,

(c)

where 

KM1

is the natural-frequency coefﬁcient of the system with the mass

and translation spring. The value of k

(as determined from its non dimen-

sional counterpart K

) is obtained by using Eq. (10a) in Table 2; that is,

(d)

where M

is given and 

 0.5969p is the lowest root of

(e)cos1

2cosh1

2 1  0

 a







b3cos1

2sinh1

2 sin1

2cosh1

24 cos1

2cosh1

2 1  0





KM1

 r



 1E

 c



 c

r

9.3 Free Oscillations 587

588 CHAPTER 9 Vibrations of Beams

which is obtained from Eq. (4a) in Table 9.2. Consequently, Eq. (d) simpli-

ﬁes to

(f)

Since the quantity inside the right-hand brackets does not equal zero, we see

that Eq. (f) is satisﬁed when

(g)

Thus, by choosing a value of K

as indicated in Eq. (g), the system with the

attached mass and translation spring will have the same ﬁrst natural frequency

as the system without the mass and spring. For example, if we again select the

ratio M

 1, and since 

 0.5969p, Eq. (g) leads to K

 (0.5969p)

 12.37. From Table 2.3, we ﬁnd that the stiffness of a cantilever beam with

a load at its free end is k

 3EI/ L

. Therefore, from the deﬁnition of K

Eq. (9.67), we obtain that K

 3k

. Thus, k

 K

/3  (12.37/3)k



4.12k

. In other words, the stiffness of the spring attached at the free end has

to have a stiffness that is about four times that of the stiffness of the beam it-

self when loaded at its free end.

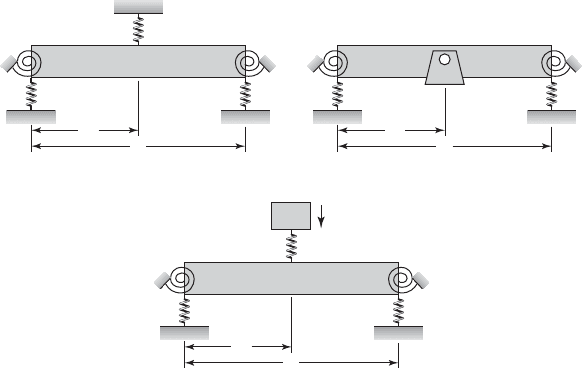
9.3.4 Effects of Stiffness and Inertial Elements Attached

at an Interior Location

In the previous sections, we considered free vibrations of beams that had

springs and inertia elements attached at the boundaries. In this section, we

shall extend these results and examine the free vibrations of beams that have

springs and inertia elements attached to an interior point of the beam. In par-

ticular, we shall determine the natural frequency coefﬁcients and modes

shapes for the three conﬁgurations shown in Figure 9.11: (1) a beam with an

attached translation spring, (2) a beam with an attached mass, and (3) a beam

with an attached undamped single degree-of-freedom system. In all three

cases, it is assumed that the beam has a uniform cross-section. We shall ﬁrst

give the governing equation for each of these systems and then determine the

complete solution only for the case of a beam with an attached single degree-

of-freedom system. It will be shown that special cases of this system are the

beam with an attached mass and the beam with an attached spring. The case

of a beam with a single degree-of-freedom system attached at an interior point

is in effect a beam with a vibration absorber,

which was discussed in the

context of two degree-of-freedom systems in Section 8.6.





a







b3cos1

2sinh1

2 sin1

2cosh1

24 0

This type of analysis has been extended to include two degree-of-freedom systems. See, for

example, M. U. Jen and E. B. Magrab, “Natural Frequencies and Modes Shapes of Beams

Carrying a Two Degree-of-Freedom Spring-Mass System,” J. Vibrations Acoustics, Vol. 115,

pp. 202–209 (April 1993) and H. Ashraﬁuon, “Optimal Design of Vibration Absorber Systems

Supported by Elastic Base,” J. Vibrations Acoustics, Vol. 114, pp. 280–283 (April 1992).

For the attachment of absorbers to more complex systems, see E. O. Ayorinde and G. B. War-

burton, op cit., 1980.

9.3 Free Oscillations 589

For each of the beams shown in Figure 9.11, at the left boundary x  0,

there is a linear translation spring with stiffness k

and a linear torsion spring

with stiffness k

. At the right boundary x  L, there are a linear translation

spring with stiffness k

and a linear torsion spring with stiffness k

. In addi-

tion, as shown Figure 9.11a, a linear translation spring with stiffness k

attached at x  L

,0  L

 L. In Figure 9.11b, a mass M

is attached at

x  L

,0  L

 L. Finally, in Figure 9.11c, an undamped single degree-of-

freedom system of mass M

and stiffness k

is attached to the beam

at x  L

0  L

 L.

The discussion is limited to cases where the axial load p(x,t)  0 and the

externally applied transverse load f (x,t)  0. Furthermore, the oscillations are

assumed to be about the equilibrium position in each case. A primary feature

of the equations in each case is the representation of the discrete element as

an equivalent distributed element by using the delta function d(x).

Linear Spring Attached to an Interior Point x  L

The governing equation of motion for the system shown in Figure 9.11a

is obtained from Eq. (9.40) by representing the discrete spring k

as a

(a)

(b)

(c)

z(t)

It is mentioned that these limits on L

can be removed in certain cases. Consider a cantilever

beam with a mass attached to its free end. One could formulate this situation by considering the

beam to be uniform and to include the mass as part of the boundary condition. On the other hand,

one could assume that the moment and shear are zero at the free end of the beam and that the at-

tached mass on the interior of the beam is moved to L

 L.

FIGURE 9.11

Uniform and homogeneous beam with discrete elements at the boundaries and the following:

(a) a spring attached at x  L

; (b) a mass attached at x  L

; and (c) an undamped single

degree-of-freedom system attached at x  L