Baca A.G., Ashby C.I.H. Fabrication of GaAs Devices

Подождите немного. Документ загружается.

Cleaning and passivation of GaAs and related alloys

of the actual III–As semiconductor in the oxide-etching solution.

Phosphide semiconductors such as InP and InGaP can etch in HCl-

based solutions without the addition of oxidiser. It is worth noting

that the main difference between cleaning and etching solutions

for III–V semiconductors is the addition of an oxidising agent to

form an etching solution that continually produces fresh oxide for

the acid or base to dissolve. Consequently, any wafer that has been

etched may have an oxide on the surface that is at least as thick

and possibly thicker than the native oxide.

Depending on the particular acid or base employed, the surface

stoichiometry of the deoxided surface may be different. A surface

may be either Ga-rich, As-rich or nearly stoichiometric (equal

amounts of Ga and As), depending on whether one element is

preferentially removed by the cleaning solution. Acidic cleaning

solutions tend to leave an As-rich surface due to their preferen-

tial reaction with Ga. Surface studies of GaAs deoxided with HCl

and H

show evidence for Ga-Cl and Ga-PO

bonding before

the surfaces are rinsed with DI water [1]. Water rinsing effectively

removes the anions. However, neither HCl nor H

react effect-

ively with As; this results in a surface enrichment of elemental As.

When these cleans are performed in a nitrogen atmosphere, the As

remains unoxidised. When performed in air, the elemental As can

oxidise upon removal from the solution. More As is seen with the

HCl solution than with H

. As will be discussed in Section 3.3

on passivation, a surface excess of As can be highly undesirable

from an electronic perspective.

In contrast to its low solubility in acids, As dissolves readily in

basic solutions. Consequently, NH

OH solutions preserve a more

nearly stoichiometric surface (Ga/As = 0.99). The As surplus left

by acidic solutions can be removed by a step-wise treatment with

HCl, water, NH

OH and then water. Changing the solvent system

can affect the stoichiometric ratio. While HCl/H

O leaves an As-

rich surface, diluting the concentrated HCl with ethanol instead

of DI water produces a surface closer to stoichiometric (Ga/As =

0.93) [2]. A solution of (NH

)

S will dissolve oxide, but it has the

additional property of reacting with the surface to produce cova-

lently bound sulphides. As discussed extensively in Section 3.3,

this profoundly alters the electronic properties of the surface.

3.2.3 Regrowth of native oxide

Although the oxide may be effectively dissolved while in solu-

tion, a surface oxide begins to form again as soon as the surface

is re-exposed to air. For highly reproducible device results, it

is important to understand this reoxidation process and to take

Cleaning and passivation of GaAs and related alloys

care to handle wafers in a manner that keeps it as reproducible as

possible.

Because an oxide-free surface is hydrophobic, water beads up

on the surface as a deoxided wafer emerges from an aqueous solu-

tion. Exposure to oxygen and reoxidation of the surface begins as

soon as the water withdraws from a portion of the surface. In con-

trast to water, alcohols such as methanol and ethanol wet the deox-

ided surface. If an oxide-free surface is really important, one needs

to deoxide the surface in an oxygen-free atmosphere. This can be

easily accomplished by removing the oxide within a glove box

or a glove bag ﬁlled with nitrogen or argon. The deoxided wafer

should ideally be kept under nitrogen or argon to keep it oxide free.

Unfortunately, the transfer of the wafer into a tool for subsequent

processing, such as metallisation, will almost certainly re-expose

it to some oxygen unless a nitrogen-purged load lock is part of

the system. The base pressure of a typical electron beam metal

evaporator is a few 10

−7

torr. At this pressure, the time to adsorb

a monolayer of gas is a few seconds. It also takes tens of minutes

to pump the system down to that level. A monolayer can form in

a few milliseconds at atmospheric pressure. Since most of these

tools do not include load locks, some reoxidation is inevitable.

The reoxidation of a deoxided GaAs surface is characterised

by at least two types of reaction sites [2]. One site is extremely

reactive with even trace oxygen in a nitrogen atmosphere. Surface

studies that monitor the O 1(s) signal are more sensitive to the total

amount of oxygen present than are the more typical XPS (X-ray

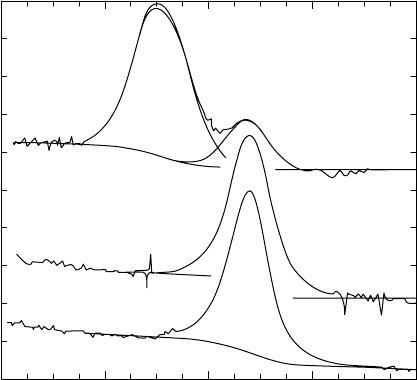
photoelectron spectroscopy) studies (FIGURE 3.1) that monitor

the oxidation state of Ga and As species at the surface. Oxide

removal under nitrogen using 1 : 20 NH

OH/H

O or using 1 : 10

conc. HCl/ethanol leave surfaces with 7 and 4 atomic %, respect-

ively, as measured by the O 1(s) signal. Wafers cleaned in air

exhibit 2–3 times more residual oxide signals than those cleaned

under nitrogen gas originating as boil-off nitrogen from a liquid

nitrogen tank (the most common type of nitrogen gas available

in fabs and labs). The lower value achieved with HCl/ethanol is

probably due to a reduced time of exposure to trace oxygen in the

nitrogen gas while the ethanol wets the surface before loading in

the UHV chamber for analysis.

Additional sites on the surface reoxidise more slowly over a

period of minutes, as measured by changes in the photolumines-

cence intensity. Photoluminescence as a method for monitoring

surface composition is discussed in Section 3.3.2. The most uni-

form device results may be expected when care is taken to perform

subsequent process steps with the times between and the times for

those subsequent steps being as uniform as possible following

deoxidation. The presence of oxygen at the interface between a

Cleaning and passivation of GaAs and related alloys

1334 1329 1325 1321 1316

binding energy, eV

N(E)/E

As(2p

3/2

)

native oxide

HCl/EtOH

FIGURE 3.1 As(2p

3/2

) XPS spectra showing chemical shift for As bound

to O in native oxide versus “oxide-free” surfaces.

contact metal and GaAs can affect the process metallurgy and the

formation of defects at the interface, so consistency in cleaning

can be important in determining consistency in contact behaviour.

The structure of the surface oxide can depend on how it was

formed. When an oxide is grown by thermal oxidation at 420

◦

Cto

a thickness of about 4 nm, the oxide is Ga-rich with an As gradi-

ent that has more As-oxide present as one moves towards the free

surface. In contrast, an ECR-plasma-grown oxide of comparable

thickness is nearly stoichiometric (equal amounts of Ga and As)

and contains a larger amount of As

versus As

than does the

thermal oxide [3]. These differences may be due to the products of

the thermal oxidation being determined by their relative thermo-

dynamic stability while the plasma oxidation is controlled more by

the kinetics of the reactions. XPS results suggest that the thermal

oxide is somewhat closer to Ga

while the ECR plasma oxide is

closer to the stoichiometric GaAsO

. In either case, the quality of

the semiconductor-oxide surface is equally poor in terms of inter-

face charges in C-V measurements after deposition of PECVD

SiO

and Al metallisation. Composition, structure and electronic

surface quality are the topics of Section 3.3.

3.3 PASSIVATION OF GaAs

The term “passivation” carries with it some rather distinct yet

related connotations. The ﬁrst deals with the alteration of the

Cleaning and passivation of GaAs and related alloys

surface of the semiconductor to achieve more favourable electronic

properties than those obtained with the native oxide. The second

has to do with protection from reaction with the ambient over the

lifetime of the device. Since reaction with the ambient, especially

oxygen, can alter the electronic properties, even a device with

excellent initial operating characteristics may gradually degrade

over time. The third common use of the term “passivation” relates

to the deactivation of dopants or defects through reaction with

readily diffusing species such as H atoms. Surface treatments

and especially plasma processing conditions can be a source of

hydrogen that can diffuse deeply into the semiconductor before

reacting with a dopant or a defect. While defect passivation is

generally desirable, dopant passivation alters free carrier concen-

trations and can negatively affect device performance. This third

type of “passivation” will be discussed in Section 5.7.2.

We will begin this section with a discussion of the physical and

chemical issues that affect the important surface and interfacial

electronic properties of GaAs. Since reaction with the ambient,

especially oxygen, can alter critical electronic properties, we will

conclude with a discussion of some methods that seek to isolate

the surface from such effects.

3.3.1 Electronic properties of the GaAs surface

The bulk electronic properties of semiconductors in general and

GaAs in particular were discussed in Section 2.2. Many of the con-

cepts, such as band structure and bandgap, are derived assuming

the three-dimensional translational symmetry of an inﬁnite bulk

crystal. However, the presence of a surface immediately introduces

different boundary conditions that make the bulk description no

longer adequate. The most signiﬁcant effect of the surface is the

introduction of additional energy levels that may lie within the

forbidden energy gap between the valence and conduction bands.

The number and energy of these levels, called surface states, will

determine how important they are in controlling the electronic

behaviour near the surface.

An abrupt termination of the three-dimensional crystal lattice

would leave surface atoms coordinatively unsaturated with many

unpaired electrons; this would produce an unacceptably high-

energy state. In reality, this condition is avoided by either surface

reconstruction or reaction with other chemical species that may be

present.

In surface reconstruction, the surface atoms move from their

hypothetical bulk positions into positions where they can bond

with each other to reduce the total energy. There are a vari-

ety of different reconstructions that can occur, depending on the

Cleaning and passivation of GaAs and related alloys

particular exposed crystal face and whether the surface is primar-

ily terminated by Ga or As atoms. Since the GaAs(100) surface

is of the greatest technological importance, we will restrict most

of our discussion to that crystal face. However, any mesa-type

device will have other crystal faces exposed on the sidewalls. The

ideal GaAs(110) surface, which is a natural cleavage facet and

can be obtained as vertical facets by crystallograpically selective

etching (Section 4.3.2), is supposed to be free of surface states

within the bandgap; however, real device facets can be expected

to deviate somewhat from ideal since they are not perfectly planar.

The kinks and steps found on surfaces that are not planar at the

atomic level can introduce additional energy states. Many studies

of the surface structure of GaAs(100) using LEED (low-energy

electron diffraction) or RHEED (reﬂection high-energy electron

diffraction) have been reported. From the LEED electron diffrac-

tion patterns, it is possible to determine the crystal symmetry of

the surface layers; from the LEED geometry, the possible atomic

arrangements are deduced. There are a number of reported recon-

structions for GaAs(100) depending on the history of the surface,

and they have different work functions, i.e. energies relative to

the vacuum level. This is to be expected since they will have dif-

ferent surface electronic states that determine the work function.

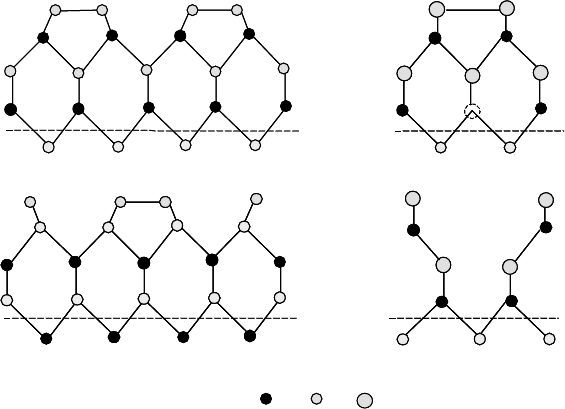
Some surface geometries are shown in FIGURE 3.2. While it is

possible to measure structures of carefully prepared samples under

ultra-high-vacuum (UHV) conditions (<10

−8

torr), it is important

C(2×1)

with S

(100)

C(2×1)

with S

(100)

GaAs S

(2×4)

(100)

C(4×4)

As rich

(100)

FIGURE 3.2 Side view of C(4×4) and (2×4)GaAs(100) and C(2×1)S of

sulphided surface.

Cleaning and passivation of GaAs and related alloys

to remember that the surface of an actual device will not usually

have a perfectly planar topography, exposing only one crystal

plane, nor will it be in UHV. Therefore, great care must be taken

in concluding which scientiﬁc studies will map appropriately onto

the situation for a particular device.

The thermal history of the wafer can profoundly inﬂuence its

surface structure. It is common to describe a surface geometry

using (A×B) nomenclature. For example, the (2×4) surface recon-

struction refers to a two-dimensional unit cell that is 2× larger in

one direction and 4× larger in the other direction compared to

the bulk unit cell. If a GaAs wafer capped with amorphous As

in an MBE machine to prevent oxidation is heated in vacuum to

380

◦

C, it assumes a C(4×4) geometric structure with 1 mono-

layer of adsorbed As. If it is further heated to 480

◦

C, it assumes

a(2×4) geometry. Further heating to 600

◦

C without an As over-

pressure converts it to (4×1) with a slight excess of Ga on the

surface [4]. Reactions with species such as oxygen or sulphur pro-

duce other changes in the surface geometry. While it is possible

to obtain well-deﬁned geometries under controlled vacuum con-

ditions, great care must be taken in extrapolating the results to

real-world wafers undergoing device fabrication. The character of

the surface may be very different from that described in the literat-

ure, and very different behaviour may result when that is the case.

Nevertheless, such studies can provide starting points for thinking

about the condition of a particular wafer.

An alternative way to lower the surface energy is by reaction

with the ambient, especially with oxygen, to form an oxide sur-

face. This will generally be the condition of the GaAs surface

unless deliberate effort has been taken to remove the oxide and

replace it using a more favourable surface reaction. A major goal

for many years has been the achievement of an insulator/III–V

interface of the same quality as the nearly ideal SiO

/Si inter-

face that is the foundation of CMOS technology. Unfortunately,

unlike the SiO

/Si interface with its extremely low density of

interface states (as low as 10

/cm

) and a very high-quality

oxide yielding trapped charge densities of about 10

/cm

, the

oxides of III–V semiconductors suffer from many interface states

that are located within the bandgap. This is true whether the oxide is

formed thermally or by plasma oxidation. For GaAs with its native

oxide, the density of these interface states is in excess of 10

/cm

roughly one state for every 100 surface atoms. This high interface-

state density results in Fermi level “pinning”, as discussed

below, and has so far precluded a successful metal-insulator-

semiconductor (MIS) technology in the III–V systems. The

majority of “passivations” involve reactions whose products are

meant to be more electronically favourable than the native oxide.

Cleaning and passivation of GaAs and related alloys

Replacement of the native oxide with an alternative interfacial

species is essential because the formation of the native oxide itself

can generate defects that ensure poor electronic behaviour [5].

During the initial period of oxidation with atomic oxygen, even

before a general disruption of the GaAs lattice or the formation

of unstable arsenic oxides that can liberate elemental As (Sec-

tion 10.2.1), oxygen atoms displace the top-layer As atoms and

bind to the second-layer Ga atoms. Scanning tunnelling micro-

scopy (STM) of GaAs (100) with the (2×4) surface structure has

shown that the displaced As atoms localise in surface trenches and

on top of surface As-dimer rows to place As atoms in what would

normally be Ga sites, forming the donor As

antisite defect and

an acceptor state that may be associated with an undimerised As

atom with a half-ﬁlled dangling bond. Increases in total O cover-

age lead to preferential formation of oxygen dimers on the surface

with reduction of the undimerised As sites. Such dimer formation

appears to be the natural step for oxidation with molecular oxygen.

In addition, metallic clusters of As atoms can form as the oxygen

reaction proceeds. With sufﬁcient oxidation, the Fermi level is

pinned for p-GaAs somewhere between 0.75 and 0.5 eV, which

are the energy levels for singly and doubly ionised As

antisite

defects.

Vapour deposition of Ga

oxide onto GaAs in vacuum can

minimise the formation of As-oxides. This is signiﬁcant because

is thermodynamically unstable in contact with GaAs, with

the reaction

+ 2GaAs → Ga

+ 4As

being thermodynamically favoured (ΔG

=−62 kcal/mol).

Vapour-deposited Ga-oxides [6] have shown some of the best elec-

tronic behaviour to date (FIGURE 3.9), but problems still remain

(Section 3.3.5).

An important point to remember when considering surface reac-

tions is that even a partial monolayer of reactedmaterialcan domin-

ate the electronic structure of the surface or interface. The chemical

bonding of the surface monolayer deﬁnes the energetics of the

interface states and determines whether they lie within the bandgap

(undesirable) or not (highly desirable). Therefore, the preparation

and cleaning of the surface before any passivation process may

totally dominate the results and great care must be taken to ensure

reliable and reproducible results. When differences between liter-

ature predictions and personal fabrication results occur, they can

often be traced to differences in the starting surface condition.

A word of caution is in order about relying simply on surface

stoichiometry or geometry as a predictor of electronic properties.

Cleaning and passivation of GaAs and related alloys

The surface state energies and the density of states at each energy

are not merely dependent on what chemical elements are at the

surface. Details of the bonding conﬁguration of those atoms will

determine the energetics of the surface, and the same nominal com-

position can display quite different bonding. The standard methods

of identifying surface chemical composition, like Auger electron

spectroscopy (AES), X-ray photoelectron spectroscopy (XPS),

secondary ion mass spectrometry (SIMS) or energy-dispersive

X-ray analysis (EDS, EDX or EDAX) typically are not sensit-

ive to species that are present below a tenth of a percent. However,

elements present at even lower levels can be not only important but

even dominant in determining surface electronic properties if they

are properly positioned within the forbidden gap and exhibit rapid

recombination kinetics. Additionally, it is possible for surfaces

of different elemental compositions to assume the same geometric

pattern as measured by LEED, so having the same structure should

not be construed as convincing evidence of the same chemical

composition and same electronic properties.

Either reconstruction or chemical reaction will introduce surface

states that generally serve as traps for free carriers. These levels

may be quite discrete or they may be spread over a band. Both

electron and hole surface traps are known. Elements that are more

electronegative than Ga and As, such as oxygen, act as electron

acceptors. The more electronically deleterious states tend to lie

toward the middle of the gap and are called “midgap states”.

3.3.1.1 Midgap states and Fermi level pinning

The Fermi level and its position relative to the valence band max-

imum, VBM or E

, and conduction band minimum, CBM or

, are discussed for bulk semiconductors in Section 2.2.2. The

presence of midgap states at the surface or interface can have a

profound effect on the Fermi level position relative to the VBM

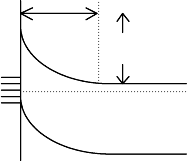
and CBM near the surface, as illustrated in FIGURE 3.3 for an

n-type semiconductor.

FIGURE 3.3 Fermi level pinning

at energy of midgap surface states.

When there are surface states lying below the Fermi level, they

will be ﬁlled by electrons from the conduction band. When there

are N

states per cm

below the Fermi level, the surface will acquire

a negative charge equal to −eN

/cm

. This will repel electrons

from the surface region and produce a depletion region nearly

devoid of free electrons which will have a positive space-charge

approximately equal to +eN

d, where N

is the donor atom con-

centration and d is the depth of the space-charge region. There

is a strong electric ﬁeld directed towards the surface that opposes

the ﬂow of more electrons into the unoccupied surface states. The

Fermi level in the bulk is aligned with the top of the ﬁlled surface

Cleaning and passivation of GaAs and related alloys

states, and the energy of the surface is raised relative to the bulk

by the surface potential, φ

. An exact determination of φ

requires

the solution of Poisson’s equation with appropriate boundary con-

ditions. For simplicity, one may assume that the space charge ρ is

equal to eN

d from the surface to a depth, d, where d = N

The surface potential, φ

, is then given by eN

/2N

, where  is

the semiconductor permittivity. The energy by which the bands

are raised at the surface is approximately ΔE = eφ

. If the surface

potential is sufﬁciently high that the valence band at the surface

becomes closer to the Fermi level than the conduction band is,

the near-surface region becomes effectively p-type and an inver-

sion layer is formed. The response of these various quantities to

externally applied voltages is discussed in Section 7.2.1.

When there is an extremely high density of midgap surface

states, as in the case of GaAs(100), the Fermi level is effectively

pinned at the energy corresponding to that of the midgap states.

Changing applied voltages may merely move carriers into and out

of surface states, leaving the position of the Fermi level unchanged

and “pinned” at the surface-state energy. The primary goal of sur-

face passivation is the unpinning of the Fermi level. Changing the

chemical composition of the surface layer will change the energy

of the dominant surface states. If the new surface states are moved

close to the VBM or CBM or, even better, are moved out of the

forbidden gap, the Fermi level can become unpinned. However,

changing the surface composition may serve merely to pin the

Fermi level at another position within the gap if there is still a high

density of midgap states at the new energy position.

Fermi level pinning can occur at a wide range of inter-

faces. It is seen at “clean” semiconductor surfaces grown by

MBE, naturally oxidised surfaces, chemically modiﬁed sur-

faces, semiconductor/insulator interfaces, metal/semiconductor

interfaces and regrown semiconductor/semiconductor interfaces.

While the remainder of this chapter will be focusing on chem-

ical modiﬁcation of exposed surfaces, most work on Fermi

level pinning has been directed towards the understanding of the

metal/semiconductor interface because of its importance in device

performance.

A number of models have been put forth to explain the ori-

gin of Fermi level pinning and its effect on the Schottky barrier

height [7] of Schottky contacts (Chapter 7). These include the

uniﬁed defect (UDF) or advanced uniﬁed defect (AUD) models

of Spicer et al., the metal-induced-gap state (MIGS) model of

Heine and later Tersoff, the disorder-induced gap state (DIGS)

model of Hasegawa and Ohno, and the effective work function

(EWF) model of Woodall and Freeouf. The proposed origin of

the pinning states varies with the model employed. In the UDF

Cleaning and passivation of GaAs and related alloys

model, pinning is ascribed to deep levels related to stoichiometric

variation, especially the As antisite. In the MIGS model, the

midgap states originate in the penetration of the metal wave func-

tion into the semiconductor. In the DIGS model, the pinning states

result from the loss of two-dimensional periodicity due to disorder

of the bonding at the interface. In the EWF model, pinning occurs

at metallic clusters formed by precipitation of As at the interface.

The most appropriate model for a particular case varies with the

details of the interface.

It is important to remember that band bending is caused by the

total surface charge. This charge is the combination of surface-

trapped charge due to surface states lying within the forbidden

gap and surface-ﬁxed charge, i.e. ionic charge on the surface that

may not be related to the surface traps. With only a surface-ﬁxed

charge, the Fermi level can still move freely. In contrast, with

sufﬁciently high densities of surface-trap states, the Fermi level

is pinned and neither bulk doping nor externally applied electric

ﬁelds can move it.

Band bending due to surface-ﬁxed charge can repel minority

carriers sufﬁciently to reduce the surface recombination velocity

(Section 3.3.1.2). The change in the surface recombination velo-

city as a function of forward injection parameters can provide a

measurement of surface-ﬁxed charge and help distinguish contri-

butions from ﬁxed charge and surface states. Better results will be

obtained if injection of carriers by absorption of light is avoided.

3.3.1.2 Recombination and surface recombination

The free carriers in a semiconductor participate in a dynamic pro-

cess with the constant generation and recombination of individual

carriers determining their concentration in a particular region of

the material. The details of these processes are outside the scope

of this book and are discussed quantitatively in many books on

semiconductor and device physics [8]. The free carrier concen-

tration in a particular region of the wafer or device is determined

by the balance between generation and recombination. Dopant

atom concentrations, temperature and illumination are the factors

that dominate the generation process. The equilibrium concentra-

tions of electrons and holes are discussed in Section 2.2.2 for the

situation without illumination. Illumination will alter these con-

centrations by producing an equal number of electrons and holes

when and where photons are being absorbed in the material. The

location of creation depends on the absorption coefﬁcient of the

semiconductor; there is an exponential decrease in the number

of photogenerated carriers as the light penetrates deeper into the

material. The carriers generated within a region of depth dx is