ASME Section VIII div 2 2010. ASME Boiler and Pressure Vessel Code. Alternative Rules
Подождите немного. Документ загружается.


2010 SECTION VIII, DIVISION 2
5-99
5.E.4.4 The elasticity matrix
[]E for the triangular and square patterns that are used in the stress analysis
of the effective solid plate are provided in Tables 5.E.8 and 5.E.9, respectively.
5.E.5 Pressure Effects in Tubesheet Perforations
5.E.5.1 The effect of pressure in the perforations is not directly included in the stress analyses of the
effective solid plate. Pressure in the tubesheet perforations tends to expand the perforated portion of the
plate. This expansion is resisted by the solid rim and by the ligaments themselves. The effect of this
pressure on the local stresses in the ligaments can be included in the stress analysis results by use of the
superposition principle.
5.E.5.2 In order to include the effect of pressure, an additional analysis needs to be performed. In this
analysis, the boundary conditions are shown in Figure 5.E.2 (c). These boundary conditions can be achieved
by superposing the results from the boundary conditions shown in Figures 5.E.2 (a) and 5.E.2 (b),
respectively. The solution for the hydrostatic compression boundary condition case, see Figure 5.E.2 sketch
(a) is
***
rzs
p
θ
σσσ
===− for tubes welded on the tubeside or
***
rzt
p
θ
σσσ
=
==− for tubes welded on the
shellside. The solution for the boundary conditions of Figure 5.E.2 (b) can be obtained from the stress
analyses considering the loading conditions shown.
5.E.5.3 A further modification to the boundary conditions used in paragraph 5.E.2 can be used to obtain
more exact results. The modified boundary conditions are shown in Figure 5.E.3.
5.E.5.4 The stress results obtained using the boundary conditions shown in Figures 5.E.2 and Figure 5.E.3
must be superposed before
m
P ,
Lb
PP+ , and
Lb
PPQ
+
+ stresses are evaluated and before transforming
the cylindrical coordinate stresses to the local x-y-z coordinate system for computing
Lb
PPQF
+
++
stresses.
5.E.6 Protection Against Plastic Collapse
5.E.6.1 Uniform Hole Pattern – The following equations shall be used for a perforated plate with a uniform
hole pattern. The equivalent stresses
m
P ,
Lb
PP
+
, and
Lb
PPQ
+
+ are determined from the in-plane stress
components determined by stress analysis of the equivalent solid plate. The loads to be considered in the
design shall include, but not be limited to, those given in Table 4.1.1. The load combinations that shall be
considered for each loading condition shall include, but not be limited to those given in Table 4.1.2.
a) General Primary Membrane Equivalent Stress
()
m
P – evaluate the through thickness stress distribution
from the numerical analysis of the equivalent plate for mechanical plus pressure loading. The membrane
stress is determined by linearization of the through thickness stress distribution. The maximum stress
intensity,
m
P , shall satisfy the following:
*
m
m
P
S
μ
≤ (5.E.5)
b) Primary Membrane (General or Local) Plus Primary Bending Equivalent Stress
()
LB
PP+ – determine
the linearized surface stresses from the numerical analysis of the equivalent plate for mechanical plus
pressure loading. The maximum stress intensity,
Lb
PP
+
, shall satisfy the following:
2010 SECTION VIII, DIVISION 2
5-100
()
*
1.5
LbPS
m
PPK
S
μ
+
≤
(5.E.6)
where
0.5
2
23
1.07802 0.342503 1.50452
1 0.0706632 1.15182 0.158343
PS
K
ββ
ββ β
⎡⎤
−+
=
⎢⎥
−++
⎣⎦
(5.E.7)
*
*
**
11
r
r
or where
θ
θ
σ
σ
ββ
σσ
=−≤≤ (5.E.8)
5.E.6.2 Primary Membrane (General or Local) Plus Primary Bending Plus Secondary Equivalent Stress
()
LB
PPQ++ – if a fatigue analysis is required, determine the linearized surface stresses from the numerical
analysis of the equivalent plate for mechanical, pressure, and thermal loading. The maximum primary plus
secondary equivalent stress,
LB
PPQ++, shall satisfy the following where
P
S
K is computed using Equation
(5.E.7).
*
()
LB PS
P
S
PPQK
S
μ
++
≤
(5.E.9)
5.E.7 Protection Against Cyclic Loading
5.E.7.1 Stress Components From The Equivalent Plate – The stress components
*
x
σ
,
*
y
σ
,
*
z
σ
,
*
x
y
τ
,
*
x
z
τ
,
and
*
yz
τ
, are determined from the stress analysis of the equivalent solid plate.
a) Triangular Hole Pattern – For material constants evaluated using Option A, B, or C (see paragraph
5.E.4.3), the perforated plate displacements and stresses are minimally affected by the orientation with
respect to the hole pattern. Therefore, the numerical stress results can be used directly in the
evaluation.
b) Square Hole Pattern – The determination of the stress results depends on the material property model.
1) For material constants evaluated using Option A or Option C (see paragraph 5.E.4.3), the
perforated plate displacements and stresses are minimally affected by the orientation with respect
to the hole pattern. Therefore, the numerical stress results can be used directly in the evaluation.
2) For material constants evaluated using Option B, the square pattern displacements do not
dependent strongly on the orientation with respect to the hole pattern; therefore, the axisymmetric
approximation provides sufficiently accurate strain results. However, the stress components have
directional dependence with the stiffness properties. Therefore, the stress components shall be
determined using the equations in Table 5.E.10 where the elasticity matrix is dependent on the
orientation of the hole pattern with respect to the equivalent plate cylindrical coordinate system.
Using the axisymmetric strains in the plate, the non-axisymmetric nominal stresses in the pitch and
diagonal directions for the square pattern perforations can be obtained from the equations below:
() () ()()
** ****
1
2
rr pdr
pa
GG
θ
σ
σεε
=−− − (5.E.10)
标准分享网 www.bzfxw.com 免费下载

2010 SECTION VIII, DIVISION 2
5-101
() () ()()
** ****
1
2
pdr
pa
GG
θ
θθ
σ
σεε
=+− − (5.E.11)
() () ()()
** ****
1
2
rr pdr
da
GG
θ
σ
σεε
=+− −
(5.E.12)
() () ()()
** ****
1
2
pdr
da
GG
ϑ
θθ
σ
σεε
=−− − (5.E.13)
()
**
zz
a
σσ
= (5.E.14)
()
**
rz rz
a
σσ
=
(5.E.15)
c) For both the triangular and square pattern perforated plates, the hydrostatic compression stress must be
superposed prior to transformation to the x-y-z Cartesian coordinate system (see Figure 5.E.4 or 5.E.5)
for computing the peak stresses.
5.E.7.2 Stress Components For Fatigue Assessment – The stress components for the fatigue assessment
are computed using the following equations.
a) Stress Results In The Perforated Region Of The Plate – the stress multipliers
x
K ,
y
K ,
x
y
K ,
x
z
K , and
yz
K
for triangular and square hole patterns are given in Tables 5.E.11 through 5.E.15 and 5.E.16
through 5.E.18, respectively. The stress orientation associated with the stress multipliers for triangular
and square hole patterns are shown in Figures 5.E.4 and 5.E.5, respectively. The stress results in
paragraph 5.E.7.1 must be transformed to the local x-y-z Cartesian coordinate system before the stress
multipliers can be applied.
()
***
11
*
1
x
xyyxyxy
KKK
θ
σ
σσστ
μ
== + + (5.E.16)
()
**
12
*
1
zxzxzyzyz
KK
θ
σ
τττ
μ
== + (5.E.17)
13
0.0
σ
= (5.E.18)
At the tubeside surface of the plate,
22 t
p
σ
=− (5.E.19)
At the shellside surface of the plate,
22
s
p
σ
=− (5.E.20)
Between the surfaces of the plate through the thickness,
()
()
***
22 11
*
hzxy
z
E
p
E
σνσ σνσσ
⎛⎞
⎡⎤
=−+ −+
⎜⎟
⎣⎦
⎝⎠
(5.E.21)
and
2010 SECTION VIII, DIVISION 2
5-102
23
0.0
σ
= (5.E.22)
33 h
p
σ
=− (5.E.23)
b) Stress Results At The Rim – the region of the perforated plate outside the diameter
o
D is called the
plate rim (see Figure 5.E.1). The stress components for the fatigue assessment at the outer most hole
shall be computed using the following equations. The stress results in paragraph 5.E.7.1 must be
transformed to the local x-y-z Cartesian coordinate system before the stress multipliers can be applied
11, , , , , ,rim rim rim rim r rim r rim
KK
θθθ
σ
σσσ
== + (5.E.24)
12, , , 12,rim z rim rz rim rim
K
θ
σ
ττ
==
(5.E.25)
13,
0.0
rim
σ
= (5.E.26)
At the tubeside surface of the plate,
22,rim t
p
σ
=−
(5.E.27)
At the shellside surface of the plate,
22,rim s
p
σ
=− (5.E.28)
Between the surfaces of the plate through the thickness,
22, 11, , , ,
()[( )]
rim rim h z rim r rim rim
p
θ
σ
νσ σ νσ σ
=−+−+ (5.E.29)
and
23,
0.0
rim
σ
=
(5.E.30)
33,rim h
p
σ
=− (5.E.31)
The stress multipliers are computed using the information in Tables 5.E.11 through 5.E.18, as applicable,
with the following modifications.
,
*1.0
rim x
K K evaluated at
θ
μ
== (5.E.32)
,
*1.0
rrim y
K K evaluated at
μ
== (5.E.33)
,
*1.0
rz rim xz
K K evaluated at
μ
==
(5.E.34)
c) Thermal Skin Stresses – the temperature gradient through the thickness of a perforated plate can be
closely approximated by a step change in the metal temperature near the surface of the plate.
Significant thermal stresses develop only in the skin layer of the plate at the surface where the
temperature change occurs and the thermal stresses in the remainder of the plate are negligible. The
thermal skin stresses at any location on the surface of the equivalent solid plate are given by Equation
(5.E.35). The thermal skin stresses should be added to the stresses determined from the numerical
analysis
标准分享网 www.bzfxw.com 免费下载

2010 SECTION VIII, DIVISION 2
5-103
()
()
**
1
ms
rr skin
ET T
K
α
σσ
ν
⎡⎤
−
==
⎢⎥
−
⎢⎥
⎣⎦
(5.E.35)
with
**2
**2*3
9.43983 421.179 6893.05
1 4991.39 6032.92 1466.19
skin
K
μμ
μ
μμ
−+
=
++ −
(5.E.36)
d) Stress Results In The Solid Rim And Other Locations – the stress components from the solid rim and
other locations that do not contain perforations can be used directly in the fatigue assessment.
5.E.7.3 Stress Range For Fatigue Assessment – The stress range to be used in the fatigue assessment is
determined in accordance with paragraph 5.5.
5.E.8 Nomenclature
A
unit cell area
0
A tube open area
α
coefficient of thermal expansion
β
biaxiality stress factor
*d effective tube hole diameter
t
d nominal outside diameter of tubes
o
D equivalent diameter of outer tube limit circle
E
modulus of elasticity for tubesheet material at tubesheet design temperature
t
E modulus of elasticity for tube material at tube design temperature
*
E effective Young's modulus for the perforated plate for a triangular hole pattern
*
p
E effective Young's modulus for the perforated plate for a square hole pattern – pitch direction
*
d
E effective Young's modulus for the perforated plate for a square hole pattern – diagonal
direction
*
z
E
effective Young's modulus for thickness direction loading for perforated plate
11
E coefficient of the elasticity matrix
12
E coefficient of the elasticity matrix
13
E coefficient of the elasticity matrix
21
E coefficient of the elasticity matrix
22
E coefficient of the elasticity matrix
23
E coefficient of the elasticity matrix
31
E coefficient of the elasticity matrix
32
E coefficient of the elasticity matrix
33
E coefficient of the elasticity matrix
44
E coefficient of the elasticity matrix
55
E coefficient of the elasticity matrix
66
E coefficient of the elasticity matrix
2010 SECTION VIII, DIVISION 2
5-104
0
F Poisson’s ratio factor
1
F Poisson’s ratio factor
G shear modulus for the perforated plate,
(
)
21GE
ν
=
+
*
G effective shear modulus for the perforated plate for a triangular hole pattern
*
p
G effective shear modulus for the perforated plate for a square hole pattern – pitch direction
*
d
G effective shear modulus for the perforated plate for a square hole pattern – diagonal direction
*
z
G effective Shear modulus for transverse shear loading for perforated plate.
h tubesheet thickness
L
K stress intensity load factor (see Part 5)
P
S
K stress multiplier applied to surface stresses to determine local membrane plus bending
primary stress averaged across the width of the ligament but not through the thickness.
s
kin
K stress multiplier for the thermal skin stress
x
K Local Stress Multiplier for perforated material
y
K Local Stress Multiplier for perforated material
x
y
K Local Stress Multiplier for perforated material
x
z
K Local Stress Multiplier for perforated material
yz
K Local Stress Multiplier for perforated material
,rrim
K Local Stress Multiplier for solid rim plate
,rz rim
K Local Stress Multiplier for solid rim plate
,rim
K
θ
Local Stress Multiplier for solid rim plate
tx
l expanded length of tube in tubesheet
(
)
0.0 1.0
tx
l≤≤ . An expanded tube-to-tubesheet joint
is produced by applying pressure inside the tube such that contact is established between the
tube and tubesheet. In selecting an appropriate value of expanded length, the designer shall
consider the degree of initial expansion, differences in thermal expansion, or other factors that
could result in loosening of the tubes within the tubesheet (see Figure 4.18.2.Sketch (b)).
μ
ligament efficiency
*
μ
effective ligament efficiency
ν
Poisson’s ratio for the perforated plate
*
ν
effective Poisson’s ratio for the perforated plate for a triangular hole pattern
*
p
ν
effective Poisson’s ratio for the perforated plate for a square hole pattern – pitch direction
*
d
ν
effective Poisson’s ratio for the perforated plate for a square hole pattern – diagonal direction
b
P primary bending stress intensity.
m
P general primary membrane stress intensity.
L
P local primary membrane stress intensity.
p
tube pitch
*
p
effective tube pitch
s
p
shellside pressure acting on the surface of the plate
t
p
tubeside pressure acting on the surface of the plate
标准分享网 www.bzfxw.com 免费下载
2010 SECTION VIII, DIVISION 2
5-105
h
p
pressure acting in the hole of the plate
1
p
tubeside pressure acting on the surface of the plate
2
p
shellside pressure acting on the surface of the plate
3
p
shellside pressure acting on the surface of the rim
4
p
pressure acting on the shell
5
p
tubeside pressure acting on the surface of the rim
Q secondary stress intensity.
o
r radius to outermost tube hole center
ρ
tube expansion depth ratio; 0.0 1.0
ρ
≤
≤
m
S allowable stress intensity for the perforated plate material at the design temperature
P
S
S allowable limit on the primary plus secondary stress range.
tm
S allowable stress intensity for the tube material at the design temperature
*
r
ε
calculated radial strain from the stress analysis of a equivalent perforated plate
*
θ
ε
calculated circumferential strain from the stress analysis of a equivalent perforated plate
*
z
ε
calculated axial strain from the stress analysis of a equivalent perforated plate
*
r
θ
γ
calculated shear strain from the stress analysis of a equivalent perforated plate
*
rz
γ
calculated shear strain from the stress analysis of a equivalent perforated plate
*
z
θ
γ
calculated shear strain from the stress analysis of a equivalent perforated plate
11
σ
normal stress in the 1-direction
12
σ
shear stress in the 1-direction on the 2-plane
13
σ
shear stress in the 1-direction on the 3-plane
22
σ
normal stress in the 2-direction
23
σ
shear stress in the 2-direction on the 3-plane
33
σ
stress in the 3-direction
11,rim
σ
normal stress in the 1-direction at the rim
12,rim
σ
shear stress in the 1-direction on the 2-plane at the rim
13,rim
σ
shear stress in the 1-direction on the 3-plane at the rim
22,rim
σ
normal stress in the 2-direction at the rim
23,rim
σ
shear stress in the 2-direction on the 3-plane at the rim
33,rim
σ
stress in the 3-direction at the rim
*
r
σ
normal radial stress calculated from the stress analysis of a equivalent perforated plate
*
θ
σ
normal circumferential stress calculated from the stress analysis of a equivalent perforated
plate
*
z
σ
normal axial stress calculated from the stress analysis of a equivalent perforated plate
*
r
θ
τ
normal shear stress calculated from the stress analysis of a equivalent perforated plate
2010 SECTION VIII, DIVISION 2
5-106
*
rz
τ
normal shear stress calculated from the stress analysis of a equivalent perforated plate
*
z
θ
τ
normal shear stress calculated from the stress analysis of a equivalent perforated plate
()
*
r
a
σ
normal radial stress from the stress analysis of the equivalent plate
()
*
a
θ
σ
normal circumferential stress from the stress analysis of the equivalent plate
()
*
r
d
σ
normal radial stress computed from the elasticity matrix for the square hole pattern in the
diagonal direction
()
*
d
θ
σ
normal circumferential stress computed from the elasticity matrix for the square hole pattern
in the diagonal direction
()
*
r
p
σ
normal radial stress computed from the elasticity matrix for the square hole pattern in the
pitch direction
()
*
p
θ
σ
normal circumferential stress computed from the elasticity matrix for the square hole pattern
in the pitch direction
*
r
σ
normal radial stress at the perforated plate to solid ring interface calculated from the stress
analysis
*
θ
σ
normal circumferential stress at the perforated plate to solid ring interface calculated from the
stress analysis
*
rz
τ
shear stress at the perforated plate to solid ring interface calculated from the stress analysis
t
t nominal tube wall thickness
m
T mean temperature averaged through the thickness of the plate
s
T temperature at the surface of the plate
L
U largest center-to-center distance between adjacent tube rows, but not to exceed 4p
θ
orientation for the stress calculation in the referenced to the local Cartesian coordinate
system (see Figures 5.E.4 and 5.E.5)
标准分享网 www.bzfxw.com 免费下载

2010 SECTION VIII, DIVISION 2
5-107
5.E.9 Tables
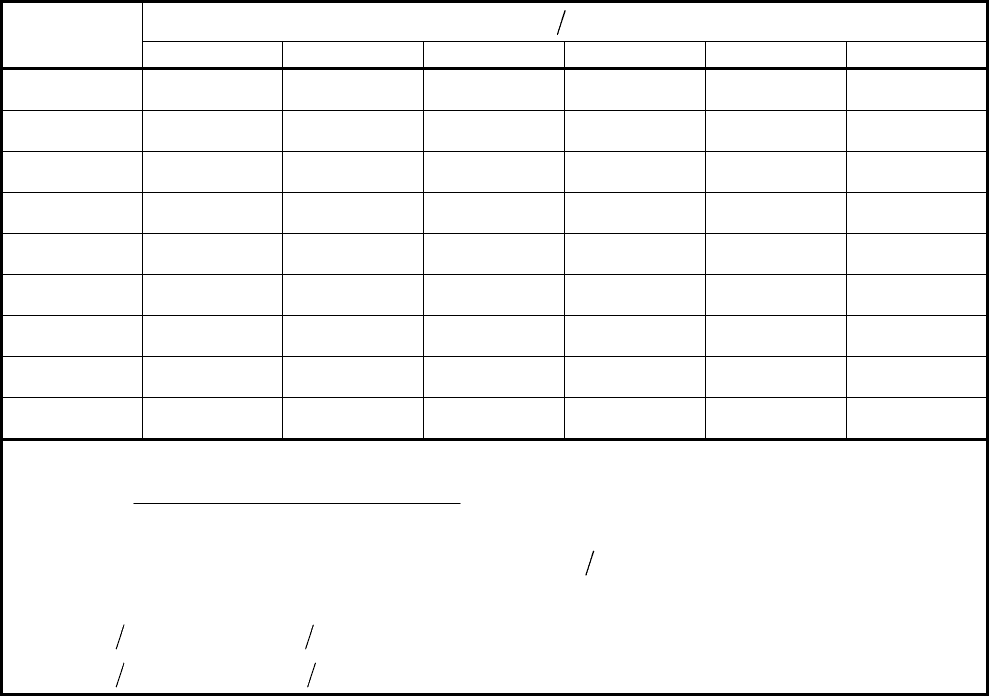
Table 5.E.1 – Values of
E
∗
For Perforated Tubesheets With An Equilateral Triangular Pattern
Coefficients
hp
0.1 0.25 0.5 2.0
0
A
1.496410E-05 6.352630E-06 -1.333850E-03 7.285910E-04
1
A
6.620641E+01 1.151132E+03 -2.556960E+00 -7.005325E-01
2
A
4.301938E+00 1.275654E+01 6.777985E-01 1.151695E-01
3
A
-3.393999E+01 -1.643137E+03 3.994838E+00 2.440697E+00
4
A
7.672133E+01 1.202564E+03 1.550319E-01 4.439786E+00
5
A
1.497235E+00 1.257743E+03 -4.396305E+00 -5.840415E+00
6
A
-3.012851E+01 -9.998214E+02 -2.431697E+00 -8.312674E+00
7
A
1.613346E+01 -5.511521E+02 2.177133E+00 4.123150E+00
8
A
0.0 0.0 1.819010E+00 4.780299E+00
Note:
1.
234
02 4 6 8
234
1357
1
AA A A A
EE
AA A A
μμ μ μ
μμ μ μ
∗∗ ∗ ∗
∗
∗∗ ∗ ∗
⎡⎤
++ + +
=
⎢⎥
++ + +
⎣⎦
2. These coefficients are valid for 01.0
μ
∗
≤≤, data for 2.0
μ
∗
= is provided for information only.
3. If
0.1hp< , then use 0.1hp= .
4. If
2.0hp> , then use 2.0hp= .
2010 SECTION VIII, DIVISION 2
5-108
Table 5.E.2 – Values of
*
ν
For Perforated Tubesheets With An Equilateral Triangular Pattern
Coefficients
hp
0.1 0.15 0.25 0.5 1.0 2.0
0
B
1.722338E-02 -7.248304E-01 3.824487E+02 2.860400E+00 1.483591E+00 9.823512E-01
1
B
-3.203150E+01 -3.180156E+01 1.539472E+04 5.679314E+01 1.504975E+01 9.655558E-01
2
B
1.765880E+00 1.599207E+01 2.264094E+03 4.717135E-01 -1.976814E+00 -2.811381E+00
3
B
2.321240E+02 2.859222E+02 -4.175812E+04 -2.166572E+02 -6.193608E+01 4.633821E+00
4
B
-2.593198E+01 -9.517465E+01 -1.461539E+04 -3.787845E+01 -7.869233E+00 5.917858E+00
5
B
-2.171148E+02 -1.388896E+02 7.152697E+04 3.226507E+02 1.094078E+02 7.097756E+00
6
B
9.357422E+01 2.547347E+02 2.981678E+04 8.368412E+01 2.733155E+01 0.0
7
B
1.107294E+02 7.201617E+01 1.387188E+04 0.0 0.0 0.0
8
B
-4.095396E+01 -1.182527E+02 0.0 0.0 0.0 0.0
Note:
1.
234
02 4 6 8
234
13 5 7
1
BB B B B
BB B B
μ
μμμ
ν
μ
μμμ
∗∗ ∗ ∗
∗
∗∗ ∗ ∗
++ + +
=
++ + +
2. These coefficients are valid for 01.0
μ
∗
≤≤ except if 0.1hp
=
, then 0.184 1.0
μ
∗
≤≤, data for
2.0
μ
∗
= is provided for information only.
3. If
0.1hp< , then use 0.1hp= .
4. If
2.0hp> , then use 2.0hp= .
标准分享网 www.bzfxw.com 免费下载
