Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V

Подождите немного. Документ загружается.

558 Chapter 9

eigenfunctions, the series was shown to have the “real” form

f(x) =

A(0)

√

∞



n=1



A(n) cos



nπx



√

B(n) sin



nπx



√



With some manipulation, using the Euler formulas

cos(u) =

−iu

and

sin(u) =

−e

−iu

the real form of the preceding series can be modiﬁed and rewritten in what is called the

“complex” form of the Fourier series and it reads

f(x) =

∞



n=−∞

F(n) e

inπx

The terms F(n) are the “complex” Fourier coefﬁcients, and, from the Sturm-Liouville theorem,

the preceding series converges to the average of the left- and right-hand limits of the function

at any point within the open interval I.

The preceding form of the Fourier series suggests that f(x) is being expanded in terms of a

generalized Sturm-Liouville series whose orthonormalized eigenfunctions φ

(x) are given as

(x) =

√

inπ,x

√

for n =−3, −2, −1, 0, 1, 2, 3 ....

Because these eigenfunctions arise from the solution of a regular Sturm-Liouville problem

with periodic boundary conditions, we could have referred to them as “regular”

eigenfunctions. The statement of orthonormality for these complex eigenfunctions is given as

the “complex” inner product (the product of the function times its complex conjugate), with

respect to the weight function w(x) = 1, over the interval I. In terms of the Kronecker delta

function (see references for a more complete description of the mathematical chararcteristics of

the Kronecker delta function and the Dirac delta function used following), this reads



−L

inπx

−

imπx

dx = δ(n, m)

Inﬁnite and Semi-inﬁnite Spatial Domains 559

Thus, to evaluate the Fourier coefﬁcients as we did in Chapter 2, we take the complex inner

product of both sides of the preceding series with respect to the orthonormalized

eigenfunctions and the weight function w(x) = 1, and we get



−L

f(x) e

−

imπx

dx =

∞



n=−∞

F(n)

⎛

⎜

⎝



−L

inπx

−

imπx

⎞

⎟

⎠

Taking advantage of the earlier statement of orthonormality, this series reduces to



−L

f(x) e

−

imπx

dx =

∞



n=−∞

F(n)δ(n, m)

Due to the mathematical nature of the Kronecker delta function, only the m = n term survives

the sum on the right, and from this sum, we evaluate the complex Fourier coefﬁcients F(n)

to be

F(n) =



−L

f(x) e

−

inπx

Many textbooks cover the Fourier integral theorem, which deals with the transition from

Fourier series to Fourier integrals. The Fourier integral theorem is a generalization of the

Fourier series expansion as we go from ﬁnite domains to inﬁnite domains. The theorem states

the following (see related references): let f(x) be piecewise smooth and absolutely integrable

on the real line. Then f(x) can be represented by the following Fourier integral

f(x) =

∞



−∞

F(ω)e

iωx

dω

This integral converges to the average of the left- and right-hand limits of f(x) at any point

over the inﬁnite domain.

Comparing the preceding Fourier integral expansion of f(x) over inﬁnite domains with the

earlier Fourier series expansion of f(x) over ﬁnite domains, we see a remarkable parallel.

Similar to Fourier series, the terms F(ω) are the singular Fourier coefﬁcients of f(x).

By convention, these terms F(ω) are more commonly called the “Fourier transform” of f(x).

We now recognize the transition from Fourier series to Fourier integrals: As the domain goes

from ﬁnite to inﬁnite, the series summation is replaced by an integration, and the discrete

summing index over real integers is replaced by an integration over a continuous variable.

560 Chapter 9

It can be shown (see references) that the corresponding Fourier transform F(ω) (singular

Fourier coefﬁcient) can be found from the following integral:

F(ω) =

∞



−∞

f(x) e

−iωx

The functions f(x) and F(ω) constitute what is called a “Fourier transform pair.”

In the development of the transition of the concepts of Sturm-Liouville theory for regular

eigenvalue problems over ﬁnite intervals to that over inﬁnite intervals, we can view the Fourier

integral for f(x) as an expansion in terms of the “singular” eigenfunctions given here:

√

iωx

√

for −∞ <ω<∞.

The extension of these concepts would be complete except for the lack of an equivalent

statement of orthonormality. If we substitute f(x) in the integral for F(ω) and formally

interchange the order of integration, we get

F(ω) =

∞



−∞

∞



−∞

F(β) e

iβx

−iωx

2π

dx dβ

We now compare this integral with the formal integral deﬁnition of the Dirac delta function

δ(β −ω) shown here:

∞



−∞

F(β) δ(β −ω) dβ = F(ω)

From a comparison of the two preceding integrals, we can identify the previous operation as

the integral representation of the Dirac delta function in terms of the singular

eigenfunctions—that is,

∞



−∞

iβx

−iωx

2π

dx = δ(β −ω) (9.1)

Parallel with the statement of orthonormality for regular eigenfunctions, the preceding

equation can be considered to be the equivalent to the statement of orthonormality for the

“singular” eigenfunctions given earlier. Similar to the Kronecker delta function, the preceding

Dirac delta function has the value zero when β = ω and has the value ∞ for β = ω. Because of

the sharp character of this function, it is often called the “impulse” function. Thus, the parallel

between singular eigenfunctions over inﬁnite domains has been shown to be analogous to that

of regular eigenfunctions over ﬁnite domains.

Inﬁnite and Semi-inﬁnite Spatial Domains 561

9.3 Fourier Sine and Cosine Integrals

If f(x) is an even or odd function of x, then the preceding Fourier integral reduces to a slightly

different form. We now demonstrate this. Recall the Euler formula:

= cos(u) +i sin(u)

If we use the Euler formula in the expansion of the Fourier integral, we get

f(x) =

∞



−∞

F(ω)

(

cos(ωx) +i sin(ωx)

)

2π

dω

Similarly, if we use the Euler formula in the expansion of the Fourier transform, we get

F(ω) =

∞



−∞

f(x)

(

cos(ωx) −i sin(ωx)

)

Combining the two expressions and formally interchanging the order of integration yields

F(ω) =

∞



−∞

∞



−∞

F(β)

(

cos(βx) +i sin(βx)

)(

cos(ωx) −i sin(ωx)

)

2π

dx dβ

To evaluate this integral, we must ﬁrst recall the properties of integration of even and odd

functions of x over symmetric intervals as shown:

∞



−∞

even(f(x)) dx = 2

⎛

⎝

∞



even(f(x)) dx

⎞

⎠

and

∞



−∞

odd(f(x)) dx = 0

The ﬁrst states that an integration of an even function of x over a symmetric interval is equal to

twice the integral over half that interval. The second states that an integration of an odd

function of x over a symmetric interval is equal to zero.

We focus on evaluation of the earlier integral F(ω) for the two cases where f(x) is an even or

odd function of x.

If f(x) is an even function of x, then, from the preceding Fourier transform, F(β) is an even

function of β, and the term F(β) cos(βx) cos(ωx) is the only term given that is even with

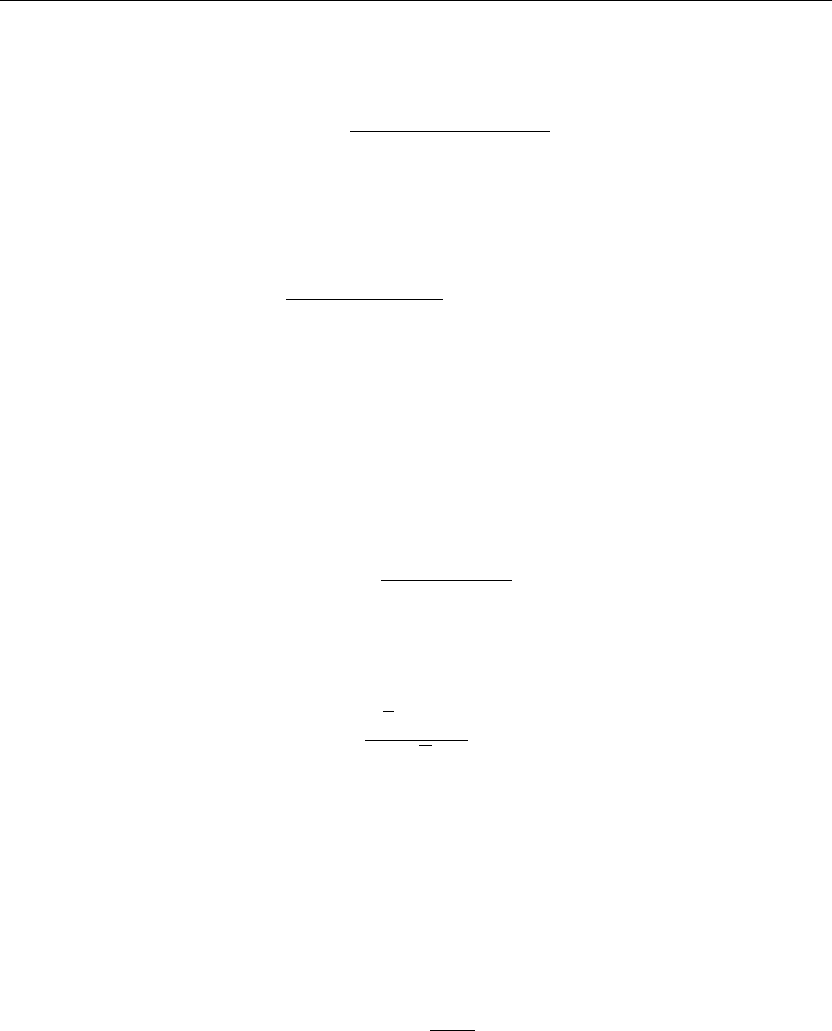
562 Chapter 9

respect to both x and β; all other terms are odd. Thus, the resulting Fourier transform reduces

to the following expression:

F(ω) =

∞



∞



2F(β) cos(βx) cos(ωx)

dx dβ

If we compare this integral with the formal integral deﬁnition of the preceding Dirac delta

function, we can write the statement of orthonormality for the “singular” eigenfunctions as

∞



2 cos(βx) cos(ωx)

dx = δ(β −ω)

If we deﬁne the Fourier cosine transform C(ω) as the integral

C(ω) =

∞



f(x) cos(ωx) dx

then we can express the even function f(x) as the Fourier cosine integral

f(x) =

∞



2C(ω) cos(ωx)

dω

We view this equation to be the generalized expansion of an even function of x in terms of the

singular, orthonormalized eigenfunctions

√

2 cos(ωx)

√

for 0 <ω<∞. The preceding is the analog of the Fourier cosine series expansion of an even

periodic function of x. The evaluation of the Fourier coefﬁcients C(ω) can be viewed as the

result that comes about from taking the inner product of both sides of the preceding with

respect to the singular eigenfunctions and taking advantage of the statement of orthonormality.

The integral and its corresponding transform C(ω) constitute a “Fourier cosine transform” pair.

We note here that for even functions of x, the relation between the cosine transform C(ω) and

the regular Fourier transform is

C(ω) =

F(ω)

where F(ω) is the Fourier transform of the even extension of f(x).

Similarly, for odd functions, we do the following. If f(x) is an odd function of x, then, from the

preceding Fourier transform, the term F(β) is an odd function of β, and F(β) sin(βx) sin(ωx) is

Inﬁnite and Semi-inﬁnite Spatial Domains 563

the only term given that is even with respect to both β and x; all other terms are odd. Thus, the

resulting integral reduces to

F(ω) =

∞



∞



2F(β) sin(βx) sin(ωx)

dx dβ

If we compare this integral with the formal integral deﬁnition of the Dirac delta function given

earlier, we can write the statement of orthonormality for the “singular” eigenfunctions as

∞



2 sin(βx) sin(ωx)

dx = δ(β −ω)

If we deﬁne the Fourier sine integral S(ω) as

S(ω) =

∞



f(x) sin(ωx) dx

then we can express the odd function f(x) as a Fourier sine integral

f(x) =

∞



2S(ω) sin(ωx)

dω

We view this equation to be the generalized expansion of an odd function of x in terms of the

singular orthonormalized eigenfunctions

√

2 sin(ωx)

√

for 0 <ω<∞. The preceding is the analog of the Fourier sine series expansion of an odd

periodic function of x. The evaluation of the Fourier coefﬁcients S(ω) can be viewed as the

result that comes about from taking the inner product of both sides of the preceding with

respect to the singular eigenfunctions and taking advantage of the statement of orthonormality.

The preceding integral and its corresponding transform S(ω) constitute a “Fourier sine

transform” pair. We note here that for odd functions of x, the relation between the sine

transform and the regular transform is

S(ω) =

iF(ω)

where F(ω) is the Fourier transform of the odd extension of f(x).

564 Chapter 9

9.4 Nonhomogeneous Diffusion Equation over Inﬁnite

Domains

We now use the Fourier integral method (singular eigenfunction expansion) to formulate the

solutions to diffusion partial differential equations over inﬁnite or semi-inﬁnite intervals.

We ﬁrst consider the nonhomogeneous diffusion partial differential equation over the inﬁnite

interval I ={x |−∞<x<∞}. The equation reads

∂

∂t

u(x, t) = k



∂

∂x

u(x, t)



+h(x, t)

with the initial condition

u(x, 0) = f(x)

Here, f(x) denotes the initial concentration or temperature distribution, and h(x, t) denotes the

presence of any internal source terms within the system. The boundary conditions are such that

u(x, t) is piecewise smooth and absolutely integrable over the inﬁnite interval

I ={x |−∞<x<∞}.

As we did in Chapter 8, we ﬁrst consider the homogeneous version of the given equation.

Using the method of separation of variables as in Chapter 3, we arrive at two ordinary

differential equations: one in t and one in x. The x-dependent differential equation is of the

Euler type, and it reads

X(x)+λX(x) = 0

A set of basis vectors for this differential equation for λ>0 is, from Chapter 1,

x1(x) = e

√

λx

and

x2(x) = e

−i

√

λx

If we set λ = ω

and we require that our x-dependent portion of the solution be bounded as x

goes to plus or minus inﬁnity, then our x-dependent orthonormalized singular eigenfunctions,

as shown in Section 9.2, can be written

√

iωx

√

for −∞ <ω<∞. From the Fourier integral theorem, these singular eigenfunctions are

“complete” with respect to piecewise smooth, absolutely integrable functions over the inﬁnite

Inﬁnite and Semi-inﬁnite Spatial Domains 565

interval I ={x |−∞<x<∞}. Thus, we can write our solution as the superposition of the

preceding singular eigenfunctions, or, equivalently, as the Fourier integral

u(x, t) =

∞



−∞

U(ω, t) e

iωx

2π

dω

From Section 9.2, U(ω, t) is the time-dependent Fourier transform of u(x, t). If we substitute

the initial condition u(x, 0) = f(x) in this solution, we get

f(x) =

∞



−∞

U(ω, 0) e

iωx

2π

dω

Obviously, U(ω, 0) is the Fourier transform of the initial condition function f(x) where we

have assumed that f(x) is piecewise smooth and absolutely integrable over the inﬁnite interval.

We now deal with the nonhomogeneous driving term in the same manner as we did in

Chapter 8. If the source term h(x, t) is piecewise smooth and absolutely integrable with respect

to x over the inﬁnite domain, then we can express its Fourier integral as

h(x, t) =

∞



−∞

H(ω, t) e

iωx

2π

dω

where H(ω, t) is the Fourier transform of h(x, t) as given here:

H(ω, t) =

∞



−∞

h(x, t) e

−iωx

We now require that all functions u(x, t), f(x), and h(x, t) be absolutely integrable with respect

to x over the inﬁnite interval. We also require that the solution u(x, t) and its ﬁrst derivatives

with respect to x vanish at both positive and negative inﬁnity. With these assumptions, we

substitute the assumed solution into the given partial differential equation for u(x, t), and this

yields

∂

∂t

⎛

⎝

∞



−∞

U(ω, t) e

iωx

2π

dω

⎞

⎠

= k

⎛

⎝

∂

∂x

⎛

⎝

∞



−∞

U(ω, t) e

iωx

2π

dω

⎞

⎠

⎞

⎠

∞



−∞

H(ω, t) e

iωx

2π

dω

Assuming the validity of the formal interchange between the differentiation and the integration

operators, the preceding equation reduces to

∞



−∞



∂

∂t

U(ω, t) +kω

U(ω, t)



iωx

dω =

∞



−∞

H(ω, t) e

iωx

dω

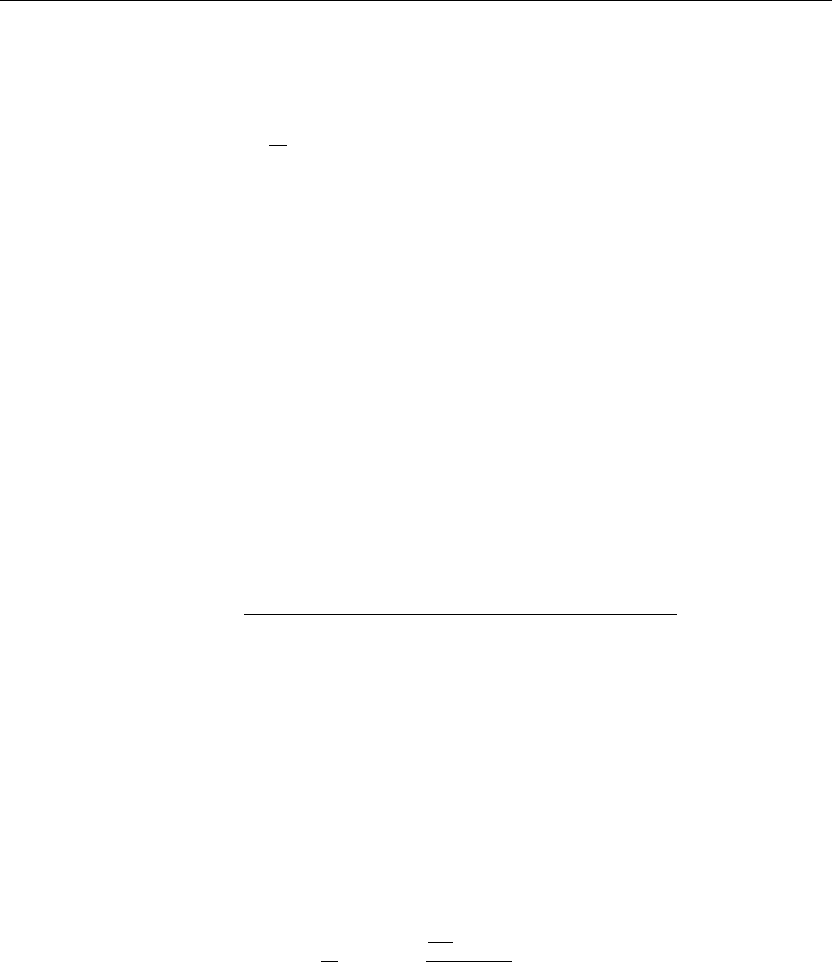
566 Chapter 9

In order for this equation to hold, due to the linear independence of the singular eigenfunctions,

we set the integrands equal to each other, and we arrive at the following ﬁrst-order

nonhomogeneous differential equation in time t:

∂

∂t

U(ω, t) +kω

U(ω, t) = H(ω, t)

with the initial condition

U(ω, 0) =

∞



−∞

f(x) e

−iωx

This is a ﬁrst-order initial value problem and, from Chapter 1, Section 1.3, the solution to this

differential equation is

U(ω, t) = U(ω, 0) e

−kω



H(ω, τ) e

−kω

(t−τ)

dτ

Thus, from knowledge of the Fourier transform, the general form of the solution to our

nonhomogeneous diffusion partial differential equation can be written as the Fourier integral

u(x, t) =

∞



−∞



U(ω, 0) e

−kω



H(ω, τ) e

−kω

(t−τ)

dτ



iωx

2π

dω

We demonstrate the preceding concepts with the solution of an illustrative problem.

DEMONSTRATION: Consider a long, thin cylinder over the inﬁnite interval I ={x |−∞<

x<∞} containing a ﬂuid that has an initial salt concentration density f(x) given as follows.

We seek the spatial and time dependence of the density u(x, t) of the salt as it diffuses into the

ﬂuid. There is no source term in the system, and the diffusivity is k = 1/4.

SOLUTION: Because there is no source term, the system homogeneous diffusion partial

differential equation reads

∂

∂t

u(x, t) =

∂

∂x

u(x, t)

The boundary condition requires that the solution be absolutely integrable over the inﬁnite

spatial domain—that is,

∞



−∞

|u(x, t)|dx<∞

Inﬁnite and Semi-inﬁnite Spatial Domains 567

For this particular problem, the initial condition is u(x, 0) = f(x) where

f(x) = e

−x

From the method of separation of variables, the spatial-dependent differential equation yields

the singular orthonormal eigenfunctions:

√

iωx

√

The Fourier integral form of the solution, in terms of these spatial eigenfunctions, is

u(x, t) =

∞



−∞

U(ω, t) e

iωx

2π

dω

The Fourier transform of the initial condition term for the given f(x) is

U(ω, 0) =

∞



−∞

−x

−iωx

Evaluation of this integral yields

U(ω, 0) = e

−

√

From the method of separation of variables, the resultant time-dependent Fourier transform

term satisﬁes the ﬁrst-order differential equation

∂

∂t

U(ω, t) +

U(ω, t)

= 0

The solution of this differential equation, which satisﬁes the preceding initial condition, is

U(ω, t) = e

−

√

π e

−

Thus, we can write the solution to the problem as the Fourier integral

u(x, t) =

∞



−∞

−

iωx

√

dω

Evaluation of this integral yields the ﬁnal form of the equation for the salt density

u(x, t) =

−

1+t

√

1 +t

The details for the development of this solution along with the graphics are given later in one

of the Maple worksheet example problems.