Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V
Подождите немного. Документ загружается.


548 Chapter 8
Nonhomogeneous Heat Equations
We consider the temperature distribution along a thin rod whose lateral surface is insulated
over the finite interval I ={x |0 <x<1}. We allow for an external heat source h(x, t) to act on
the system. The nonhomogeneous diffusion partial differential equation in rectangular
coordinates reads
∂
∂t
u(x, t) = k
∂
2
∂x
2
u(x, t)
+h(x, t)
with the initial condition
u(x, 0) = f(x)
In Exercises 8.1 to 8.16, we seek solutions to the preceding problem for various given initial
conditions and boundary conditions. Resolve the solution into two parts: the variable and the
linear, as was done in Section 8.4. Determine the eigenfunctions and eigenvalues for the
corresponding homogeneous problem, and write the solution as a sum of the partitioned
solutions. Generate the animated solution for u(x, t), and plot the animated sequence for
0 <t<5. Note whether the solution satisfies the given boundary and initial conditions.
8.1. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 is up against a
sinusoidal temperature reservoir, there is no external heat source, k =1/10:
Boundary conditions:
u(0,t)= 0 and u(1,t)= sin(t)
Initial condition:
f(x) = x(1 −x)
8.2. Boundary x = 1 is held at fixed temperature 0, boundary x = 0 is up against a
sinusoidal temperature reservoir, there is no external heat source, k =1/10:
Boundary conditions:
u(0,t)= cos(t) and u(1,t)= 0
Initial condition:
f(x) = x(1 −x)
8.3. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 has an external heat flux
input, there is no external heat source, k = 1/10:
Boundary conditions:
u(0,t)= 0 and
u
x
(1,t)= te
−t

Nonhomogeneous Partial Differential Equations 549
Initial condition:
f(x) = x
1 −
x
2
8.4. Boundary x = 0 has an external heat flux input, x = 1 is held at fixed temperature 2,
there is no external heat source, k = 1/10:
Boundary conditions:
u
x
(0,t)=−cos(t) and u(1,t)= 2
Initial condition:
f(x) = 1 −x
2
8.5. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 is losing heat by
convection into a time-varying surrounding temperature medium, there is no external
heat source, k = 1/10:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)+u(1,t)= sin(t)
Initial condition:
f(x) = x
1 −
2x
3
8.6. Boundary x = 0 is losing heat by convection into a time-varying surrounding
temperature medium, boundary x = 1 is held at fixed temperature 1, there is no
external heat source, k = 1/10:
Boundary conditions:
u
x
(0,t)−u(0,t)= cos(t) and u(1,t)= 1
Initial condition:
f(x) =
1
3
+
x
3
−
2x
2
3
8.7. Boundary x = 0 has an external heat flux input, boundary x = 1 is losing heat by
convection into a time-varying surrounding temperature medium, there is no external
heat source, k = 1/10:
Boundary conditions:
u
x
(0,t)= sin(t) and u
x
(1,t)+u(1,t)= sin(t)

550 Chapter 8
Initial condition:
f(x) = 1 −
x
2
3
8.8. Boundary x = 0 is losing heat by convection into a time-varying surrounding
temperature medium, boundary x = 1 has an input heat flux, there is no external heat
source, k = 1/10:
Boundary conditions:
u
x
(0,t)−u(0,t)= te
−t
and u
x
(1,t)= 1
Initial condition:
f(x) = 1 −
(
x −1
)
2
3
8.9. Boundaries x = 0 and x = 1 are held at the fixed temperature of 0, there is an external
heat source term h(x, t), k = 1/10:
Boundary conditions:
u(0,t)= 0 and u(1,t)= 0
Initial condition and heat source:
f(x) = x(1 −x) and h(x, t) = sin(t)
8.10. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 is insulated, there is an
external heat source term h(x, t), k = 1/10:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)= 0
Initial condition and heat source:
f(x) = 0 and h(x, t) = x
1 −
x
2
sin(t)
8.11. Boundary x = 0 is insulated, boundary x = 1 is held at fixed temperature 0, there is an
external heat source term h(x, t), k = 1/10:
Boundary conditions:
u
x
(0,t)= 0 and u(1,t)= 0
Initial condition and heat source:
f(x) = 0 and h(x, t) =
1 −x
2
cos(t)

Nonhomogeneous Partial Differential Equations 551
8.12. Boundaries x = 0 and x = 1 are both insulated, there is an external heat source term
h(x, t), k = 1/10:
Boundary conditions:
u
x
(0,t)= 0 and u
x
(1,t)= 0
Initial condition and heat source:
f(x) = 0 and h(x, t) = te
−t
x
2
1 −
2x
3
8.13. Boundary x = 0 is held at fixed temperature 10, boundary x = 1 is held at fixed
temperature 5, there is an external heat source term h(x, t), k = 1/10:
Boundary conditions:
u(0,t)= 10 and u(1,t)= 5
Initial condition and heat source:
f(x) = 0 and h(x, t) = x(1 −x) sin(t)
8.14. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 has an external heat flux
input, there is an external heat source term h(x, t), k = 1/10:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)= sin(t)
Initial condition and heat source:
f(x) = 0 and h(x, t) = x
1 −
x
2
cos(t)
8.15. Boundary x = 0 is held at fixed temperature 0, boundary x = 1 is losing heat by
convection into a time-varying surrounding medium, there is an external heat source
term h(x, t), k = 1/10:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)+u(1,t)= sin(t)
Initial condition and heat source:
f(x) = 0 and h(x, t) = x
1 −
2x
3
cos(t)
8.16. Boundary x = 0 is held at fixed temperature 2, boundary x = 1 is losing heat by
convection into a time-varying surrounding medium, there is an external heat source
term h(x, t), k = 1/10:

552 Chapter 8
Boundary conditions:
u(0,t)= 2 and u
x
(1,t)+u(1,t)= sin(t)
Initial condition and heat source:
f(x) = x and h(x, t) = x
1 −
2x
3
cos(t)
Nonhomogeneous Wave Equations
We consider transverse wave propagation over taut strings and longitudinal wave propagation
in rigid bars over the finite, one-dimensional interval I ={x |0 <x<1}. We allow for an
external force to act on the system. The nonhomogeneous wave partial differential equation in
rectangular coordinates reads
∂
2
∂t
2
u(x, t) = c
2
∂
2
∂x
2
u(x, t)
+h(x, t)
with the two initial conditions
u(x, 0) = f(x) and u
t
(x, 0) = g(x)
In Exercises 8.17 through 8.23, we seek solutions to the preceding problem for various given
initial and boundary conditions. Resolve the solution into two parts: the variable and the linear
as was done in Section 8.7. Determine the eigenfunctions and eigenvalues for the
corresponding homogeneous problem, and write the solution as a sum of the partitioned
solutions. Generate the animated solution for u(x, t), and plot the animated sequence for
0 <t<5. Note whether the solution satisfies the given boundary and initial conditions.
8.17. Consider transverse motion in a taut string, boundary x = 0 is held secure, boundary
x = 1 is attached to a vertical oscillator, there is no external force acting, c = 1/2:
Boundary conditions:
u(0,t)= 0 and u(1,t)= sin(t)
Initial conditions:
f(x) = x(1 −x) and g(x) = 0
8.18. Consider transverse motion in a taut string, boundary x = 0 is attached to a vertical
oscillator, boundary x = 1 is held fixed, there is no external force acting, c = 1/2:
Boundary conditions:
u(0,t)= cos(t) and u(1,t)= 0

Nonhomogeneous Partial Differential Equations 553
Initial conditions:
f(x) = 0 and g(x) = x(1 −x)
8.19. Consider longitudinal waves in a rigid bar whose boundary x = 0 is held fixed,
boundary x = 1 has an applied compression (Young’s modulus E = 1), there is no
external force, c = 1/2:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)= 2
Initial conditions:
f(x) = x
1 −
x
2
and g(x) = 0
8.20. Consider longitudinal waves in a rigid bar whose boundary x = 0 has an applied
compression, boundary x = 1 is held fixed, there is no external force, c = 1/2:
Boundary conditions:
u
x
(0,t)= cos(t) and u(1,t)= 0
Initial conditions:
f(x) = 0 and g(x) = 1 −x
2
8.21. Consider transverse waves in a taut string whose boundary x = 0 is held fixed,
boundary x = 1 is attached to an elastic hinge with a time-varying support, there is no
external force, c = 1/2:
Boundary conditions:
u(0,t)= 0 and u
x
(1,t)+u(1,t)= sin(t)
Initial conditions:
f(x) = x
1 −
2x
3
and g(x) = 0
8.22. Consider transverse waves in a taut string whose boundary x = 0 is attached to an
elastic hinge with a time-varying support, boundary x = 1 is held fixed, there is no
external force, c = 1/2:
Boundary conditions:
u
x
(0,t)−u(0,t)= cos(t) and u(1,t)= 1

554 Chapter 8
Initial conditions:
f(x) = 0 and g(x) =
1
3
+
x
3
−
2x
2
3
8.23. Consider longitudinal waves in a rigid bar whose boundary x = 0 has a compressive
force, boundary x = 1 is attached to an elastic hinge with a time-varying support,
there is no external force, c = 1/2:
Boundary conditions:
u
x
(0,t)= cos(t) and u
x
(1,t)+u(1,t)= sin(t)
Initial conditions:
f(x) = 1 −
x
2
3
and g(x) = 0
8.24. Consider longitudinal waves in a rigid bar whose boundary x = 0 is attached to an
elastic hinge with a time-varying support, boundary x = 1 has a compressive force,
there is no external force, c = 1/2:
Boundary conditions:
u
x
(0,t)−u(0,t)= te
−t
and u
x
(1,t)= 1
Initial conditions:
f(x) = 0 and g(x) = 1 −
(
x −1
)
2
3
Natural Angular Frequency and Resonance
In Chapter 4, we investigated homogeneous problems for transverse wave propagation on a
taut string. Earlier in this chapter, we considered the corresponding nonhomogeneous problem
for transverse wave propagation. From our analysis for the case of no damping in the system,
we arrived at a natural angular frequency of oscillation of the wave for the nth mode:
ω
n
= c
λ
n
Here, λ
n
is the allowed eigenvalue and c is the speed of propagation of the wave with
dimensions of distance per time. For a taut string of linear density ρ under tension T , c is
given as
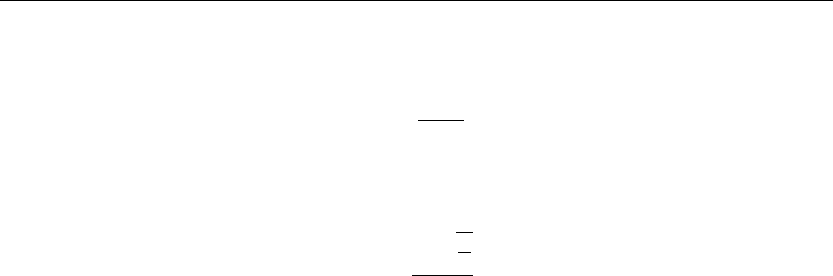
c =
T
ρ
Nonhomogeneous Partial Differential Equations 555
For a string of length l, which is secure at both ends, the allowed eigenvalues for the nth mode
are given as
λ
n
=
n
2
π
2
l
2
for n = 1, 2, 3,....Thus, the nth-mode natural angular frequency of oscillation for the
transverse wave on a taut string is given as
ω
n
=
nπ
T
ρ
l
In the following exercises, we consider the nonhomogeneous problem for transverse wave
propagation on taut strings. We consider source functions whose driving frequency may or may
not coincide with the natural angular frequency of the string. In the case where the two are
identical, we have what is called “resonance” as demonstrated in Example 8.7.4.
For Exercises 8.25 through 8.30, we consider a taut string of length l = π, which is secure at
both ends. The initial conditions are given as follows along with the boundary conditions and
the source function.
Boundary conditions:
u(0,t)= 0 and u(π, t) = 0
Source function:
h(x, t) = x(π −x) sin(ω t)
Initial conditions:
f(x) = x(π −x) and g(x) = 0 (8.183)
8.25. For the given boundary and initial conditions, let the string tension and linear density
be such that c = 1. Evaluate the eigenvalues and the corresponding eigenfunctions and
the general solution for u(x, t) for a generalized value of ω. What should the value of ω
be in order to trigger resonance for the n = 1 (fundamental) mode of the system? For
this value of ω, generate the animated solution for u(x, t), and develop the animated
sequence for 0 <t<5.
8.26. For the string in Exercise 8.25, let the tension in the string be increased by a factor of 4,
keeping everything else the same. Evaluate the eigenvalues and the corresponding
eigenfunctions and the general solution for u(x, t) for a generalized value of ω. What
should the value of ω be in order to trigger resonance for the n = 3 mode of the system?
For this value of ω, generate the animated solution for u(x, t), and develop the animated
sequence for 0 <t<5.

556 Chapter 8
8.27. For the string in Exercise 8.25, let the linear density of the string be increased by a
factor of 4, keeping everything else the same. Evaluate the eigenvalues and the
corresponding eigenfunctions and the general solution for u(x, t) for a generalized
value of ω. What should the value of ω be in order to trigger resonance for the n = 1
mode of the system? For this value of ω, generate the animated solution for u(x, t), and
develop the animated sequence for 0 <t<5.
8.28. For the string in Exercise 8.25, let the length of the string be decreased by a factor of 3,
keeping everything else the same. Evaluate the eigenvalues and the corresponding
eigenfunctions and the general solution for u(x, t) for a generalized value of ω. What
should the value of ω be in order to trigger resonance for the n = 5 mode of the system?
For this value of ω, generate the animated solution for u(x, t), and develop the animated
sequence for 0 <t<5.
8.29. For the string in Exercise 8.25, let the tension in the string be increased by a factor of 4,
and let the length be increased by a factor of 2, keeping everything else the same.
Evaluate the eigenvalues and the corresponding eigenfunctions and the general solution
for u(x, t) for a generalized value of ω. What should the value of ω be in order to
trigger resonance for the n = 3 mode of the system? For this value of ω, generate the
animated solution for u(x, t), and develop the animated sequence for 0 <t<5.
8.30. For the string in Exercise 8.25, let the linear density of the string be decreased by a
factor of 4, and let the tension be decreased by a factor of 9, keeping everything else the
same. Evaluate the eigenvalues and the corresponding eigenfunctions and the general
solution for u(x, t) for a generalized value of ω. What should the value of ω be in order
to trigger resonance for the n = 5 mode of the system? For this value of ω, generate the
animated solution for u(x, t), and develop the animated sequence for 0 <t<5.
8.31. For the string in Exercise 8.25, let the tension in the string be increased by a factor of 4,
the density be increased by a factor of 9, and the length of the string be decreased by a
factor of 2, keeping everything else the same. Evaluate the eigenvalues and the
corresponding eigenfunctions and the general solution for u(x, t) for a generalized
value of ω. What should the value of ω be in order to trigger resonance for the n = 3
mode of the system? For this value of ω, generate the animated solution for u(x, t), and
develop the animated sequence for 0 <t<5.

CHAPTER 9
Infinite and Semi-infinite
Spatial Domains
9.1 Introduction
We now look at partial differential equations over spatial domains that are infinite or
semi-infinite. These problems definitely do not fall into the category of “regular”
Sturm-Liouville problems and are often referred to as “singular” Sturm-Liouville problems.
We treat these problems by way of an extension of the concepts of regular problems over finite
domains.
We shall see that our eigenvalues and corresponding eigenfunctions are no longer “discrete”
and indexed by integers. Instead, for infinite domains, the eigenvalues consist of a
“continuum” of real values, and we term the corresponding eigenfunctions as “singular.”
Further, the extension of the superposition principle for regular problems, where our solutions
gave us a “series” that consisted of summations over the discrete, indexed eigenvalues, now
gives way to “integrations” over the continuum of eigenvalues.
Finally, we develop an equivalent concept of orthonormality for “singular” eigenfunctions
where the Kronecker delta for regular problems gives way to the Dirac delta function for
singular problems.
9.2 Fourier Integral
We first consider recollection of the Fourier series for periodic functions over finite domains.
From Section 2.6, we saw that such a series was a special case of the generalized
Sturm-Liouville series. If the function f(x) was piecewise continuous and it satisfied the
periodic boundary conditions over the finite interval I ={x|−L<x<L}, then we could
express this function as a series of eigenfunctions that were said to be “complete” with respect
to piecewise continuous functions over the interval. In terms of the orthonormalized
© 2009, 2003, 1999 Elsevier Inc. 557
