Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


50
W.
F.
Ames
Again, the generators form an infinite-dimensional Lie algebra with
four arbitrary functions of time,
f,
g,
h,
and
k,
2.5
Related Equations
The already mentioned Bousinesq equation
(2.8),
together with five
related Bousinesq- type equations have been analyzed by Clarkson
(1986).
Korobeinikov
(1983)
and Zakharov and Korobeinikov
(1980)
have generated the Lie algebra for
a
generalized I<-dV-Burgers’ equa-
tion
ju
Ut
+
--
+
7UrnUX
-
puxx
+
Puxxx
=
0,
2t
in which
j
=
0,1,2
and
7,p,P
are real constants. Levi and Winter-
nitz
(1987)
study
a
generalized cylindrical I<-P equation
Q
[U~+~UU~+U~~~+(U/~~)+(H(~)+YG(~))~X+F(~)U~]~+
suYy
=
0
with
F,G,H
arbitrary and
a
=
fl.
Dorizzi et al.
(1986)
finds the
infinite symmetry group
for the second member
of
a
K-P hierarchy,
called the Jumbo-Miwa equation
Uxxxa/
+
3uxyux
+
3uyuxx
+
2uyt
-
3ux*
=
0.
The reader can find all
of
these groups tabulated in Rogers and Ames
(1989).
Symmetries of
a
wide class of partial differential equations is
the subject of
a
book edited by Vinogra.dov
(1989).
The equations

Symmetry in Nonlinear Mechanics
51
called the Kadomtsev-Pogutse equations are of interest in this sec-
tion. Their symmetry group is to be found on page
23
of this vol-
ume.
Related work is also discussed in
a
comprehensive book by
Konopelchenko
(1987).
3
Fluid Mechanics and Group Invariance
Similarity solutions,
as
fluid mechanicians called invariant solutions,
were developed early for the boundary layer equations (see Schlicht-
ing,
1960,
for details and history). They were developed, mostly, by
ad hoc use
of
the scaling and spiral subgroups. The first calculation
of the Lie symmetry group for the two dimensional boundary layer
equations appeared in
1961.
A
corresponding calculation in gas dy-
namics was published in
1962
(Ovsiannikov).
For
the Navier Stokes
equations Poochnachev
(1960)
was
the first to obtain its symmetry
group
for
two dimensional flows. The motivator in many
of
these
studies was
L.
V. Ovsiannikov. Since the aforementioned pioneering
studies
a
considerable literature has been generated. It is described
below in several subsections.
3.1
The Boundary Layer Equations
Extensive studies of the boundary layer equations, beginning with
(see Ovsiannokov,
1982),
have been published. Vyryshagina
(1978)
studied the three dimensional incompressible boundary layer system
21,
+
vy+w,
=
0
Ut
+
Wt
+
21%
+
vuy
+
wu,
=
-p-Ip,
+
uuyy
UW,
+
vwy
+
ww,
=
-p-lp,
-t
uwyy
P,
=
0
(34
with density
(p)
constant. The algebra contains an arbitrary func-
tion,
d(x,
z,
t),
and
so
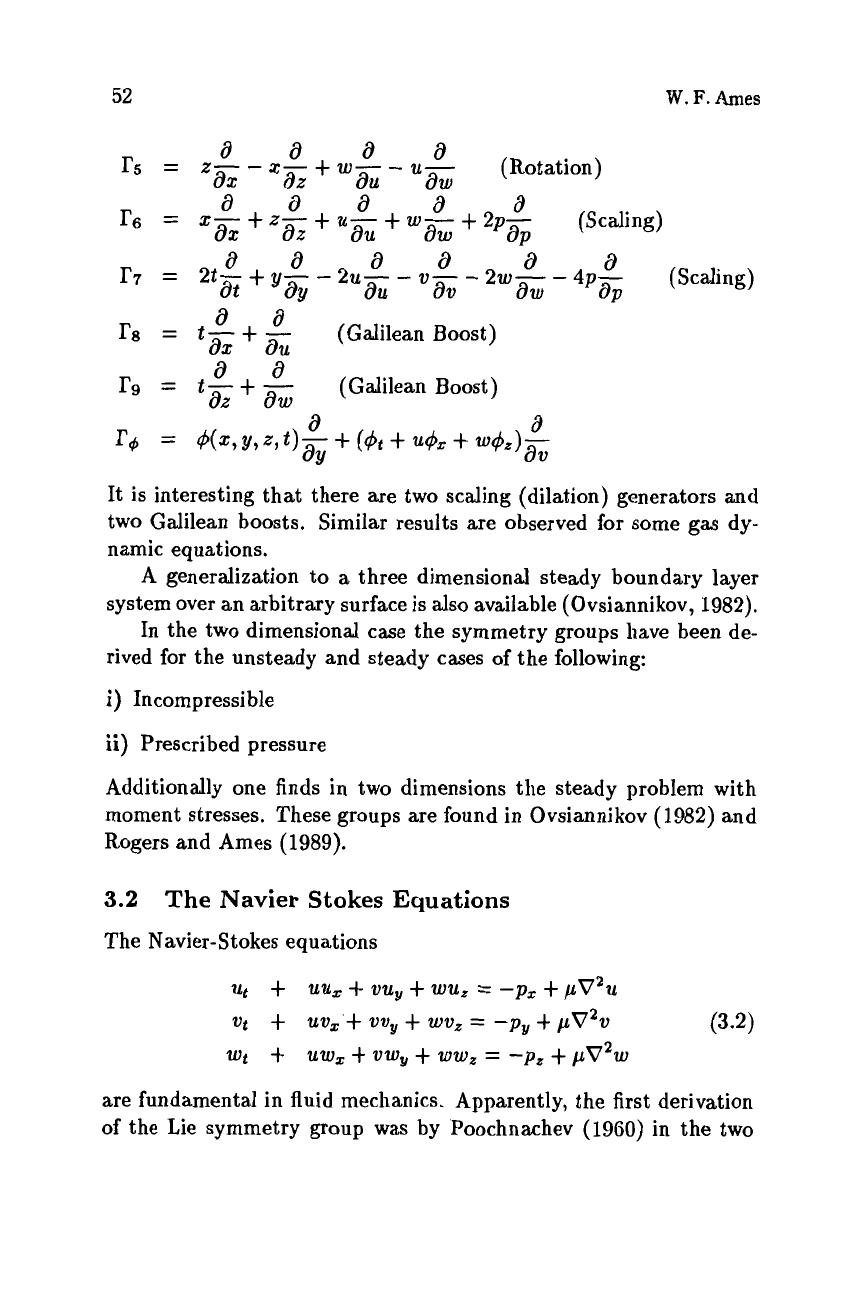
is infinite dimensional with generators
(Translations)
a
a
r3
=
-
a
a
rl=-
rz=-
at
ax
az
'
r4
=
ap
52
W.
F.
Ames
(Rotation)
a a a
a
r5
=
z--x-++--u-
ax
az
au
aw
a
a a a a
rs
=
x-
+
z-
+
u-
+
w-
+
2p-
ax
az
au
aw
ap
a a
a
d
8
d
r7
=
2t-
+
y-
-
2u-
-
v-
-
2w-
-
4p-
at
dy
au
av
aW
bP
aa
r8
=
t-
+-
ax
au
ad
r9
=
t-+-
az
aw
rd
=
4(x,
Y,
2,
t)-
+
(4t
+
(Scaling)
(
S
Cali ng)
(Galilean
Boost)
(Galilean Boost)
a a
dY
+
w4*)a,
It is interesting that there are two scaling (dilation) generators and
two Galilean boosts. Similar results are observed
for
some
gas
dy-
namic equations.
A
generalization to
a
three dimensional steady boundary layer
system over an arbitrary surface is also available (Ovsiannikov,
1982).
In the two dimensional case the symmetry groups have been de-
rived
for
the unsteady and steady cases of the following:
i)
Incompressible
ii)
Prescribed pressure
Additionally one finds in two dimensions the steady problem with
moment stresses. These groups are found in Ovsiannikov
(1982)
and
Rogers and Ames
(1989).
3.2
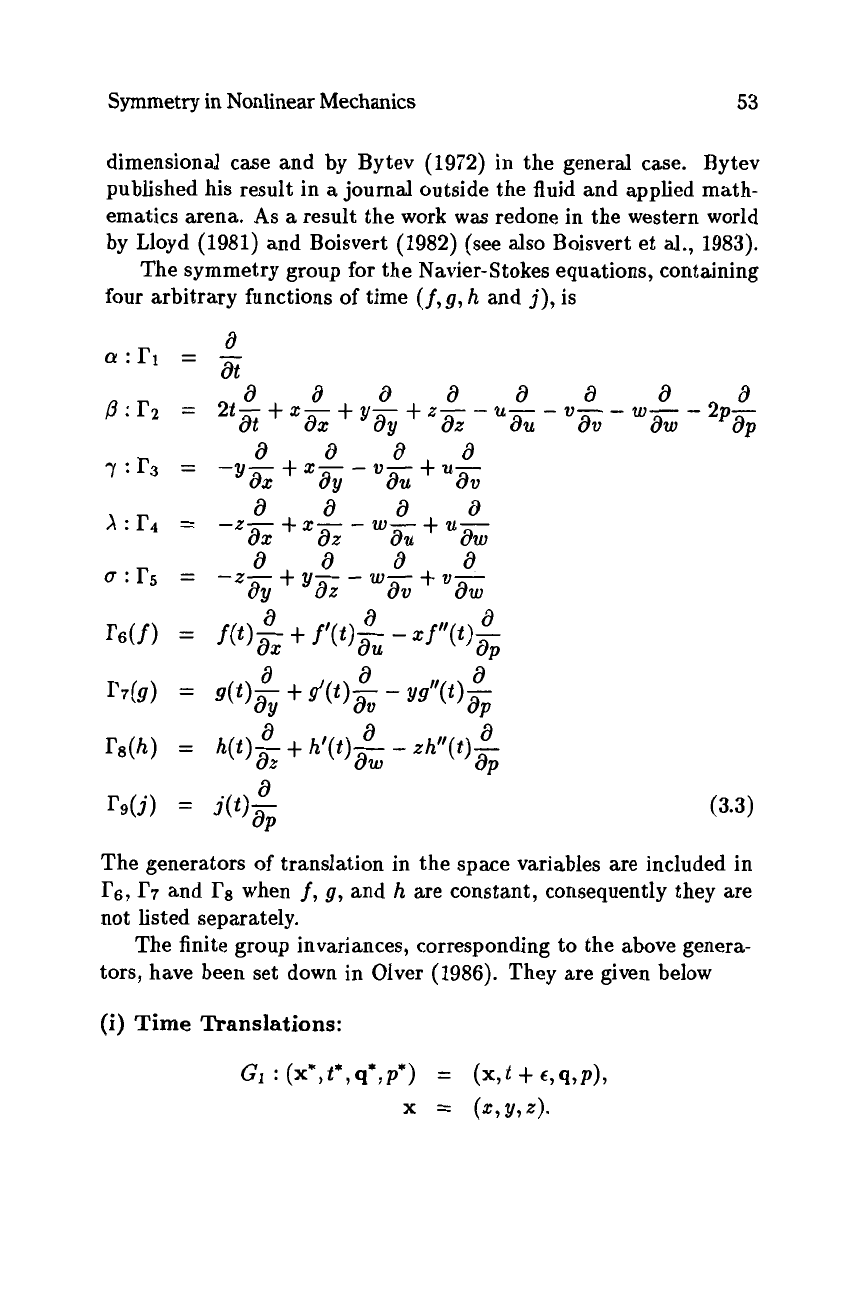
The Navier Stokes Equations
The Navier-Stokes equations
ut
+
uu,
+
vuy
+
wu,
=
-p,
+
pv2u
uw,
+
vwy
+
ww,
=
-p,
+
pv2w
vt
+
UV,
+
vvy
+
wvu,
=
-py
+
pv2v
(3.2)
Wt
+
are fundamental in fluid mechanics. Apparently, the first derivation
of
the Lie symmetry group was by Poochnachev
(1960)
in the two
Symmetry
in Nonlinear Mechanics
53
dimensional case and by Bytev
(1972)
in the general case. Bytev
published his result in
a
journal outside the fluid and applied math-
ematics arena.
As
a
result the work was redone in the western world
by Lloyd
(1981)
and Boisvert
(1982)
(see also Boisvert et
al.,
1983).
The symmetry group for the Navier-Stokes equations, containing
four arbitrary functions
of
time
(f,g,
h
and
j),
is
a
a:rl
=
-
at
a
a
a
a
a
a
a a
p:
rz
=
2t-
+x-
+y-
+
z-
-
21-
-v-
-
w-
-2p-
at
ax
ay
az
au
av
aw
ap
a
a
a
a
y:r3
=
-y-++--v-++-
ax
ay
au
av
a
a
a
a
x:r4
=
-~-++--~-++--
ax
az
au
aw
a
a a a
u:r5
=
-z~+yz-wav+v-
dW
The generators
of
translation in the space variables are included in
r6,
I’7
and
r8
when
f,
g,
and
h
are constant, consequently they are
not listed separately.
The finite group invariances, corresponding to the above genera-
tors, have been set down in Olver
(1986).
They are given below
(i)
Time Translations:

54
W.
F.
Ames
(ii) Scaling Transformations:
Here,
X
=
e'
is
a
multiplicative group parameter.
(iii) Transformation to an Arbitrary Moving Coordinate
System:
1
2
G,
:
(x*
t*,
q*,
p*)
=
(X
+
€a,
t,
q
+
€a',
p
-
EX
*
a"
-
*
a").
Here
a=
(a(t),P(t),r(t))
and
G,
is generated by the linear combi-
nation
r,
=
r4
+
r5
+
r6.
(iv) Simultaneous Rotation in Space and the Velocity Vector
Field:
SO(3)
:
(x*,t*,q*,p*)
=
(Rx,t,Rq,p).
Here
R
is an arbitrary
3
x
3
orthogonal matrix.
SO(3)
is
generated
by
a
linear combination
of
I'7,
I's
and
rg.
(v) Pressure Changes:
Go
:
(x*,t*,q*,p*)
=
(x,t,q,p
t
4t))
The action
of
the various above subgroups on
a
solution set
{q(x,t),p(x,t)}
of
the Navier-Stokes system is to generate new
so-
lutions as set down below:
{q(x
-
€cu,t)
+
m',p(x
-
m7t)
-
EXd
-
-€
12
CY
a/'},
2

Symmetry in Nonlinear Mechanics
55
Subgroups of the symmetry group may be employed to generate
solutions of the Navier-Stokes system by symmetry reduction
(Bo-
sivert, 1982 and Rogers and Ames, 1989).
To
save space we shall indicate several applications of the sub-
groups of
(3.3)
in two space dimensions
(2,
y)
and time t. Eliminating
all
terms in
z
and
w
leaves the operators
rl,
r2,
r3,
r6,
r7,
and
rg
for
the two-dimensional system. With
a
=
1
and
,L?
=
7
=
0
(deleting
r2
and
r3),
the Lie operator
r
becomes
The first order equation
r1
=
0
has the characteristics (invariants)
where
F‘
=
f,
G’
=
g,
while
where
The functions,
U,
.ii,
and
jj
satisfy
the
steady
Navier-Stokes equations
As is true in other systems in fluid mechanics the generator of
pure time translation,
rl,
exists. Hence it is possible to “factor
out” the explicit time dependence, as indicated above. This is
a
useful result since any solution of the steady equation,
(3.6),
can
be transformed by means
of
equations
(3.5),
into
a
time dependent

56
W.
F.
Ames
solution containing three arbitrary functions of time.
A
similar result
holds in three dimensions.
Many applications of group analysis for the determination of
so-
lutions by symmetry reduction are available (Bosivert,
1982,
Rogers
and Ames,
1989).
We illustrate one surprising one here.
Dropping the bars on equations
(3.6)
and using the stream func-
tion form with
v
=
+x
and
u
=
-$y,
there results
+X+YYY
+
+x+xxy
-
+ybyy
-
$y+zxx
-
P(+ZXXX
+
2+xxy,
+
+yyyy)
=
0
(3-7)
The rotation group is obtained from
(3.3)
by setting
(Y
and
p
equal to
zero and including only
r3
(in stream function form). The invariants
are
7
=
-z2
+
y2,
+
=
f(q),
and when these are substituted into
(3.7)
one obtains the
linear equation
which is independent of
p!
This is
a
surprising result. The general
solution, first obtained by Hamel (using ad hoc methods), is
where the c; are arbitrary constants. The associated time-dependent
solutions are
1
p
=
--z
f'(t)
-
ygt(t)
+
k(t)
-
$7-1
where
77
=
[-z
-
F(t)I2
+
[y
-
G(t)I2.
Gusyatnikova and Yumaguzhin
(1989)
revisited the Navier-Stokes
equations and they derive the above result when potential extrinsic

Symmetry in Nonlinear Mechanics
57
force is employed.
A
complete answer for nonpotential extrinsic force
is also found
as
is the space of all conservation laws for the Navier-
Stokes equations.
Some symmetry group generation has taken place in other coordi-
nate systems.
For example Kapitanski
(1978, 1980)
does the group
analysis of the Euler and Navier-Stokes equations in the presence
of
rotational symmetry. Nucci
(1987)
finds the algebra for
a
non-
steady axisymmetric incompressible viscous system. In related areas
the collisonless plasma equations have been investigated by Taranov
(1978),
a
nonsteady dissipative magneto-hydrodynamic system by
Nucci
(1984),
a
steady non-dissipative magneto-hydrodynamic sys-
tem by Rogers and Winternitz
(1988)
and the Zakharov equations
i$t
+
$,,
-
rill,
=
0,
nt
+
u,
=
0
Ut
+
nx
+
(1$I2)x
=
0
by Verbovetsky
(1989).
This system describes the interaction of
acoustic and Langmuir plasma waves.
3.3
Gas
Dynamics Equations
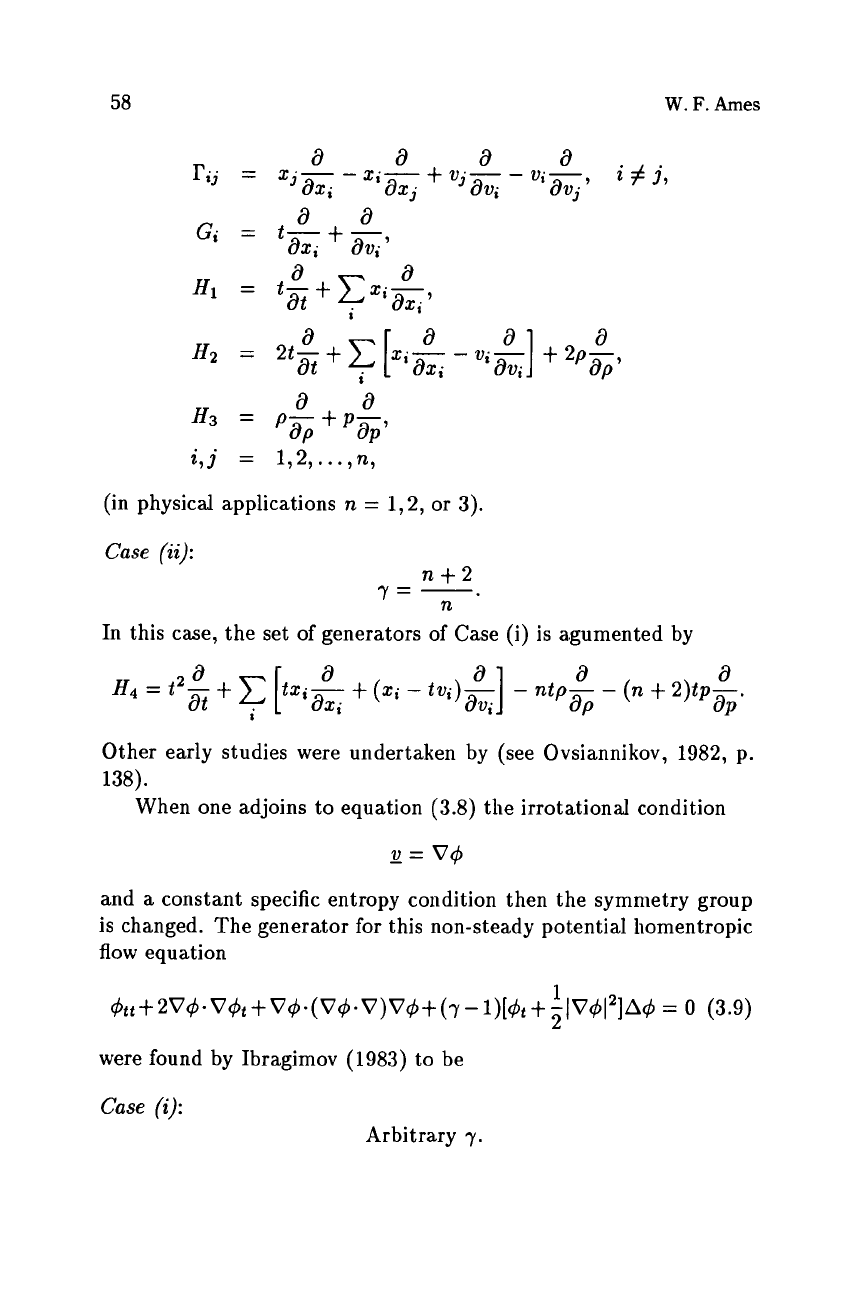
Various problems in gas dynamics, and other systems modeled with
equations that resemble the equations of gases, have benefited by the
availability of the symmetry group. Ovsiannikov
(1962)
obtained the
generators for the equations
pt+g.Vp+pdivg
=
0
pt+g-Vp+ypdivg
=
0
p[gt
+u.Vg]
+
vp
=
0
v=
(%...,%)
(3.8)
of motion for
a
polytropic gas.
Case
(i):
Arbitrary
7
upon n(n
+
3)/2
+
4
parameters. The generators are
d d
8s;
In this case, the system admits an invariance group dependent
ro
=
-
ri
=
-9
58
W.
F.
Ames
a a a
,
+v~--v;-,
i#j,
a
r..
=
x.--x.-
QX;
axj
av;
avj
13
aa
axj
avj
a a
G;
=
t-+-,
HI
=
t-+cx;-,
at
;
ax;
a
a
a
H2
=
2t,t+q
a a
H3
=
p-
+
P-,
ap
ap
i,j
=
1,2
,...,
n,
(in physical applications
n
=
1,2,
or
3).
Case
(ii):
n+2
y
=
-*
n
In this case, the set
of
generators
of
Case (i) is agumented by
2a
a
a
a
H4
=
t
-
+
C
tx;-
+
(x;
-
tv;)-
-
ntp-
-
(n
+
2)tp-.
at
;
[
ax;
dv;
"1
ap
aP
Other early studies were undertaken by (see Ovsiannikov,
1982,
p.
138).
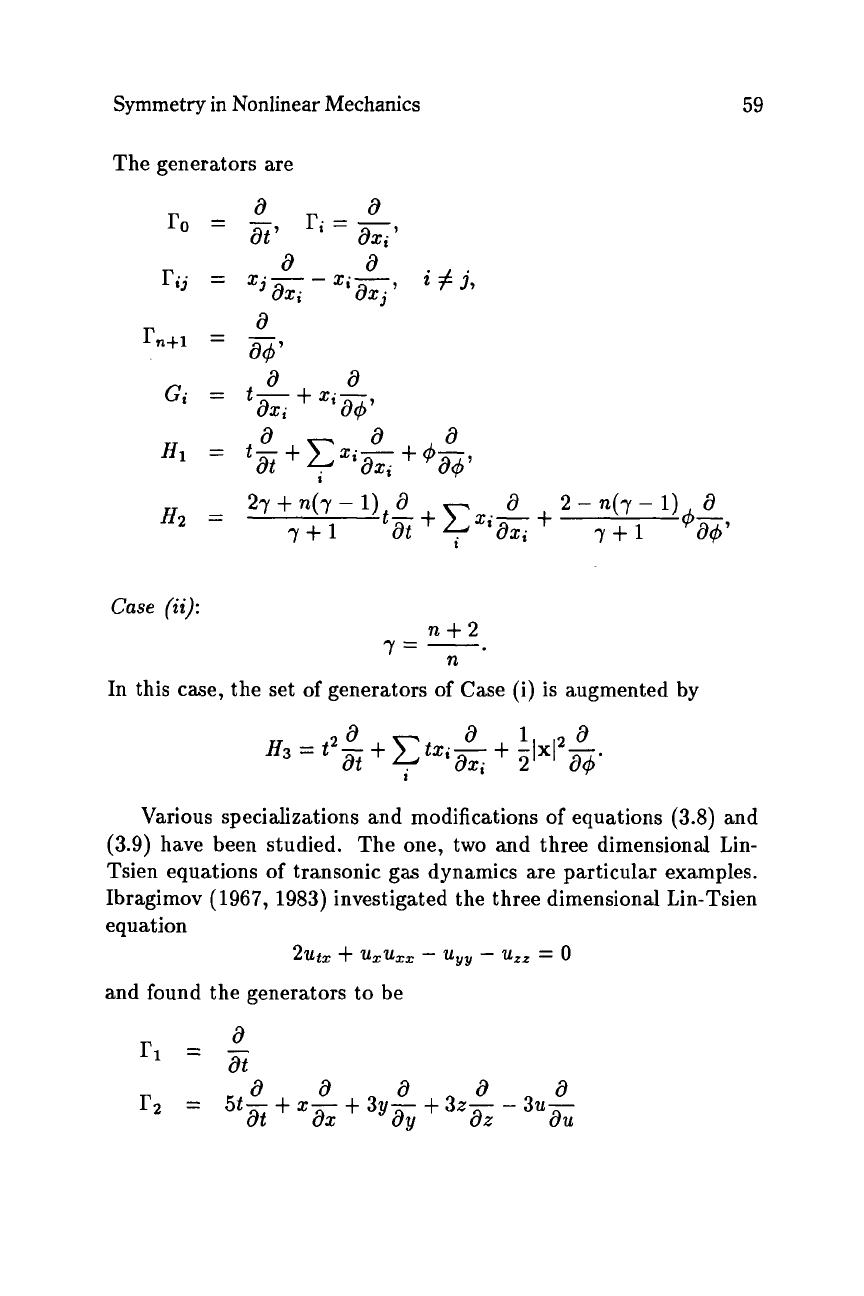
When one adjoins to equation
(3.8)
the irrotational condition
-
v
=
vq5
and
a
constant specific entropy condition then the symmetry group
is changed. The generator for this non-steady potential homentropic
flow equation
1
drt+2Vq5.Vq5,+Vq5.(Vq5.V)Vq5+(y-1)[~,+21Vq512]Aq5
=
0
(3.9)
were found by Ibragimov
(1983)
to be
Case
(i):
Arbitrary
y.
Symmetry
in Nonlinear
Mechanics
59
The generators are
Case
(ii):
nt2
n
y
=
-*
In this case, the set
of
generators
of
Case (i) is augmented by
a
dld
H3
=
t2-
+
xtxj-
+
-1x12-
at
.
axj
2
aqs
Various specializations and modifications
of
equations
(3.8)
and
(3.9)
have been studied. The one, two and three dimensional Lin-
Tsien equations
of
transonic
gas
dynamics are particular examples.
Ibragimov
(1967, 1983)
investigated the three dimensional Lin-Tsien
equation
2%
+
U-X~xz
-
uyy
-
u,,
=
0
and found the generators to be
d
at
rl
=
-
d
I3
d
d
d
rz
=
5t-
+
x-
+
3y-
+
3z-
-
3u-
at
ax
ay
at.
aU
