Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


This page intentionally left blank

Symmetry
in
Nonlinear
Mechanics
W.
F.
Ames
School
of
Mathematics
Georgia
Institute
of
Technology
Atlanta,
GA
30332
1
Introduction
The mathematical models of many problems in mechanics consist of
nonlinear partial differential equations. This chapter is concerned
with invariance of those equations under groups of Lie transforma-
tions and the resulting qualitative properties and symmetry reduc-
tions.
A
systematic investigation of continuous transformation groups
was carried out by Lie
(1882-1899).
His original goal was the creation
of
a
theory
of
integration for ordinary differential equations analogous
to the Abelian theory for the solution of algebraic equations. He in-
vestigated the fundamental concept of the invariance group admitted
by
a
given system of differential equations. Today, the mathemat-
ical approach whose object is the construction and analysis of the
full invariance group admitted by
a
system
of
differentid equations
is called
group
analysis
of differential equations. These groups, now
usually called Lie groups, and the associated Lie algebras have im-
portant real world applications. In particular, they bear upon the
concepts of homogeneity and isotropy of space and time, the dynamic
similarity of physical phenomena, as well
as
Galilean and Lorentzian
invariance.
The task of determining the largest group of Lie transformations
that leaves invariant
a
system of differential equations involves purely
mechanical, albeit lengthy procedures, well-suited to symbolic com-
Nonlinear Equations
in
the Applied Sciences
31
Copyright
0
1992
by Academic
Press.
Inc.
M
rights
of
reproduction
in
any
form
reserved.
ISBN
0-12-056752-0

32
W.
F.
Ames
putation. Once the group is known, one can study the action of the
group on the set of solutions to the equations. Thus, the group in-
variance may be utilized to generate new solutions from known ones.
Moreover, the admitted group introduces an algebraic structure that
allows important classifications of solutions. Subalgebras may be
analyzed and group invariants used in reductions of the original sys-
tem. Exact solutions
of
these reduced systems may, on occasion, be
derived.
The differential equations
of
engineering and the physical sci-
ences often involve parameters
or
constitutive laws that are deter-
mined experimentally (here these are called
arbitmry
elements).
The
equations of the mathematical model should be simple enough to be
amenable to analysis. The group approach suggests the acceptance
of, as
a
simplicity criterion, the requirement that the arbitrary ele-
ment be such that, with it, the model differentid equation admits
appropriate group invariance.
There is
a
considerable literature on group analysis. We must
begin with the basic works of Lie
(1888a, 1890, 1891, 1893).
El-
ementary introductory books include those by Page
(1897),
Cohen
(1911)
and Ince
(1956).
They use Lie’s theory of one-parameter
groups with special application to ordinary differential equations.
Birkhoff’s monograph discusses the symmetry concepts in fluid me-
chanics in
a
group-theoretic manner
(1960).
Since that fine study, the
application and generalization of group analysis has blossomed. By
1962,
Ovsiannikov’s first work had appeared and was subsequently
translated by Bluman (Ovsiannikov,
1962)
in
1967.
In
1964,
Hansen
(Hansen,
1964)
published
a
volume devoted to
a
number of engineer-
ing studies in diffusion and fluid mechanics. In
19G5,
the first book
on nonlinear problems by Ames
(1965)
treated similarity by specific
groups (dilation and translation) and gave applications in engineer-
ing. In
1968,
Ames
(1968,
Ch.
2
and
3),
provided an introduction to
the group concept wtih
a
number
of
applications to ordinary differ-
ential equations in fluid mechanics.
More detailed group analysis, aimed primarily at group construc-
tion and analysis for partial differential equations, is to be found in
a
number of recent books (arranged chronologically). These include

Symmetry in Nonlinear Mechanics
33
Ames
(1972),
Bluman and Cole
(1974),
a
second definitive volume by
Ovsiannikov
(1978)
and
a
related work by Anderson and Ibragimov
(1979).
Barenblatt
(1979),
Na
(1979)
and Seshadri and
Na
(1985)
include many applications in engineering, while Hill
(1982b)
has one-
parameter group applications. Ibragimov
(1983)
is concerned with
appljcations
of
mathematical physics and Winternitz
(1983)
with
nonlinear equations. Applications in physics, geometry and mechan-
ics are given by Sattinger and Weaver in
(1986).
An excellent general
theory of the subject
is
presented by Olver
(1986).
Rogers and Ames
(1989)
have collected
a
large table of available groups. Bluman and
Kumei
(1989)
give
a
comprehensive treatment of Lie groups in
a
book
which can be used as
a
textbook.
Collections of papers, reporting group calculations, include
Group
Theomtical Methods in Mechanics
(Ibragimov and Ovsiannikov, eds.)
in
1978,
Group Theoretical Methods in Physics
(Beiglbock, Bohm and
Takasugi, eds.) in
1979,
Symposium on Nonlinear Integrable Systems
(Jumbo and Miwa, eds.,
1983),
Nonlinear Phenomena
(Wolf, ed.) in
1983,
and
Symmetry Methods in Diflerential Equations
(Anderson
and Olver, eds.) in
1987,
and
Symmetries
of
Partial Diflerential
Equations
-
Conservation
LQWS
-
Applications
-
Algorithms
in
1989
(A.
M.
Vinogradov, ed.). Lastly, in
a
more general context, the
book of Konopelchenko
(1987)
is an outstanding compendium, with
over
600
references
on
the literature
of
nonlinear integrable equations.
1.1
Some authors, in their studies of nonlinear wave equations, carry
out
a
direct application
of
the scaling
or
other simple groups such
as translation. This leads, when applicable
to
similar
or
travelling
wave solutions, respectively. This time saving approach is carried
out because in many physical models, that are based upon Newto-
nian mechanics, invariance under scaling (dilation), translation and
rotation is to be expected.
Wave Equations and Group Invariance
34
W.
F.
Ames
1.2
Many applications of the direct approach are found.
For
example
Suliciu et a1
(1973),
Ames and Suliciu
(1982),
Ames and Donato
(1988)
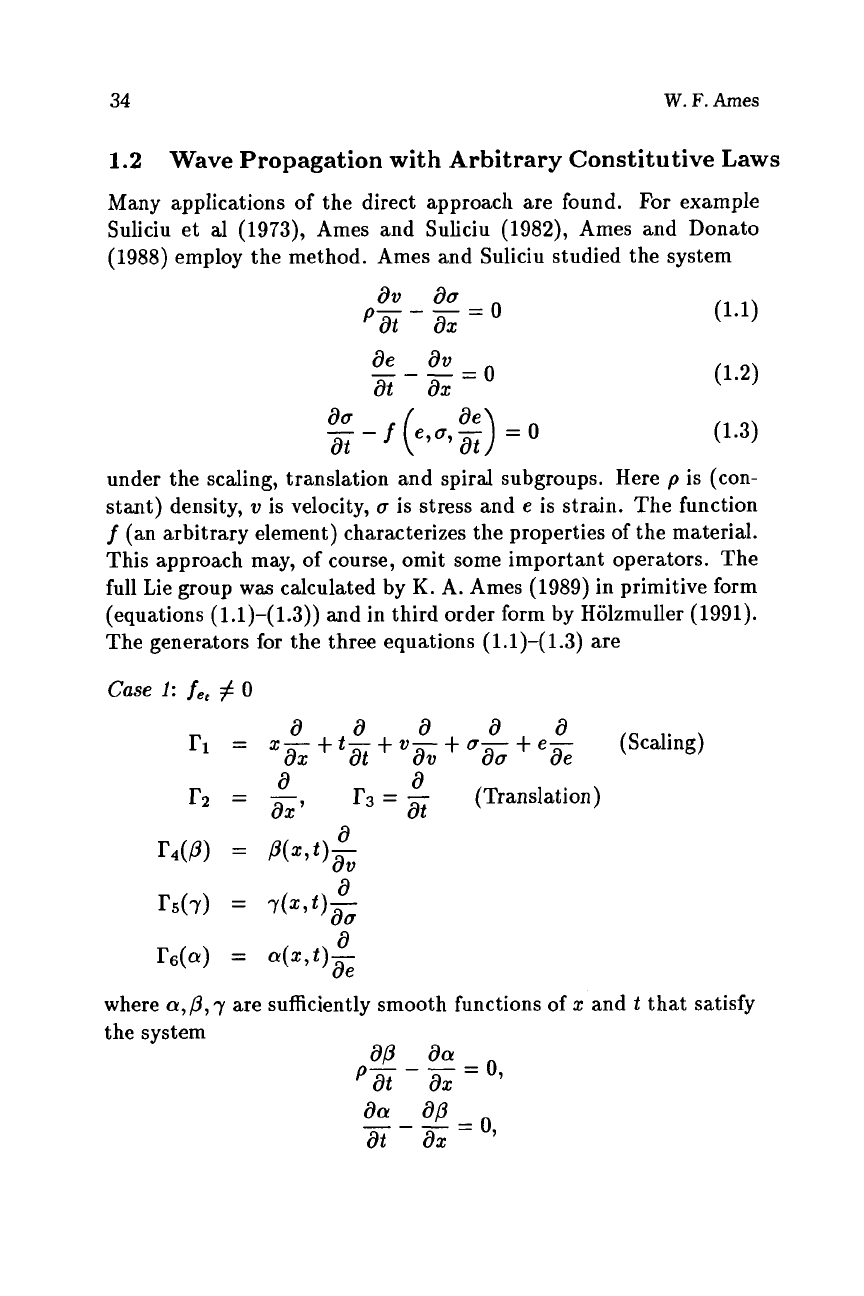
employ the method. Ames and Suliciu studied the system
Wave Propagation with Arbitrary Constitutive Laws
av
du
p----
-0
at
ax
de
av
at ax
=o
---
-
aU
-
f
(e,u,$)
=
o
at
under the scaling, translation and spiral subgroups. Here
p
is (con-
stant) density,
v
is velocity,
u
is stress and
e
is
strain. The function
f
(an arbitrary element) characterizes the properties of the material.
This approach may, of course, omit some important operators. The
full Lie group was calculated by
K.
A. Ames
(1989)
in primitive form
(equations
(1.1)-( 1.3))
and in third order form by Holzmuller
(1991).
The generators for the three equations
(1.1)-( 1.3)
are
Case
1:
fet
#
0
aaa
a a
ax
at
80
ae
rl
=
x-
+
t-
+
v-
-t
u-
+
e-
(Scaling)
(Translation
)
a a
r
=
-,
r,=%
ax
2
where
a,@,
7
are sufficiently smooth functions of
x
and
t
that satisfy
the system
ap
aa
aa
ap
at
ax
PZ-z=
0,
----
-
0,
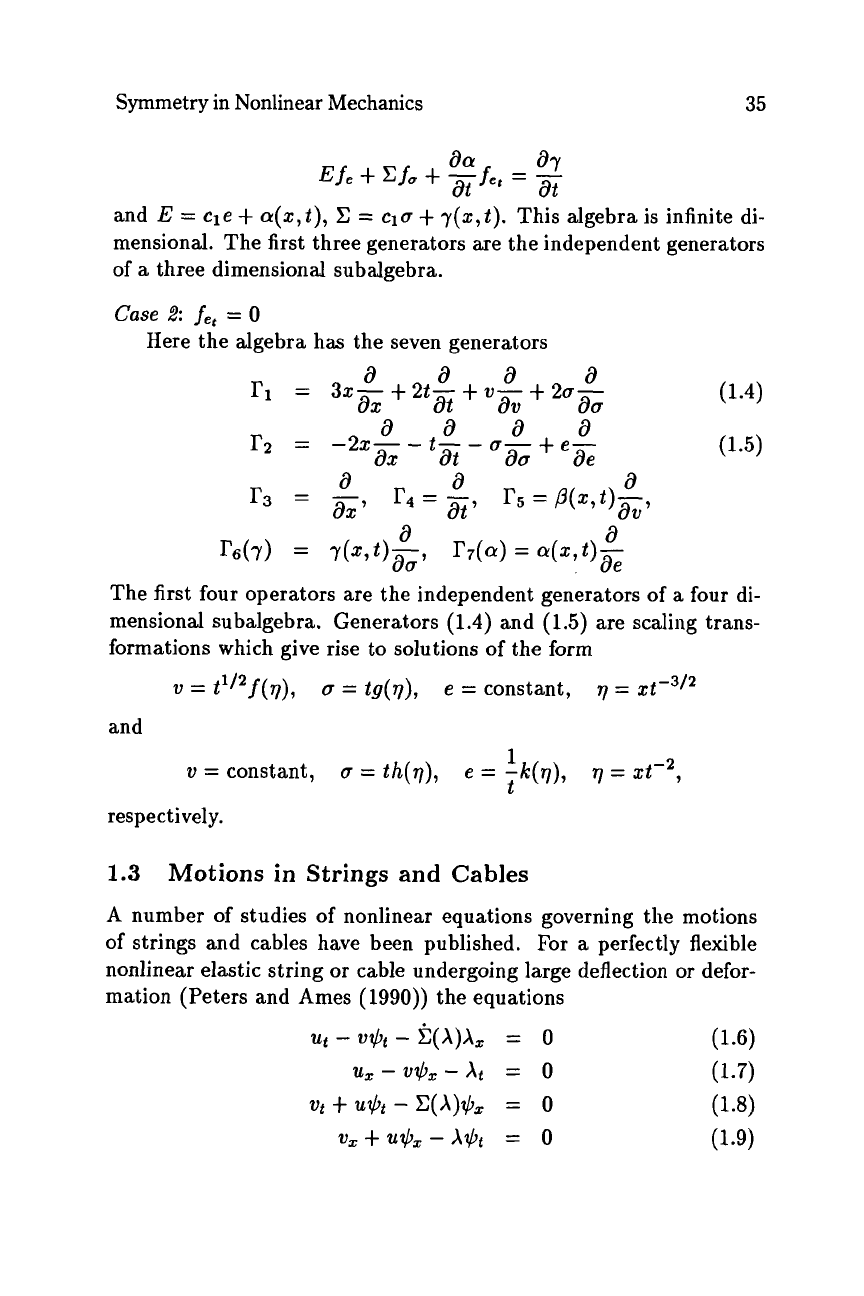
Symmetry in Nonlinear Mechanics
35
and
E
=
cle
+
a(x,t),
C
=
c1u
+
y(x,t).
This algebra is infinite di-
mensional. The first three generators are the independent generators
of
a
three dimensional subalgebra.
Case
2
fe,
=
0
Here the algebra has the seven generators
a
a
d d
ax
at
av
au
ad
a
a
dx
dt
du
ae
a a
a
ax
’
rl
=
3x-++2t-+v-++2a-
(1.4)
r2
=
-2x--t--u-+e-
(1.5)
r3=-
r-
4
-
at,
rs
=
P(2,t)Z’
a
r7(a)
=
a(x,t)-
r6(7)
=
7(x,
t)G,
de
a
The first four operators are the independent generators
of
a
four di-
mensional subalgebra. Generators (1.4) and
(1.5)
are scaling trans-
formations which give rise to solutions of the form
and
respectively.
1.3
A number of studies of nonlinear equations governing the motions
of strings and cables have been published.
For
a
perfectly flexible
nonlinear elastic string
or
cable undergoing large deflection
or
defor-
mation (Peters and Ames
(1990))
the equations
Motions
in
Strings
and
Cables
Ut
-
v+t
-
k(X)X,
=
0
(1.6)
u,
-
v+,
-At
=
0
(1.7)
vt
+
u+t
-
C(X)&
=
0
(1.8)
v,
+
U+Z
-
X$t
=
0
(1.9)
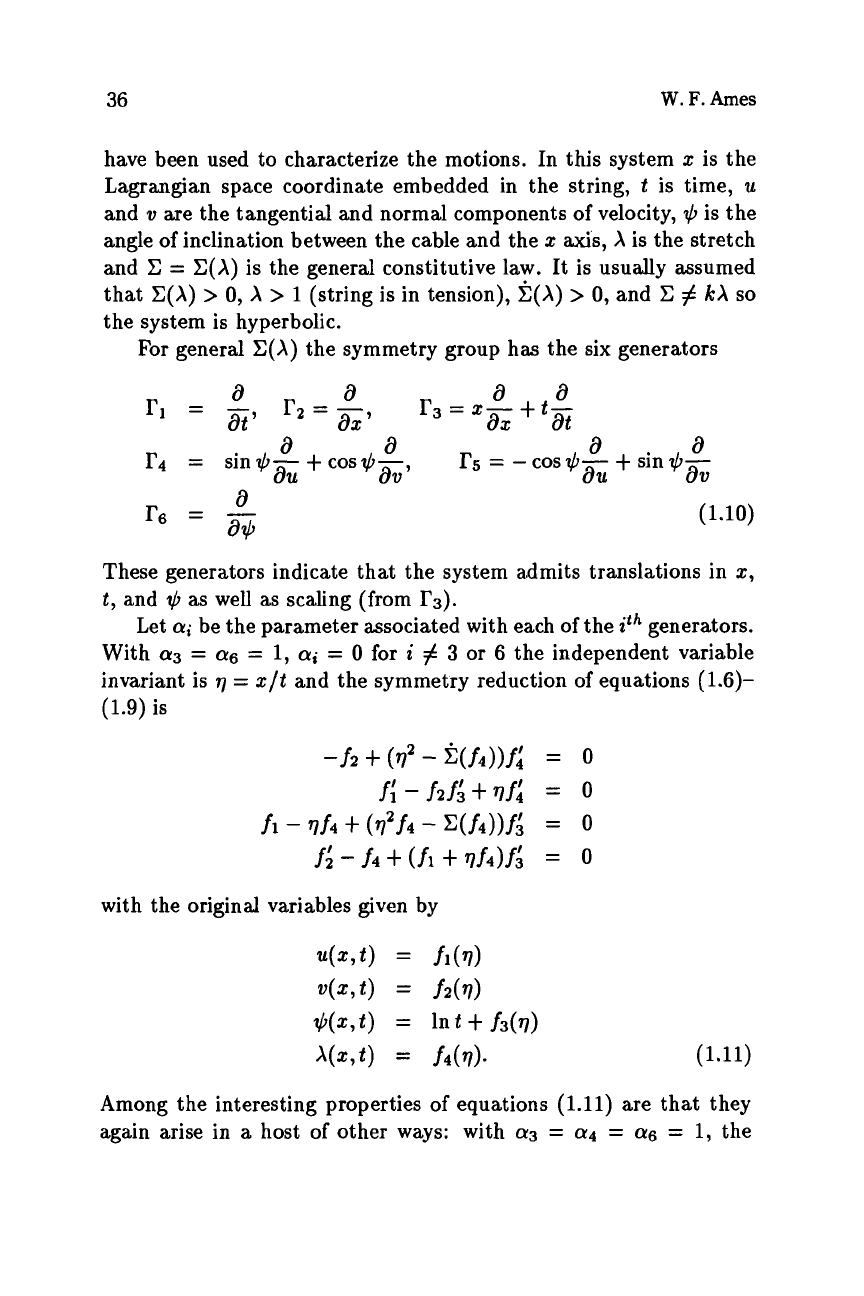
36
W.
F.
Ames
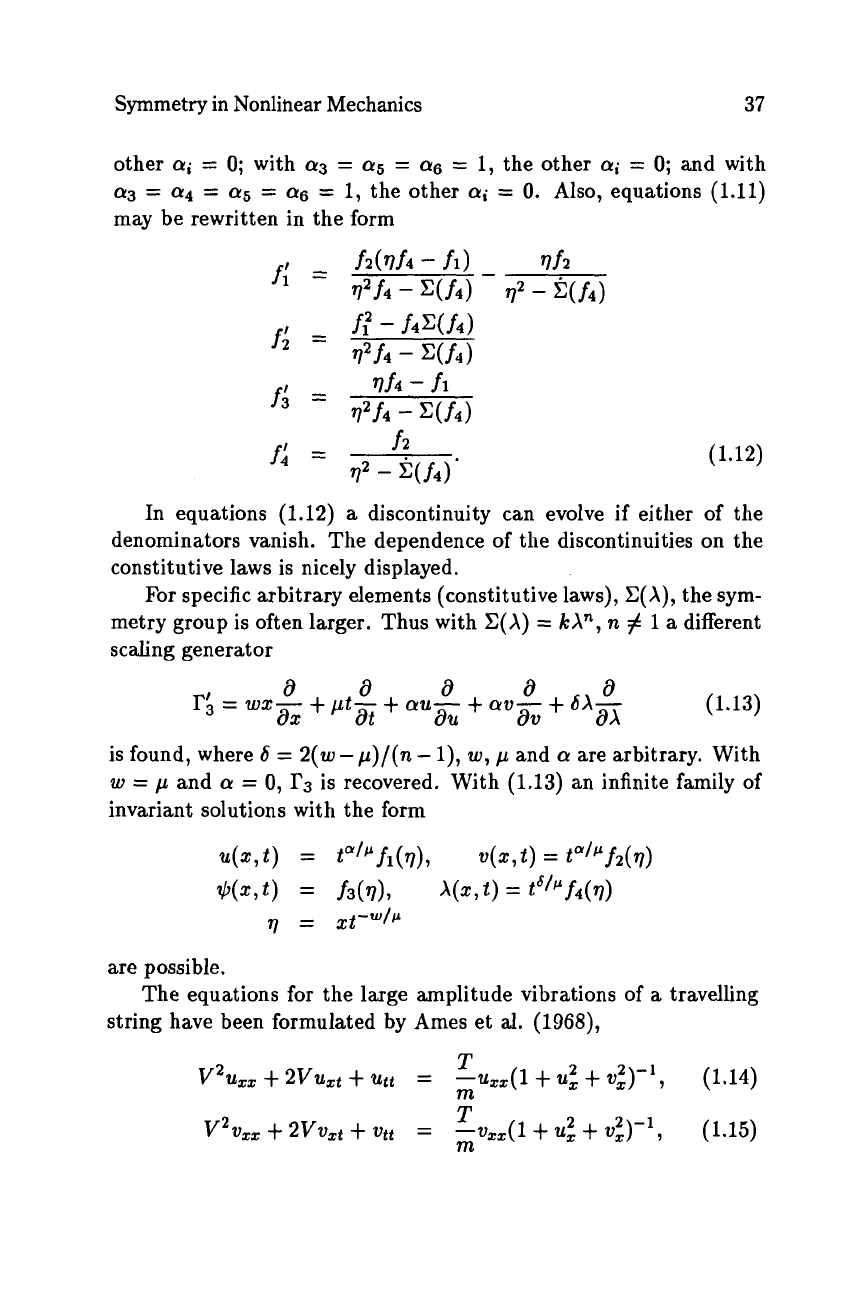
have been used to characterize the motions. In this system
x
is the
Lagrangian space coordinate embedded in the string,
t
is time,
u
and
v
are the tangential and normal components of velocity,
$
is the
angle of inclination between the cable and the
x
axis,
A
is
the stretch
and
C
=
C(X)
is the general constitutive law. It is usually assumed
that
C(X)
>
0,
X
>
1
(string is in tension),
&(A)
>
0,
and
C
#
kX
so
the system is hyperbolic.
For
general
C(X)
the symmetry group has the six generators
aa
r3
=
5-
tt-
at
’
ax
,
ax
at
a
r2
=
-
a
rl
=
-
a a
a
a
r4
=
sin
$-
+
cos
y3-,
r5
=
-
cos
y3-
t
sin
y3-
au
dV
bU
dV
(1.10)
These generators indicate that the system a,dmits translations in
x,
t, and
$
as well as scaling (from
r3).
Let
a;
be the parameter associated with each of the
ith
generators.
With
a3
= =
1,
a;
=
0
for
i
#
3
or
6
the independent variable
invariant is
7
=
x/t
and the symmetry reduction of equations
(1.6)-
(1.9)
is
-f2
t
(q2
-
k(f4))fi
=
0
fi
-
f2fi
t
17fi
=
0
fl
-
rlf4
+
h2f4
-
C(f4))f::
=
0
f;
-
f4
t
(fl
t
77f4)fi
=
0
with the original variables given by
Among the interesting properties
of
equations
(1.11)
are that they
again arise in
a
host of other ways: with
a3
=
a4
=
a6
=
1,
the
Symmetry in Nonlihear Mechanics
37
other
ai
=
0;
with
a3
=
a5
=
=
1,
the other
ai
=
0;
and with
a3
=
a4
=
a5
=
a6
=
1,
the other
a;
=
0.
Also, equations (1.11)
may be rewritten in the form
f;
=
fz
rlz
-
2(f4)’
(1.12)
In equations (1.12)
a
discontinuity can evolve if either
of
the
denominators vanish. The dependence of the discontinuities on the
constitutive laws is nicely displayed.
For specific arbitrary elements (constitutive laws),
C(X),
the sym-
metry group is often larger. Thus with
C(X)
=
kXn,
n
#
1
a
different
scaling generator
a
a
a a a
r;
=
wx-
+
pt-
+
au-
+
av-
+
~x-
ax at dU
av
ax
(1.13)
is found, where
S
=
2(w
-p)/(n
-
l),
w,
p
and
a
are arbitrary. With
w
=
p
and
a
=
0,
r3
is recovered. With (1.13) an infinite family of
invariant solutions with the form
are
possible.
string have been formulated by Ames et
al.
(1968),
The equations for the large amplitude vibrations of
a
travelling
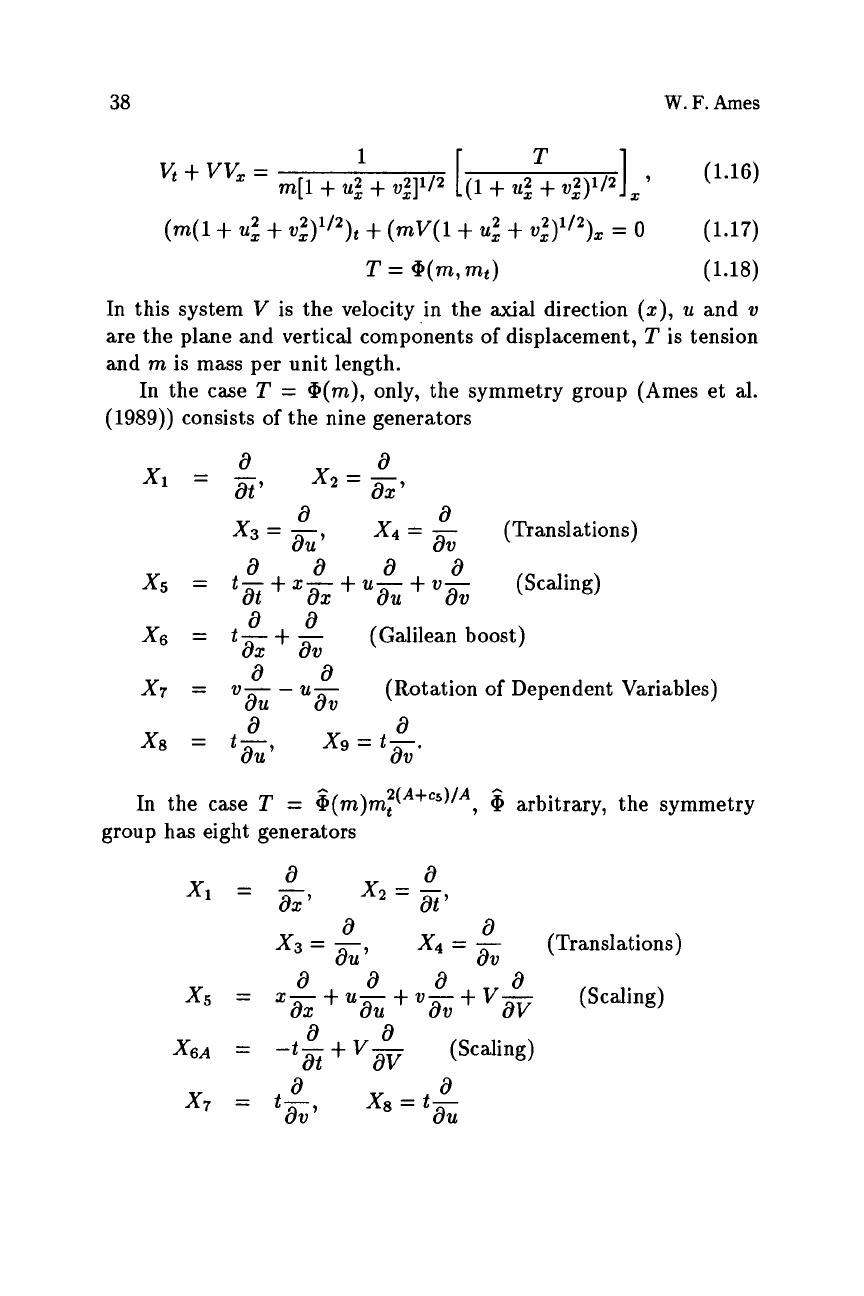
38
W.
F.
Ames
21:
+
v,)
2
112
)t
+
(mV(l+
u:
+
v,)
2
112
)z
=
0
(1.17)
T
=
@(m,mt)
(1.18)
In this system
V
is the velocity in the axial direction
(x),
u
and
v
are the plane and vertical components
of
displacement,
T
is
tension
and
m
is mass per unit length.
In the case
T
=
@(m),
only, the symmetry
group
(Ames et al.
(1989))
consists
of
the nine generators
a
a
x1
=
-
at’
x2
=
-
ax
’
(Translations)
a
a
x3=-,
xq=-
aU
aV
a a
a
a
at
ax
du
dv
X5
=
t-++-+u-+v- (Scaling)
aa
ax
av
x6
=
t-
+
-
(Galilean boost)
X7
=
v-
-
u-
(Rotation
of
Dependent Variables)
a
a
au
av
In the case
T
=
6(m)mt
2(A+c5)1A,
6
arbitrary, the symmetry
group
has eight generators
a
d
XI
=
-
ax,
x2
=
8
(Translations)
d
x3=-,
x,=-
bU
aV
a
a
a a
x5
=
x-+u-+v-+v-
ax
du
av
av
(Scaling)
a
d
at
av
(S
Cali ng
)
X6A
=
-t-+vV--

Symmetry in Nonlinear Mechanics
39
where
A
is the arbitrary real number appearing in the constitutive
law and
c5
is the scalar associated with the scaling generator
Xs.
The scaling generator in the
T
=
@(m)
case has been used by
Ames and Donato
(1988)
to study the evolution
of
weak discontinu-
ities
of
this system in the plane, in
a
state characterized by invariant
solutions. Donato and Oliveri
(1988)
give
a
more convenient formu-
lation of equations
(1.14)-(1.18)
in order to study the occurrence
of
shock waves. They determine, in terms
of
the initial conditions, the
critical time when the weak discontinuity becomes unbounded. Also
studied is the interaction of
a
weak discontinuity with
a
shock wave.
1.4
Quasilinear Wave Equations
In Ames et
al.
(1981)
it
was demonstrated how
a
number of physical
problems from gas dynamics, shallow water waves, dynamics
of
a
nonlinear string, elastic-plastic materials and electromagnetic trans-
mission line satisfied the common quasilinear hyperbolic equation
where we assume
f
E
C2(IR),
f
>
0
and
f'
#
0.
The symmetry
group was found by Ames et al.
(1981).
The group is presented in
several special cases in dependence on
f(u).
Case
A:
f(u)
arbitray
(1.20)
dd
r3
=
2-
+
t-
r2
=
-
at
'
ax
at
rl
=
-
d
d
dX
'
Case
B:
f
=
cexp(mu),
c,
m
real and arbitrary.
a
a
r1,r2,r3
of
(1.20)
and
r4
=
2-
+
mx-
dU
dX
Case
C:
f
=
c(u
-+
d)",
c,
m
real and arbitrary.
d d
rl,r2,r3
of
(1.20)
and
rl,
=
2(~
+
d)-
+
WZX-
(1.21)
aU
ax
