Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


10
Karen
A.
Ames
or,
observing that
Jot
Jn
u,uPdzdr
=
&
Jn
Ihdz,
FN(t)
-4(
1
+
2a)
E(0)
+
-
J
fP+ldz}
+
2k.
(3.12)
{
P+1
n
We now form the expression
FF"
-
(1
+
Q)(F'>~
using
(3.4), (3.6)
and
(3.12).
After observing that the term 4aFllgrad
.[I2
is
clearly
nonnegative, we obtain the inequality
2
FF"
-
(1
+
CY)(F')~
2
4(
1
+
a)s2
+
-F(P
-
1
-
4a)
A
uP+'dz
P+1
-2(1+ 2a)F{k
+
2E(O)} (3.13)
where
and
Since
s2
2
0
by the Cauchy-Schwarz inequality, we need to ensure
that the second and third terms on the right side
of
the inequality
in
(3.13)
are nonnegative in order to obtain
(3.5).
The second term
will be nonnegative if we choose
a
=
>
0.
We then have the
inequality
FF"
-
(1
+
a)(F')2
2
-(/3
+
l)F{k
+
2E(O)). (3.15)
Using
(3.15)
we can now obtain the following result:
Theorem
1
A
global solution
of
(3.1)-(3.3)
cannot exist
if
(u)
&O)
<
0
or
(b)
z(0)
=
0
and
Jn
fgdx
>
0.
To
prove part (a) of the theorem we choose
k
=
-22(0)
>
0.
Then
(3.15)
becomes
FF"
-
(1
+a)(
F1)2
2
0
and therefore
(
F-cI)"
5
0.
If we integrate this inequality twice, we find that
F-O(t)
5
F-a(0)
-
aF-Q-l (O)F'(O)t

Improperly Posed Problems for Nonlinear PDEs
11
from which it follows that
(3.16)
Recalling that
F'(0)
=
2
Jn
fgdx+2kto,
we choose
to
so
that
P(0)
>
0
and observe that the right side of (3.16) becomes unbounded at
t*
=
a.
Consequently,
~(t)
must cease
to
exist at some time less
than
or
equal to
t'.
It follows that the solution
u
cannot exist for
all
time.
To
establish
(b)
we take
k
=
0.
In this case, we have
F'(0)
=
2
Jn
fgds
>
0
by assumption. Similar arguments as those used in
part
(a)
lead to the global nonexistence result. In both situations
we also obtain an upper bound
t*
for the time
at
which the solution
ceases to exist. Such upper bounds can usually be read directly from
the inequality that establishes nonexistence
of
solutions in most
of
the methods used
to
determine these type
of
results. Lower bounds
for this time are more difficult to obtain but can be found for some
problems, e.g., the Cauchy problem
for
a
nonlinear heat equation
(see Payne, 1975).
We remark that there exist nonexistence results in the literature
for
(3.1)-(3.3) if E(0)
>
0
but the arguments needed to derive them
are somewhat more detailed (Knops et.
al.
1974; Levine 1974b,c;
Straughan, 1975b). Similar results can be demonstrated
if
we re-
place the nonlinearity
d
in
(3.1)
by any nonlinear function
h(u)
that satisfies
h(0)
=
0
and the condition
(3.17)
In fact, such problems can be analyzed in
a
more abstract setting
(see Payne, 1975 for
a
model problem). We observe that global
nonexistence theorems for nonlinear hyperbolic equations are typi-
cally desired for weak solutions since
a
number
of
these equations
may not possess classical solutions. Concavity arguments
can
be
easily modified to deal with appropriately defined weak solutions.
The concavity technique extends to problems where the nonlin-
earity appears in the boundary condition. Nonexistence results, for
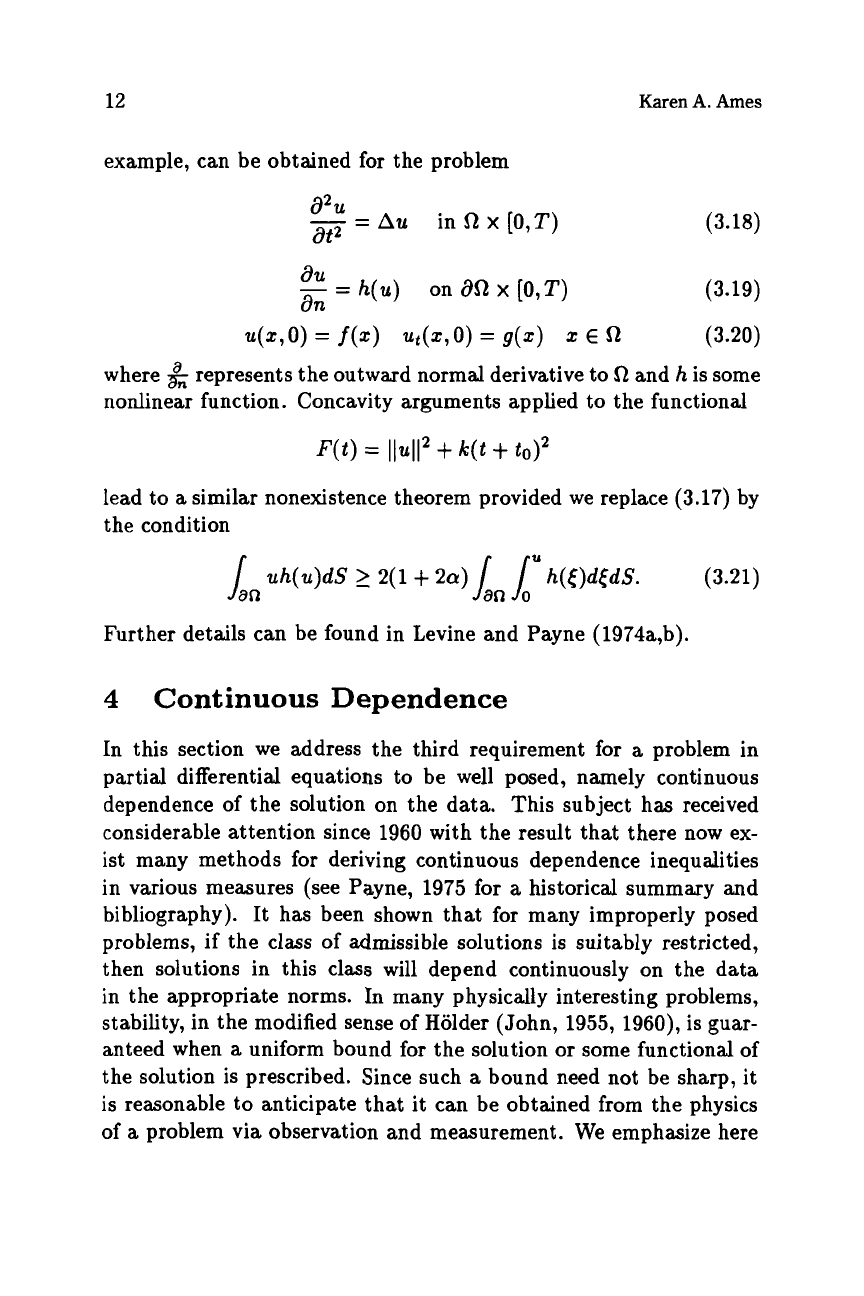
12
Karen
A.
Ames
example, can be obtained for the problem
8%
-
=
Au
at2
in
52
x
[O,T)
aU
-
=
h(u)
an
on
852
x
[O,T)
(3.18)
(3.19)
(3.20)
where
&
represents the outward normal derivative to
52
and
h
is some
nonlinear function. Concavity arguments applied to the functional
lead to
a
similar nonexistence theorem provided we replace (3.17) by
the condition
uh(u)dS
2
2(1+ 2a)l
lU
h(()d(dS.
(3.21)
an
0
Further details can be found in Levine and Payne (1974a,b).
4
Continuous Dependence
In this section we address the third requirement for
a
problem in
partial differential equations to be
well
posed, namely continuous
dependence of the solution on the data. This subject has received
considerable attention since 1960 with the result that there now ex-
ist many methods for deriving continuous dependence inequalities
in various measures (see Payne, 1975 for
a
historical summary and
bibliography). It has been shown that for many improperly posed
problems, if the class of admissible solutions is suitably restricted,
then solutions in this class will depend continuously on the data
in the appropriate norms. In many physically interesting problems,
stability, in the modified sense of Holder (John, 1955,
1960),
is guar-
anteed when
a
uniform bound for the solution or some functional of
the solution is prescribed. Since such
a
bound need not be sharp, it
is reasonable to anticipate that it can be obtained from the physics
of
a
problem via observation and measurement. We emphasize here

Improperly Posed Problems for Nonlinear PDEs
13
that this type of continuous dependence should not be confused with
asymptotic stability or with the usual notion of continuous depen-
dence defined
for
well posed problems.
an important pursuit because of the central role such estimates play
in obtaining numerical solutions to ill-posed problems for both lin-
ear and nonlinear equations. We point out that such inequalities also
imply uniqueness
of
solutions.
Our
discussion of stability in the context
of
improperly posed
problems will focus on three important types of errors that can be
introduced in the derivation and analysis of
a
mathematical model
for
a
physical process:
The derivation of continuous dependence inequalities has remained
1.
errors in measuring data (initial or boundary values, coeffi-
cients in the equations
or
boundary operators, parameters),
2. errors in characterizing the spatial geometry of the underlying
domain and the initial-time geometry,
3.
errors in formulating the model equation.
In order to recover continuous dependence in ill posed problems, we
must constrain the solution in some mathematically and physically
realizable manner. It would be optimal if this constraint could si-
multaneously stabilize the problem against all possible errors that
might arise
as
we set up the model. However, it is usually the case
that the appropriate constraint is difficult to determine. Moreover,
such
a
restriction will turn an otherwise linear problem into
a
nonlin-
ear one and thus caution must be exercised in any attempt to study
the effects of various errors separately and to then superpose these
effects.
4.1
Questions
of
continuous dependence of solutions on Cauchy data
have received the most attention in the literature.
A
number
of
such studies have appeared for various nonlinear
ill
posed problems:
the final value problem for the Navier-Stokes equations (Knops and
Continuous Dependence on Cauchy Data

14
Karen
A.
Ames
Payne, 1968; Cannon and Knightly, 1970; Galdi and Straughan,
1988);
the conduction4iffusion Boussinesq equations backward in
time (Straughan, 1975a, 1977); first and second order nonlinear op-
erator equations (Levine, 1970a, b); and nonstandard Cauchy prob-
lems for nonlinear parabolic equations (Bell, 1981a,b; Payne, 1985;
Payne and Straughan, 1990a).
The logarithmic convexity and the weighted energy methods have
been particularly successful in deriving continuous data dependence
inequalities for nonlinear
ill
posed problems.
To
illustrate the type
of
results that can be expected,
we
shall briefly outline here how the
logarithmic convexity technique leads to Holder continuous depen-
dence of solutions on the Cauchy data for the problem
vt
+
Av
=
G(t,
v)
in
8
x
[0,
T)
(4-1)
v
=
0
on
88
x
[O,T)
(4.2)
v(5,O)
=
f(5) 5
E
8.
(4.3)
The nonlinear function
G(t,v)
is assumed to satisfy
a
uniform Lips-
chitz condition. The stability question may be studied by assuming
that
01
and
v2
are
two solutions of (4.1)-(4.3) corresponding to the
initial data
f1
and
f2,
respectively. Let us define the functional
for
t
E
[0,
T)
and
a
positive constant
p.
A
judicious use of inequalities
yields the second order differential inequality
44"
-
(4'y
2
-%d2
-
K244'
(4.5)
for explicit, nonnegative constants
~1
and KZ. The change of variable
=
e-'(2'
transforms (4.5) into
We thus
see that 1n4(t)
t
zt
is
a
convex function of
e-'(Zt.
Integra-
tion of (4.6) by means of Jensen's inequality results in
+(t)
I
C[4(0)]'-s(')[4(T)]s(t),
0
5
t
I
T
(4.7)
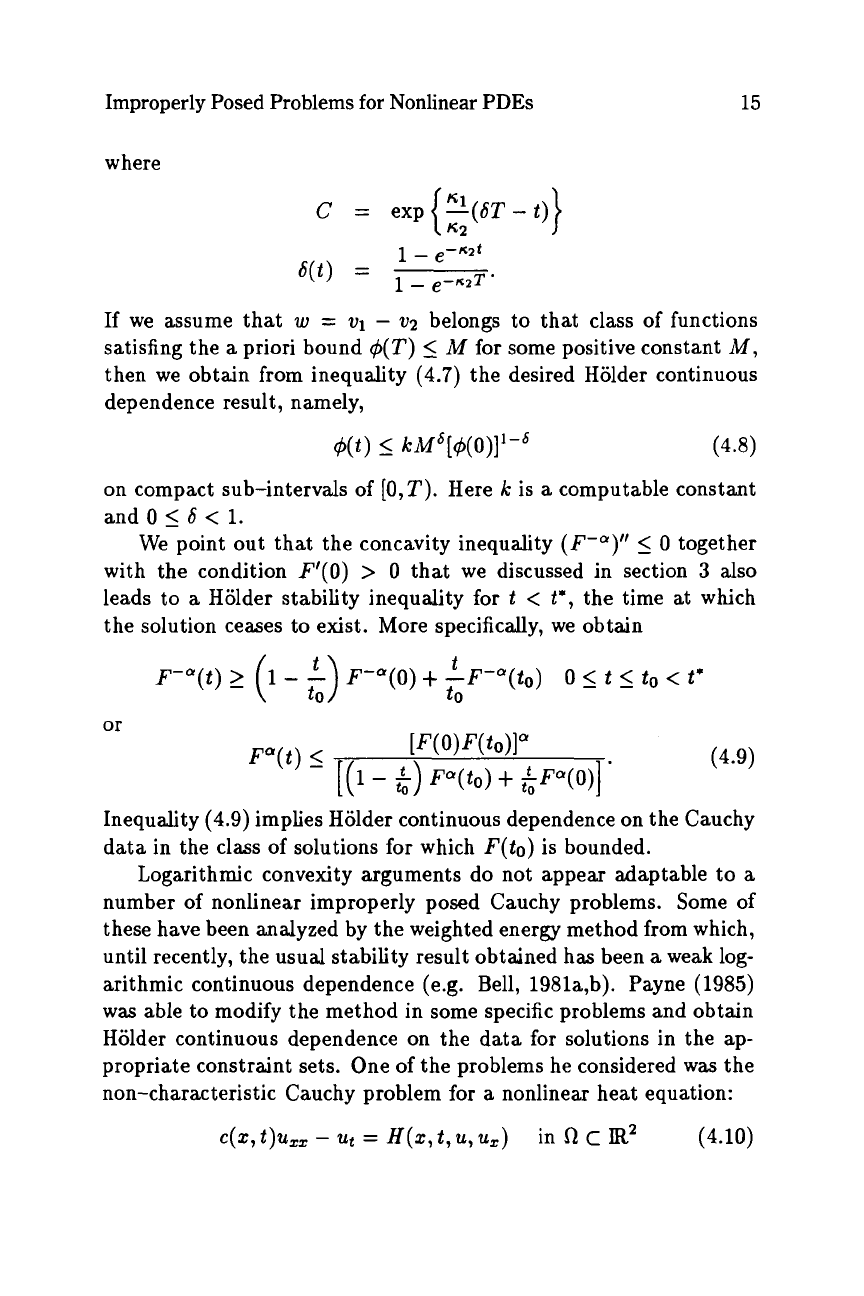
Improperly Posed Problems for Nonlinear PDEs
where
15
1
-
e-Kzt
1
-
e-KZT.
b(t)
=
If we assume that
20
=
81
-
212
belongs to that class of functions
satisfing the
a
priori bound
+(T)
5
M
for some positive constant
M,
then we obtain from inequality (4.7) the desired Holder continuous
dependence result, namely,
4(t)
I
kMs[4(0)1'-s
(4.8)
on compact sub-intervals of
[O,T).
Here
k
is
a
computable constant
and
0
5
6
<
1.
We point out that the concavity inequality
(F-")'I
<
0
together
with the condition
F'(0)
>
0
that we discussed in section
3
also
leads to
a
Holder stability inequality for
t
<
t',
the time at which
the solution ceases to exist. More specifically, we obtain
t
to
F-"(t)
2
(1
-
;-
F-yO)
i-
-F-O(to)
0
5
t
5
to
<
t'
or
Inequality (4.9) implies Holder continuous dependence on the Cauchy
data in the class of solutions for which
F(t0)
is bounded.
Logarithmic convexity arguments do not appear adaptable to
a
number of nonlinear improperly posed Cauchy problems. Some of
these have been analyzed by the weighted energy method from which,
until recently, the usual stability result obtained has been
a
weak log-
arithmic continuous dependence (e.g. Bell, 1981a,b). Payne (1985)
was
able to modify the method in some specific problems and obtain
Holder continuous dependence on the data for solutions in the ap-
propriate constraint sets. One
of
the problems he considered was the
non-characteristic Cauchy problem
for
a
nonlinear heat equation:
c(z,
t)u,
-
ut
=
~(z,
t,
u,
u,)
in
Q
c
IR'
(4.10)

16
Karen
A.
Ames
U(0,t)
=
f(t)
on
r
(4.11)
u,(o,~)
=
g(t)
on
r.
(4.12)
Here
R
is an open region in the first quadrant of the
(2,
t)
plane with
a
part
I'
of
its boundary lying on
a
segment
0
5
t
5
T
of the
t-axis.
The nonlinear function
EI
is assumed to be Lipschitz in its last two
arguments and the coefficient
c(x,
t)
is continuous and positive for
all
(x,t)
E
R.
Payne
(1985)
derived
C2
Holder continuous dependence
estimates for classical solutions that are suitably constrained.
More recently, Payne and Straughan (1990a) adapted
a
weighted
energy argument to study
a
one-space dimension problem for
a
piece
of cold ice, given data on only part of its boundary. They considered
the non-standard problem
-
au
=
-
a
{
(1
+
€121
+
E2U2)@}
x
>
0,
t
E
(-T,
T)
at
ax
OX
(4.13)
where
€2
>
ici
and obtained pointwise and
C2
Holder continuous
dependence inequalities on the Cauchy data.
As
they point out,
such estimates play an important role in the numerical solution
of
these problems.
4.2
Continuous Dependence on Geometry
One source of error that has not received much attention even with
regard to
well
posed problems is that attributable to inaccuracies in
describing the geometry
of
the problem. Such errors include both
errors in characterizing the geometry of the physical domain and in
the initial time geometry. These latter errors arise since initial data
is
typically not measured at precisely the same time.
The first results
on
the question of continuous dependence on
spatial geometry for ill posed problems were obtained by Crooke and
Payne
(1984).
These investigators were successful in stabilizing
so-
lutions of the initial-boundary value problem for the backward heat

Improperly Posed Problems for Nonlinear PDEs
17
equation with Dirichlet data under perturbations of the spatial do-
main. Persens (1986) extended the analysis to the case in which the
domains vary with time and to the analogous problem with Neu-
mann boundary data. He also studied the Dirichlet initial-boundary
value problem of linear elastodynamics with indefinite strain energy
under perturbations of the spatial geometry and the Cauchy prob-
lem for the Poisson equation under variations in the Cauchy surface.
Extension of the results
of
Crooke and Payne (1984) to the exterior
problem has recently been accomplished by Payne and
S
traughan (to
appear).
All
of
the aforementioned studies deal with ill posed prob-
lems
for
linear equations and essentially comprise the aggregate
of
known results. Despite the fact that the equations involved in these
investigations are linear, we again emphasize that the stabilization
of solutions
to
ill
posed problems against errors in spatial geometry
can occur provided we restrict the class of admissible solutions. Such
restrictions effectively transform these linear problems into nonlinear
problems.
The first treatment of errors in the initial time geometry for
an
ill posed problem appeared in the work
of
Knops and Payne (1969)
who investigated this question in the context
of
linear elastodynamics
and then
later
improved their original continuous dependence results
(see Knops and Payne, 1988). Additional studies of continuous de-
pendence
on
initial time geometry can be found in Song (1988)
and
more recently in Payne and Straughan (1990b) whose analysis for
the heat equation on an exterior region is adaptable to several other
parabolic systems.
We refer the reader to the surveys of Payne (1987a,b; 1989) for
a
more complete discussion of the current state of research on con-
tinuous dependence on both spatial and initial time geometry.
4.3
Continuous Dependence on Modeling
While the task of stabilizing ill posed problems under perturbations
of the geometry is difficult, it is not
as
formidable
as
that of regulariz-
ing against errors made in formulating the mathematical model, e.g.
errors made in treating
a
fluid
as
a
continuum, in assuming inexact
physical laws,
or
in approximating the model equation. These latter

18
Karen
A.
Ames
errors are more serious primarily because it is impossible to charac-
terize them precisely. As Bennett
(1986)
illustrated, constraints that
stabilize
a
problem against other sources
of
error may
fail
to
stabilize
it against modeling errors. Consequently, predictions for the phys-
ical process that are based on the improperly posed mathematical
problem may not be reliable unless they can be verified by actual
experiments.
Perhaps the first attempt at some kind
of
continuous dependence
on modeling investigation
for
ill posed problems
was
made by Payne
and Sather
(1967)
who compared the solution of
a
backward heat
conduction problem with that of
a
perturbed Cauchy problem for
an elliptic equation. Adelson
(1973, 1974)
later considered
a
class of
problems in which both the perturbed and unperturbed problems are
Cauchy problems
for
quasilinear elliptic equations. He was interested
in the following system:
in
R
c
IR"
~6Lv
+
v
=
u
Lu
=
F(x,
€,
u,
v)
(4.14)
u,
v,
grad
u,
grad
v
prescribed on
I'.
(4.15)
Here
c
is
a
smd positive parameter,
6
is
a
constant, L is
a
strongly
elliptic operator,
F
is assumed to be Lipschitz in its last three
ar-
guments, and
I'
is
a
portion of the boundary
of
R.
Adelson
(1973,
1974)
asked several questions about
(4.14)-(4.15)
including
i)
can we find
a
reasonable approximation solution of this sytem
in some neighborhood
0,
of
I'
by appropriately constraining
u
and/or
v?
ii)
if
is "very small", can we obtain
a
good enough approxima-
tion by setting
c
=
0
and approximating the solution w
of
the
simpler Cauchy problem
Lw
=
F(x,O,
w,
w)
in
R
(4.16)
w,grad
w
prescribed on
I?,
assuming the data of w and
v
are close?
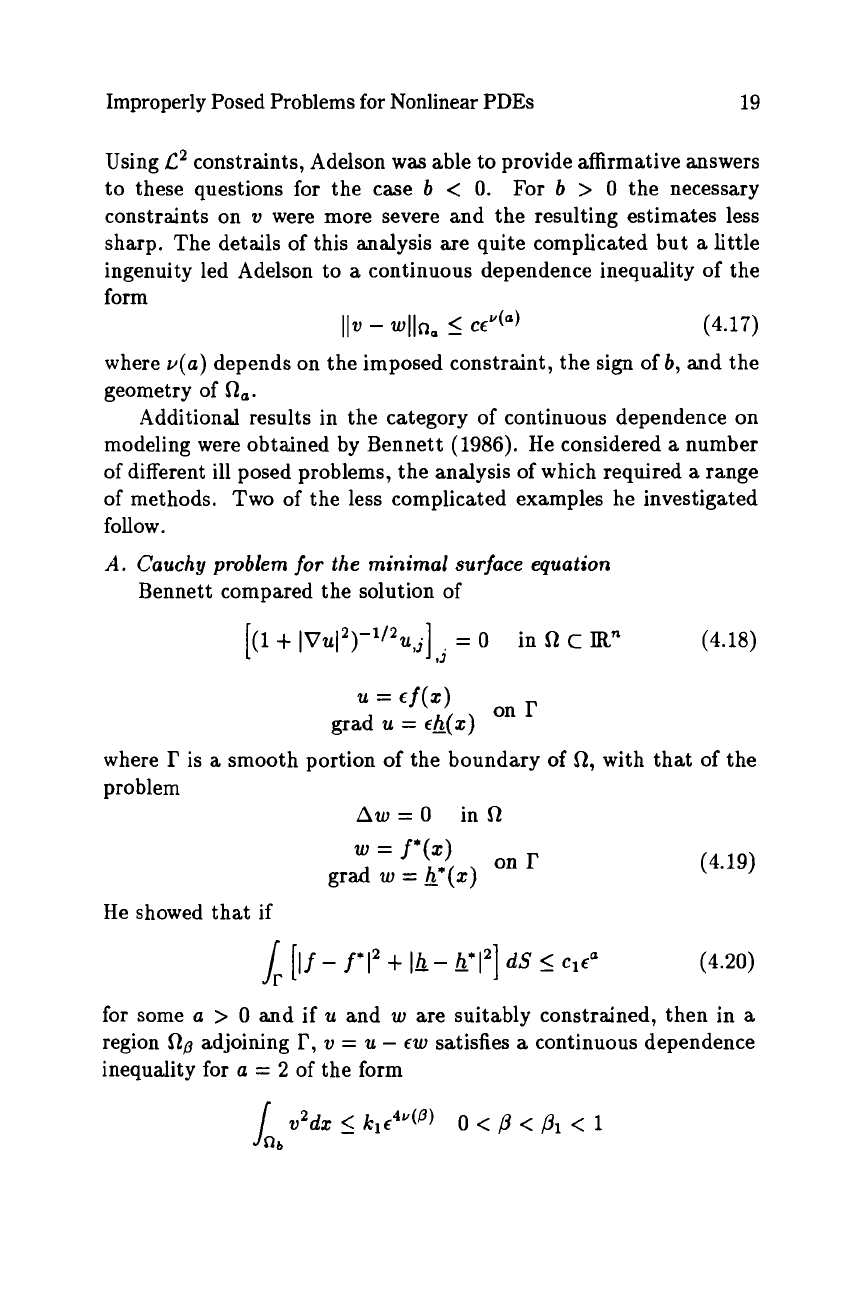
Improperly Posed Problems for Nonlinear PDEs
19
Using
C2
constraints, Adelson was able to provide affirmative answers
to
these questions for the case
b
<
0.
For
b
>
0
the necessary
constraints on
ZI
were more severe and the resulting estimates less
sharp. The details of this analysis are quite complicated but
a
little
ingenuity led Adelson to
a
continuous dependence inequality of the
form
11.
-
wlln,
5
c8+)
(4.17)
where
.(a)
depends on the imposed constraint, the sign of
b,
and the
geometry of
0,.
Additional results in the category of continuous dependence on
modeling were obtained by Bennett
(1986).
He considered
a
number
of different ill posed problems, the analysis of which required
a
range
of methods. Two of the less complicated examples he investigated
follow.
A.
Cauchy problem
for
the minimal surface equation
Bennett compared the solution of
[(I
+
1vu12)-1/2u,j]
,
=
o
in
R
c
IR~
(4.18)
13
u=ef(z)
on
r
grad
u
=
&(z)
where
r
is
a
smooth portion of the boundary
of
52,
with that of the
problem
Aw=O
in52
(4.19)
He showed that if
J,
[If
-
f*I2
+
lh-
YI2]
dS
5
Cleo
(4.20)
for
some
a
>
0
and if
u
and
w
are suitably constrained, then in
a
region
Rp
adjoining
r,
ZI
=
u
-
€20
satisfies
a
continuous dependence
inequality for
a
=
2
of the form
