Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.

This page intentionally left blank
Improperly
Posed
Problems
for
Nonlinear Partial
Differential Equations
Karen
A.
Ames
Department
of
Mathematical Sciences
University
of
Alabama
in Huntsville
Huntsville,
AL
35899
Dedicated
to
Lawrence
E.
Payne.
1
Introduction
The study of improperly posed problems has received considerable
recent attention in response to the realization that
a
number of phys-
ical situations lead to these kinds of mathematical models. Many
classes of improperly posed problems include nonlinear partial dif-
ferential equations. In this Chapter, we review some of the questions
confronted in the investigation of
a
particular class of such problems,
namely ill-posed Cauchy problems.
A
boundary
or
initial value problem for
a
partial differential equa-
tion
is
understood to be well posed in the sense of Hadamard if it
possesses
a
unique solution that depends continuously on the pre-
scribed data. This definition is made precise by indicating the space
to which the solution must belong and the measure in which continu-
ous
dependence
is
desired.
A
problem is called improperly posed
(or
ill-posed) if it fails to have
a
global solution,
a
unique solution,
or
a solution that depends continuously on the data. We shall discuss
the questions of uniqueness, existence, and stabilization of solutions
to ill-posed problems for nonlinear partial differential equations in
the subsequent sections.
Although we intend to focus on improperly posed Cauchy prob-
lems for nonlinear equations,
a
few comments about these kinds of
Nonlinear Equations
in
the Applied Sciences
1
Copyright
0
1992
by
Academic Press, Inc.
AU
rights
of
reproduction
in
any
form
reserved.
ISBN 0-12-056752-0

2
Karen A. Ames
ill-posed problems for linear equations should be included here. A
number of interesting challenges arise in the study of such problems
despite the “cloak” of linearity. Mathematicians now know that some
linear partial differential equations without singular points have no
nontrivial solutions, e.g. the classical construction of Lewy
(1957).
In addition, the literature contains examples that belie the hypothe-
sis that ill-posed Cauchy problems for linear equations have
at
most
one solution. While it is typical that solutions (if they exist) of such
problems are unique, examples of nonunique continuation for linear
elliptic and parabolic equations have been constructed by Plis
(1960,
1963)
and Miller
(1973, 1974).
Even in those cases where unique
solutions exist, they may not depend continuously on
all
of the data.
In attempting to derive stability inequalities for these linear prob-
lems, we are faced with the difficulty that the spaces in which we
are guaranteed continuous data dependence are nonlinear. Conse-
quently, we again encounter the obstacles inherent in the study of
nonlinear problems.
Recently, considerable progress has been made in the investiga-
tion of improperly posed problems for partial differential equations.
We refer the reader to Payne’s monograph
(1975)
as
a
primary source
on various topics in this field
as
well
as
substantial bibliography
of
the work done prior to
1975.
In the last twenty years, research on ill-
posed problems has intensified and moved in
so
many diverse direc-
tions that it would be virtually impossible to give
a
comprehensive,
up-to-date account of all the work that has appeared on even the
particular class
of
problems treated here. It is no surprise then that
the few existing books on improperly posed problems deal with spe-
cific classes of these problems. In addition to Payne’s volume, there
are contributions from the Russian researchers Lavrentiev
(1967),
Tikhonov and Arsenin
(1977),
Morozov
(1984),
and Lavrentiev, Ro-
manov, and Sisatskii
(1986).
We also mention the book by Latths
and Lions
(1969)
that describes
a
regularization method for approx-
imating solutions to improperly posed problems. Collections of pa-
pers or research notes in this area include
Symposium
on
Non-
Well-
Posed Problems and Logarithmic Convexity
(edited by
R.
J.
Knops)
in
1973,
Improperly Posed Boundary Value Problems
(Carasso and

Improperly Posed Problems
for
Nonlinear PDEs
3
Stone, 1975),
Instability, Nonexistence and Weighted Energy Meth-
ods
in Fluid Dynamics and Related Theories
(Straughan, 1982), and
Inverse and Ill-Posed Pmblems
(edited by Engl and Groetsch, 1987).
Because many ill-posed problems for partial differential equations
have not usually yielded to standard methods
of
analysis,
a
variety
of techniques have been developed in order to study these problems.
We
will
focus on three of these methods since they have proven to be
useful in treating nonlinear problems, namely logarithmic convexity,
weighted energy, and concavity. In Section 3 we shall make
a
few
remarks concerning the quasireversibility method when we address
the question of existence
of
solutions.
The first applications of logarithmic convexity arguments to im-
properly posed problems for partial differential equations have been
attributed to Pucci (1955), John (1955,1960), and Lavrentiev (1956).
A
detailed treatment
of
this method can be found in the compre-
hensive work
of
Agmon (1966). Basically, this procedure employs
second order differential inequalities to investigate the properties
of
solutions. Solution properties can
also
be obtained via the weighted
energy method. The development of this technique, which was uti-
lized by
M.
H.
Protter early in the 1950s,
as
well
as
examples
of
its applications in fluid dynamics are covered
in
the monograph
of
Straughan (1982). Weighted energy arguments have more recently
been employed to study ill-posed problems
for
nonlinear equations by
Bell (1981a,b), Bennett (1986), Lavrentiev et.
al.
(1986), Payne and
Straughan (1990a) and Straughan (1983) among others. Modifica-
tions of the original ideas of Protter and his co-workers (see Lees and
Protter, 1961; Murray, 1972; Murray and Protter, 1973) has gener-
ated improved results in vaTous problems. We cite the work
of
Payne
(1985) and Ames, Levine and Payne (1987). Finally, the concavity
method has proved to be useful in establishing nonexistence theo-
rems for problems in such areas
as
nonlinear elasticity and nonlinear
continuum mechanics. Extensive use
of
this technique has been made
by Hills and Knops (1974), Knops, Levine, and Payne (1974), Knops
and Straughan (1976), Levine (1973; 1974a,b,c), Levine and Payne
(1974a,b; 1976) and Straughan (1975b, 1976). We refer the reader to
Payne (1975) for
a
detailed description of these three methods. In the
4
Karen
A.
Ames
following three sections, we
shall
illustrate how such techniques can
be exploited to study the topics
of
uniqueness, existence, and con-
tinuous data dependence of solutions to improperly posed Cauchy
problems for nonlinear partial differential equations. We emphasize
that this is not intended to be an exhaustive treatment
of
the subject
but is meant to give some indication
of
the difficulties encountered in
our attempts to understand what constitutes
a
well-posed problem
for
a
differential equation.
2
Uniqueness
Mathematicians who investigated ill-posed Cauchy problems for par-
tial differential equations before
1950
dealt primarily with questions
of uniqueness for linear equations since,
as
mentioned earlier, such
problems typically have at most one solution. The literature of the
1950’s
and early
1960’s
is rich with uniqueness studies, some of which
involve nonlinear equations. Serrin
(1963),
for example, established
uniqueness for classical solutions of the Navier-Stokes equations on
a bounded spatial domain backward in time using weighted energy
arguments based on work by
Lees
and Protter
(1961).
Knops and
Payne
(1968)
gave an alternative proof of backward uniqueness
for
the same problem when they addressed the question of continuous
dependence on the final data.
A
number of uniqueness studies for
various problems associated with the Navier-Stokes equations back-
ward in time have appeared since then (cf. Straughan
(1982),
Payne
and Straughan
(199Oc
and references therein)).
Uniqueness ques-
tions for ill-posed problems that arise in nonlinear elasticity have
also received recent attention (Knops and Payne,
1979, 1983).
In this section we present
a
nonlinear example of nonuniqueness.
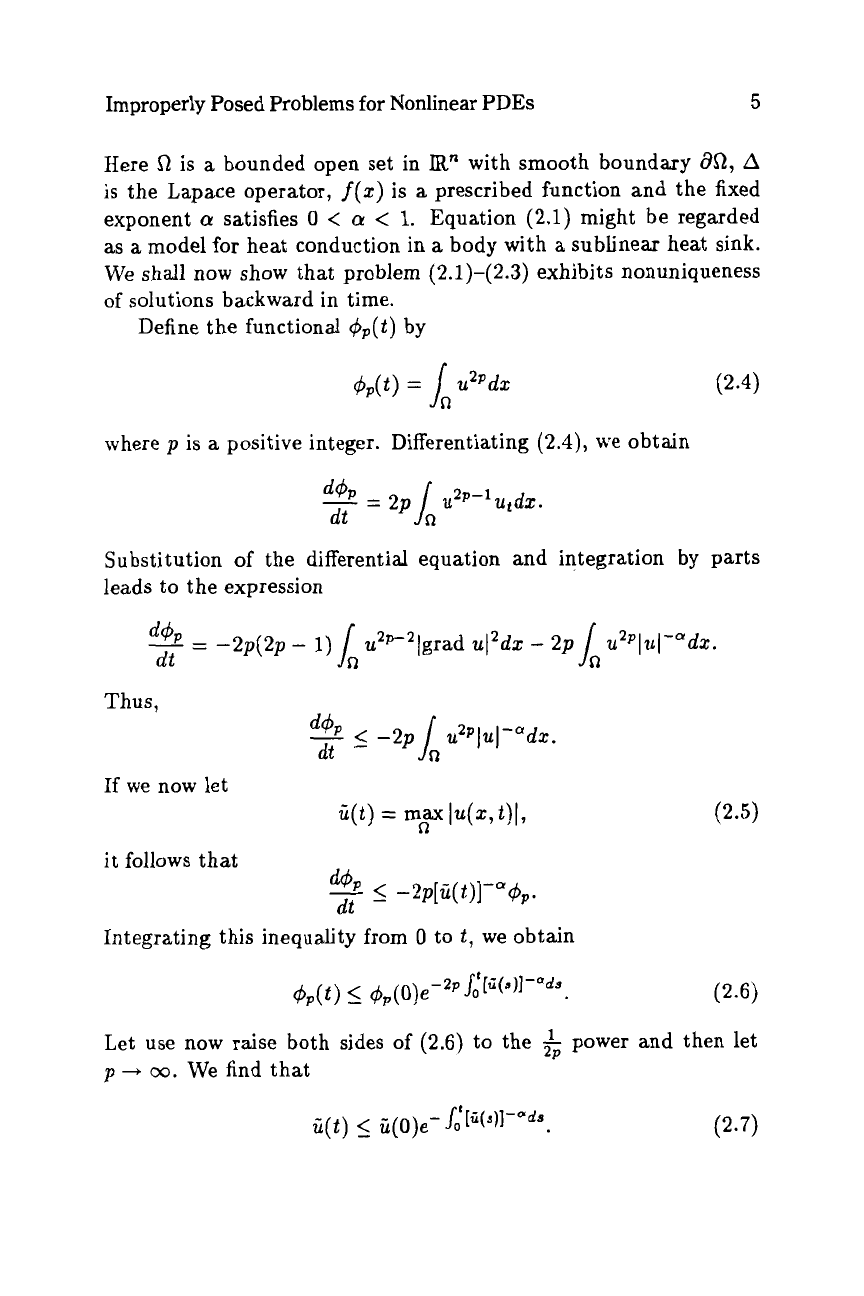
The initial-boundary value problem we consider is the following:
u
=
o
on
i3R
x
[O,co)
(2.2)
U(2,O)
=
f(z)
z
E
R.
(2-3)
Improperly Posed Problems for Nonlinear
PDEs
5
Here
R
is
a
bounded open set in
IR,"
with smooth boundary
80,
A
is
the Lapace operator,
f(x)
is
a
prescribed function and the fixed
exponent
Q
satisfies
0
<
Q
<
1.
Equation
(2.1)
might be regarded
as
a
model
for
heat conduction in
a
body with
a
sublinear heat sink.
We shall now show that problem
(2.1)-(2.3)
exhibits nonuniqueness
of solutions backward in time.
Define the functional
+,(t)
by
$(t)
=
J,
UZPdX
where
p
is
a
positive integer. Differentiating
(2.4),
we obtain
Substitution
of
the differential equation and integration
by
parts
leads to the expression
-
d+P
=
-2p(2p
-
1)/
u2P-21grad
u12dx
-
2p/n
u2Plul(-Qdx.
dt
n
Thus,
If we now let
G(t)
=
14x7 41,
it follows that
-
d4P
5
-2p[qt)]-a+p.
dt
Integrating this inequality
from
0
to
t,
we obtain
Let use now raise both sides
of
(2.6)
to the
$
power and then let
p
-,
00.
We find that

6
Karen
A.
Ames
The assumption that
ii(t)
>
0
allows use to write
(2.7)
in the form
1
-a
J,"qs)]-%
<-
1
[ii(O)]"
-
[ii(i)]ae
and an integration from
0
to t results in the inequality
-a
J0t[7i(
s)]
-ads
1
<-
1-e
"
a
t
[ii(O)]"
-
a
Consequently, we conclude that
1
t
5
-[ii(0)Ia.
a
Observing that
(2.8)
cannot hold for all time, we see that if
a
solution
to
(2.1)-(2.3)
exists, it must decay to zero in
a
finite time
T
satisfying
the upper bound
1
T
5
-[ii(O)]".
a
IVe thus have an example for which we fail to have
a
unique solution
backward in time. Similar results (Payne,
1975)
can be obtained for
the Cauchy problem for nonlinear equations of the form
where the point function
f
satisfies the condition
for
0
<
cr
<
1
and
a
constant
c
>
0.
\.Ve see that
a
loss of uniqueness may be one price we have to
pay
for
introducing nonlinear terms into
a
mathematical model. We
note here that the uniqueness studies appearing in the literature on
ill-posed problems are frequently concerned with the determination
of
criteria necessary to ensure unique solutions to the problems un-
der consideration. These results are often consequences of unique
continuation theorems (cf. Payne,
1975).

Improperly Posed Problems for Nonlinear PDEs
7
3
Existence
We begin our discussion of existence by remarking that throughout
this chapter, the term “existence” refers
to
global existence. For some
of the problems considered here, local existence can be deduced from
the Cauchy- Kovalevski theorem.
The question of global existence of solutions to ill-posed problems
is difficult to treat with much generality. It is frequently the case that
very strong regularity and compatibility conditions must be imposed
on the data in order for
a
solution to exist. Such conditions cannot
always be guaranteed in practice because of the possibility of error
in data measurement. From
a
practical viewpoint, these problems
probably require
a
theorem asserting the existence of
a
solution that
satisfies an appropriate stabilizing condition and exhibits data that
are close in some measure to the given values.
Attempts to deal with the existence question have often followed
the strategy of modifying either the concept
of
a
solution
or
the
mathematical model. For many physical situations, it is sufficient
to define the “solution”
as
a
domain function that belongs to an
appropriate class and best approximates the prescribed data. Alter-
natively, changing the underlying model has led to the development
of techniques to generate approximate solutions, including Tikhonov
regularization (Tikhonov,
1963) and the quasi reversibility method
(Latths and Lions,
1969). The primary aim of these methods is the
numerical solution of classes of ill-posed initial-boundary value prob-
lems. Since existence theorems for such problems are generally not
available, approximate solutions may
be
as
close
as
we can come to
establishing the existence of
a
“solution.”
We remark that Lattks and Lions
(1969) formulate and briefly
discuss quasi reverisiblity methods for several nonlinear problems of
evolution, including the Navier-Stokes equations. They give
a
few
simple numerical examples but one surmises from their sketchy devel-
opment that nonlinearity further complicates the task of constructing
approximate solutions to ill-posed problems. One issue we encounter
in attempting to compute solutions of these problems (both linear
and nonlinear) is the question
of
stability. Not only do we need
to
derive stability estimates but we also need to develop numerical

8
Karen
A.
Arnes
methods that effectively incorporate the prescribed global bounds
usually required to stabilize ill-posed problems. We shall not pursue
these topics here but refer the reader to the work of Miller
(1973),
Showalter
(1974, 1975),
and Ewing
(1975).
We leave the problem of generating approximate solutions and
turn to the related topic of identifying those nonlinear equations
for which certain types
of
data are incompatible with global exis-
tence of solutions. Nonexistence results
for
initial-boundary value
problems
for
classes of nonlinear parabolic equations have been ob-
tained by Kaplan
(1963),
Fujita
(1966, 1970),
Levine and Payne
(1974),
Straughan
(1975b),
and more recently by Ames, Payne and
Straughan
(1989).
Operator equation analogues of such problems
have been treated by Levine
(1973)
and Tsutsumi
(1971, 1972),
among others. Similar studies for nonlinear hyperbolic equations
have been done by Sattinger
(1968),
Glassey
(1973, 1981),
Levine
(1974a,
b), Knops et.
al.
(1974)
and John
(1979).
The recent review
article by Levine
(1990)
collects
a
number of the available nonex-
istence results and provides an existence list of references on the
subject. These investigations have relied on
a
variety of methods to
establish global nonexistence theorems. We focus here
on
one par-
ticular method, the concavity method, in order to illustrate the type
of results that can be obtained.
Let us use
as
our model the problem
azU
at2
-
=
AU
-+
UP
in
Q
x
[o,T)
u
=
o
on
aR
x
[O,T)
(3.2)
u(x,
0)
=
f(x)
ut(z,
0)
=
g(z)
for
x
E
0
(3.3)
where
R
is
a
bounded domain in
R",
A
is the Laplace operator,
p
2
2
is
a
positive integer, and
T
is
understood to be either infinity
or
the limit of the existence interval. For any classical solution of
(3.1)-(3.3)
we define
a
functional

Improperly Posed Problems for Nonlinear PDEs
9
for nonnegative constants
k
and
to
to be chosen. The idea of the
concavity method is to show that
F-"
for some
a
>
0
is
a
concave
function oft.
If
we choose
k
and
to
so
that
F(0)
>
0,
it will follow
that
F(t)
>
0
for
all
t
in the existence interval and thus
F-"
will be
concave if it satisfies the inequality
FF"
-
(1
+
2
o
(3.5)
for
all
such
t.
We shd now show that
(3.4)
satisfies the inequality
(3.5).
Differentiation
of
(3.4)
leads to
F'(t)
=
ZJ,
uutdz
+
2k(t
+
to)
F"(t)
=
2/
u:dz
+
2
J,
uuttdz
+
Zk.
(3.6)
(3.7)
and then
n
Substituting the differential equation, we find that
FN(t)
=
4(1+a)ll~t(1~-2(1+2a)11~~1)~-2llgrad
u1I2+2
uP+ld2+2k.
If we multiply
(3.1)
by
ut
and integrate with respect to
t,
we obtain
(3.8)
J,
or
where
(3.10)
Let
us
next use
(3.9)
to eliminate the -2(1
+
2a)llutl12
term in ex-
pression
(3.8).
We thus have
1 1
E(O)
=
sllsl12
+
sllgrad
AI2*
F"(t)
=
4(1
+
a)ll~t11~
+
4allgrad
+
2/n
uP+ldz
-4(
1
+
2a)
J'
/
u,ddzd~
-4(
1
+
2a)E(O)
+
2k
on
(3.11)
