Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


40
W.
F.
Ames
Case
Cl:
pn
=
-413.
of
(1.21)
and
82
a
r;
=
~X(U
+
d)-
-
2
-
au
dX
Case
C2:
m
=
-4
a
a
rl,
r2,r3r;
of
(1.21)
and
r;
=
t(u
+
d)-
au
+
l2%
The group analysis has been extended to the equation
2111
=
[f(.,.).Z]Z (1.22)
by Torrisi and Valenti
(1985).
Suhubi and Bakkaloglu
(1991)
consider
the equation
Utt
=
[f(%
Ut,
74
2,
t)lz
(1.23)
for
the hyperbolic case,
(af/a~t)~
+4(af/aux)
>
0,
by means of the
methods of the exterior calculus.
Lastly, the semilinear equation
uxt
=
f(u) (1.24)
has been analyzed by Pucci and Salvatori
(1986).
If
8
d
a
r
=
xz
+T%
+
u-
aU
the authors find
X
=
X(z),
T
=
T(t),
U
=
au
+
b(2,t)
where
auf’(u)
+
bf’(u)
-
(u
-
X‘
-
T’)f(u)
-
b,t
=
0.
A
number
of
special cases are detailed but only that of
f(u)
=
(hu
+
IC)l-’,
s
#
0,
h
#
0
is given here. The symmetry generators are found
to be
a
r3
=
-
aa
a
rl
=
-x-+t-,
r
--
ax
at
-
at’
8X
r4
=
-2-+-t-+
u+-
.
(
3;
sa sa
2
ax
2
at
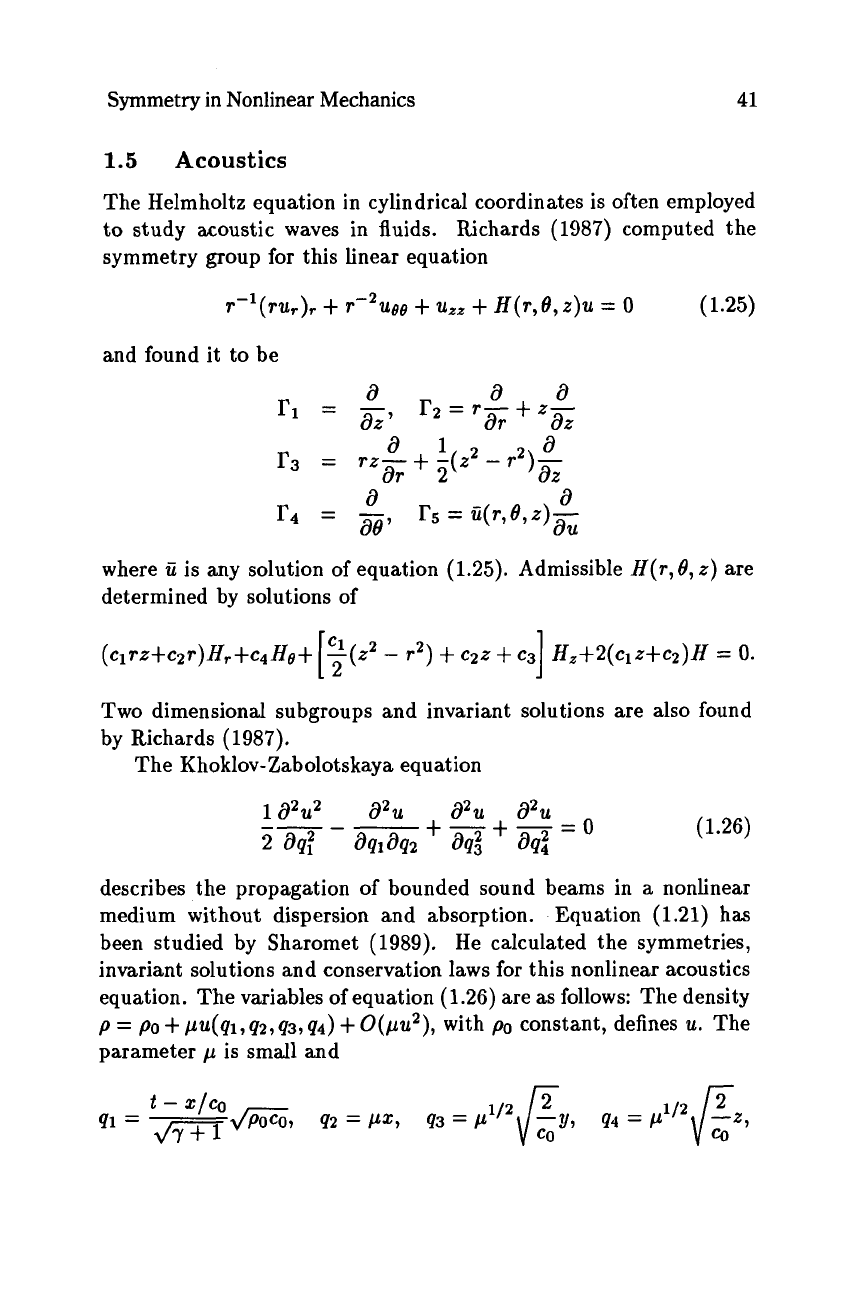
Symmetry in Nonlinear Mechanics
41
1.5
Acoustics
The Helmholtz equation in cylindrical coordinates is often employed
to
study acoustic waves in fluids.
Richards
(1987)
computed the
symmetry group for this linear equation
and found it to be
d
8
r2
=
T-
+
t-
d
dz
'
dr
dx
dT
2
at
rl
=
-
r3
=
TZ-+
8
-(z
12
-r
28
)-
where
.li
is any solution of equation
(1.25).
Admissible
H(T,
8,
t)
are
determined by solutions
of
Two dimensional subgroups and invariant soh tions are also found
by Richards
(1987).
The Khoklov-Zabolotskaya equation
describes the propagation of bounded sound beams in
a
nonlinear
medium without dispersion and absorption. Equation
(1.21)
has
been studied by Sharomet
(1989).
He calculated the symmetries,
invariant solutions and conservation laws
for
this nonlinear acoustics
equation. The variables of equation
(1.26)
are as follows: The density
p
=
Po
+
pU(ql,qz,
93,44)
+
O(pu2),
with
po
constant, defines
u.
The
parameter
p
is small and

42
W.
F.
Ames
where
co
is
a
reference sound speed in the medium and
7
is the
adiabitic index.
For
beams invariant under rotation of the
(43’94)
plane, equation
(1.26)
is replaced by
(1.27)
Equations
(1.26), (1.27)
and the two dimensional analog of
(1.26)
are
considered in the paper.
1@(u2)
6%
d2u
1
au
2
&I;
&Ild92
&l3
43
a93
+
2
+
--
=
0.
--_-
1.6
The symmetry groups for the classical linear equations have been
given in
a
variety of places (see e.g., Olver
(1986)
and Ibragimov
(1983),
Rogers and Ames
(1989)).
These Lie algebras are infinite
dimensional.
A
number of authors have noted that an
nth
order equation and
the (equivalent) first order systems of
n
equations may not have the
same symmetry group. The first detailed paper in the area was by
Bluman
and
Kumei
(1987).
They studied the invariance properties
of the second order hyperbolic equation
Some Comments on
Linear
Systems
and the corresponding system
Dt
=
u,,
ut
=
c
2
(+,.
(1.28)
(1.29)
In spite
of
the apparent equivalence of
a
single
PDE
and
a
cor-
responding system of
PDE’s
it does not necessarily follow that their
respective invariance groups of point transformation are the same.
It could happen that the group of point transformations leaving in-
variant the system is larger than that leaving invariant the single
equation; also the converse could be true. This is indeed the case for
the single equation
(1.28)
and the corresponding system
(1.29).
For
example if
c(z)
=
(Az+B)~,
then
(1.28)
is invariant under an infinite
Lie group of point transformations, whereas the Lie group of point
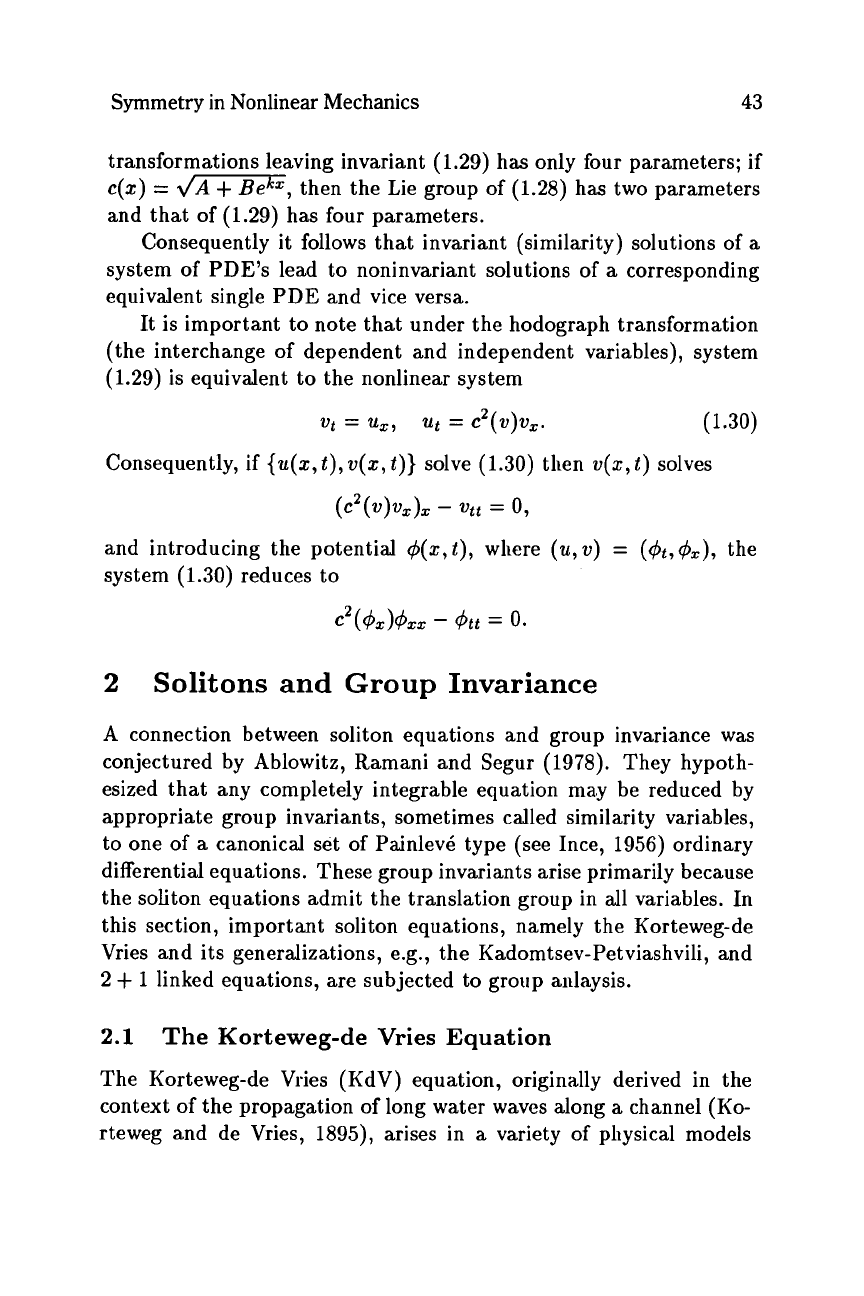
Symmetry in Nonlinear Mechanics
43
transformations leaving invariant
(1.29)
has only four parameters;
if
C(Z)
=
dw,
then the Lie group of
(1.28)
has two parameters
and that of
(1.29)
has four parameters.
Consequently
it
follows that invariant (similarity) solutions of
a
system
of
PDE’s lead
to
noninvariant solutions
of
a
corresponding
equivalent single PDE and vice versa.
It
is important
to
note that under the hodograph transformation
(the interchange
of
dependent and independent variables), system
(1.29)
is equivalent
to
the nonlinear system
vt
=
u,,
ut
=
c
2
(v)v,.
(1.30)
Consequently, if
{u(x,t),
v(z,
t)}
solve
(1.30)
then
v(x,
t)
solves
and introducing the potential
+(z,t),
where
(u,v)
=
(+t,+,),
the
system
(1.30)
reduces to
c2(+z)4zz
-
4tt
=
0.
2
Solitons and
Group
Invariance
A connection between soliton equations and group invaria.nce was
conjectured by Ablowitz, Ramani and Segur
(1978).
They hypoth-
esized that any completely integrable equation may be reduced by
appropriate group invariants, sometimes called similarity variables,
to one
of
a
canonical set
of
PainlevC type (see Ince,
1956)
ordinary
differential equations. These group invariants arise primarily because
the soliton equations admit the translation group in all variables. In
this section, important soliton equations, namely the Korteweg-de
Vries and its generalizations, e.g., the Kadomtsev-Petviashvili, and
2
+
1
linked equations, are subjected to group anlaysis.
2.1
The Korteweg-de Vries Equation
The Korteweg-de Vries (KdV) equation, originally derived in the
context
of
the propagation of long water waves along
a
channel (KO-
rteweg and de Vries,
1895),
arises in
a
variety of physical models

44
W.
F.
Ames
involving finite amplitude waves (Miles,
1981;
Newell,
1985;
Rogers
and Shadwick,
1982;
Rogers and Ames,
1989).
Indeed,
a
large class
of nonlinear evolution equations may be reduced to consideration of
this important canonical form (Su and Gardner,
1968
and Taniuti
and Wei,
1968).
The Kadomtev-Petviashvili (KP) equation is also
of considerable physical importance.
It arises in the study
of
long
gravity waves in
a
single layer
or
multilayered shallow fluids wherein
waves propagate predominantly in one direction with
a
small orthog-
onal perturbation (Kadomtsev and Petviashvili,
1970
and Satsuma
and Ablowitz,
1979).
The KdV equation
has been shown to be invariant under the four parameter class of Lie
transformations (Shen and Ames,
1974)
I
2’
=
X+€
-+ot+p
[T
t’
=
t
+
€[7t
+
S]
U’
=
u
+
~[(-27~/3)
+
a]
with generators of the associated Lie algebra given by
a
r2
=
-
at
(Translations in
x
and
t),
a
rl
=
-
ax
’
(2.3)
ad
I’3
=
t-
+
-
(Galilean “boost”),
ax
au
ia
a
20
3
ax
at
3
au
=
-x-
+
t-
-
-11-
(Scaling
or
dilation).
If
X
is
a
real number and
u
=
j(x,t)
is
a
solution of
(2.1),
then
from
rr,
so
is
u1
=
f(x
-
A,t).
From
r2,
so
is
212
=
f(x,t
-
A)
a
solution. From
r3
a
solution is also found to be
‘113
=
f(x
-
Xt,t),
while
r4
gives rise to the solution 214
=
e-2X/3f(e-X/3x,e-Xt).
The
study of many soliton equations is facilitated by combining and
r2
as
I’
=
Arl
+
I’2
with solutions of the form
u
=
j(x
-
At).
The
quantity
w
=
x
-
At
is the group invariant of the subgroup formed by

Symmetry in Nonlinear Mechanics
45
rl
and
r2.
Upon substituting
u
=
f(z
-
At)
into
(2.1)
there results
the ordinary differential equation
f"'
+
f
f'
-
x
f'
=
0.
(2.4)
This constitutes the so-called symmetry reduction of
(2.1)
under the
translation subgroup. Zabusky
(1967)
based his study of solitons on
equation
(2.4)
without knowledge of the symmetry group.
Although the list of symmetries for the KdV equation is small
it turns out that group invariance plays
a
key role in the construc-
tion of multi-soliton solutions via Backlund transformations. Thus,
in particular the celebrated auto-Backlund transformation (Rogers
and Shadwick,
1982)
for the KdV equation in fact represents
a
con-
jugation of
a
Bianchi transformation (Eisenhart,
1972)
and the Lie
symmetry corresponding to Galilean invariance (Dodd et al.,
1982).
Such combinations have also been described for the cubic Schrodinger
equation by Stendel
(1980)
and for
a
variety
of
nonlinear integral
equations by Huang
(1982, 1983).
For
the general approach to
the conjugation of Lie symmetries with Bianchi- type symmetries see
Rogers and Shadwick
(1982).
2.2
The Kadomt sev-Petviashvili Equation
One two dimensional version of the KdV equation
is
the
I<-P
equation
3
1 3
(ut
t
pux
t
-uxxx
4
)
X
t
;Uyy
=
0.
The invariance group for this equation was first obtained by Ames
and Nucci
(1985)
manually and later calculated by David et
al.
(1986)
using MACSYMA and by Schwarz
(1988)
using REDUCE-
SPDE.
A
versatile
intemtive
computer algebra package called
NUSY
(Nucci,
1990)
computed Lie symmetries by the classical and non-
classical methods. The generators for the
KP
equation involve three
arbitrary functions
f(t),
g(t)
and
h(t)
in
C"(IR)
and are given by
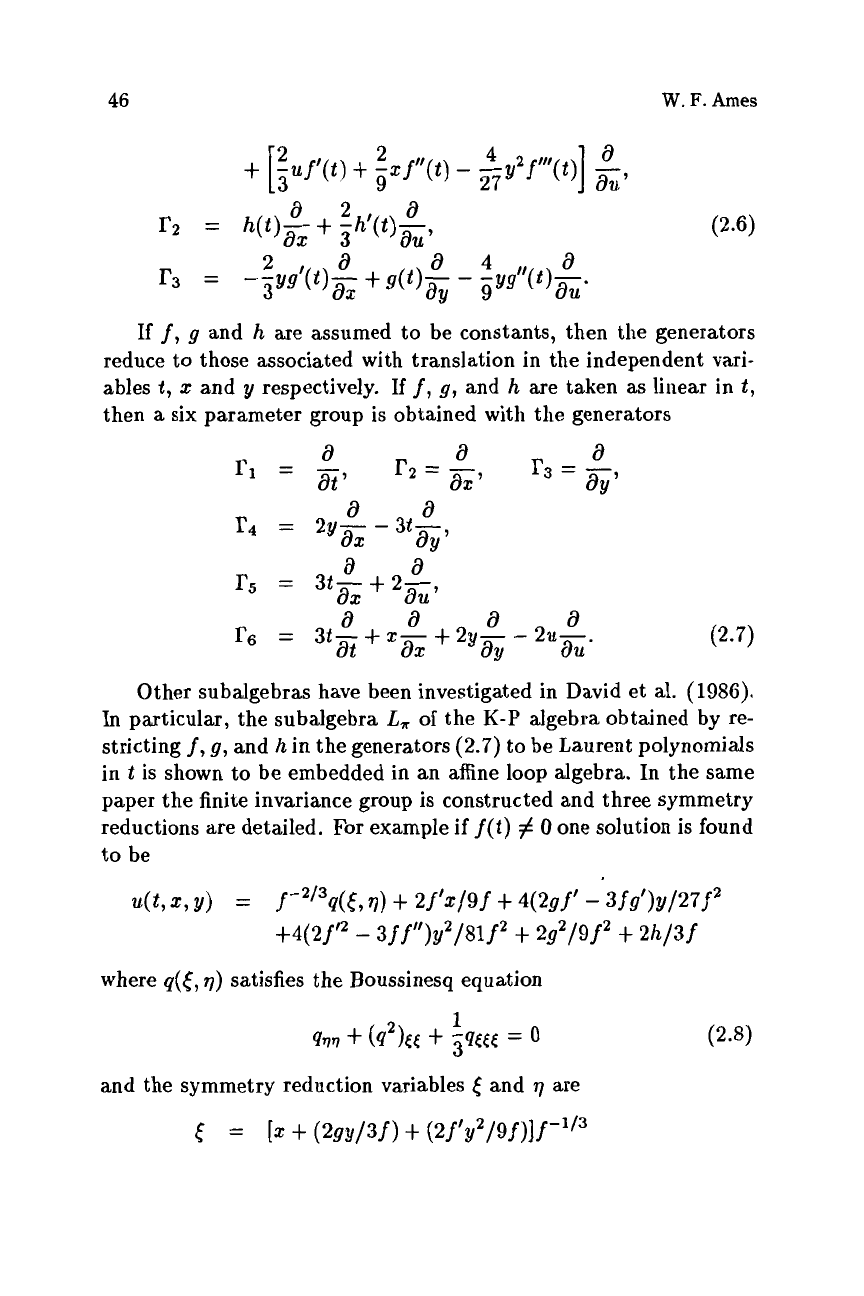
46
W.
F.
Ames
If
f,
g
and
h
are assumed to be constants, then the generators
reduce to those associated with translation in the independent vari-
ables
t,
x
and
y
respectively. If
f,
g,
and
h
are taken as linear in
t,
then
a
six parameter group is obtained with the generators
Other subalgebras have been investigated in David et al.
(1986).
In particular, the subalgebra
L,
of the
K-P
algebra obtained by re-
stricting
f,
g,
and
h
in the generators
(2.7)
to
be Laurent polynomials
in
t
is shown to be embedded in an affine loop algebra. In the same
paper the finite invariance group is constructed and three symmetry
reductions are detailed.
For
example if
f(t)
#
0 one solution is found
to be
210,
x,
Y)
=
f
-2/39(t,
17)
+
2f’x/9f
+
4(2gf‘ -’3fg‘)y/27f
+4(2
f
-
3
f
f“)y2/81
f
t
2g2/9
f2
+
2h/3
f
where
q(t,
17)
satisfies the Boussinesq equation
and the symmetry reduction variables
(
and
7
are
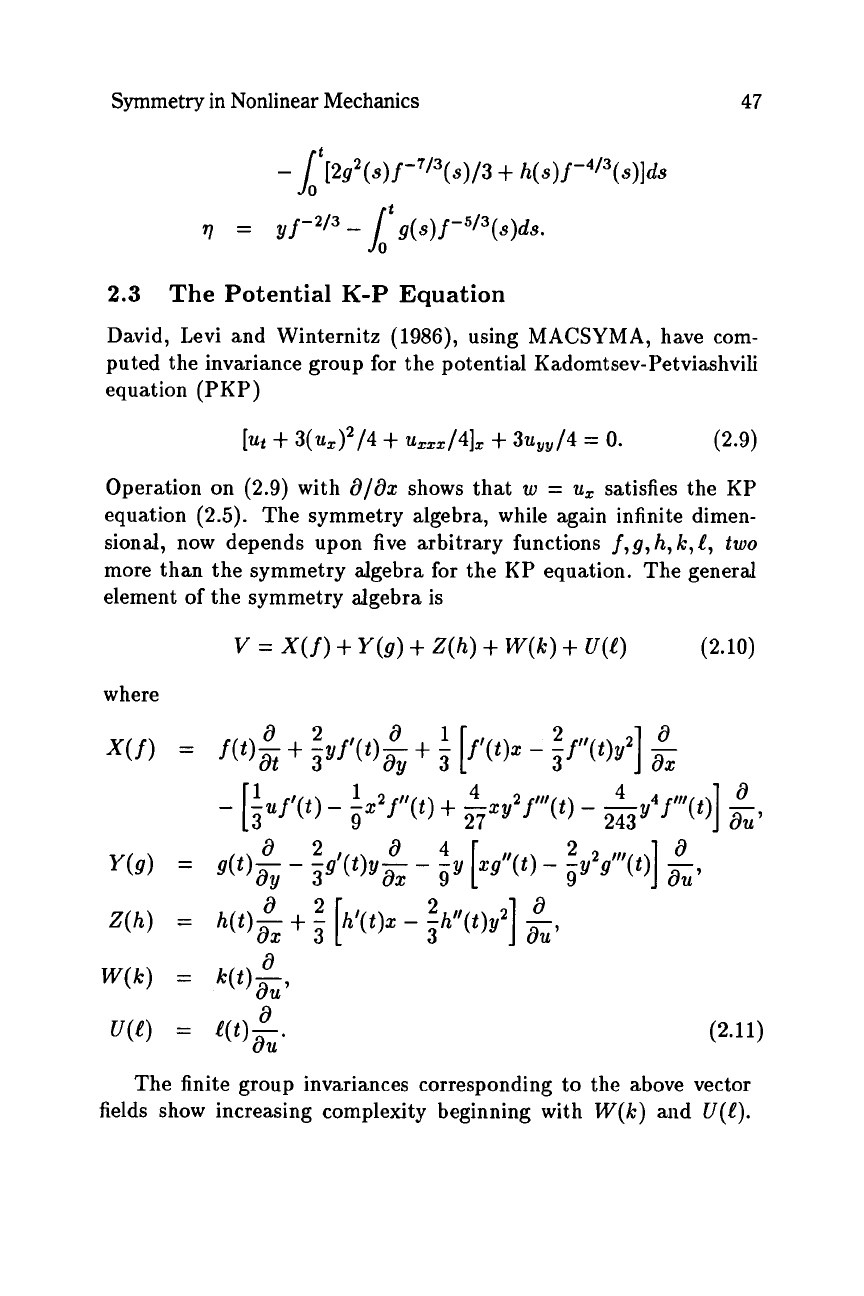
Symmetry in Nonlinear Mechanics
47
2.3
The
Potential K-P
Equation
David, Levi and Winternitz
(1986),
using MACSYMA, have com-
puted the invariance group for the potential Kadomtsev-Petviashvili
equation (PKP)
[ut
+
3(~2)~/4
+
~,,/4],
+
3uYy/4
=
0.
(2.9)
Operation on
(2.9)
with
d/Ox
shows that
w
=
u,
satisfies the
KP
equation
(2.5).
The symmetry algebra, while again infinite dimen-
sional, now depends upon five arbitrary functions
f,g,
h,
k,
t,
two
more than the symmetry algebra for the
KP
equation. The general
element of the symmetry algebra is
v
=
X(f)
+
Y(9)
+
Z(h)
+
W(k)
+
U(t)
(2.10)
where
a
W(k)
=
k(t)-,
au
a
U(t)
=
t(t)-.
dU
(2.11)
The finite group invariances corresponding to the above vector
fields show increasing complexity beginning with
W(k)
and
U(t).

48
W.
F.
Ames
They correspond to the invariance of the
PKP
equation under the
transformation
ii
=
u
+
x(e(t)
+
k(t)y),
2=
2,
fj=
y,
t
=
t,
I
-
whence, if
u(z,y,t)
is
a
solution of the
PKP
equation, then
so
also
is
G(Z,F,T)
=
u(Z,5,Tj
+
X[L(Tj
+
The group invariances corresponding to
Z(h)
and
Y(g)
show, in
turn, that if
u(z,
y,
t)
is
a
solution
of
the
PKP
equation, then
so
also
are
-
2
4
1
3 3
3
G(5,
5,
t)
=
u[Z
-
Ah@),
c,
8
+
-Ah%
-
-
-X2hh1,
and
3
1
G(Z,
5,
Tj
=
11
2
+
-xg‘g
-
-x2gg1,
5
-
xg,
t
[:
3
with
h
=
h(t)
=
h(q,
g
=
g(t)
=
g(Tj,
Finally, corresponding to the vector field
X(f),
it is found that
if
u(2,
y,
t)
is
a
solution of the
PKP
equation, then
so
also
is
1-1-2
4
G(Z,c,g
=
e’/3u(2,y,t)
-
-h
2
+
-(3p
-
?1I2)2G2
9
81
-
-(g?1lt1-gh
4
-1-11
h
+“h‘),
2187

Symmetry in Nonlinear Mechanics
49
where
and
The specializations h
=
1
and
h
=
t
in equations
(2.11)
corre-
spond, in turn, to translational invariance and Galilean invariance in
the
x
direction. The specializations
g
=
1
and
g
=
t
in equations
(2.11)
give translational invariance in the
y
direction and invariance
under quasi-rotations.
For
f(t)
=
1
or
f(t)
=
t
in equations
(2.11),
we obtain invariance under time translation
or
space-time scaling,
respectively.
2.4
Lastly,
a
group analysis has been performed by Schwarz
(1988)
for
the potential modified Kadomtsev-Petviashvili equation
The Potential Modified
KP
Equations
obtained by setting
v
=
u,
in the modified Kadomtsev-Petviashvili
equation
X
X
vt
=
v,,,
-
6v2v,
t
3
1
vyydx
-
6vx
1
vydx
The latter equation is linked to the K-P equation by the Miura type
transformation (Rogers and Shadwick
(1982))
w
=
v,
+
v
t
vydx.
2.1
