Amano R.S., Sunden B. (Eds.) Computational Fluid Dynamics and Heat Transfer: Emerging Topics
Подождите немного. Документ загружается.

Sunden CH008.tex 10/9/2010 15: 19 Page 316
316 Computational Fluid Dynamics and Heat Transfer
into theNLEDHM, theNLEDHM successfullypredicts turbulentbuoyant flows as
mentioned in Hattori et al. [24].
α
t
jk
=
C
∗
θ1
f
RT
u
i
u
j
A
ik
τ
m
(78)
f
RT
= 1 +
1
2
τ
2
m
[C
2
θ2
(W
2
− S
2
) +(C
2
θ3
− C
2
θ2
)W
2
] (79)
whereC
∗
θ1
,C
θ2
,andC
θ3
aremodelconstantsandτ
m
isthecharacteristictime-scale.
Inintroducingthe dimensionlessReynoldsstress tensor A
ij
, α
t
jk
(∂ /∂x
k
)ofthe
streamwise turbulent heat flux,
uθ (j =1), is given as follows:
α
t
xy
=
C
∗
θ1
f
RT
uv
k
u
2
+ v
2
τ
m
∂
∂y
(80)
where, regarding the derivation of equation (80), a fully developed channel flow is
assumed.
The original model of equation (57) makes for the streamwise turbulent heat
flux α
t
xy
=(C
∗
θ1
/f
RT
)v
2
τ
m
. Comparing both equations, the effect of dimensionless
Reynolds stress tensor for the prediction of the streamwise turbulent heat flux is
obviously obtained, i.e., the streamwise velocity intensity,
u
2
, which has a strong
correlation with
uθ [47], evidently improves the prediction.
8.9 Model Performances
8.9.1 Prediction of rotating channel flow using NLEDMM
The evaluations for the newly improved NLEDM (or NLEDMM) are shown in
Figures8.32–8.42. Inorderto calculatethepresent model,the numericaltechnique
used is a finite-volume method [48].
First, cases of wall-normal rotating channel flows are shown in Figures [8.32–
8.42]. In this case, the streamwise mean velocity decreases with the increasing
rotationnumberduetotheexchangemomentum betweenthestreamwise andspan-
wise velocity as indicated in Figures 8.32a and c. Thus, the spanwise velocity
increases at the lower rotation numbers, but the spanwise velocity reaches max-
imum value at a critical rotation number. Then, the spanwise velocity decreases
because the streamwise velocity becomes almost zero at higher rotation numbers.
In comparison with DNS results, the present NLEDMM gives better predictions
than the predictions of the NLCLS model. In particular, Reynolds shear stresses,
vw, are predicted very well as shown in Figure 8.32d. Figure 8.33 shows relation
betweenstreamwise andspanwisevelocitiesinthiscase, inwhich thelaminarsolu-
tionsare includedforcomparison. Obviously, theflowtendsto belaminarizedwith
theincreasing rotationnumber, andthe presentmodelcan predictproperlythisten-
dency. Also, anisotropy in turbulent intensities is reproduced by the present cubic
Sunden CH008.tex 10/9/2010 15: 19 Page 317
Recent developments in DNS 317
Ro
τ
=0.15
Ro
τ
=0.04
Ro
τ
=0.02
Ro
τ
=0.01
Ro
τ
=0.1
20
10
0
0
0
0
0
W
+
DNS
NLCLS(1996)
Present model
012
Ro
τ
=0.15
Ro
τ
=0.04
Ro
τ
=0.02
Ro
τ
=0.01
Ro
τ
=0.1
10
0
0
0
0
0
U
+
DNS
NLCLS(1996)
Present model
0
(a)
y/δ y/δ
12
Ro
τ
=0.15
Ro
τ
=0.1
Ro
τ
=0.04
Ro
τ
=0
Ro
τ
−0.02
Ro
τ
−0.01
1
0
0
0
0
0
0
−1
DNS
NLCLS(1996)
Present model
0
(b)
(c)
y/δ y/δ
(d)
12
uυ
+
Ro
τ
=0.15
Ro
τ
=0.04
z
τ
=0.02
Ro
τ
=0.01
Ro
τ
=0.1
DNS
NLCLS(1996)
Present model
012
5
0
0
0
0
0
−5
υw
+
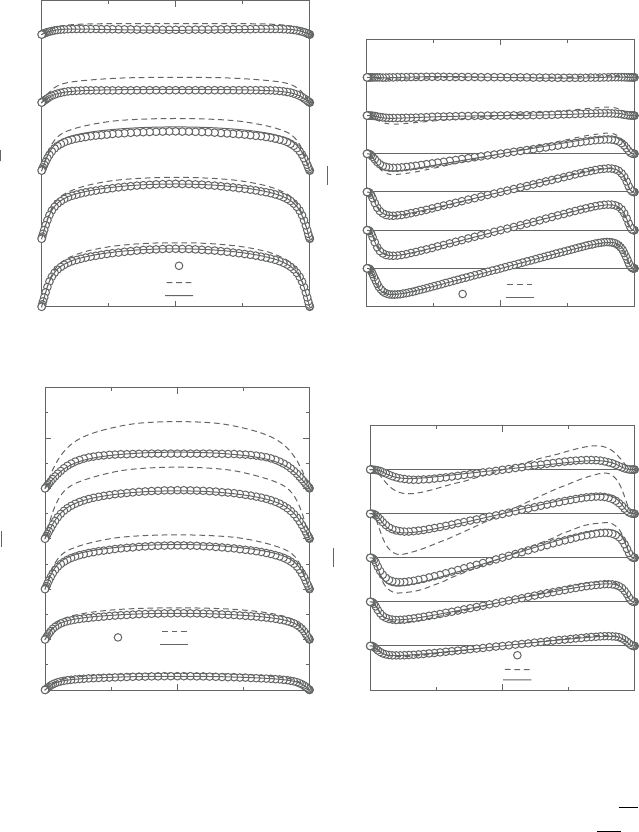
Figure 8.32. Distributions of predicted wall-normal rotating flows (Case 1):
(a) streamwise mean velocities, (b) Reynolds shear stresses,
uv,
(c) spanwise mean velocities, and (d) Reynolds shear stresses,
vw.
NLEDMMasshown inFigure8.34a.Asmentionedabove, theNLEDMM canpre-
dictaturbulentveolocityfieldsimilarinmanyrespectstotheNLHNmodelasshown
in Figure 8.28, but the wall-limiting behavior is satisfied exactly by the improved
model, as indicated in Figure 8.34b.Therefore, it is obvious that the present cubic
NLEDMM gives higher performance for the predictions of rotating channel flows
while maintaining the performance of the basis model, e.g., NLHN model.
Next, the predictions of fully developed streamwise rotating channel flows
calculated by the present model are shown in Figure 8.35. The results with the
Sunden CH008.tex 10/9/2010 15: 19 Page 318
318 Computational Fluid Dynamics and Heat Transfer
Predictions
5
4
3
2
1
0510
Ro
τ
=0.10
Ro
τ
=0.15
Ro
τ
=0.30
: Laminar solution
: DNS
U
+
W
+
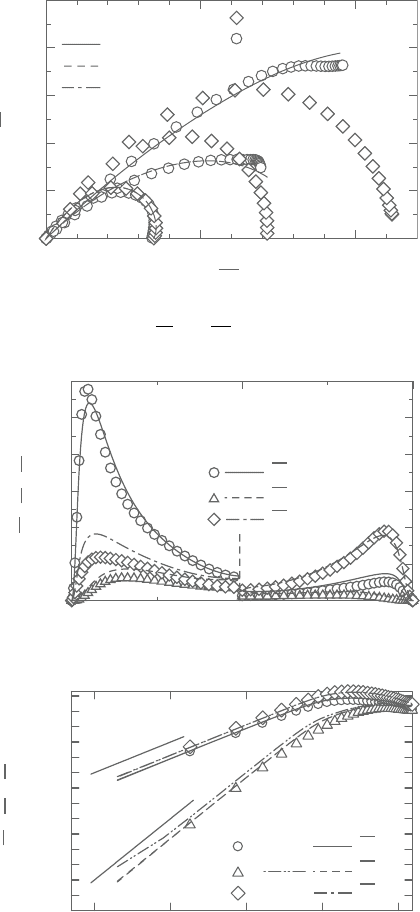
Figure 8.33. Relation of between U and W in wall-normal rotating channel flow.
Ro
τ
=0.10 Ro
τ
=0.1
Symbols : DNS
Lines : Present model
u
2
+
υ
2
+
w
2
+
6
4
2
u
2
+
υ
2
+
w
2
+
,,
012
(a)
y/δ
10
0
10
−2
10
−4
10
−6
10
−8
10
−10
10
−12
10
−14
10
−2
10
−1
y
+
y
+2
y
+4
(b)
10
0
10
1
10
2
u
2
+
υ
2
+
w
2
+
,,
Ro
τ
=0.1
DNS NLHN Present
u
2
+
υ
2
+
w
2
+
Figure 8.34. Distributionsofpredictedwall-normalrotatingflows(Case1): (a)tur-
bulent intensities and (b) wall-limiting behavior of Reynolds stress
components.
Sunden CH008.tex 10/9/2010 15: 19 Page 319
Recent developments in DNS 319
Ro
τ
= 1.0
Ro
τ
= 2.5
Ro
τ
= 7.5
Ro
τ
= 10
Ro
τ
= 15
DNS
20
U
+
0
0
0
0
012
y/δ
NLCLS(1996)
Present model
Ro
τ
= 1.0
Ro
τ
= 2.5
Ro
τ
= 7.5
Ro
τ
= 10
Ro
τ
= 15
1
uυ
+
0
0
0
0
0
−1
012
y/δ
DNS
NLCLS(1996)
Present model
(a) (b)
Ro
τ
= 1.0
Ro
τ
= 2.5
Ro
τ
= 7.5
Ro
τ
= 10
Ro
τ
= 15
DNS
2
W
+
0
0
0
0
0
012
y/δ
NLCLS(1996)
Present model
−2
Ro
τ
= 1.0
Ro
τ
= 2.5
Ro
τ
= 7.5
Ro
τ
= 10
Ro
τ
= 15
0.2
uw
+
0
0
0
0
0
012
y/δ
DNS
NLCLS(1996)
Present model
(c) (d)
(e) (f)
0
2
4
6
8
10
0
10
−2
10
−4
10
−6
10
−8
10
−10
10
−12
10
−2
10
−1
10
0
10
1
10
2
12
y/δ
u
2
+
v
2
+
y
+
2
y
+
y
+
1
w
2
+
Symbols : DNS
Lines : Preset model
DNS
NLHN
Present
Ro
τ
=10.0
Ro
τ
=1.0
Ro
τ
=10.0
u
2
+
υ
2
+
w
2
+
,,
u
2
+
υ
2
+
w
2
+
,,
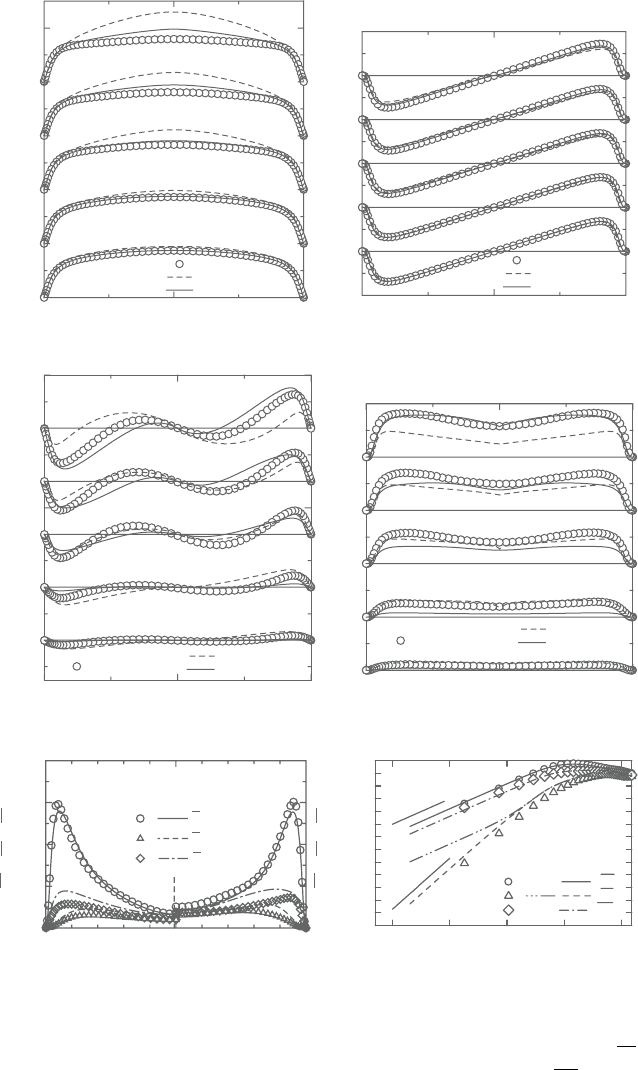
Figure 8.35. Distributions of predicted streamwise rotating flows (Case 2):
(a) streamwise mean velocities, (b) Reynolds shear stresses,
uv,
(c)spanwise meanvelocities,(d) Reynoldsshearstresses,
vw, (e) tur-
bulent intensities and (f) wall-limiting behavior of Reynolds stress
components.
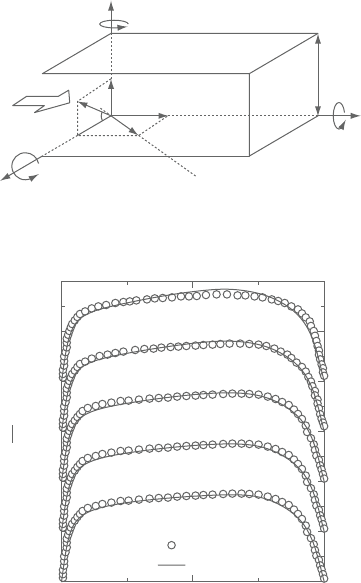
Sunden CH008.tex 10/9/2010 15: 19 Page 320
320 Computational Fluid Dynamics and Heat Transfer
Ω
3
Ω
2
2δ
Ω
1
x
3
Ro
τy
Ro
τx
Ro
τz
θ
x
2
x
1
Flow
Figure 8.36. Coordinatesystemandarbitraryrotatingaxisinrotatingchannelflow.
Ro
x
= 15, Ro
z
= 2.5
Ro
x
= 11, Ro
z
= 2.5
Ro
x
= 7.5, Ro
z
= 2.5
Ro
x
= 5, Ro
z
= 2.5
Ro
x
= 2.5, Ro
z
= 2.5
20
10
0
0
0
0
0
01 2
y/δ
U
+
DNS
Present model
Figure 8.37. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case STSP: streamwise mean velocities.
NLCLS model[40] arealso includedin the figurefor comparison. Itcan beseen in
Figures8.35a–dthatthepresentNLEDMMgivesaccuratepredictionsofbothmean
velocities and Reynolds shear stresses in all cases, and the test case of the highest
rotational number is adequately reproduced by the NLEDMM.Also, the Reynolds
normal stresses are indicated in Figures 8.35e and f. Now, the present NLEDMM
adequately reproduces redistribution of Reynolds normal stresses, and can pre-
dict wall-limiting behaviour exactly.These are because the modified characteristic
time-scale τ
Rw
given as equation (46) is introduced in the proposed NLEDMM.
To confirm the performance of theNLEDMM model, cases of rotating channel
flow with combined rotational axes are calculated as shown in Figure 8.36, in
which DNS databases of these cases are provided by Wu and Kasagi [17]. The
rotation numbers ofCase STSP are given asRo
τx
=2.5∼15 andRo
τz
=2.5, those
of Case WNSP1 as Ro
τy
=0.04 and Ro
τz
=2.5∼11 and those of CaseWNSP2 as
Ro
τy
=0.01∼0.04 andRo
τz
=2.5, respectively.The predicted meanvelocities are
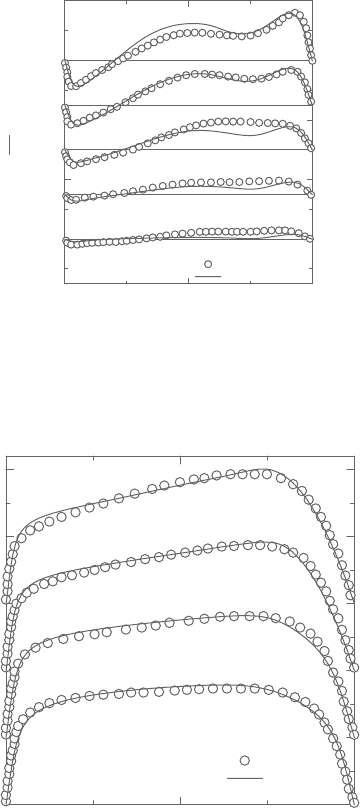
Sunden CH008.tex 10/9/2010 15: 19 Page 321
Recent developments in DNS 321
1.5
−1.5
y/δ
0
0
0
0
0
DNS
Present model
012
Ro
x
=2.5 , Ro
z
=2.5
Ro
x
=5 , Ro
z
=2.5
Ro
x
=7.5 , Ro
z
=2.5
Ro
x
=11 , Ro
z
=2.5
Ro
x
=15 , Ro
z
=2.5
W
+
Figure 8.38. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case STSP: spanwise mean velocities.
01
DNS
y/δ
Present
2
R
o
y
= 0.04,Ro
z
= 2.5
R
o
y
= 0.04,Ro
z
= 5.0
R
o
y
= 0.04,Ro
z
= 7.5
R
o
y
= 0.04,Ro
z
= 11
0
0
0
10
20
U+
Figure 8.39. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case WNSP1: streamwise mean velocities.
shown in Figures 8.37–8.42 with DNS data [17]. It can be seen that the proposed
NLEDMM gives accurate predictions of all cases, since the proper modeling for
rotatingflowswith arbitrary rotatingaxesis introducedin thepresent two-equation
model with the cubic NLEDMM.

Sunden CH008.tex 10/9/2010 15: 19 Page 322
322 Computational Fluid Dynamics and Heat Transfer
01
DNS
y/δ
Present
2
R
o
y
= 0.04,Ro
z
= 2.5
R
o
y
= 0.04,Ro
z
= 5.0
R
o
y
= 0.04,Ro
z
= 7.5
R
o
y
= 0.04,Ro
z
= 11
0
0
0
10
20
W
+
Figure 8.40. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case WNSP1: spanwise mean velocities.
20
0
10
0
0
0
12
y/δ
DNS
Present model
Ro
y
= 0.04
Ro
y
= 0.02
Ro
y
= 0.01
Ro
z
= 2.5
U
+
Figure 8.41. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case WNSP2: streamwise mean velocities.
8.9.2 Prediction of rotating channel-flow heat transfer using NLEDHM
Calculations identicalwith DNSconditions indicatedinTable8.5using turbulence
models are carried out in comparison with DNS results. Again, Figures 8.32 and
8.35 indicate predicted streamwise and spanwise mean velocities in both cases,
and it is obvious that mean velocities in both cases are adequately predicted by the
NLEDMM. Since a thermal field is passive scalar, turbulent quantities of veloc-
ity field should be accurately predicted by the turbulence model for the velocity
field. Therefore, the proposed NLEDHM can closely incorporate the NLEDMM.
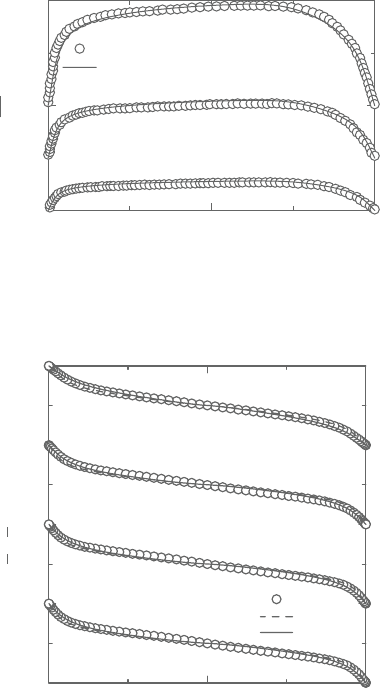
Sunden CH008.tex 10/9/2010 15: 19 Page 323
Recent developments in DNS 323
10
0
5
0
0
0
12
y/δ
DNS
Present model
Ro
y
= 0.04
Ro
y
= 0.02
Ro
y
= 0.01
Ro
z
= 2.5
W
+
Figure 8.42. Distributions of mean velocities in an arbitrary axis rotating channel
flow: Case WNSP2: spanwise mean velocities.
1
0
0
0
012
y/δ
DNS
NLHN
Present
Ro
τ
= 0.10
Ro
τ
= 0.04
Ro
τ
= 0.02
Ro
τ
= 0.01
(Θ − Θ
H
)/∆Θ
Figure 8.43. Predicted mean temperatures (Case 1).
Note that the following results are given from a calculation of full transpor t equa-
tions given in equations (4)–(12) with equation (44) and the present NLEDHM or
the NLHN model.
First,the distributions of meantemperature, whichareestimated by the present
NLEDHM are shown in Figure 8.43. The NLHN model (16) is also included in
the figures for comparison. Accurate prediction of the mean temperature of Case
1 can be done with both models. On the other hand, the proposed NLEDHM
is apparently improved to predict a streamwise turbulent heat flux as indicated
in Figure 8.44a. Although the streamwise turbulent heat flux does not affect the
distribution of mean temperature directly, the turbulent heat transfer model should
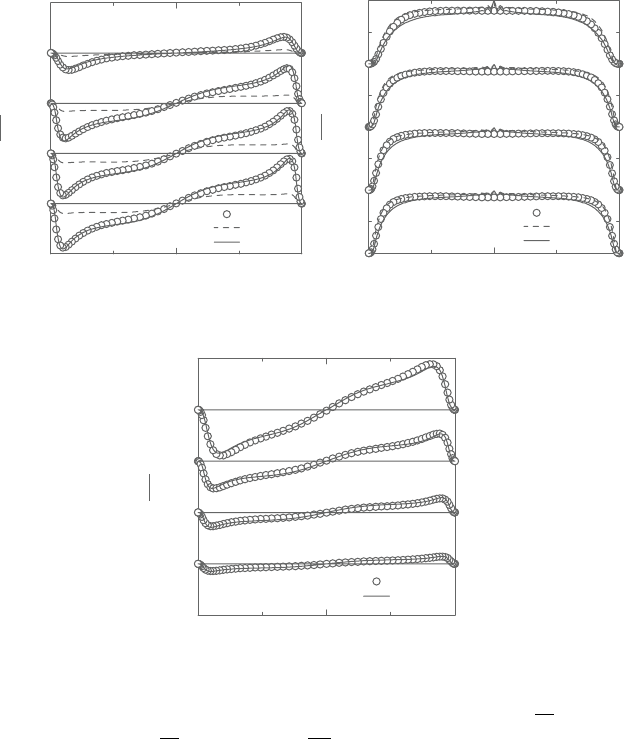
Sunden CH008.tex 10/9/2010 15: 19 Page 324
324 Computational Fluid Dynamics and Heat Transfer
(a) (b)
1
0
0
0
012
DNS
NLHN
Present
Ro
τ
= 0.10
Ro
τ
= 0.04
Ro
τ
= 0.02
Ro
τ
= 0.01
v u
+
y /δ
6
0
0
0
0
012
DNS
NLHN
Present
−6
uu
+
Ro
τ
= 0.10
Ro
τ
= 0.04
Ro
τ
= 0.02
Ro
τ
= 0.01
y/δ
(c)
DNS
Present
6
0
0
0
0
−6
ωu
+
Ro
τ
= 0.1
Ro
τ
= 0.04
Ro
τ
= 0.02
Ro
τ
= 0.01
012
y/δ
Figure 8.44. Predictedturbulentheatfluxes(Case1);(a)streamwise(uθ), (b)wall-
normal (
vθ), (c) spanwise (wθ).
predict the streamwise turbulent heat flux to apply some turbulent heat transfer
problemsuchas buoyant flow.As for the predicted wall-normal heat flux as shown
inFigure8.44b,adiscontinuouslinecanbefaintlyseeninthechannelcenterregion.
Thisiscausedbythepredictedwall-normalvelocityintensity.Sincethewall-normal
velocity intensity is slightly overpredicted in this case by the NLEDMM, the wall-
normal turbulent heat flux is remarkably affected by the prediction. The predicted
spanwise turbulent heat flux is shown in Figure 8.44c. The present predictions are
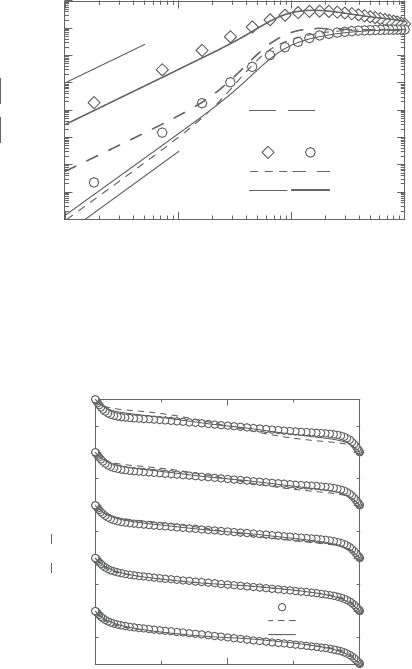
ingood agreement withDNS.Figure8.45 showsthe wall-limiting behaviorof both
streamwise and wall-normal turbulent heat fluxes. Since the heat is carried from
the wall in the case of the heated wall condition, the wall-limiting behavior should
be reproduced to exactly estimate heat transfer rate. It can be seen that the present
Sunden CH008.tex 10/9/2010 15: 19 Page 325
Recent developments in DNS 325
DNS
NLHN
Present
10
−1
10
−5
10
−7
10
−3
10
1
u u
+
v u
+
10
−1
10
0
10
1
y
+
10
2
y
+2
y
+3
Ro
t
= 0.04
u u
+
v u
+
Figure 8.45. Wall-limiting behavior of turbulent heat fluxes (Case 1).
1
0
0
0
0
012
y/δ
DNS
NLHN
Present
Ro
τ
− 15
Ro
τ
10
Ro
τ
7.5
Ro
τ
2.5
Ro
τ
1.0
(Θ − Θ
H
)/∆Θ
Figure 8.46. Predictions of mean temperature (Case 2).
NLEDHM appropriately predicts both turbulent heat fluxes. Note that the present
NLEDHM exactly satisfies the difference between turbulent heat fluxes, i.e., the
streamwise turbulent heat flux is propor tional to y
2
, and the wall-normal turbulent
heat flux is proportional to y
3
.
In Case 2, although the mean temperature indicated in Figure 8.46 is slightly
underpredicted by the NLHN model in the highest rotation number, the proposed
model can satisfactorily reproduce it. Also, in this case, it can be seen that the
proposed NLEDHM adequately predicts both the streamwise and the wall-normal
turbulentheatfluxesasshowninFigure8.47. Ontheotherhand, itisdifficultforthe
present model to predict the spanwise turbulent heat flux in Case 2. The spanwise
