Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling

Подождите немного. Документ загружается.

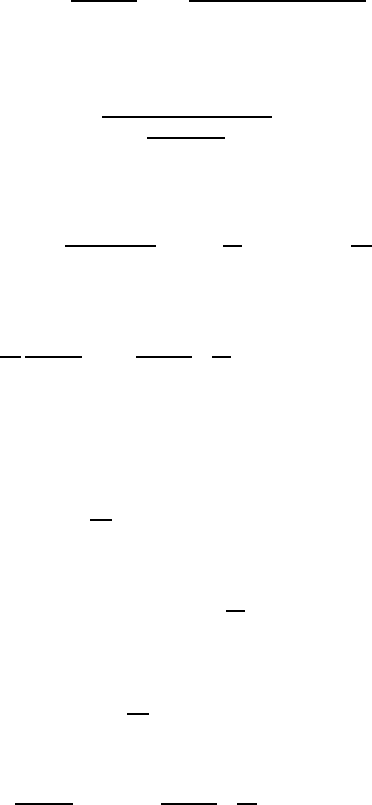
236

Superconducting state of cuprates

The effective potential about a point charge in the charged Bose gas (CBG)

was calculated by Hore and Frankel [290]. Its static dielectric function is:

(q, 0) = 1 +

4π(e

∗

)









− f

k−q

/2m

∗∗

− k · q/m

∗∗



(8.72)

in which e

∗

= 2e the boson charge,  is the volume of the system and

exp



/2m

∗∗

−µ



− 1

Eliminating the chemical potential, for small q the dielectric function for T < T

(q, 0) = 1 +

4(m

∗∗

)



1 −





3/2



+ O





(8.73)

and, for T →∞,

(q, 0) = 1 +

∗∗



1 +

ζ(3/2)

3/2





3/2

+···



+ O(q

) (8.74)

with ω

= 4π(e

∗

)

/(

∗∗

) and n

the boson density. If the unscreened

scattering potential is the Coulomb potential (V (r) = V

/r), then performing

the inverse Fourier transforms, one ﬁnds that, for T < T

[290],

lim

r→∞

V (r) =

exp[−K

r]cos[K

r]≡V

(r) (8.75)

with

= (m

∗∗

)

1/2



1 −





3/2



1/4

(8.76)

and for T →∞,

lim

r→∞

V (r) =

exp[−K

r]≡V

(r) (8.77)

with



∗∗



1/2



1 +

ζ(3/2)

3/2





3/2

+···



1/2

. (8.78)

The T < T

result is exact for all r at T = 0.

There are two further important analytical results; the ﬁrst (Levinson’s

theorem [289]) states that for ‘regular’ potentials (which include all those with

which we shall be concerned), the zero-energy phase shift is equal to π multiplied

by the number of bound states of the potential. The second is the well-known

Sharp increase of the quasi-particle lifetime below

237

Figure 8.14. (a) Plots of zero-energy scattering cross sections (i) σ

and (ii) σ

against screening wavevector K for the potentials (i) V

(r) =−(1/r )e

−K

and (ii)

(r) =−(1/r )e

−K

cos(K

r).(b)Plotofσ

/σ

for a range of K = K

= K

which both potentials have no bound states. In each case the units are those used to derive

the phase equation.

Wigner resonance scattering formula, which states that for slow-particle scattering

of a particle with energy E off a potential with a shallow bound state of the

binding energy 

E, the total scattering cross section is

σ =

2π

E +||

. (8.79)

The zero-energy scattering cross sections for the potentials V

(r) =

238

Superconducting state of cuprates

−(V

/r)e

−Kr

and V

(r) =−(V

/r)e

−Kr

cos(Kr) are shown in ﬁgure 8.14(a).

These graphs are plotted for V

= 1; in each case, the equivalent graph for

arbitary V

may be found by rescaling σ and K . According to the Wigner formula

(equation (8.79)) as K decreases, when a new bound state appears there should be

a peak in the cross section, as there will then be a minimum in the binding energy

of the shallowest bound state. This is the origin of the peaks in ﬁgure 8.14(a),

which may be checked using Levinson’s theorem. It can also be seen that as K

decreases, the ﬁrst few bound states appear at higher K in the ordinary Yukawa

potential; this agrees with the intuitive conclusion that the bound states should,

in general, be deeper in a non-oscillatory potential. Another intuitive expectation

which is also borne out is that for a given V

and K , the non-oscillatory potential

should be the stronger scatterer. In ﬁgure 8.14(b) it may be seen that this is the

case when K is large enough, so that both potentials have no bound states (the

difference in cross sections is then, in fact, about three orders of magnitude).

At zero temperature, the screening wavevector is K

= (m

∗∗

)

1/2

,and

at a temperature T = T

+ 0 just above the transition, K

= (m

∗∗

)

1/2

Substituting ω

and T

 3.3n

2/3

∗∗

, we obtain



2.1e

√

∗∗



1/2

1/6



1/2

. (8.80)

From this, we see that the ratio is only marginally dependent on the boson density,

so substituting for n

= 10

−3

, e and m

, we obtain

αT

= 3.0



∗∗





1/4

. (8.81)

With a realistic boson mass m

∗∗

= 10m

and dielectic constant 

= 100,

and K

, while different, are of the same order of magnitude. If the screening

wavevectors are such that neither the normal state nor condensate impurity

potentials have bound states, with these parameters it would then follow that the

quasi-particle lifetime is much greater in the superconducting state, ﬁgure 8.14(b).

This effect could then explain the appearance of sharp quasi-particle peaks in

ARPES and tunnelling, and the enhancement of the thermal conductivity in

cuprates below T

[203] due to a many-fold increase in the quasi-particle lifetime.

8.6 Symmetry of the order parameter and stripes

In bipolaron theory, the symmetry of the BEC on a lattice should be distinguished

from the ‘internal’ symmetry of a single-bipolaron wavefunction and from the

symmetry of a single-particle excitation gap. As described in section 4.7.9, a

Bose condensate of bipolarons might be d-wave, if bipolaron bands have their

minima at ﬁnite K in the centre-of-mass Brillouin zone. The d-wave condensate

Symmetry of the order parameter and stripes

239

reveals itself as a checkerboard modulation of the hole density and of the global

gap (equation (8.19)) in the real (Wannier) space (ﬁgure 4.14). At the same time,

the single-particle excitation spectrum might be an anysotropic s-wave providing

an explanation of conﬂicting experimental observations.

The two-dimensional pattern (ﬁgure 4.14) is oriented along the diagonals,

i.e. the d-wave bipolaron condensate is ‘striped’. Hence, there is a fundamental

connection between stripes detected by different techniques [292,293] in cuprates

and the symmetry of the order parameter [123]. Originally antiferromagnetic

interactions were thought to give rise to spin and charge segregation (stripes)

[291]. However, the role of long-range Coulomb and Fr¨ohlich interactions has

not been properly addressed. Here we show that the Fr¨ohlich electron–phonon

interaction combined with the direct Coulomb repulsion does not lead to charge

segregation like strings or stripes in doped insulators and the antiferromagnetic

exchange interaction is not sufﬁcient to produce long stripes either [294].

However, the Fr¨ohlich interaction signiﬁcantly reduces the Coulomb repulsion,

and allows much weaker short-range electron–phonon and antiferromagnetic

interactions to bound carriers into small bipolarons. Then the d-wave Bose

condensate of bipolarons naturally explains superstripes in cuprates.

As discussed in section 4.4. the extention of the deformation surrounding

(Fr¨ohlich) polarons is large, so their deformation ﬁelds overlap at a ﬁnite density.

However, taking into account both the long-range attraction of polarons due to

the lattice deformations and the direct Coulomb repulsion, the net long-range

interaction is repulsive. At distances larger than the lattice constant (|m − n|≥

a ≡ 1), this interaction is signiﬁcantly reduced to



|m − n|

. (8.82)

Optical phonons reduce the bare Coulomb repulsion at large distances in ionic

solids if 

 1, which is the case in oxides.

Let us ﬁrst consider a non-adibatic and intermediate regime when the

characteristic phonon energy is comparable with the kinetic energy of holes.

In this case the problem is reduced to narrow-band fermions with a repulsive

interaction (equation (8.82)) at large distances and a short-range attraction at

atomic distancies. Because the net long-range repulsion is relatively weak, the

relevant dimensionless parameter r

(= m

∗

/

(4πn/3)

1/3

) is not very large in

doped cuprates and the Wigner crystallization does not appear at any physically

interesting density. In contrast, polarons could be bound into small bipolarons

and/or into small clusters as discussed in sections 4.6.3 and 5.4.2 but, in any

case, the long-range repulsion would prevent any clustering in inﬁnitely charged

domains.

In the opposite adiabatic limit, one can apply a discrete version [80] of

the continuous nonlinear Pekar equation [61], taking into account the Coulomb

repulsion and lattice deformation for a single-polaron wavefunction, ψ

(the

240

Superconducting state of cuprates

amplitude of the Wannier state |n):

−



m=0

t (m)[ψ

− ψ

n+m

]−eφ

= Eψ

. (8.83)

The potential φ

n,k

actingonafermion,k, at the site n is created by the

polarization of the lattice, φ

n,k

, and by the Coulomb repulsion with the other

M − 1 fermions, φ

n,k

= φ

n,k

+ φ

n,k

. (8.84)

Both potentials satisfy the discrete Poisson equation:

κφ

n,k

= 4πe



p=1

|ψ

n, p

(8.85)

and



∞

φ

n,k

=−4πe



p=1, p=k

|ψ

n, p

(8.86)

with φ



(φ

− φ

n+m

). Then the functional J [61], describing the total

energy in this self-consistent Hartree approximation, is given by

J =−



n, p,m=0

∗

n, p

t (m)[ψ

n, p

− ψ

n+m, p

]−

2πe



n, p,m,q

|ψ

n, p



−1

|ψ

m,q

2πe



∞



n, p,m,q=p

|ψ

n, p



−1

|ψ

m,q

The single-particle function of a hole trapped in a string of the length N is

= N

−1/2

exp(ikn) with periodic boundary conditions, so that the functional J

is expressed as J = T +U,whereT =−2t (N − 1) sin(π M/N)/[N sin(π/N)]

is the kinetic energy, proportional to t,and

U =−



∞

M(M − 1)I

(8.87)

corresponds to the polarization and Coulomb energies. Here the integral I

given by

(2π)



−π



−π



−π

sin(Nx/2)

sin(x/2)

(3 − cos x − cos y − cos z)

−1

It has the following asymptotics at N  1:

1.31 +ln N

. (8.88)

Symmetry of the order parameter and stripes

241

If we split the ﬁrst (attractive) term in equation (8.87) into two parts by

replacing M

for M + M(M − 1), then it becomes clear that the net interaction

between polarons remains repulsive in the adiabatic regime as well because

κ>

∞

. And this shows the absence of strings or stripes. The energy of M

well-separated polarons is lower than the energy of polarons trapped in a string.

When a short-range e–ph (or exchange) interaction is taken into account,

a string of ﬁnite length can appear (sections 4.6.3 and 5.4.2). We can readily

estimate its length by the use of equation (8.87) for any type of short-range

interaction. For example, dispersive phonons, ω

= ω + δω(cos q

+ cos q

cos q

) with a q-independent matrix element γ(q) = γ , yield a short-range

polaron–polaron attraction:

att

(n − m) =−E

att

(δω/ω)δ

|n−m|,1

(8.89)

where E

att

= γ

ω/2. Taking into account the long-range repulsion as well, the

potential energy of the string with M = N polarons is now

U =



−

att

δω

. (8.90)

Minimizing this energy yields the length of the string as

N = exp





att

δω

− 2.31



. (8.91)

Actually, this expression provides a fair estimate of the string length for

any kind of attraction (not only generated by phonon dispersion) but also by

antiferromagnetic exchange and/or by Jahn–Teller type of e–ph interactions. Due

to the numerical coefﬁcient in the exponent in equation (8.91), one can expect

only short strings (if any) with realistic values of E

att

(about 0.1 eV) and with a

static dielectric constant 

≤ 100.

We conclude that there are no strings in ionic narrow-band doped

insulators with only the Fr¨ohlich interaction. Short-range electron–phonon and/or

antiferromagnetic interactions could give rise to a bipolaronic liquid and/or short

strings only because the long-range Fr¨ohlich interaction signiﬁcantly reduces the

Coulomb repulsion in highly polarizable ionic insulators. However, with the

typical values of 

= 30, a = 3.8

A, one obtains N 2 in equation (8.91)

even with E

att

as high as 0.3 eV. Hence, the short-range attractive forces are not

strong enough to segregate charges into strings of any length, at least not in all

high-T

cuprates.

If (bi)polaronic carriers in many cuprates are in a liquid state, one can

pose the key question of how one can see stripes at all. In fact, the bipolaron

condensate might be striped owing to the bipolaron energy band dispersion,

as previously discussed. In this scenario, the hole density, which is about

twice that of the condensate density at low temperatures, is striped, with the

242

Superconducting state of cuprates

characteristic period of stripes determined by inverse wavevectors corresponding

to bipolaron band minima. Such an interpretation of stripes is consistent with

the inelastic neutron scattering in YBa

7−δ

, where the incommensurate

peaks were observed only in the superconducting state [154]. The vanishing

at T

of the incommensurate peaks is inconsistent with any other stripe

picture, where a characteristic distance needs to be observed in the normal

state as well. In contrast, with the d-wave striped Bose–Einstein condensate,

the incommensurate neutron peaks should disappear above T

, as observed.

Importantly, a checkerboard modulation (ﬁgure 4.14) has been observed in STM

experiments with [295] and without [296] an applied magnetic ﬁeld in Bi cuprates.

Chapter 9

Conclusion

The main purpose of this book was to lead the reader from the basic principles

through detailed derivations to a description of the many facinating phenomena

in conventional and unconventional (high T

) superconductors. The seminal work

by Bardeen, Cooper and Schrieffer taken further by Eliashberg to intermediate

coupling solved the major scientiﬁc problem of Condensed Matter Physics in

the ﬁrst half of the 20th century. High-temperature superconductors present a

challenge to the conventional theory. While the BCS theory gives a qualitatively

correct description of some novel superconductors like magnesium diborade and

doped fullerenes, if the phonon dressing of carriers (i.e. polaron formation)

is properly taken into account, cuprates remain a real problem. Here strong

antiferromagnetic and charge ﬂuctuations and the Fr¨ohlich and Jahn–Teller

electron–phonon interactions have been identiﬁed as an essential piece of physics.

We have discussed the multi-polaron approach to the problem based on our

recent extension of BCS theory to the strong-coupling regime. The low-energy

physics in this regime is that of small bipolarons, which are real-space electron

(hole) pairs dressed by phonons. They are itinerant quasi-particles existing

in the Bloch states at temperatures below the characteristic phonon frequency.

We have discussed a few applications of the bipolaron theory to cuprates, in

particular the bipolaron theory of the normal state, of the superconducting

critical temperature and the upper crtitical ﬁeld, the isotope effect, normal and

superconducting gaps, the magnetic-ﬁeld penetration depth, tunnelling and the

Andreev reﬂection, angle-resolved photoemission, stripes and the symmetry of

the order parameter. These and some other experimental observations have been

satisfactorily explained using this particular approach, which provides evidence

for a novel state of electronic matter in layered cuprates. This is the charged Bose

liquid of bipolarons. A direct measurement of a double elementary charge 2e on

carriers in the normal state could be decisive.

243

Appendix A

Bloch states

A.1 Bloch theorem

If we neglect the interaction of carriers with impurities and ion vibrations, and

take the Coulomb interaction between carriers in the mean-ﬁeld (Hartree–Fock)

approximation (similar to the central-ﬁeld approximation for atoms) into account,

we arrive at a one-electron Hamiltonian with a periodic (crystal ﬁeld) potential

V (r). The wavefunction obeys the Schr¨odinger equation



−

∇

+ V (r)



ψ(r) = Eψ(r). (A.1)

The Bloch theorem states that one-particle eigenstates in the periodic potential

are sorted by the wavevector k in the ﬁrst Brillouin zone and by the band index n

and have the form

(r) = u

(r)e

ik·r

. (A.2)

To prove the theorem, let us deﬁne the translation operator T

, which shifts the

argument by the lattice vector l while acting upon any function F(r):

F(r) = F(r + l). (A.3)

Since the Hamiltonian has the translation symmetry, H (r + l) = H (r),

it commutes with T

. Hence, the eigenstates of H can be chosen to be

simultaneously eigenstates of all the T

ψ(r) = c(l)ψ(r) (A.4)

where c(l) is a number depending on l. The eigenvalues of the translation

operators are related as follows:

c(l + l



) = c(l)c(l



) (A.5)

244

Bloch theorem

245

since shifting the argument by l +l



leads to the same function as two successive

translations, T

l+l



= T



. This relation is satisﬁed if

c(l) = exp(ik · l) (A.6)

with any k. Imposing an appropriate boundary condition on the wavefunction

makes the allowed wavevectors k real and conﬁned to the ﬁrst Brillouin zone. The

most convenient condition is the Born–von Karman periodic boundary condition

ψ(r + N

) = ψ(r) (A.7)

where j = 1, 2, 3, the a

are three primitive vectors of the crystal lattice and N

are large integers, such that N = N

is the total number of primitive cells

in the crystal (N  10

−3

). This requires that

exp(iN

k · a

) = 1. (A.8)

Therefore, the general form for allowed wavevectors is

k =

. (A.9)

Here, n

are integers and b

are primitive vectors for a reciprocal lattice, which

satisfy

= 2πδ

(A.10)

where δ

is the Kronecker delta symbol (δ

= 1, if i = j, and zero otherwise).

Replacing k by k + G does not change any of the c(l) if G is a reciprocal lattice

vector (i.e. a linear combination of the b

with integer coefﬁcients). Hence, all

eigenstates of the periodic Hamiltonian can be sorted by the wavevectors conﬁned

to the primitive cell of the reciprocal lattice, for example to the region

−π<ka

π (A.11)

which is the ﬁrst Brillouin zone. In particular for a simple cubic lattice with period

k =

2π



i=1,2,3

(A.12)

where −N

/2 < n

/2ande

are unit vectors parallel to the primitive lattice

vectors. The number of allowed wavevectors in the ﬁrst Brillouin zone is equal to

the number of cells, N, in the crystal. The volume, k, of the reciprocal k -space

per allowed value of k is just the volume of a little parallelepiped with edges

k =

· b

× b

. (A.13)