Alciatore D.G., Histand M.B. Introduction to Mechatronics and Measurement Systems
Подождите немного. Документ загружается.


Confirming Pages
4.11 System Modeling and Analogies 151
The only problematic analogy in the table is hydraulic resistance, because this
quantity is usually not constant. It is typically a nonlinear function of flow rate and
geometry. Analogies can also be extended to other phenomena such as heat transfer,
where temperature difference is the “effort,” heat flow is the “flow,” the amount of
heat transferred is the “displacement,” heat capacity due to mass and specific heat
is “capacitance,” and thermal resistance due to convection and conduction is “resis-
tance.” However, heat transfer and many other phenomena cannot be adequately
modeled by second-order linear differential equations, so there are no direct analo-
gies for the relations given in the table.
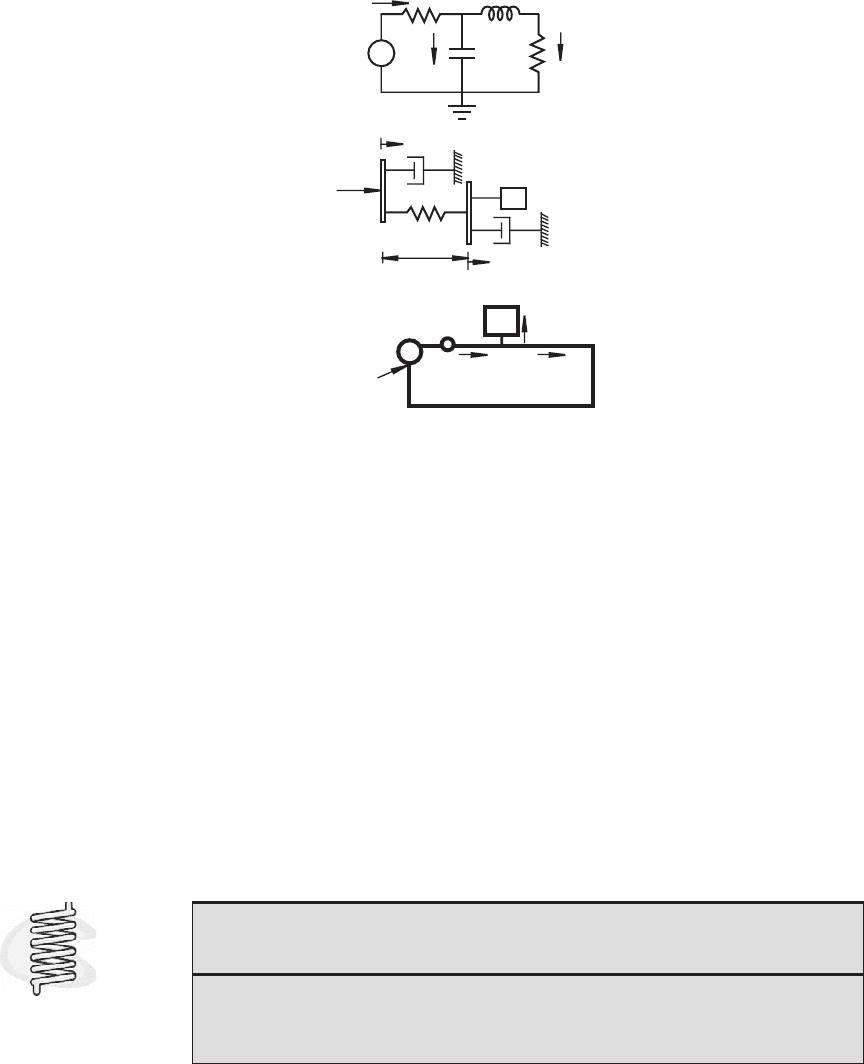
Figure 4.21 illustrates an example of electrical, mechanical, and hydraulic
system analogies along with their governing equations. Each of these systems is
described by differential equations of exactly the same form. The only differences
are the constant parameters that describe the analogous elements. Any quantity in
one system is directly analogous to a quantity in each of the other two systems.
For example, the capacitor in the electrical system is analogous to the spring in the
mechanical system and to the tank in the hydraulic system, and the current through
the capacitor (I
2
= I
1
− I
3
) is analogous to the rate of compression of the spring
(v
2
= v
1
− v
3
) in the mechanical system and to the flow rate into the tank (Q
2
= Q
1
− Q
3
)
in the hydraulic system.
Knowledge of these analogies helps one gain a deeper understanding and intui-
tive feel for how different systems respond. It also provides a framework to model and
analyze a great variety of systems in a generic form. An understanding of the char-
acteristics and analysis of one type of system can directly apply to any other system.
In the past, before the advent of digital computers, modeling analogies were
essential in the application of analog computers to simulating and analyzing the
Table 4.1 Second-order system modeling analogies
Generic
quantity
Mechanical
translation
Mechanical
rotation Electrical Hydraulic
Effort (E ) Force (F) Torque (T) Voltage (V) Pressure (P)
Flow (F ) Speed (v)
Angular speed ()
Current (i)
Volumetric flow
rate (Q)
Displacement (q) Displacement (x)
Angular displacement (θ)
Charge (q)
Volume (V
)
Momentum (p)
Linear momentum
(p = mv)
Angular momentum
(h = Jω)
Flux linkage
(I = NΦ = Li)
Momentum
/area
(Γ = IQ)
Resistor (R) Damper (b) Rotary damper (B) Resistor (R) Resistor (R)
Capacitor (C )
Spring (1
/k) Torsion spring (1/k)
Capacitor (C) Tank (C)
Inertia (I ) Mass (m) Moment of inertia (J) Inductor (L) Inertance (I)
Inertia energy storage
(special case)
F = p
(F = ma)
T = h
(T = Jα)
V = λ
(V = L di
/dt)
P =
Γ
(P = I dQ/dt)
Capacitor energy
storage
F = kx (T = kθ)
V = (1
/C)qP = (1/C) V
Dissipative
F = bv T = B V = Ri P = RQ
.
..
.
alc80237_ch04_117-160.indd 151alc80237_ch04_117-160.indd 151 20/01/11 5:45 PM20/01/11 5:45 PM
Confirming Pages
Figure 4.21 Example of system analogies.
+
L
C
fixed support (reference)
rigid,
massless
platform
V = 0 (reference)
F
k
m
V
I
1
R
1
I
2
R
2
I
3
F = F
b
1
+ F
k
v
1
b
1
b
2
v
3
x
2
= x
1
– x
3
V = V
R
1
+ V
C
= R
1
I
1
+ (1/C)q
2
V
C
= V
L
+ V
R
2
= L dI
3
/dt + R
2
I
3
= b
1
v
1
+ k(x
1
– x
3
)
F
k
= F
m
+ F
b
2
= m dv
3
/dt + b
2
v
3
P = P
R
1
+ P
C
P
C
= P
I
+ P
R
2
X
P
pump
P = 0 (reference)
long pipe
valve
tank
C
R
2
, I
Q
2
Q
3
Q
1
R
1
152 CHAPTER 4 System Response
behavior of various types of systems. The procedure was to develop an electrical
system analogous to the physical system of interest; build the analogous electrical
system with the aid of an analog computer, which provided scaling, integration,
differentiation, mixing, and other functions; and perform simulations by providing
inputs in the form of voltage signals resulting in voltage or current outputs analogous
to quantities of interest in the physical system being simulated. As an example, the
behavior of the mechanical system shown in Figure 4.21 could be studied by build-
ing the electrical circuit in the figure with C = s/k, R
1
= s
.
b
1
, R
2
= s
.
b
2
, and L = s
.
m,
where s is some constant scaling factor. Predicting the force on and speed of the mass
m given some applied force F(t) would be a simple matter of applying V(t) = s
.
F(t)
volts to the circuit and monitoring the voltage across and the current through the
inductor. The force and speed that would be experienced by the mass in the actual
mechanical system would be F
m
= (V
L
/s) and v
m
= (I
L
/s). Before the availability of
numerical integration simulation software on digital computers, this was the only
method for simulating the behavior of complex physical systems.
■ CLASS DISCUSSION ITEM 4.9
Initial Condition Analogy
What is the electrical analogy for an initial displacement of a spring in a mechanical
system? In other words, what is the corresponding initial condition in the electrical
system?
alc80237_ch04_117-160.indd 152alc80237_ch04_117-160.indd 152 1/4/11 3:48 PM1/4/11 3:48 PM

Confirming Pages
Figure 4.22 Mechanical system analogy example.
m
m
Free-Body Diagrams:
Equations of Motion:
1
2
3
F
m
= –ma
3
v
3
b
1
F
1
k
1
F
2
b
2
k
2
v
2
v
1
F
1
F
2
F
b
1
= b
1
(v
1
– v
3
)
F
k
1
= k
1
(x
3
– x
2
)
F
k
2
= k
2
(x
1
– x
2
)
F
b
2
= b
2
v
2
F
1
– F
b
1
– F
k
2
= 0
F
b
1
– F
k
1
= F
m
F
k
1
+ F
k
2
– F
2
– F
b
2
= 0
Mechanical System:
4.11 System Modeling and Analogies 153
The following procedure facilitates converting from a system in one form to an
analogous system in some other form. As an illustrative example, the mechanical
system shown in Figure 4.22 is converted to its analogous electrical system.
Steps in Converting from One System to an Analogous System
1. Label the flows with the appropriate sign for each element in the system and its
analogy.
The algebraic sign given to a flow depends on the direction of the effort on the
element. For the example in Figure 4.22, the applied load F
1
is in the direction
of speed v
1
, so its flow is v
1
, but applied load F
2
is in the opposite direction
to v
2
, so its flow is −v
2
. For the spring and damper flows, because the forces
are drawn assuming compression, the flow for b
1
is (v
1
− v
3
) and the flow for
k
1
is (v
3
− v
2
). The quantities for the analogous system are obtained by direct
substitution with quantities based on Table 4.1. The flows and analogies for
the entire example are shown in the table that follows.
Mechanical
element
Mechanical
flow
Electrical
flow
Electrical
element
F
1
v
1
I
1
V
1
b
1
v
1
− v
3
I
1
- I
3
R
1
mv
3
I
3
L
k
1
v
2
− v
2
I
3
- I
2
C
1
k
2
v
2
− v
2
I
1
- I
2
C
2
b
2
v
2
I
2
R
2
F
2
−v
2
-I
2
V
2
2. Formulate the system equations at each node using the equations of motion
(ΣF = ma) for mechanical systems or KVL loop equations for electrical systems.
For the example, see the free-body diagrams and system equations in Figure 4.22.
Note that for the massless, rigid platforms, the equation is of the form ΣF = 0,
because they are assumed to have negligible mass. For the mass m, the form
alc80237_ch04_117-160.indd 153alc80237_ch04_117-160.indd 153 20/01/11 5:45 PM20/01/11 5:45 PM
Confirming Pages
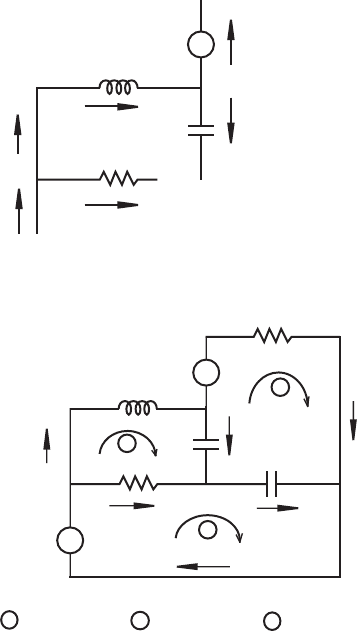
Figure 4.23 Beginning the analog schematic.
+
L
(I
3
– I
2
)
I
2
V
2
C
1
I
3
(I
1
– I
3
)
I
1
I
3
R
1
154 CHAPTER 4 System Response
is ΣF = ma. Or, alternatively, the inertial term (ma) can be considered as an
inertial force (Fm = −ma) as shown in Figure 4.22. In this case, the system
equation is again of the form F = 0.
3. Pick an element to start with and begin constructing the schematic for the
analogy using the fl ows for guidance. It is best to start with an element that is
embedded in the system (i.e., its fl ow affects many other elements), although
any element would suffi ce.
Figure 4.23 shows how the schematic is started, beginning with inductor L
(mass m) and recursively branching to other elements that involve its fl ow (I
3
).
Note that the free-body diagram (FBD) or KVL equations and the element
fl ow relationships need to be enforced as the schematic is constructed. The
completed circuit is shown in Figure 4.24.
4. Verify the graph with the analogous system equations.
For the example, the KVL loop equations indeed are the same form as the
free-body diagram equations of motion (see Figures 4.22 and 4.24).
Figure 4.24 Electrical system analogy example.
+
+
L
+
+
+
+
+
–
–
–
–
1
3
KVL loop equations:
1
2
3
V
1
= V
R
1
+ V
C
2
2
V
L
= V
R
1
– V
C
1
V
2
= V
C
1
+ V
C
2
– V
R
2
R
2
R
1
(I
3
– I
2
)
(I
1
– I
2
)(I
1
– I
3
)
I
1
I
2
I
3
C
2
C
1
–
V
1
V
2
alc80237_ch04_117-160.indd 154alc80237_ch04_117-160.indd 154 1/4/11 3:48 PM1/4/11 3:48 PM

Confirming Pages
Questions and Exercises 155
QUESTIONS AND EXERCISES
Section 4.2 Amplitude Linearity
4.1. If the following input (V
in
) and output (V
out
) relationships exist for different measure-
ment systems, indicate whether each is linear or nonlinear?
a. V
out
(t) = 5 V
in
(t)
b. V
out
(t)/V
in
(t) = 5t
c. V
out
(t) = V
in
(t) + 5
d. V
out
(t) = V
in
(t) + V
in
(t)
e. V
out
(t) = V
in
(t) V
in
(t)
f. V
out
(t) = V
in
(t) + 10t
g. V
out
(t) = V
in
(t) + sin(5)
Section 4.3 Fourier Series Representation
of Signals
4.2. What is the Fourier series and fundamental frequency (in hertz) of the waveform
f(t) 5 sin(2πt)?
4.3. What is the Fourier series and fundamental frequency (in rad/sec) of a standard
U.S. household AC voltage?
4.4. Verify that Equations 4.7 through 4.9 are equivalent to Equation 4.3.
4.5. Show that the Fourier series coefficients A
n
for the square wave defined by
Equation 4.10 are 0.
4.6. The discrete Fourier series representation of a half-sine-wave pulse train waveform is
mathematically represented as
Vt()
1
π
---
2πt()sin
2
---------------------
2
π
---
4πt()cos
13⋅
----------------------
8πt()cos
35⋅
----------------------
12πt()cos
57⋅
-------------------------
···++ +–+=
Using a computer plotting application, plot three cycles of V(t) displaying
a. DC component + fundamental (fi rst harmonic)
b. DC component + first 10 harmonics (Note that some harmonics have zero
amplitude)
■ CLASS DISCUSSION ITEM 4.10
Measurement System Physical Characteristics
Inertia, capacitance, and damping are almost always present in a measurement sys-
tem. Sometimes these characteristics are desirable; other times they are undesirable.
Think of examples of measurement systems containing mechanical, electrical, or
hydraulic components and discuss the advantages and disadvantages of changing
the physical characteristics of the system. Also describe how these changes might
be made.
alc80237_ch04_117-160.indd 155alc80237_ch04_117-160.indd 155 1/4/11 3:48 PM1/4/11 3:48 PM
Confirming Pages
156 CHAPTER 4 System Response
c. DC component + as many harmonics as you think are necessary to provide a good
reproduction of the waveform
Section 4.4 Bandwidth and Frequency Response
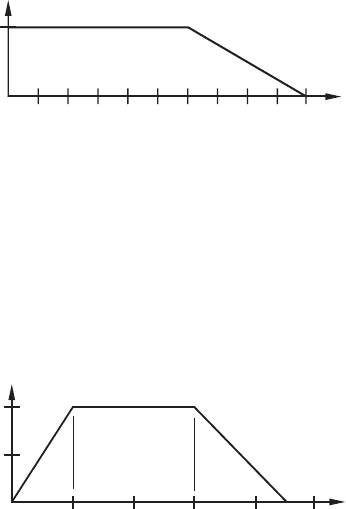
4.7. Given the following measurement system frequency response curve,
a. What is the bandwidth of the measurement system?
b. If the input signal (V
in
) is a 2 V, peak-to-peak, square wave of period 1 sec, what
will the steady state output signal (V
out
(t)) from the measurement system be? Use
the square-wave Fourier series representation given in Equation 4.15 or 4.16
for V
in
(t).
c. Plot the resulting output on a computer for t = 0 to 2 sec.
24
6
810
f (Hz)
1
A
out
/A
in
4.8. For an RC low-pass filter with R = 1 kΩ and C = 1 μF,
a. What is the bandwidth of the filter?
b. If the input is a 2 V, peak-to-peak, square wave of period 1 sec, what will the
filter output be? Use the square wave Fourier series representation given in
Equation 4.15 or 4.16 for V
in
(t).
c. Plot the resulting output on a computer for t = 0 to 2 sec.
4.9. Solve Question 4.8 with R = 100 kΩ instead.
4.10. What is the bandwidth (in Hz) of a system with the frequency response that follows?
0.5
1.0
A
out
/A
in
ω (rad/sec)1
23 4
5
4.5
4.11. If a signal is given by
F
t() nnωt()sin
n 1=
5
∑
=
where = 1 rad/sec,
a. What is the range of frequencies of the signal?
b. Plot the spectrum of F(t).
alc80237_ch04_117-160.indd 156alc80237_ch04_117-160.indd 156 1/4/11 3:48 PM1/4/11 3:48 PM
Confirming Pages
Questions and Exercises 157
c. Assuming the signal is being measured by a measurement system with the fre-
quency response curve shown in Question 4.10, plot the spectrum of
the resulting output of the system.
4.12. Determine and plot the frequency response curve for a high-pass filter (see Example 4.1).
Also derive an expression for the cutoff frequency.
4.13. Derive an expression for the phase angle between the output and input voltages of the
low-pass filter in Example 4.1 . Plot the result for frequencies between 0 and
2.5 times the cutoff frequency.
4.14. Document a complete and thorough answer to Class Discussion Item 4.2.
Section 4.6 Distortion of Signals
4.15. Using the Fourier series representation of a square wave in Equation 4.15 or 4.16,
plot a square wave using 20 harmonics. Then plot it with the first three harmon-
ics attenuated by 1/4. Finally, plot it with the first three harmonics unattenuated
and the next 17 attenuated by 1/4. What do you conclude about the influence of the
amplitudes of low and high harmonics?
4.16. Plot the frequency response curves for the exponential attenuation terms in Class
Discussion Item 4.3.
Section 4.8 Zero-Order System
4.17. A simple static spring scale is an example of a zero-order system where the input
is the mass to be measured and the output is the calibrated deflection of the spring.
Given this, what is the gain or sensitivity of the scale?
4.18. We generally assume that an oscilloscope is a zero-order measurement system. Why
is this so? When will this assumption be in error?
Section 4.9 First-Order System
4.19. Relate the time constant, cutoff frequency, and band width for the low-pass filter
circuit shown in Example 4.1 by writing a general first-order differential equation
for the circuit. What is the dependent variable in the differential equation? Write an
expression for the output voltage as a function of time V
out
(t) for a step input voltage
of amplitude A
i
. Assume the capacitor is discharged to begin with.
4.20. A simple glass bulb thermometer is an example of a first-order system where the
input is the surrounding temperature (T
in
) and the output is the temperature of the
liquid inside the bulb (T
out
), which expands to provide a reading on a scale. Using
basic heat transfer principles, where the rate of convective heat transfer in equals the
rate of change of internal energy of the fluid, derive the system equation and put it in
standard form. Identify the time constant and relate the heat transfer parameters and
fluid properties to the electrical parameters in an RC circuit (see Question 4.19). The
parameters in your equation should include the fluid mass (m) and specific heat (c),
and the bulb external area (A) and heat transfer coefficient (h).
4.21. The following set of data were collected from a system. Would it be appropriate to
assume that this system is first order? If so, find the time constant of the system.
Also, what is an approximate value for the static sensitivity?
alc80237_ch04_117-160.indd 157alc80237_ch04_117-160.indd 157 1/4/11 3:48 PM1/4/11 3:48 PM

Confirming Pages
158 CHAPTER 4 System Response
tX
out
(t)
0.0 0.0
0.1 1.4
0.2 2.3
0.3 3.0
0.4 3.6
0.5 4.1
0.6 4.2
0.7 4.6
0.8 4.7
0.9 4.8
1.0 4.9
Section 4.10 Second-Order System
4.22. Is the damped natural frequency greater or smaller than the natural frequency of a
second-order system? Explain why.
4.23. Draw a schematic and write the system equation for an example second-order system
for each of the following categories:
a. mechanical rotary
b. electrical
c. hydraulic
4.24. Use the procedure presented in Section 4.10.2 to derive the amplitude ratio and phase
relationship for a first-order system expressed in standard form. MathCAD Example
4.7 shows plots of the results.
4.25. For a spring-mass-damper system with F
ext
(t) = 20 sin(0.75t) N, m = 10 kg,
k = 12 N/m, and b = 10 Ns/m, what is the equation for the steady state sinusoidal
response of x(t)?
4.26. A cheap, mechanically actuated, strip chart recording system consisting of a plotter head
of mass m driven by an elastic belt of stiffness k is illustrated in the following figure.
k
x
in
output
m
The input displacement x
in
is coupled mechanically to the pen head through the
spring. The spring constant k is 32.4 N/m and the mass of the pen head is 0.10 kg.
Derive the differential equation of motion for the pen head, given the displacement
input. Assume that the damping constant between the pen and paper is 10 Ns/m.
Using the procedure in Section 4.10.2, determine the steady state output displacement
magnitude and phase angle for each steady state input that follows. Explain the dif-
ferences between the output and input displacements and phase angles.
MathCAD Example
4.7First-order
system frequency
response
alc80237_ch04_117-160.indd 158alc80237_ch04_117-160.indd 158 20/01/11 6:02 PM20/01/11 6:02 PM
Confirming Pages
Questions and Exercises 159
4.27. Document a complete and thorough answer to Class Discussion Item 4.5.
4.28. Derive the expression for the step response x(t) in Design Example 4.1.
4.29. Document a complete and thorough answer to Class Discussion Item 4.8.
Section 4.11 System Modeling and Analogies
4.30. Using the capacitor energy storage equation, derive the expression for the capacitance
of a cylindrical tank of diameter D and fluid height h holding bottom-fed fluid of spe-
cific weight γ.
4.31. Using the inertia energy storage equation, derive the expression for the inertance of
the fluid (of density ρ) in a straight pipe of length L and cross-sectional area A. Start
by applying F = ma to a control volume surrounding the fluid.
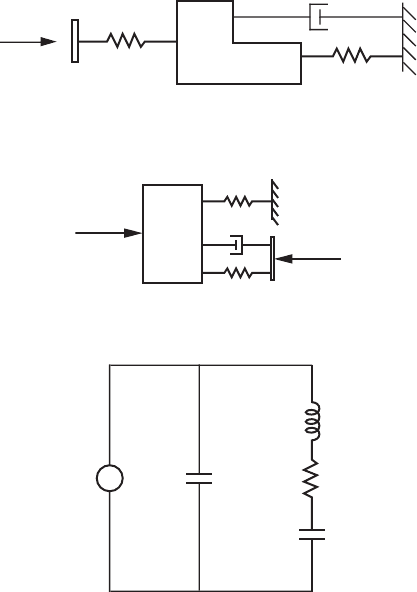
4.32. Convert the translational mechanical system that follows to an analogous electrical
system.
F
in
k
1
k
2
b
1
m
4.33. Convert the following translational mechanical system to an analogous electrical
system.
F
1
k
1
b
1
k
2
F
2
m
4.34. Convert the following electrical system to an analogous hydraulic system.
+
V
in
C
1
L
R
1
C
2
a.
b.
c.
x
in
0.05 10t()sin=
x
in
0.05 1000t()sin=
x
in
0.05 10 000t,()sin=
alc80237_ch04_117-160.indd 159alc80237_ch04_117-160.indd 159 1/4/11 3:48 PM1/4/11 3:48 PM
Confirming Pages
160 CHAPTER 4 System Response
4.35. For the translational mechanical system that follows, draw free-body diagrams for
each node, list the flow for each element, and construct (draw) an analogous electri-
cal system with all elements and flows labeled.
F
in
v
1
v
2
v
3
k
1
b
1
k
2
b
2
a
L
m
4.36. For the three-degree-of-freedom mechanical system that follows, draw free-body
diagrams for each of the components, carefully showing all external forces and
moments. Be sure to show the reference positions for the position variables on the
free-body diagrams. Directly from the component free-body diagrams, write down
the equations of motion.
m
1
k (spring)
b (dashpot)
F
1
= F cos(ωt)
coefficient of
friction
m
2
, I
2
r
y+
x+
y ref
x ref
θ
θ
ref
BIBLIOGRAPHY
Beckwith, T., Marangoni, R., and Lienhard, J., Mechanical Measurement, 5th Edition,
Addison-Wesley, Reading, MA, 1993.
Doebelin, E., Measurement Systems Application and Design, McGraw-Hill, New York, 1990.
Figliola, R. and Beasley, D., Theory and Design for Mechanical Measurements, John Wiley,
New York, 1995.
Holman, J. P., Experimental Methods for Engineers, McGraw-Hill, New York, 1994.
Ross, S., Introduction to Ordinary Differential Equations, 3rd Edition, John Wiley, New
York, 1980.
Shearer, J., Murphy, A., and Richardson, H., Introduction to System Dynamics, Addison-
Wesley, Reading, MA, 1971.
Thomson, W., Theory of Vibration with Applications, 2nd Edition, Prentice-Hall, Englewood
Cliffs, NJ, 1981.
alc80237_ch04_117-160.indd 160alc80237_ch04_117-160.indd 160 1/4/11 3:48 PM1/4/11 3:48 PM
