Zhu J. Applications of Fourier Transform to Smile Modeling: Theory and Implementation

Подождите немного. Документ загружается.

210 9 Integrating Various Stochastic Factors

We can also conveniently combine stochastic volatility and L

evy jump, such a

model is certainly of interest for practical applications due to its strong ability for

smile modeling. A particular example may be a combination of the Sch

obel-Zhu

model and the variance-gamma model, whose CFs are simply composed of the fol-

lowing ingredients,

(

)=e

+rT)

(3.32) × f

(8.16) (9.7)

and

(

)=e

+rT)

(3.36) × f

(8.17). (9.8)

By the modular pricing, more new special options pricing formulas may be con-

tinuously listed in this way. We do not deal with all of the possible models. In Ta-

ble (9.3), 108 of 256 possible models are illustrated and the corresponding option

prices are computed for different moneynesses. In a practical implementation, ev-

ery element in the CFs f

(

) can be programmed as a module or a procedure. By

assembling these modules, we can efﬁciently compute the probabilities F

in all pos-

sible option pricing models via the Fourier inversion where a step-by-step Gaussian

quadrature routine is applied. The detailed numerical techniques have already dis-

cussed in Chapter 4. Here I give again some prototype programming codes for the

modular pricing based on the codes given in Section 4.5. The strike vector integra-

tion is implemented in codes to calculate a range of option prices.

\\This function implements the exponents of CFs

\\of stochastic volatility models.

Complex CF_Vola(int index, double phi, ProcessType type)

{

....

}

\\This function implements the exponents of CFs

\\of stochastic interest models.

protected Complex CF_Rate(int index, double phi, ProcessType type)

{

....

}

\\This function implements the exponents of CFs of Poisson jumps.

Complex CF_Poisson(int index, double phi, JumpType type)

{

....

}

\\This function implements the exponents of CFs of Poisson jumps.

Complex CF_Levy(int index, double phi, LevyType type)

{

....

}

//This function performs strike vector integration, two CFs version

double[] CFs(int index, double phi, double[] strikes)

{

//If index is 0, we go to one CF version

if ( index = 0 )

return CFs(phi, strikes);

Complex value = new Complex(0);

9.2 Integration Approaches 211

value = CF_Vola(index, phi) + CF_Rate(index, phi)

+ CF_Poisson(index, phi) + CF_Levy(index, phi);

double[] x = new double[strikes.Length];

Complex y, v;

for (int i = 0; i < strikes.Length; i++)

{

y = new Complex(0, Log(spot / strikes[i])

phi, 0);

v = value + y;

x[i] = Exp(v.Real)

Sin(v.Imaginary) / phi;

}

return x;

}

//This function performs strike vector integrations, one CF version

//according to Attari’s formulation

double[] CFs(double phi, double[] strikes)

{

Complex value= new Complex(0);

double r = interestRate;

Complex lns = new Complex(0, ( Log(spot)+ r

phi);

// Note Attari formulation is not valid for stochastic interest rate

// CF_Rate is not integrated

value = CF_Vola(2, phi) + CF_Jump(2, phi) + CF_Levy(2, phi);

value = value.Exp();

double real = value.Real;

double imag = value.Imaginary;

double[] x = new double[strikes.Length];

double beta, v;

for (int i = 0; i < strikes.Length; i++)

{

beta = Log( Exp(-r

strikes[i] / spot)

phi;

v = (real + imag / phi)

Cos(beta) + (imag - real / phi)

Sin(beta);

x[i] = v/(1+phi

phi);

}

return x;

}

The option prices of the various models given in Table (9.3) are computed with

the parameters given in Table (9.2), and serve as a theoretical illustration only. We

choose the values of model parameters so that all ATM option prices are close to

each other as possible. Keeping ATM option prices at same level, we can easily

conclude which models are able generate more skewness than the Black-Scholes

model. For example, both the Heston model encoded as (SR ×No×No×No) and

the Sch

obel-Zhu model encoded as (OU ×No×No×No) with negative correlation

produce the higher prices for ITM options and the lower prices for OTM options

than the Black-Scholes model, this indicates that both stochastic volatility models

can generate a down-sloping smile. The alpha log-stable model of Carr and Wu

(2003a) is labeled as (BS×No×No×ALS), and exhibits the similar price pattern as

stochastic volatility models due to its maximal pre-setting of skewness parameter.

Not surprisingly, adding additional stochastic factor to the Black-Scholes model

produces higher prices than the pure Black-Scholes prices, for example, a model

with constant volatility and the variance-gamma encoded as (BS×No×No×VG).

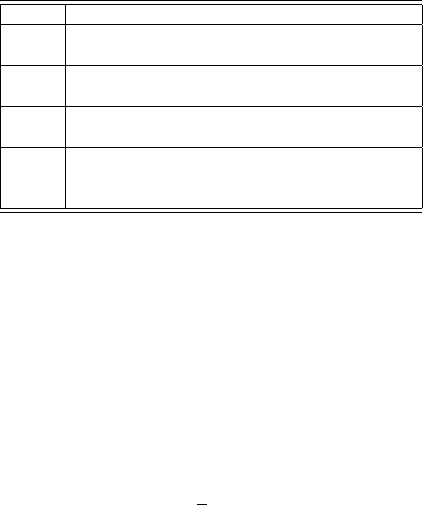
212 9 Integrating Various Stochastic Factors

S = 100 T = 1 r

=0.05 v

=0.2

SV:

=0.04

=0.1

=-0.5

=0.195

=0.1

=-0.5

SI:

=0.05

=0.1

=0.05

=0.1

Poisson:

LogN

=0.2

LogN

=0.15

LogN

=0.05

Paret o1

=0.5

Paret o1

=0.2

Paret o2

=0.3

Paret o2

=0.25

evy:

=0.1

=0.15

=0.05

NIG

=0.2

NIG

=0.15

ALS

=1.8

ALS

=0.03

Table 9.2 Parameter values for the selected models. The theoretical values of options are given in

Table 9.3.

9.2.2 Time-Change Approach

Huang and Wu (2004) proposed a time-changed L

evy process to unify some option

pricing models, in order to examine the relative performance of jump component

and diffusion component in capturing volatility smile. In details, their formulation

for the underlying return process takes the following form,

x(t)=x

+ rt +



(t)

−

(t)





(t)

−mY

(t)



, (9.9)

where the volatility v and the interest rate r are assumed to be constant, W(t) and

L(t) denote a standard Brownian motion and a compensated pure L

evy jump pro-

cess respectively. Following the time-change approach to L

evy process in Section

8.3, Huang and Wu applied two subordinators Y

(t) and Y

(t) to time-change the

Brownian motion W(t) and the pure jump process L(t), respectively.

As discussed in Section 8.3, stochastic volatility models, particularly, the Heston

model, may be created via applying stochastic time change to Brownian motion,

and the most L

evy models in ﬁnancial literature are resulted from stochastic time

change, with or without leverage effect. In this sense, the time-change approach is a

feasible method to unify the most option pricing models in this book and in the vast

ﬁnancial literature. So far, as shown in Chapter 8 and the assumption here, stochastic

interest rates are never taken into account in the time-change approach. However,

with some slight extensions, we may incorporate independent stochastic interest

rates discussed in Chapter 6 into the process in (9.9). For discussion simplicity, we

neglect stochastic interest rates here.

Similarly to the procedure in Section 8.3, the generalized Fourier transform is

given by

9.2 Integration Approaches 213

SV SI Poisson L

evy K=80 K=90 K=95 K=100 K=105 K=110 K=120

BS No No No 24.59 16.70 13.35 10.45 8.02 6.04 3.25

SR No No No 24.69 16.80 13.41 10.47 7.98 5.95 3.09

OU No No No 24.78 16.88 13.46 10.47 7.93 5.84 2.92

BS SR No No 24.98 17.07 13.69 10.76 8.29 6.27 3.40

SR SR No No 25.07 17.17 13.76 10.78 8.26 6.18 3.24

OU SR No No 25.17 17.25 13.81 10.79 8.21 6.08 3.08

BS OU No No 24.98 17.10 13.73 10.82 8.36 6.34 3.46

SR OU No No 25.07 17.19 13.80 10.84 8.33 6.26 3.31

OU OU No No 25.17 17.28 13.85 10.84 8.28 6.16 3.15

BS No LogN No 24.70 16.95 13.67 10.84 8.45 6.50 3.69

SR No LogN No 24.80 17.03 13.72 10.84 8.41 6.41 3.55

OU No LogN No 24.89 17.11 13.76 10.83 8.35 6.31 3.40

BS SR LogN No 25.08 17.31 14.00 11.14 8.72 6.72 3.85

SR SR LogN No 25.18 17.40 14.06 11.15 8.68 6.64 3.71

OU SR LogN No 25.27 17.47 14.10 11.14 8.62 6.54 3.56

BS OU LogN No 25.09 17.34 14.05 11.19 8.78 6.79 3.91

SR OU LogN No 25.18 17.42 14.10 11.20 8.74 6.71 3.77

OU OU LogN No 25.27 17.50 14.14 11.20 8.69 6.61 3.62

BS No Pareto No 24.68 16.91 13.62 10.77 8.37 6.39 3.55

SR No Pareto No 24.78 16.99 13.67 10.77 8.32 6.31 3.41

OU No Pareto No 24.87 17.07 13.70 10.76 8.26 6.20 3.25

BS SR Pareto No 25.07 17.27 13.95 11.07 8.63 6.62 3.70

SR SR Pareto No 25.16 17.36 14.01 11.08 8.60 6.54 3.56

OU SR Pareto No 25.25 17.43 14.05 11.07 8.54 6.44 3.41

BS OU Pareto No 25.07 17.30 14.00 11.13 8.70 6.69 3.77

SR OU Pareto No 25.25 17.39 14.05 11.14 8.66 6.61 3.63

OU OU Pareto No 25.25 17.46 14.09 11.13 8.60 6.51 3.48

BS No No VG 24.74 17.01 13.73 10.88 8.49 6.51 3.67

SR No No VG 24.84 17.09 13.78 10.89 8.45 6.43 3.53

OU No No VG 24.93 17.16 13.81 10.89 8.39 6.33 3.38

BS SR No VG 25.13 17.37 14.06 11.19 8.75 6.74 3.82

SR SR No VG 25.22 17.45 14.12 11.20 8.72 6.66 3.69

OU SR No VG 25.30 17.53 14.16 11.20 8.67 6.57 3.54

BS OU No VG 25.13 17.40 14.11 11.24 8.81 6.80 3.88

SR OU No VG 25.22 17.48 14.16 11.26 8.78 6.73 3.75

OU OU No VG 25.30 17.55 14.20 11.25 8.73 6.64 3.61

BS No LogN VG 24.87 17.26 14.04 11.26 8.90 6.94 4.09

SR No LogN VG 24.95 17.33 14.08 11.26 8.86 6.87 3.96

OU No LogN VG 25.04 17.39 14.11 11.24 8.80 6.77 3.83

BS SR LogN VG 25.24 17.61 14.37 11.55 9.16 7.17 4.25

SR SR LogN VG 25.33 17.69 14.42 11.56 9.12 7.10 4.12

OU SR LogN VG 25.41 17.75 14.45 11.55 9.07 7.01 3.99

BS OU LogN VG 25.25 17.64 14.42 11.61 9.22 7.23 4.31

SR OU LogN VG 25.33 17.71 14.46 11.61 9.18 7.16 4.19

OU OU LogN VG 25.41 17.77 14.49 11.60 9.13 7.07 4.06

BS No Pareto VG 24.85 17.22 13.99 11.19 8.81 6.84 3.94

SR No Pareto VG 24.93 17.29 14.03 11.19 8.77 6.76 3.82

OU No Pareto VG 25.02 17.35 14.05 11.17 8.71 6.67 3.68

BS SR Pareto VG 25.22 17.57 14.32 11.49 9.08 7.07 4.10

SR SR Pareto VG 25.31 17.65 14.36 11.49 9.04 6.99 3.98

OU SR Pareto VG 25.39 17.71 14.39 11.48 8.99 6.90 3.85

BS OU Pareto VG 25.23 17.60 14.36 11.54 9.13 7.13 4.16

SR OU Pareto VG 25.31 17.67 14.40 11.54 9.10 7.06 4.04

OU OU Pareto VG 25.39 17.73 14.43 11.53 9.05 6.97 3.91

continuing in the next page

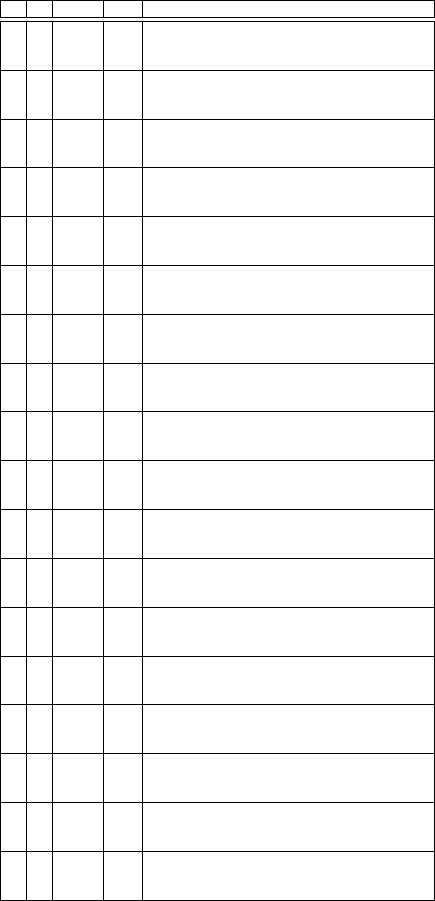
214 9 Integrating Various Stochastic Factors

continued from the previous page

SV SI Poisson L

evy K=80 K=90 K=95 K=100 K=105 K=110 K=120

BS No No NIG 24.75 17.02 13.73 10.89 8.49 6.51 3.66

SR No No NIG 24.84 17.10 13.79 10.90 8.45 6.43 3.53

OU No No NIG 24.93 17.17 13.82 10.89 8.40 6.33 3.38

BS SR No NIG 25.13 17.38 14.07 11.19 8.75 6.74 3.82

SR SR No NIG 25.22 17.46 14.12 11.21 8.72 6.66 3.68

OU SR No NIG 25.31 17.53 14.16 11.21 8.68 6.57 3.54

BS OU No NIG 25.13 17.41 14.11 11.25 8.82 6.80 3.88

SR OU No NIG 25.22 17.49 14.17 11.26 8.79 6.73 3.75

OU OU No NIG 25.31 17.56 14.20 11.26 8.74 6.64 3.61

BS No LogN NIG 24.87 17.27 14.05 11.26 8.90 6.94 4.09

SR No LogN NIG 24.96 17.34 14.09 11.26 8.86 6.87 3.96

OU No LogN NIG 25.04 17.40 14.12 11.25 8.80 6.77 3.83

BS SR LogN NIG 25.25 17.62 14.38 11.56 9.16 7.17 4.25

SR SR LogN NIG 25.34 17.69 14.42 11.56 9.13 7.10 4.12

OU SR LogN NIG 25.42 17.76 14.45 11.55 9.08 7.01 3.99

BS OU LogN NIG 25.25 17.65 14.42 11.61 9.22 7.23 4.31

SR OU LogN NIG 25.34 17.72 14.46 11.62 9.19 7.16 4.18

OU OU LogN NIG 25.42 17.78 14.49 11.61 9.14 7.07 4.05

BS No Pareto NIG 24.85 17.23 13.99 11.19 8.81 6.84 3.94

SR No Pareto NIG 24.94 17.30 14.03 11.19 8.77 6.76 3.81

OU No Pareto NIG 25.02 17.36 14.06 11.18 8.72 6.67 3.68

BS SR Pareto NIG 25.23 17.58 14.32 11.49 9.08 7.07 4.10

SR SR Pareto NIG 25.32 17.65 14.37 11.50 9.04 6.99 3.98

OU SR Pareto NIG 25.40 17.72 14.40 11.49 8.99 6.91 3.85

BS OU Pareto NIG 25.23 17.61 14.37 11.54 9.14 7.13 4.16

SR OU Pareto NIG 25.32 17.68 14.41 11.55 9.10 7.06 4.04

OU OU Pareto NIG 25.40 17.74 14.44 11.54 9.05 6.97 3.91

BS No No ALS 25.91 17.69 14.10 10.94 8.24 5.98 2.69

SR No No ALS 26.00 17.80 14.19 10.99 8.23 5.92 2.54

OU No No ALS 26.09 17.90 14.26 11.02 8.21 5.84 2.38

BS SR No ALS 26.30 18.08 14.47 11.28 8.54 6.24 2.87

SR SR No ALS 26.39 18.19 14.56 11.34 8.54 6.19 2.73

OU SR No ALS 26.49 18.29 14.64 11.37 8.52 6.11 2.57

BS OU No ALS 26.30 18.10 14.51 11.34 8.60 6.31 2.94

SR OU No ALS 26.39 18.21 14.60 11.39 8.60 6.26 2.80

OU OU No ALS 26.48 18.30 14.67 11.42 8.59 6.19 2.65

BS No LogN ALS 26.00 17.91 14.40 11.31 8.66 6.44 3.16

SR No LogN ALS 26.09 18.00 14.47 11.34 8.64 6.37 3.02

OU No LogN ALS 26.18 18.09 14.53 11.36 8.61 6.29 2.88

BS SR LogN ALS 26.39 18.29 14.76 11.64 8.95 6.69 3.34

SR SR LogN ALS 26.48 18.39 14.83 11.68 8.94 6.64 3.21

OU SR LogN ALS 26.57 18.47 14.90 11.70 8.92 6.56 3.07

BS OU LogN ALS 26.39 18.31 14.80 11.69 9.01 6.76 3.41

SR OU LogN ALS 26.47 18.41 14.87 11.73 9.00 6.70 3.28

OU OU LogN ALS 26.56 18.49 14.93 11.75 8.98 6.63 3.14

BS No Pareto ALS 25.98 17.87 14.35 11.24 8.58 6.34 3.02

SR No Pareto ALS 26.07 17.97 14.42 11.28 8.56 6.28 2.89

OU No Pareto ALS 26.16 18.06 14.48 11.30 8.53 6.20 2.74

BS SR Pareto ALS 26.38 18.26 14.71 11.58 8.88 6.60 3.21

SR SR Pareto ALS 26.47 18.35 14.79 11.62 8.87 6.54 3.08

OU SR Pareto ALS 26.55 18.44 14.85 11.64 8.84 6.47 2.94

BS OU Pareto ALS 26.37 18.28 14.75 11.63 8.94 6.67 3.28

SR OU Pareto ALS 26.46 18.37 14.82 11.67 8.93 6.61 3.15

OU OU Pareto ALS 26.55 18.46 14.88 11.69 8.90 6.54 3.01

Table 9.3 Theoretical values of European options in 108 different models by applying the modular

approach.

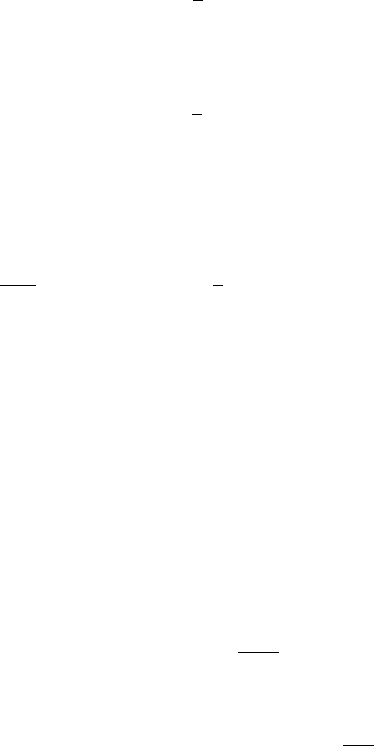
9.2 Integration Approaches 215

f (

)=E

x(t)

]

= e

+rT)i

× E



exp



[vW

(t)

−

(t)] + i

(t)

−mY

(t)]



= e

+rT)i

−

∗

(

(t)−

∗

(

(t)

, (9.10)

with

∗

(

and

∗

(

) is the characteristic exponent whose form depends on the concrete spec-

iﬁcation of the pure L

evy process L(t).

The complex-valued Radon-Nikodym derivative changing the risk-neutral mea-

sure Q to the leverage-neutral measure Q

is deﬁned by

= exp



[vW

(t)

−

(t)] +

∗

(

(t)

+ i

(t)

−mY

(t)] +

∗

(

(t)



. (9.11)

Now we show how to incorporate the various stochastic factors into a single

valuation framework by the time-change approach, and check three cases: stochastic

volatilities, Poisson jumps and L

evy jumps.

Stochastic Volatilities:

1. The Black-Scholes model:

(t)=1, Y

(t)=t.

2. The Heston model:

(t)=





(s)ds,

where y

(t) follows under Q

a mean-reverting square root process as follows

(t)=[

κθ

−

(t)+i

φρσ

(t)]dt +



y(t)dW

(t).

3. The Sch

obel and Zhu model: In this case, a strictly positive stochastic time can

not be deﬁned. But if we relax the strictly positive condition for stochastic time,

the technique of complex-valued measure is still applicable.

(t)=



(s)ds,

where y

(t) follows under Q

a mean-reverting Ornstein-Uhlenbeck process as

follows

216 9 Integrating Various Stochastic Factors

(t)=[

κθ

−

(t)+i

φρσ

(t)]dt +

(t).

4. The double square root model: This is similar to the Heston model.

(t)=





(s)ds,

where y

(t) follows under Q

a mean-reverting double square root process as

follows

(t)=[

−



(t)+(i

φρσ

−

(t)]dt +



y(t)dW

(t).

Poisson Jumps:

If the L

evy process L(t) itself is a compound jump process, the stochastic time-

change Y

(t) is trivial,

(t)=1, Y

(t)=t.

evy Jumps:

If the L

evy process L(t) is speciﬁed as a L

evy process with inﬁnite activity, the

stochastic time-change is not necessary,

(t)=1, Y

(t)=t.

However, if L(t) is speciﬁed as a Brownian motion, we can time-change L(t) with

gamma process or inverse Gaussian process to obtain L

evy jumps, as discussed in

Section 8.2. In this case, we have

(t)=



(s)ds,

where y

(t) is a gamma process or an inverse Gaussian process.

Time-changed L

evy Jumps:

This case is essentially identical to the one discussed in Section 8.3. If the stochastic

time change Y

(t) is not correlated with L(t), any subordinator Y

(t) may be applied

to L(t). If there is a dependence between Y

(t) and L(t), they should be of the same

type of L

evy process.

Summing up, although we can embrace different pricing models into a single

framework in (9.9) by the time-change approach, but it is only of theoretical consid-

eration, and lacks a structural transparency and clear instructions to implementation.

9.3 Pricing Kernels for Options and Bonds 217

9.3 Pricing Kernels for Options and Bonds

In the previous chapters, we have ampliﬁed how to incorporate stochastic factors,

especially SV and SI, into a comprehensive option pricing model. To some surprise,

similar functions are repeatedly used in computing CFs as long as SV and SI are

speciﬁed to follow a same stochastic process. More interestingly, these functions

display the same structure as the zero-coupon bond pricing formulas. This indicates

that the pricing kernels for options are connected with the pricing kernels for bonds.

Additionally, as shown in Carr and Wu (2002), time-change approach in fact applies

also the zero-coupon bond pricing analogy to compute the CF under the so-called

leverage-neutral measure. To see this, we summarize the similarity between these

two kernels case by case:

1. Mean-reverting square-root process z(t):

The key function in pricing both options and bonds is

y(z

,T)=E



exp



−s



z(t)dt + s

z(T)



. (9.12)

Setting s

= 1 and s

= 0 yields the CIR (1985) bond pricing formula while

y(z

,T) plays a central role in deriving the CFs for options in the Heston model,

as shown in Section 3.2 and Section 6.2.

2. Mean-reverting Ornstein-Uhlenbeck process z(t):

In this case, the following function is crucial for pricing options and bonds:

y(z

,T)=E



exp



−s



(t)dt −s



z(t)dt + s

(T)



. (9.13)

Once again, setting s

= 0, s

= 1 and s

= 0 reduces y(z

,T) to the well-known

bond pricing formula of Vasicek (1977) while y(z

,T) is a key function to arrive

at a closed-form solution for CFs in the option pricing formula as in Sch

obel and

Zhu (1999).

3. Mean-reverting double square root process z(t):

In this case, we have a function that takes the following form:

y(z

,T)=E



exp



−s



z(t)dt −s





z(t)dt + s

z(T)



. (9.14)

We obtain the Longstaff (1989) bond pricing formula by setting s

= 1, s

= 0

and s

= 0, and the closed-form solution for CFs and options by using the general

formula of y(z

,T).

All three functions y(z

,T) correspond to a PDE in accordance with the Feynman-

Kac formula and are available analytically. This makes the modular pricing feasible.

While zero-coupon bond prices naturally are the expected value of the exponential

function of r(t) (here, z(t)) by the argument of the local expectations hypothesis,

the CFs also are the expected value of the exponential function of r(t) or v(t) by

218 9 Integrating Various Stochastic Factors

Fourier transform. In the light of spanning security market, the pricing kernels for

bonds and options are nested in different spanned market spaces. While the pric-

ing kernels for bonds with an exponential form are located in the original market

space, the pricing kernels for options with the function y(z

,T) are generated in the

transformed space. Both spaces are interchangeable by applying simple operators

such as differentiation or translation (see Bakshi and Madan, 2000). The last step in

option pricing in our new framework is the Fourier inversion which transports the

valuation of options from the transformed space into the original one. Thus, at the

end of whole pricing procedure, option prices return to the market space spanned by

underlying assets, and options are valued in the original market spaces.

Regardless of whether in the original market space or in the Fourier transformed

one, Kolmolgov’s backward equation works, this means, the probabilities F

and

the corresponding CFs satisfy the same backward equation. This conjunction can be

seen in Section 2.2, and establishes the mathematical basis for the identical pricing

kernel for options and bonds. Thus, it is no longer surprising that as long as we can

obtain a closed-form formula for a zero-coupon bond by applying a particular pro-

cess, we can also obtain a closed-form formula for options in a stochastic volatility

model by applying the same process.

9.4 Criterions for Model Choice

In this chapter, we have discussed how to efﬁciently and ﬂexibly build up a large

number of option pricing models by combining different stochastic factors. To show

the power of the modular approach, 256 possible variants of option pricing models

are given by 4 alternative volatility factors, 4 alternative interest rate factors, 4 alter-

native Poisson jumps and 4 alternative L

evy jumps. Of course, adding new speciﬁ-

cation for each factor creates new potential pricing models. Two natural questions

now are: (1) which factor is more important for option valuation? (2) what condi-

tions shall a good pricing model satisfy? These two questions are related, and in

general, the second question is more extensive than the ﬁrst one.

The ﬁrst question is also referred to as the speciﬁcation problem of option pric-

ing models, and is intensively addressed in ﬁnancial literature. Speciﬁcation issue

is examined mostly with European-style options. For example, Bates (1996), Bak-

shi, Cao and Chen (1997) examined the role of stochastic volatility and Poisson

jumps, and found that stochastic volatility is the most important factor in explain-

ing the smile effect, and however, adding Poisson jumps improves the performance

remarkably. Recently, some studies including Carr, Geman, Madan and Yor (2001),

Carr and Wu (2003a), Huang and Wu (2004) documented the need for L

evy jumps,

namely inﬁnite activity jumps, in order to capture the behavior of equity index op-

tions. Most of these models are included in Table (9.3). Distinguishing a key factor

from four stochastic factors is essentially an issue of empirical tests.

Now we pay more attentions on the second question from the point of view of

practical applications. Generally, it is difﬁcult and even impossible to choose the

9.4 Criterions for Model Choice 219

best model from so a large variety of models. However, if we apply an option pricing

model to a real trading environment, we will naturally raise some requirements and

expectations that a pricing model should satisfy. As a practitioner, I summarize the

following points for an applicable pricing model.

1. Analytical Tractability: Analytical tractability is the ﬁrst of all requirements that

a pricing model should fulﬁll. A pricing model must admit an (semi-) analyti-

cal closed-form valuation for European-style options. It is not only used to value

European-style options quickly and accurately, but also, more importantly, al-

lows an efﬁcient calibration of the model to market prices, in the most cases, the

volatility surfaces. Without a closed-form solution for options, any pricing model

does not have a chance to be applied in practice.

2. Fitting to Market Prices: A good model shall and can be ﬁtted consistently to

market quoted prices. Since most liquid plain-vanilla options are valued by the

Black-Scholes (-like) models and quoted with implied volatilities, ﬁtting to mar-

ket prices is in most cases equivalent to ﬁtting to volatility smile. From the point

of view of practitioner, there are two aspects for ﬁtting. Firstly, a model should

be calibrated efﬁciently and accurately to whole smile surface, instead to a smile

at one maturity only. This requires that a model can recover not only the long-

term smile pattern, but also the short-term smile patten. Secondly, a good model

should be ﬂexible enough to capture different smile pattern, namely, symmetric,

down-sloping and even up-sloping smile. Table (9.4) gives the market FX volatil-

ities of USD/EUR, GBP/EUR and JPY/EUR, as of February 10. 2009. We can

easily observe that FX volatility surface of each foreign exchange rate presents

different smile pattern: While the volatility smile surface of USD/EUR is nearly

symmetric, the smiles (sneers) of GBP/EUR and JPY/EUR slope up and down

respectively. In these cases, the models that can only generate the decreasing or

increasing skewness, can not recover all FX option markets. According to my

experience, stochastic volatility models are the only model class that can deal

with all three smile patterns ﬁrmly.

3. Hedging Performance: Hedging performance is an other important criterion to

test a pricing model. Any pricing model delivers Delta and Gamma, but not

necessarily provides Vega since volatility risk is replaced by other risks of pa-

rameters capturing volatility smile. For example, the variance-gamma model ex-

presses volatility risk via the parameters

. Whether a pricing model pro-

vides a better and more robust hedge performance than the Black-Scholes model,

is an issue of statistical testing and practical application. Usually, we may use out-

of-sample or in-the-sample empirical testings for check hedging performance.

However, there is a consensus that a good hedging performance is conditional on

a good ﬁtting to smile surface.

4. Convenient Simulation: Good ﬁtting to market is the minimal requirement for

an applicable model, and a perfect recovery of smile is not our initial goal. The

most sophisticated models are used to value and hedge exotic derivatives and

structures in accordance with volatility smile. However, the advanced models

generally do not admit the analytical solutions for most exotic options. As a

result, Monte-Carlo simulation is most time the only way to value exotic options.