Weinan E. Principles of Multiscale Modeling

Подождите немного. Документ загружается.

2.4. MULTISCALE EXPANSIONS 67

In one dimension, if we assume that c never vanishes, we have

∂

v + p =

H(p)

c(y)

, (2.4.61)

since the right hand side does not change sign. Integrating both sides, we arrive at

H(p) =



c(y)



−1

|p|. (2.4.62)

If c(y) vanishes at some point, th en clearly

H(p) = 0.

2.4.4 Flow in porous media

Next, we consider the homogenization of ﬂows in a porous medium assuming that

the micro-structure of the medium is periodic. Let Ω

be the ﬂow domain, and Ω

the solid matrix. Ω

= ε ∪

z∈Z

(Ω + z), the union of the periodic extension of Ω rescaled

by a factor of ε. In principle, one should start with the Navier-Stokes equations in the

ﬂow domain with no-slip boundary condition at ∂Ω

, and then study the asymptotics

as ε → 0. However, since the velocity is small, we can omit the dynamic terms in the

Navier-Stokes equation and use the Stokes equation

− ν∆u

+ ∇p

= 0 in Ω

, (2.4.63)

∇ · u

= 0 in Ω

, (2.4.64)

= 0 on ∂Ω

. (2.4.65)

To study the asymptotic behavior, we make the ansatz

(x) ∼ ε

(x, x/ε) + ε

(x, x/ε) + ··· ,

(x) ∼ p

(x, x/ε) + εp

(x, x/ε) + ··· .

The leading order equation is ∇

= 0, hence p

= p

(x) is independent of y. The next

order equation is

− ν∆

+ ∇

= −∇

in Ω, (2.4.66)

∇

= 0 in Ω, (2.4.67)

= 0 on ∂Ω. (2.4.68)

68 CHAPTER 2. ANALYTICAL METHODS

Let (χ

, q

), j = 1, 2, be the solution of

− ν∆

+ ∇

= e

in Ω,

∇

= 0 in Ω,

= 0 on ∂Ω,

where e

= (1, 0)

and e

= (0, 1)

. Given ∇

, the solution to the next order equation

is given by u

(x, y) = −(χ

, χ

)∇

. The average velocity is

¯u(x) = −



Ω

(χ

, χ

) dy



∇

(x). (2.4.69)

This is Darcy’s law for ﬂows in porous medium. K =

(χ

, χ

) dy is the eﬀective

permeability tensor.

2.5 Scaling and self-similar solutions

So far we have focused on problems with disparate scales and we have discussed

techniques that can be used to eliminate the small scales and obtain simpliﬁed eﬀective

models for the large scale behavior of the problem. Another class of problems for which

simpliﬁed models can be obtained are problems that exhibit self-similar behavior. We will

begin with a discussion of the self-similar solutions in this section. In the next section,

we turn into the renormalization group method which is a systematic way of extracting

eﬀective models in systems that exhibit self-similar behavior at the small scales.

2.5.1 Dimensional analysis

Dimensional analysis is a very simple and elegant tool for getting a quick guess about

the important features of a problem. The most impressive example of dimensional analy-

sis is Kolmogorov’s prediction about the small scale structure in a fully developed tu rbu -

lent ﬂow. The quantity of interest there is the (kinetic) energy density at wave number

k, denoted as E(k). E(k) behaves diﬀerently in three diﬀerent regimes. In the large

scale regime, i.e., scales comparable to the size of the system, the behavior of E(k) is

non-universal and depends on how energy is supplied to the system, the geometry of the

system, etc. In the dissipation regime, viscous eﬀects dominates. Between the large scale

2.5. SCALING AND SELF-SIMILAR SOLUTIONS 69

and the dissipation regimes lies the so-called inertial regime. In this regime, the con-

ventional picture is that energy is simply transported from large to small scales, without

been dissipated. It is widely expected and partly conﬁrmed by experimental results [?, ?]

that the behavior of turbulent ﬂows in this inertial range is universal and exhibits some

kind of self-similarity.

To ﬁnd the self-similar behavior, Kolmogorov made the basic assumption that the

only important quantity for the inertial range is the mean energy dissipation rate:

¯ε = ν



|∇u|



where ν is the dynamic viscosity, the bracket denotes the ensemble average of the quantity

inside. To ﬁnd the behavior of E(k), which is assumed to depend on k and ¯ε only, let us

look at the dimensions of the quantities E(k), k and ¯ε. We will use L to denote length,

T to denote time, and [·] to denote the dimension of the quantity inside the bracket.

Obviously, [k] = 1/L. Since [

E(k) dk] = L

, we have [E(k)]/L = L

. Hence

[E(k)] = L

. Also,

[¯ε] = [ν][∇u]

= L

/T · (1/T )

= L

Assume that E(k) = ¯ε

. It is easy to see that to be dimensionally consistent, we must

have α = 2/3 and β = −5/3. Therefore, we conclude that

E(k) ∝ ¯ε

2/3

−5/3

Experimental results are remarkably close to the behavior predicted by this simple rela-

tion. However, as was pointed out by Landau, it is unlikely that this is the real story:

The dissipation ﬁeld ν|∇u|

is a very intermittent quantity. Its contribution to the ﬂow

ﬁeld is dominated by very intense events in very small part of the whole physical domain.

Therefore we do not expect the mean-ﬁeld picture put forward by Kolmogorov to be

entirely accurate. Indeed there are evidences suggesting that the small scale structure in

a turbulent ﬂow exhibits multi-fractal statistics:

h|u(x + r) − u(x)|

i ∼ |r|

where ζ

is a nonlinear function of p. In contrast, Kolmogorov’s argument would give

p. To this day, ﬁnding ζ

still remains to be one of the main challenges of turbulence

theory. Many phenomenological models have been proposed, but ﬁrst-principle-based (i.e.

Navier-Stokes equation) understanding is still lacking [?].

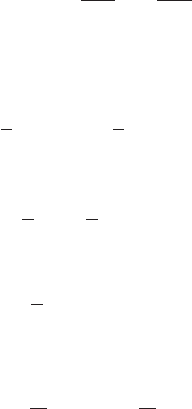
70 CHAPTER 2. ANALYTICAL METHODS

2.5.2 Self-similar solutions of PDEs

Consider the Barenblatt equation that arises from modeling gas ﬂow in porous medium

[?]:

∂

u = ∂

(2.5.1)

with initial condition u(x, 0) = δ(x). We look for a self-similar solution that satisﬁes the

relation

u(x, t) = λ

u(λx, λ

t). (2.5.2)

for all λ > 0. At t = 0, we have

δ(x) = λ

δ(λx).

Therefore α = 1. Substituting the ansatz (2.5.2) into the PDE (2.5.1), we obtain:

β+1

∂

u = λ

∂

Hence β = 3. This means that

u(x, t) = λu(λx, λ

t) =

1/3



1/3



, (2.5.3)

where U(y) = u(y, 1) and we have used λ = 1/t

1/3

. The original PDE for u now becomes

an ODE for U:

−4/3

)

′′

= −

−4/3

U −

−5/3

′

(2.5.4)

or, if we denote y = x/t

1/3

)

′′

= −

U −

′

. (2.5.5)

Integrating once, we get

)

′

= −

yU + C. (2.5.6)

As y → ±∞, we expect U → 0. Hence C = 0. Integrating one more time, we get

U(y) =







−

, U

;

0, otherwise.

(2.5.7)

The constant U

can be determined by the conservation of mass:

U(y) dy =

u(y, 1) dy = 1. (2.5.8)

2.5. SCALING AND SELF-SIMILAR SOLUTIONS 71

−5 −4 −3 −2 −1 0 1 2 3 4 5

−0.1

0.1

0.2

0.3

0.4

Figure 2.4: The proﬁle of the solution to the Barenblatt equation at t = 1.

This gives U

= 3

1/3

/4. The solution is shown in Figure 2.4. What is interesting about

this solution is that it has ﬁnite speed of propagation. This is possible due to the

degeneracy of the diﬀusion term, and is consistent with our intuition about gas ﬂow in

porous medium.

Next, we study an example that models viscous rarefaction wave:

∂

u + u

∂

u = ∂

u, (2.5.9)

with initial condition

u(x, 0) =







0, x ≤ 0

1 x > 0

(2.5.10)

It is impossible to ﬁnd a self-similar solution to this problem. In fact, we will show that

the solution has three diﬀerent regimes.

Let us ﬁrst study the eﬀect of the diﬀusion and convection terms seperately. Keeping

only the diﬀusion term, (2.5.9) becomes

∂

u = ∂

u. (2.5.11)

For this problem with the same initial condition (2.5.10), we can ﬁnd a self-similar solu-

tion. Assume that

u(x, t) = λ

u(λx, λ

t).

Since the initial condition is invariant under scaling, α = 0. Substituting into (2.5.11)

gives β = 2. Therefore,

u(x, t) = u(λx, λ

t) = U



1/2



72 CHAPTER 2. ANALYTICAL METHODS

where U(y) = u(y, 1). Moreover, U satisﬁes the ODE

′′

= −

′

. (2.5.12)

The solution to this equation with the prescribed initial condition is given by

U(y) =

√

−∞

−z

dz. (2.5.13)

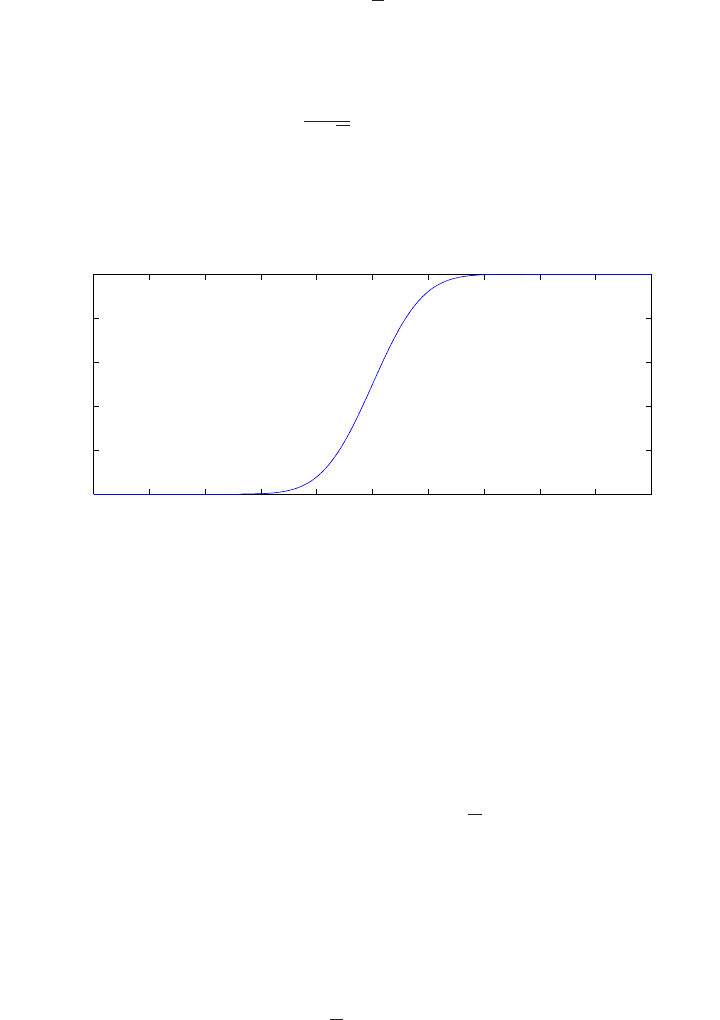
As shown in Figure 2.5, the eﬀect of the diﬀu sion term is to smear out the discontinuity

in the initial data.

−10 −8 −6 −4 −2 0 2 4 6 8 10

0.2

0.4

0.6

0.8

Figure 2.5: The proﬁle of the solution to (2.5.11) at t = 1.

If we keep only the convection term, then (2.5.9) becomes

∂

u + u

∂

u = 0. (2.5.14)

By the same argument as before, we ﬁnd a self-similar solution that satisﬁes

u(x, t) = u(λx, λt) = U





. (2.5.15)

U satisﬁes the equation

−yU

′

+ U

′

= 0. (2.5.16)

The solution is

U =











0, y ≤ 0,

√

y, 0 ≤ y ≤ 1,

1, y ≥ 1

(2.5.17)

2.6. RENORMALIZATION GROUP ANALYSIS 73

Going b ack to the original problem, it is easy to see that for small t, diﬀusion dom-

inates, and for large t, convection dominates. This can also be shown by a scaling

argument. Assume

u(x, t) = u(λx, λ

Substituting into the original PDE, we get

∂

u + λu

∂

u = λ

∂

u. (2.5.18)

When λ ≪ 1, corresponding to large time, the term λu

is more important, hence the

convective behavior is dominant. When λ ≫ 1, corresponding to small time, the viscous

behavior dominates.

What happens at the intermediate times? Using the ansatz:

u(x, t) = λ

u(λx, λ

t),

we get α = 1/2 and β = 2 by balancing both the convective and diﬀusive terms. Hence

u(x, t) ∼

1/4



1/2



As before, we obtain an equation for U

′′

= −

U −

′

+ U

′

. (2.5.19)

This can be solve numerically to give the behavior at intermediate times.

In summary, at early times, the dynamics of the solution is dominated by the smooth-

ing out of the discontinuity of the initial data. At large times, the dynamics is dominated

by the convective behavior induced by the diﬀerent asymptotic behavior of the initial data

for large |x|. The self-similar proﬁle at the intermediate times serves to connect these

two types of behavior.

2.6 Renormalization group analysis

Renormalization group method is a very powerful tool for analyzing the coarse-grained

behavior of complex systems. Its main idea is to deﬁne a ﬂow in the space of models

or model parameters induced by a coarse-graining operator, in which the scale plays the

role of time, and to analyze the large scale behavior of the original model with the help

of this ﬂow. The main components of the renormalization group analysis are:

74 CHAPTER 2. ANALYTICAL METHODS

1. The renormalization transformation, i.e. a coarse-graining operator that acts on

the state variables.

2. The induced transformation on the mod el, or the model parameters. This deﬁnes

the renormalization group ﬂow.

3. The analysis of the ﬁxed points for this transformation, as well as the behavior near

the ﬁxed points.

It is rarely the case that one can carry out step 2 described above exactly and ex-

plicitly. Very often we h ave to use drastic approximations, as we will see in the example

discussed below. The hope is that these approximations preserve the universality class

of the model that we started with. Unfortunately, there is no a prior guarantee that this

is indeed the case.

We will illustrate the main ideas of the renormalization group analysis using a classical

model in statistical physics, the Ising model.

2.6.1 The Ising model and critical exponents

Consider th e 2D Ising model on a triangular lattice L (see for example the classical

textbook on statistical physics by Reichl [?]). Each site is assigned a spin variable s

which takes two possible values ±1. The state of the system is speciﬁed by the spin

conﬁguration over the lattice. For each conﬁguration {s

}, we associate a Hamiltonian:

H{s

} = −J

hi,ji

, (2.6.1)

where hi, ji means that i and j are nearest neighbors, J > 0 is the coupling constant.

We will denote K = J/(k

T ) which is the non-dimensionalized coupling strength. The

probability of a conﬁguration is given by:

P {s

} =

−

H{s

}/k

(2.6.2)

where the partition function Z is deﬁned to be

Z = Z(N, K) =

}

−H/k

(2.6.3)

2.6. RENORMALIZATION GROUP ANALYSIS 75

The sum is carried over all possible conﬁgurations. To simplify notations, we let H{s

} =

hi,ji

P {s

} = e

H{s

}

H{s

}

Statistical averages h·i are deﬁned with respect to this probability density. The free

energy is given by the large volume limit

ζ(K) = −k

T lim

N→∞

ln Z(N, K). (2.6.4)

This is a standard model for ferromagnetic materials. The expectation of a single sp in

hsi measures the macroscopic magnetization of the system.

Strictly speaking we should consider ﬁnite lattices and take the thermodynamic limit

as the lattice goes to Z

. It is well-known that this model has a phase transition in the

thermodynamic limit: There exists a critical temperature T

∗

such that if T ≥ T

∗

, the

thermodynamic limit is unique, i.e. it does not depend on how we take the limit and

what boundary condition we use for th e ﬁnite lattices. In addition, we have hsi = 0 in

the thermodynamic limit. However, if T < T

∗

, the limit is no longer unique and does

depend on the boundary condition used for the ﬁnite lattices. In particular, hsi may

not vanish in the thermodynamic limit. Intuitively, from (2.6.1), the energy minimizing

conﬁgurations are conﬁgurations for which all the spins are aligned, i.e. s

= 1 for all j

or −1 for all j ∈ L. Therefore at low temperature, the spins prefer to be aligned giving

rise a non-zero average spin. At high temperature, the spins prefer to be random for

entropic considerations, giving rise to a zero average. However, the fact that there is a

sharp transition at some ﬁnite temperature is a rather non-trivial fact [?].

It is also of interest to consider the correlations between spins at diﬀerent sites. There

are strong evidences that when T 6= T

∗

i − hs

ihs

i ∼ e

−r/ξ

r = |i − j|

Here ξ is called the correlation length and it depends on T . It is an important intrinsic

length scale for the system. The critical point T

∗

is characterized by the divergence of ξ:

ξ → ∞ (T → T

∗

)

In fact, from various considerations, one expects that

ξ ∼ (T − T

∗

)

−ν

76 CHAPTER 2. ANALYTICAL METHODS

where ν > 0 is called the critical exponent for the correlation length.

Heuristically, correlated spins tend to form clusters in which all spins have the same

sign, and ξ is of the order of the largest diameter of th e ﬁnite volume clusters. This

is illustrated in Figure 2.6.1. What is most interesting is the situation at the critical

temperature T

∗

which exhibits self-similarity: The picture looks essentially the same

at diﬀerent scales, or with diﬀerent resolutions. This motivates looking for statistically

self-similar behavior at T = T

∗

Given the background above, our goal is to compute T

∗

(or equivalently K

∗

) and

ν. The basic idea is to divide spins into blocks, and regard the blocks as coarse-grained

spins. This corresponds to looking at the problem from a coarser resolution. One way of

deﬁning the spin of a block is a to use the majority rule:

= sgn(

i∈I

)

where I refers to the index of the blocks. Here we assume that the number of spins within

every block is odd. Denote the size of the block by L. Then each block has roughly L

spins, where d is the space dimension. Assume for simplicity that the Hamiltonian for

the coarse-grained problem is given approximately by

H{s

} = −J

|I−J|=1

= −K

|I−J|=1

Then the coarse-graining operator for the spin conﬁguration induces a transformation in

the space of model parameters:

= R(K)

This is the renormalization transformation.

The information we are looking for can be obtained from this transformation. In

particular, we have:

1. The critical temperature is given by the ﬁxed point of this transformation: K

∗

R(K

∗

2. It is easy to see that

ξ(R(K)) = L

−1

ξ(K)

Therefore at the ﬁxed point of R, ξ = ∞.