Weinan E. Principles of Multiscale Modeling

Подождите немного. Документ загружается.

10.2. NUMERICAL ALGORITHMS 467

−8 −4 0 4 8

−6

−2

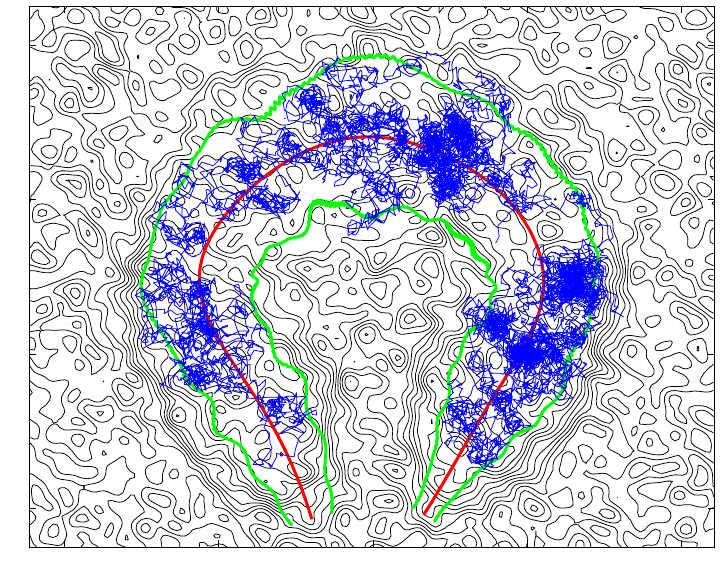
Figure 10.8: Results of the ﬁnite temperature string method applied to the rough poten-

tial shown in Figure 10.2. The green curves bound the transition tube. The red curve is

the centerline of the tube. The blue curve displays an example of a true reactive sample

path.

468 CHAPTER 10. RARE EVENTS

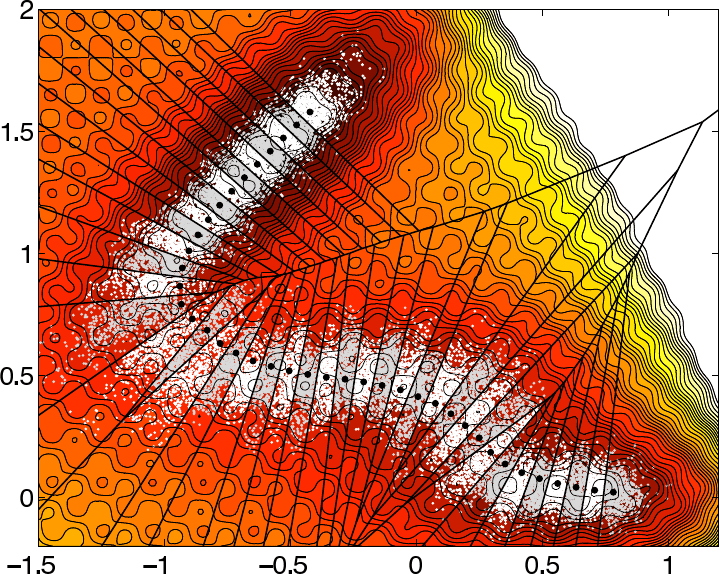
Figure 10.9: Results of the ﬁnite temperature string method based on Voronoi cells, for a

perturbed Mueller potential. Black dots show the string – the centerline of the transition

tube. The cloud of white dots show the samples in each Voronoi cell computed in the

sampling (or expectation) step. Court esy of Eric Vanden-Eijnden.

10.3. ACCELERATED DYNAMICS AND SAMPLING METHODS 469

region bounded by the green curves should be regarded as the transition tube. The blue

curve is a sample of the reactive trajectory. One can see that it lies mostly within the

transition tube.

An interesting recent development is to replace the hyperplanes in the ﬁnite tempera-

ture string method by Voronoi cells [56]. The Voronoi cells have the advantage that they

are more regular than the hyperplanes and therefore it is easier to perform constraint

sampling with the Voronoi cells. An illustration of the ﬁnite temperature string method

based on Voronoi cells is shown in Figure 10.2.3.

String method has been extended in a number of ways:

1. The growing string method: the ends of the string are allowed to grow to ﬁnd th e

metastable states [45].

2. The quadratic string method: Convergence can be accelerated using quasi-Newton

iteration algorithms [10], see also [48].

3. Combination with metadynamics in order to search more globally in conﬁguration

space [8].

4. String method using collective variables [40].

10.3 Accelerated dynamics and sampling methods

The techniques just presented require knowing beforehand the initial and ﬁnal states

of the transition. It is natural to ask whether we can accelerate the original dynamics

in such a way that it still captures the right kinetics of the system statistically. We will

discuss very brieﬂy one example of this kind: hyperdynamics [58]. We then discuss some

examples of improved sampling strategies for computing the free energy: metadynamics

[39] and temperature-accelerated molecular dynamics [41].

10.3.1 TST-based acceleration techniques

Hyperdynamics is an example of the approaches, developed mainly by A. F. Voter,

for accelerating molecular dynamics using the transition state theory. The objective

of these approaches is not to reproduce accurately the individual trajectories of the

470 CHAPTER 10. RARE EVENTS

molecular dynamics, but rather the Markov chain obtained as a result of the transition

between diﬀerent stable states (local minima of the potential energy V ). This requires

approximating accurately in a statistical sense the sequence of stable states obtained in

the MD simulation, as well as the transition rates (or transition time) between the stable

states. Other examples of the approaches developed by Voter under the same umbrella

include the temperature accelerated dynamics (TAD) and the parallel replica dynamics,

except that the parallel replica method does not rely on TST [60].

The basic idea of hyperdynamics is to add a biased potential ∆V to the original

potential V . The biased potential should satisfy the following requirements:

1. It raises the potential wells without modifying the behavior around the transition

states. By raising the potential wells, it speeds up the process of escaping the wells.

2. Transition state theory still applies. In other words, it does not introduce additional

correlated events near the transition states.

Constructing such a biased potential is in general a rather non-trivial task. Some exam-

ples are discussed in [60].

The addition of the biased potential does change the time it takes for the system to

move between diﬀerent stable states. To recover the the statistics for the original system,

we rescale time, using the formula obtained from TST [58, 59]. Denote by x(t

) the state

of the modiﬁed system at the n-th time step, and ∆t the MD time step for the modiﬁed

system. The time it takes for the original system to reach x(t

) is given by

∆t exp(∆V (x(t

))/k

T ) (10.3.1)

Clearly, (10.3.1) is only valid in a statistical sense.

10.3.2 Metadynamics

While the hyperdynamics works with the original variables and potential energy land-

scape of the system, other coarse-grained molecular dynamics methods have been de-

veloped for exploring the dynamics of some coarse-grained variables in the free energy

landscape, and acceleration methods have also been developed to speed up free energy

exploration. Here we will discuss some examples of this type.

10.3. ACCELERATED DYNAMICS AND SAMPLING METHODS 471

In Section 9.3, we showed that by simulating (9.3.19) at small γ and large µ, and

monitoring the evolution of x(t) one can indeed sample the free energy landscape in the

variables q(x). There is still one diﬃculty though: the dynamics described by (9.3.19)

still encounter barriers just as the original dynamics, and this reduces the eﬃciency of the

entire approach. To deal with this problem in metadynamics, Iannuzzi, Laio, and Par-

rinello suggested further modifying the dynamics to include in (9.3.19) (or rather (9.3.30)

in the original paper) an additional non-Markovian term which discourages the tr ajec-

tory from going back to regions that it has already visited. For instance, one may replace

(9.3.19) by











x = −∇V (x) + µ(X − q(x))∇q(x) +

2β

−1

X = −µ(X − q(x)) +

2β

−1

(X(t) − X(t

′

))e

−|X(t)−X(t

′

/∆q

′

(10.3.2)

where A and ∆q are adjustable parameters. Proceeding as before, it is easy to see that

the limiting equation for X as γ → 0 and µ → ∞ is

X = −∇

F + A

(X(t) − X(t

′

))e

−|X(t)−X(t

′

/∆q

′

2β

−1

(10.3.3)

The memory term added in (10.3.2) is a term that ﬁlls up the potential well that the

trajectory has already visited. In particular, if we let

U(X, t) =

A∆q

−|X−X(t

′

∆q

′

, (10.3.4)

then, as t → ∞, U(X, t) − U(X

′

, t) converges to an estimate of F (X

′

) − F (X) when

−1

≫ µ ≫ 1. The parameters A and ∆q control the accuracy for the resolution of the

free energy: as they are decreased, the resolution improves, but the convergence rate in

time deteriorates. Given some accuracy requirement, estimating the optimal choice of

the parameters γ, µ, A, and ∆q in a metadynamics calculation is a nontrivial question

which we will leave aside.

10.3.3 Temperature-accelerated molecular dynamics

Another interesting development is the temp erature-accelerated molecular dynamics

(TAMD), see for example [1, 41]. Here for simplicity, we will focus on the case with

472 CHAPTER 10. RARE EVENTS

overdamped dynamics. The extension to molecular dynamics is quite straightforward.

The main idea is a simple modiﬁcation of (9.3.19) in Section 9.3:







x = −∇V (x) + µ(X − q(x))∇q(x) +

2γβ

−1

X = −µ(X − q(x)) +

−1

(10.3.5)

Here

β = 1/(k

T ) and

T > T . As in Section 9.3, γ is small, so we are in the setting for

applying the averaging theorems for stochastic ODEs. In addition, µ is large, therefore

the eﬀective dynamics for the coarse-grained variables is a gradient ﬂow for the free

energy of the coarse-grained variables.

The advantage of using an elevated temperature for the coarse-grained variables is

that it accelerates the exploration of the free energy landscape for those variables. At the

same time, it does not aﬀect sampling the constrained dynamics for the original system,

i.e. it does not aﬀect the accuracy for the gradient of the free energy. See [1] for some

very interesting applications of TAMD.

TAMD should be contrasted to TAD, developed by Voter et al. [52]. The former

is a technique for accelerating the free energy calculation. The latter is a technique for

accelerating the dynamics (kinetics) of the original system based on TST.

10.4 Notes

Transition pathway s of coarse-grained systems

The ideas discussed in this chapter can be comnbined with those discussed in Chapter

6. For example, one can combine HMM and the string method, this gives rise to the

string method in collective variables (see for example [40]). The macroscale solver is the

string method in a set of coarse-grained variables. The data needed in the string method

can be estimated using constrained microscale solver, which is usually some constrained

molecular dynamics. In particular, the seamless method discussed in Chapter 6 can be

applied in this context, resulting in an algorithm in which the string moves simultaneously

with the microscopic conﬁgurations at each point along the string.

However, it should be emphasized that the notion of slow variables and reaction

coordinates are not necessarily the same, as we discussed in Section 10.1.5.

Non-gradient systems

10.4. NOTES 473

We have mostly focused our discussion on gradient systems. Noise-induced transition

in non-gradient systems can behave quite diﬀerently. For one thing, besides critical

points, non-gradient systems can have other invariant sets such as limit cycles or even

strange attractors. More importantly, the boundaries between the basins of attraction

of diﬀerent invariant sets can be very complex. The implication of these complications

to the mechanism of noise-induced transition in non-gradient systems has not been fully

investigated and we refer to [64] for a summary of the initial work done in this direction.

Quantum tunneling

Quantum tunneling is in many aspects very similar to the noise-induced transition

that we have discussed in this chapter. For example, one can formulate the transition

state theory in the quantum context. However, the mathematical issues associated with

quantum tunneling appear to be much more complex. We refer to [62] for a discussion

of some of these issues.

Application to kinetic Monte Carlo metho ds

The ideas discussed in this chapter can be used to design more accurate kinetic Monte

Carlo schemes. In standard kinetic Monte Carlo methods, t he possible states and the

transition rates are pre-determined, often empirically. This can be avoided by ﬁnding

the states and transition rates “on-the-ﬂy” from a more detailed model. See for example

[60].

474 CHAPTER 10. RARE EVENTS

Bibliography

[1] C. Abrams and E. Vanden-Eijnden, “Large-scale conformational sampling of proteins

using temperature-accelerated molecular dynamic,” Proc. Nat. Acad. Sci. USA, 107,

4961–4966, 2010.

[2] C. H. Bennett, “Exact defect calculations in model substances,” in Algorithms for

Chemical Computation, vol. 63, no. 46, pp. 73–113, A. S. Nowick and J. J. Burton,

eds., ACS Symposium Series, 1977.

[3] B. J. Berne, G. Ciccotti, and D. F. Coker eds., Classical and Quantum Dynamics in

Condensed Phase Simulations, World Scientiﬁc, 1998.

[4] A. Bovier, M. Eckhoﬀ, V. Gayrard, and M. Klein, “Metastability in reversible d iﬀu-

sion processes; Part I: Sharp estimates for capacities and exit times,” WIAS-preprint

vol. 767, 2003.

[5] A. Bovier, V. Gayrard, and M. Klein, “Metastability in reversible diﬀusion processes;

Part 2: Precise estimates for small eigenvalues,” WIAS-preprint, vol. 768, 2003.

[6] P. G. Bolhuis, C. Dellago and D. Chand ler, “Reaction coordinates of biomolecular

isomerization,” Proc. Natl. Acad. Sci. USA, vol. 97, pp. 5877–5882, 2000.

[7] P. G. Bolhuis, D. Chandler, C. Dellago, and P. L. Geissler, “Transition path sam-

pling: Throwing ropes over mountain passes in the dark,” Annual Review in Physical

Chemistry, vol. 53, pp. 291–318, 2002.

[8] D. Branduardi, F.L. Gervasio and M. Parrinello, “From A to B in free energy space,”

J. Chem. Phys., vol. 126, pp. 054103–054103-10, 2007.

475

476 BIBLIOGRAPHY

[9] R. C. Brower, D. A. Kessler, J. Koplik and H. Levine, “Geometric models of interface

dynamics,” Phys. Rev. A, vol. 29, pp. 1335–1342, 1984.

[10] S.K. Burger and W. Yang, “Quadratic string method for determining the minimum-

energy path based on multiobjective optimization,” J. Chem. Phys., vol. 124, pp.

054109–054109-13, 2006.

[11] E. A. Carter, G. Ciccotti, J. T. Hynes, and R. Kapral “Constrained reaction coor-

dinate dynamics for the simulation of rare events,” Chem. Phys. Lett. , vol. 156, pp.

472–477, 1989.

[12] D. Chandler, “Statistical mechanics of isomerization dynamics in liquids and the

transition state approximation,” J. Chem. Phys., vol. 68, pp. 2959–2970, 1978.

[13] R. Czerminski and R. Elber, “Self avoiding walk between two ﬁxed points as a tool

to calculate reaction paths in large molecular systems,” Int. J. Quantum Chem., vol.

24, pp. 167–186, 1990.

[14] C. Dellago, P.G. Bolhuis and D. Chandler, “Eﬃcient transition path sampling: Ap-

plication to Lennard-Jones cluster segments,” J. Chem. Phys., vol. 108, no. 22, pp.

9236–9245, 1998.

[15] C. Dellago, P. G. Bolhuis, P. L. Geissler, “Transition Path Sampling,” Advances in

Chemical Physics, vol. 123, pp. 1–84, 2002.

[16] W. E, W. Ren an d E. Vanden-Eijnden, “Energy landscape and thermally activated

switching of submicron-sized ferromagnetic elements,” J. Appl. Phys., vol. 93, no.

4, pp. 2275–2282, 2003.

[17] W. E, W. Ren and E. Vanden-Eijnden, “Finite temperature string method for the

study of rare events,” J. Phys. Chem. B , vol. 109, no. 14, pp. 6688–6693, 2005.

[18] W. E, W. Ren, E. Vanden-Eijnden, “String method for rare events: I. Smooth energy

landscapes”, unpublished.

[19] W. E, W. Ren, E. Vanden-Eijnden, “Simpliﬁed and improved string method for

computing the minimum energy paths in barrier-crossing events,” J. Chem. Phys.,

vol. 126, no. 16, pp. 164103–164103-8, 2007.