Weinan E. Principles of Multiscale Modeling
Подождите немного. Документ загружается.


4.2. MOLECULAR DYNAMICS 167
4.2.2 Equilibrium states and ensembles
Consider the solutions of Hamilton’s equation (4.2.2) with random initial condition.
Denote by ρ(z
1
, ··· , z
N
, t) the probability density of the particles at time t, where z
i
=
(y
i
, p
i
). It is easy to see that ρ satisfies Liouville’s equation:
∂
t
ρ +
N
X
i=1
∇
z
i
· (V
i
ρ) = 0 (4.2.16)
where V
i
= (∇
p
i
H, −∇
y
i
H), ∇
z
i
= (∇
y
i
, ∇
p
i
).
Given a system of interacting particles (atoms or molecules, etc), the first question
we should think about is their equilibirium states. These are stationary solutions of the
Liouville equation (4.2.16) that satisfy the condition of detailed balance:
∇
z
i
· (V
i
ρ) = 0
for each i = 1, ··· , N. It is obivous that probability densities of the form:
ρ
0
=
1
Z
N
f(H)
are equilibrium states. Here f is a nonnegative function, and Z
N
is the normalization
constant, also called the partition function:
Z
N
=
Z
R
6N
f(H)dz
1
···dz
N
Of particular importance are the following special cases:
1. The microcanonical ensemble: f (H) = δ(H − H
0
) for some constant H
0
.
2. The canonical ensemble: f(H) = e
−βH
where β = 1/k
B
T , k
B
is the Boltzmann
constant and T is the temperature.
The particular physical setting determines the particular equilibrium distribution that
is realized. In the context of molecular dynamics, the microcanonical ensemble is realized
through the NVE ensemble, in which the number of particles N, the volume V accessible
to th e particles and the total energy is fixed. This models a closed system with no
exchange of mass, momentum or energy with the environment. Similarly, the canonical
ensemble is realized through the NVT ensemble in which instead of the total energy, the

168 CHAPTER 4. THE HIERARCHY OF PHYSICAL MODELS
temperature of the system is fixed. Recall that temperature is defined as the average
kinetic energy per degree of freedom due to the fluctuating part of the particle velocity:
Assuming that the average velocity is zero:
1
N
*
N
X
j=1
v
j
+
= 0 (4.2.17)
where v
j
= p
j
/m
j
, then
1
N
*
N
X
j=1
1
2
m
j
|v
j
|
2
+
=
3
2
k
B
T (4.2.18)
The NVE ensemble is obtained when one solves directly the Hamilton’s equations
(4.2.2) in a box with, e.g. reflection bound ary condition. The value of the Hamiltonian
is conserved in this case. However, to obtain the NVT ensemble in which temperature
rather than the total energy of the system is fixed, we need to modify Hamilton’s equation.
There are several empirical ways of d oing this. The simplest idea is the isokinetic energy
trick (also called velocity rescaling). The idea is very straightforward for a discretized
form of (4.2.2): At each time step, one rescales the velocities v
j
simultaneously by a
factor such that, after rescaling,
1
N
N
X
j=1
1
2
m
j
|v
j
|
2
=
3
2
k
B
T (4.2.19)
holds, where T is the desired temperature. This does give the right average temperature,
but it does not capture the temperature fluctuations correctly. Indeed in this case the
quantity
˜
T =
1
3k
B
N
P
N
j=1
1
2
m
j
|v
j
|
2
does not fluctuate. This is not the case for the true
canonical ensemble. In fact, one can show that [18]
h(
˜
T − T )
2
i
hT i
2
=
2
3N
(4.2.20)
i.e. the fluctuation in
˜
T is of the order N
−
1
2
, which only vanishes in the limit when
N → ∞.
Other popular ways of generating the NVT ensemble include:
1. The Anderson thermostat: At random times, a random particle is picked and its
velocity is changed to a random vector from the Maxwell-Boltzmann distribution:
ρ(v
1
, v
2
, ··· , v
N
) ∼ e
−β
P
j
1
2
m
j
|v
j
|
2
(4.2.21)

4.2. MOLECULAR DYNAMICS 169
2. Replacing Hamilton’s equation by the Langevin equation
m
j
d
2
y
j
dt
2
= −
∂V
∂y
j
− γ
j
dy
j
dt
+ σ
j
˙
w
j
, j = 1, ··· , N, (4.2.22)
where γ
j
is the friction coefficient for the j-th particle, σ
j
is the noise amplitude:
σ
j
=
p
2k
B
T γ
j
(4.2.23)
{
˙
w
1
,
˙
w
2
, ··· ,
˙
w
N
} are independent white noises.
3. The N´ose-Hoover thermostat. This is a way of producing the canonical ensemble by
adding an additional degree of freedom to the system. The effect of this additional
degree of freedom is to supply energy or take energy away from the system according
to the instantaneous thermal energy of the system [18].
In general, these thermostats produce correctly the equilibrium properties of the system.
But it is not clear that dynamic quantities are produced correctly [18].
4.2.3 The elastic continuum limit – the Cauchy-Born rule
From the viewpoint of multiscale, multi-physics modeling, it is natural to ask how
the atomistic models are related to the continuum models discussed in the last section.
We will address this question from three different but related angles. In this subsection,
we will discuss the elastic continuum limit at zero temperature. In the next subsection,
we will discuss the relation between the continuum models and the atomistic models at
the level of the conservation laws. Finally, we will discuss how to compute macroscopic
transport coefficients using atomistic models.
At zero temperature T = 0, the canonical ensemble becomes a degenerate distribution
that is concentrated at the minimizers of the total potential energy:
min V (y
1
, . . . , y
N
). (4.2.24)
This special case is quite helpful for understanding many issues in solids.
It is well-known that at low temperature, solids are crystals whose atoms reside
on some lattices. Common lattice structures include body-centered cubic (BCC), face-
centered cubic (FCC), diamond lattice, hexagonal closed packed lattice (HCP), etc [32].
For example, at room temperature and atomspheric pressure, iron (Fe) exists in BCC
170 CHAPTER 4. THE HIERARCHY OF PHYSICAL MODELS
lattice, aluminum (Al) exists in FCC lattice, and silicon (Si) exists in diamond lattice.
Lattices are divided into two types: simple lattices and complex lattices.
Simple lattices. Simple lattices are also called Bravais lattices. Their lattice points
take the form:
L({e
i
}, o) = {x : x =
d
X
i=1
ν
i
e
i
+ o ν
i
, are integers}, (4.2.25)
where {e
i
}
d
i=1
is a set of basis vectors, d is the dimension, and o is a particular lattice
site. Note that the basis vectors are not unique.
For the examples listed above, BCC and FCC are simple lattices. For the FCC lattice,
one set of basis vectors are
e
1
=
a
2
(0, 1, 1), e
2
=
a
2
(1, 0, 1), e
3
=
a
2
(1, 1, 0).
For the BCC lattice, we may choose
e
1
=
a
2
(−1, 1, 1), e
2
=
a
2
(1, −1, 1), e
3
=
a
2
(1, 1, −1).
as the basis vectors. Here and in what follows, we use a to denote the lattice constant.
Another example of a simple lattice is the two-dimensional triangular lattice. Its basis
vectors can be chosen as:
e
1
= a(1, 0), e
2
= a(1/2,
√
3/2).
A
1
A
2
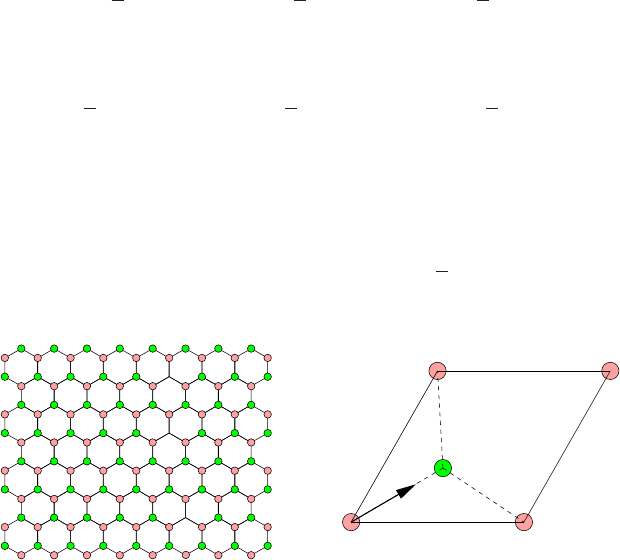
p
Figure 4.4: The graphene sheet as an example of a complex lattice. The right panel
illustrates a unit cell (courtesy of Pingbing Ming).
Complex lattices. Crystal lattices that are not simple lattices are called complex
lattices. It can be shown that every complex lattice is a union of simple lattices, each of

4.2. MOLECULAR DYNAMICS 171
which is a shift of any other: i.e., the lattice points can be expressed in the form:
L = L({e
i
}, o) ∪ L({e
i
}, o + p
1
) ∪ ··· L({e
i
}, o + p
k
)
Here p
1
, ··· , p
k
are the shift vectors. For example, the two dimensional hexagonal lattice
with lattice constant a can be regarded as the union of two triangular lattices with shift
vector p
1
= a(−1/2, −
√
3/6). The diamond lattice is made up of two interpenetrating
FCC lattices with shift vector p
1
= a/4(1, 1, 1). The HCP lattice is obtained by stacking
two simple hexagonal lattices with the shift vector p
1
= a(1/2,
√
3/6,
√
6/3). Some
solids consist of more than one species of atoms. Sodium-chloride (NaCl), for example,
has equal numbers of sodium and chloride ions placed at alternating sites of a simple
cubic lattice. This can be viewed as the union of two FCC lattices: one for the sodium
ions and one for the chloride ions.
The picture described above is only for single crystals and it neglects lattice defects
such as stacking faults, vacancies and dislocations. Most solids are polycrystals, i.e.
unions of single crystal grains with different orientations. Nevertheless, it is certainly
true that the basic building blocks of solids are crystal lattices.
Turning now to the physical models, we have discussed two approaches for analyzing
the deformation of solids: The continuum theory discussed in the last section and the
atomistic model. It is natural to ask how these two approaches are related to each
other. From the viewpoint of atomistic modeling, the relevant issue is the macroscopic
continuum limit of the atomistic models. From the viewpoint of the continuum theory,
the relevant issue is the microscopic foundation of the continuum models.
Recall that in continuum elasticity theory, the potential energy of an elastic solid is
given by
Z
Ω
W (∇u)dx (4.2.26)
where W is the stored energy density. In the last section, we discussed ways of obtaining
empirical expressions for W . We now ask: Can we derive W from an atomistic model?
This question was considered already by Cauchy, who derived atomistic expressions
for the elastic moduli. Cauchy’s work was extend ed by Born who also considered complex
lattices [4]. For crystalline solids, the Cauchy-Born rule establishes an expression for W
in terms of an atomistic model.
Let us consider first the case when the crystal is a simple lattice. In this case, given
a value of the deformation gradient A, consider a homogeneously deformed crystal with

172 CHAPTER 4. THE HIERARCHY OF PHYSICAL MODELS
deformation gradient A, i.e. the positions of the atoms in the deformed configuration are
given by y
j
= x
j
+ Ax
j
for all j. Knowing the positions of the atoms in the deformed
crystal, we can compute the energy density of the deformed crystal using the atomistic
model. This gives the value of W
CB
(A).
Figure 4.5: Illustration of the Cauchy-Born rule: W (A) is equal to the energy density of
a homogeneously deformed crystal obtained through the relation y = x + Ax (courtesy
of Pingbing Ming).
Next consider the case when the crystal is a complex lattice. In this case, to define
the configuration of a homogeneously deformed crystal, we not only have to specify
the deformation gradient A, we also need to specify the shift vectors p
1
, ··· , p
k
. Once
they are specified, we can compute the energy density (energy per unit cell) of the
homogeneously deformed crystal using the atomistic mo d el. We denote this value by
w(A, p
1
, ···p
k
). We then define:
W
CB
(A) = min
p
1
,···,p
k
w(A, p
1
, ··· , p
k
) (4.2.27)
To see where the Cauchy-Born rule comes from, consider the case of a simple lattice
with atoms interacting via a two-body potential:
V =
1
2
X
i6=j
V
0
(|y
i
− y
j
|) . (4.2.28)
Cauchy’s idea is to assume that the deformation of the atoms follows a smooth displace-
ment vector field u, i.e. [16]
y
j
= x
j
+ u(x
j
), (4.2.29)

4.2. MOLECULAR DYNAMICS 173
Then the total energy of the system is given by:
V =
1
2
X
i6=j
V
0
(|(x
j
+ u(x
j
)) − (x
i
+ u(x
i
))|), (4.2.30)
=
1
2
X
i6=j
V
0
(|x
j
− x
i
+ ∇u(x
i
)(x
j
− x
i
) + O(|x
j
− x
i
|
2
)|).
To leading order, we have
V ≈
1
2
X
i6=j
V
0
(|(I + ∇u(x
i
))(x
j
− x
i
)|) =
X
j
W (∇u(x
j
))V
a
≈
Z
W (∇u(x))dx (4.2.31)
where
W (A) =
1
2V
a
X
i6=0
V
0
(|(I + A)(x
i
)|)
and V
a
is the volume of the unit cell of the undeformed lattice. This is nothing but the
Cauchy-Born rule. In one dimension with x
k
= ka, (4.2.31) becomes
V =
1
2
X
i
X
k
V
0
1 +
du
dx
(x
i
)
ka
!
(4.2.32)
=
X
i
W
du
dx
(x
i
)
a (4.2.33)
≈
Z
W
du
dx
(x)
dx,
where
W (A) =
1
2a
X
k
V
0
((1 + A)ka) (4.2.34)
Calculations of this type have been carried out for very general cases in [3]. In
essence, such a calculation demonstrates that to leading order, the atomistic model can
be regarded as the Riemann sum of the nonlinear elasticity model where the stored energy
density is given by the Cauchy-Born rule. The validity of the Cauchy-Born rule at zero
temperature has been studied in great detail in [14] for the static case and in [15] for the
dynamic case. The essence of this analysis is to find out under what conditions, Cauchy’s
assumption that the atoms follow a smooth displacement field, is valid. These conditions
are shown be in the form of stability conditions for the phonons as well as the elastic
stiffness tensor. These conclusions seem to be sharp and quite general.

174 CHAPTER 4. THE HIERARCHY OF PHYSICAL MODELS
We will refer to the nonlinear elasticity model in which W = W
CB
as the Cauchy-Born
elasticity model.
So far we have only discussed the highly idealized situation when the temperature
is zero. The extension of the Cauchy-Born construction to finite temperature is still
somewhat of an open problem.
4.2.4 Non-equilibrium theory
From a statistical viewpoint, the basic object for the non-equilibrium theory is Liou-
ville’s equation. It contains all the statistical information we need. However, Liouville’s
equation is a very complicated object since it describes the dynamics of a function, the
probability density, of many variables. In the following, we will try to make a more
direct link with non-equilibrium continuum theory. We will do so by deriving, from the
equations of molecular dynamics, conservation laws similar to the ones in continuum the-
ory. We will restrict ourselves to the case when the inter-atomic potential is a two-body
potential:
V (y
1
, . . . , y
N
) =
1
2
X
i6=j
V
0
(|y
i
− y
j
|) (4.2.35)
Given the output from molecular dynamics, {(y
j
(t), v
j
(t)), j = 1, ··· , N}, we define
the empirical mass, momentum and energy densities as follows:
˜ρ(y, t) =
X
j
m
j
δ (y − y
j
(t)) , (4.2.36)
˜
m(y, t) =
X
j
m
j
v
j
(t)δ (y − y
j
(t)) , (4.2.37)
˜
E(y , t) =
X
j
1
2
m
j
|v
j
(t)|
2
δ (y − y
j
(t)) (4.2.38)
+
1
2
X
j
X
i6=j
V
0
(|y
j
(t) − y
i
(t)|)
!
δ (y − y
j
(t)) .
Here δ(·) is the Delta function. Let f
ij
= −∇
y
i
V
0
(|y
i
− y
j
|), we can define the analog of

4.2. MOLECULAR DYNAMICS 175
the stress tensor and energy flux:
˜σ =
X
i
m
i
v
i
(t) ⊗ v
i
(t)δ(y − y
i
(t)) (4.2.39)
+
1
2
X
i
X
j6=i
(y
i
(t) − y
j
(t)) ⊗ f
ij
(t)
Z
1
0
δ (y − (y
j
(t) + λ(y
i
(t) − y
j
(t)))) dλ,
˜
J =
X
i
v
i
(t)
1
2
m
i
v
i
(t)
2
+
1
2
X
j6=i
V
0
(|y
i
(t) − y
j
(t)|)
!
δ (y − y
i
(t)) (4.2.40)
+
1
4
X
j6=i
(v
j
(t) + v
i
(t)) · f
ij
(t) ⊗ (y
i
(t) − y
j
(t))
Z
1
0
δ (y − (y
j
(t) + λ(y
i
(t) − y
j
(t)))) dλ.
(4.2.39) is the well-known Irving-Kirkwood formula [22]. It is a straightforward but
tedious calculation to show that the following equations hold as a consequence of the
MD equation (4.2.1):
∂
t
˜ρ + ∇
y
·
˜
m = 0, (4.2.41)
∂
t
˜
m + ∇
y
· ˜σ = 0, (4.2.42)
∂
t
˜
E + ∇
y
·
˜
J = 0 (4.2.43)
These are the analog of the conservation laws in continuum theory.
Even though molecular dynamics naturally uses the Eulerian coordinates, these for-
mulas also have th eir analogs in Lagrangian coordinates. Denote x
0
j
= x
j
(0), which can
be thought of as being the Lagrangian coordinate of the j-th atom, and let [30]:
˜ρ(x, t) =
X
j
m
j
δ(x − x
0
j
), (4.2.44)
˜
m(x, t) =
X
j
m
j
v
j
(t)δ(x − x
0
j
), (4.2.45)
˜
E(x, t) =
X
j
1
2
m
j
|v
j
(t)|
2
δ(x − x
0
j
) +
1
2
X
j
X
i6=j
V
0
(|x
0
j
− x
0
i
|)
!
δ
x − x
0
j
. (4.2.46)
˜σ =
1
2
X
i
X
j6=i
(x
0
i
− x
0
j
) ⊗ f
ij
(t)
Z
1
0
δ
x −
x
0
j
+ λ(x
0
i
− x
0
j
)
dλ, (4.2.47)

176 CHAPTER 4. THE HIERARCHY OF PHYSICAL MODELS
˜
J =
1
4
X
j6=i
(v
j
(t) + v
i
(t)) · f
ij
(t) ⊗ (x
0
i
− x
0
j
)
Z
1
0
δ
x −
x
0
j
+ λ(x
0
i
− x
0
j
)
dλ (4.2.48)
we then have [30, 41]
∂
t
˜
m + ∇
x
· ˜σ = 0, (4.2.49)
∂
t
˜
E + ∇
x
·
˜
J = 0 (4.2.50)
In principle, one way of deriving the continuum models is to perform averaging on the
conservation laws we just discussed. In practice, we face the closure problem: In order
to calculate the averaged fluxes, we need to know many-particle probability density of
the atoms. We get a closed form model if this probability density can be expressed in
terms of the averaged conserved quantities (the mass density, averaged momentum and
energy densities). However, this is usually not the case. Therefore approximations have
to be made in order to solve the closure problem. This point will be made more clearly
in the next section.
4.2.5 Linear response theory and the Green-Kubo formula
The connection between the continuum and atomistic models established above is
quite general but it may not be explicit enough to offer significant physical insight. Here
we consider the linear response regime and establish a connection between the transport
coefficients, such as diffusion constants and viscosity coefficients, in terms of microscopic
quantities. We can always put a system into the linear resp onse regime by limiting the
size of the external driving force.
First, let us consider th e diffusion of a tagged particle in a fluid. We assume that its
probability distribution ρ satisfies the diffusion equation:
∂
t
ρ(x, t) = D∆ρ(x, t) (4.2.51)
Assume that the particle is at the origin initially. Since h|x(t)|
2
i =
R
|x|
2
ρ(x, t)dx, we
