Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

314
KdV
Equation
there exists one
and
only one sol'ution u(x,
t)
of
KdV equation
satisfying
the
initial condition, u(x,
0)
=
uo(x).
12.2
Symmetries
12.2.1
S~~e-t~~~
s~~~e~~~e~
It
is
easy to see that KdV equation is invariant under Gaiilei transformation
x'
=
x
+At,
{
t'=t.
Indeed, we have
au
au'
ax'
auiatt
aut
_.
-
--
where
u'
denote the composite function
Ut(X',
t')
=
ufx'
-
At',
t')
,
Therefore, KdV becomes
or
written in terms of the function
zi
=
u'
+
A,
In conclusion, KdV equation is invariant under the transformation
z+z-t-At,
u+u-A,
[
-+t,
(12.4)

Symmetries
315
12.2.2
Backlund
trans
formation
Internal symmetries,
as
usual, also play
a
relevant role in the analysis
of dy-
namical systems,
as
the following example well shows.
Example
36
Let
us
consider the Burger equation, given
by
Ut
=
22121,
+
Uxx.
It is easy to verifg that, under the map
(12.5)
Burgers’ equation becomes the heat equation
vt
=
v,,
. (12.6)
The map given by Eq.
(12.5)
is called the Hopf-Cole transformation, and can
be used as follows.
First, let
us
observe that from
Eq.
(12.5)
we can obtain
and then
v,,
=
uxv
+
UV,
=
uxv
+
u2v
=
(u,
+
2).
.
(12.7)
Second,
if
v
is a solution
of
Eq.
(12.6),
then also
v,
is a solution
of
the same
equation. The same is,
of
course, true for all higher derivatives.
Therefore, starting with a solution
v
of heat equation, we can construct, at
least, two solutions, namely
u
and
a,
of Burgers’ equation:
vx
-
vxx
u=-,
u=-
V
vx
’
so
that
-
vxx
uu=--.
V
By comparing Eqs.
(127)
and
(12.8),
we may write
2
u=-
-
u,+u
U
(12.8)

316
Kd
V
Equation
which
allows
us
to
obtain
a
new solution
f7om
a
given one, and constitutes
an
ezample of so-called Backlund transfo~ataon~.
It
expresses
the
invariance of
Burgers’ eq~atzon under translat~ons a~on~ the
5
axis.
Similarly, Miura observed that,
by
performing the transformation
1
u
=
vx
-
-112
6’
KdV tr~sforms in the so-called modified KdV equation (mKdV):
1
6
Vt
-
-v2vx
+
vxxx
=
0,
which
is
manifestly invariant under the interchange
so that,
if
v
is
a solution
of
mKdV, then
-v
is again a solution.
and
ii
of KdV:
As
a consequence, given a solution
v
of
mKdV, we obtain
two
solutions
u
u=v,-
1
12
6
6
-u2,
ii=vx+-v
F’rom previous relations,
we
may write
iz+u=2ux,
li--=zv
’
so
that
(2
-
u)x
=
-vvx
2
=
&G@
+
u).
3
The above equation allows us to obtain
a
new solution from
a
given
one,
and
consti~utes another example
of
Backfund
transformation.
By
performing a Hopf-Cole transformation
on
the modified KdV, we have
$22
+t
f
$xxx
-
3-qx
$
=
0.
(12.9)
Sy
mmetriea
317
Then,
by
composing Miura's and Hopf-Cole's transformation, we obtain
the
map
$xx
111'
u
=
-6-
for which the KdV equation becomes the more unpleasant
Eq.
(12.9).
KdV can be written in the following form:
However, the look
of
Eq.
(12.9)
can be deceiving. Indeed, we stated that
(u
=
-67,
qxx
$X%
$t
+
$xxx
-
3-$x
=
0,
1
3
which ca.n be written
as
follows:
1
.ttxx+jZ1$=O,
1
i(
111t
+
4axxx
+
uax
+
px)
111
=
0,
where the shorthand notation
has
been
introduced.
ance of KdV expressed by
Eq.
(12.4):
Moreover,
a
change for the better can be done by using the GaIiIei invari-
x+z+6Xt,
t+t,
In
fact,
it
is
easy
to
see that this change
allows
us
to
write the above system
in the following form:
318
KdV
Equation
so
that, by introducing the operators
1
L
=
a,,
+
-21,
6
1
2
B
=
-4a,,,
-
.a,
-
-21%
)
KdV equation assumes the following remarkable form:
L11,==W>
?j=B+.
(12.10)
12.3
~~nservation
Laws
From
KdV equation
we
have
+m
+m
utdx
=
-
lw
(uuZ
-t-
u,,,)dx
=
-
so
that
d
J'"
udx
=
0.
dt
--oo
Thus, the functional
4-m
s_,
lLdX,
K~[u]
z
is
a
first integral
of
KdV.
applying the same procedure. After an integration by parts, we obtain
Another first integral is easily obtained by rnuItiplying KdV by
u
and by
CW
uutdx
=
-
(~~21%
+
uu,xx)dx
lw
fw
=
-
s_,
(u2uz
-
uxaxx)dx
Conservation
Laws
319
Thus, we may write
a
second conservation law
A
third conservation law is given by
K3fUI
f
-
1’”
(g
-
u:>
ds.
2
--m
Let
us
observe that the gradients
Gi(u)
=
GKi/Su
of previous functionals
are given
by
and that the following Lenard’s recursive formula holds131
(12.11)
a
-G,+I
=
Eke,,
8X
n
=
1,2,3,
where the operator
Bk,
expressed
by
is
~tisy~etric with respect to the
L2
scalar product.
operator
Moreover,
Eq.
(12.10)
suggests that the eigenvahes
of
the Schrodinger
corresponding to
a
“potential” given by a solution
u(s,t)
of
KdV,
do not
depend on time,
so
that these eigenvalues, considered
as
functionals
X(uj
of
the
potential
u,
are first integrals
of
KdV.
The direct proof
was
given by Gardner
et
u1,1011155
algebraically solving
the eigenvalue equation
1
$x,
f
p{x,
t)$
=
W)$

320
with respect to
u:
Kd
V
Equation
$ZX
$
u
=:
6X(t)
-
6-,
and then by replacing the above expression in KdV equation.
Thus,
At$'
-
($Qz
-
$zQ)x
=
0
9
(12.13)
where
If
II,
vanishes when
1x1
00,
Eq.
(12.13),
integrated with respect to
2,
gives
$2dx
=
0,
and then
X
=
constant.
12.3.1
&us
~p~~en~u~~~n
By taking the time derivative
of
the first equation
of
the system
(lZ.lO),
we
can write
t$
+
Ld
=
Ad,
and by using the second equation, namely
4
=
B$,
we have
L$
+
LB$
=
AB$
=
BL$,
so
that we obtain
L$
=
P,
Lilt
9
where the bracket
f.,
a]
denotes the usual comm~tator between operators.
The reader can easily check that KdV
is
just represented by the equation
L=[B,Lj.
(12.14)
The above equation
is
called
Lax
representation
of
KdV and can
be
intro-
duced for many other systems with an infinite-dimensional phase manifold
M.

Conservation
Laws
321
The introduction
of
Lax
representati~n’~~ has been a relevant progress in the
study
of
integrable systems and has played an important role in formulating
the inverse scattering method, ~iversally recognized
aa
one of the standard
integration techniques
.lol
*8
Shortly, it consists in the fo~Iowing.
Let
M
be some space of functions, chosen
so
that, for each
t,
the solution
~(t)
of
a
generic evolution equation
G(t}
=
A(%),
(12.15)
lies in
M.
Suppose that, with each function
ZL
in
M,
we
can associate
a
self-adjoint
operator
L(u
-+
L)
over some Hilbert space, in such
a
way
that, if
u
changes
with
t
according to
Eq.
(12.15)j
the operators
L(t),
which also change with
t,
remain unitary equivalent to themselves:
L(t)
=
u(t)L(o)u(t)-l
j
(
12.16)
with
U(t)
denoting
a
1-parameter family of unitary operators.
By
taking the “time derivative” of the above equation,
we
have
L=
[B,L],
(1
2.17)
where the skew-symmetric operator
B
=
uu-1
is the generator of the
family
U(t).
The above equation,
is
calIed Lax repre-
sentation of the dynamics given
by
Eq.
(12.15}j and the pair
(B,
L)
is called
a
A
consequence of Eq,
(12.16)
is
that the eigendues of the operators
L(t)
Indeed, let us consider the eigenvalue equation for the Lax operator at time
Lax
pair.
do
not depend on
t.
t
=
0:
(12.18)

322
Kd
V
Equation
or equivalently
where
Therefore,
j,
=
0,
$(t)
=
B$(t)
*
For
this reason,
Eq.
(12.16)
is
called an
isospectral
flow.
(12.19)
12.3.2
The inverse scattering method
Let
us
show how, by taking advantage
of
the
Gel,fand-Lev~ta~-~~~henko
formu1a102~143~118~14
Lax
representation allows
us
to
solve the given evolution
equation (12.15).
Let
ua(z)
be
the initial condition; i.e.
uo
=
u(x,O), and
LO
be the associated
Lax operator. Suppose that we are able to solve the corresponding eigenvalue
problem
Lo$@
=
k%p,
that is to
find
0
the
free states
(continuous spectrum);
i.e.
the
states,
corresponding
to
k2
>
0,
represented by waves
$O(x,
k),
whose asymptotic behavior
is
given
by
$'(x,k)
=+Ym
Co(k)exp[-ikx]
,
$O(x,
k)
=+Tm
CO(k)
exp[-ikz]
+
Cy(lC)exp[ilCx),
where
are called the
tr~~s~issi~~ coe~cie~t
and the
re~ect~o~ coe~c~e~t,
respectively
;
Conservation
Laws 323
0
the bound states (point spectrum); i.e. the states, corresponding
to
Ic2
<
0,
represented by eigenfunctions
$:(q
kn),
with
ki
=
--xi
(or
better,
k,
=
ix,,
X,
>
0),
whose asymptotic behavior is given by
$:(.,
ixn)
z+L
exp[~nz]
7
$:(.,
ixn)
z~co
c:(xn) exp[-~n~]
i
where the coefficients
c:
(xn)
are called the normalization constants.
The set
so
=
{xn,
&Xn),
RO(W
Vk
E
is
called the set
of
scattering data.
Of course, before solving the evolution equation, only the form
of
the oper-
ator
B
generating the isospectral flow is known, but not its explicit dependence
on
(5,
t),
However,
as
it will be explicitly shown in the case of KdV, the simple
knowledge of the asymptotic behavior of the operator
B
is enough to determine
the scattering data, namely
s
=
{xn,
cn(xn,t),
R(k,t)
vlc
E
8)
of the
Lax
operator
L,
associated with the solution
u(z,t)
of
Eq.
(12.15),
corresponding to the given initial value
uo.
The knowledge
of
scattering data of the operator
L
allows
us,
by means
of the
Gel'fand-Levitan-Marchenko
formula (GLM), to explicitly write
L
and
then
u(z,
t).
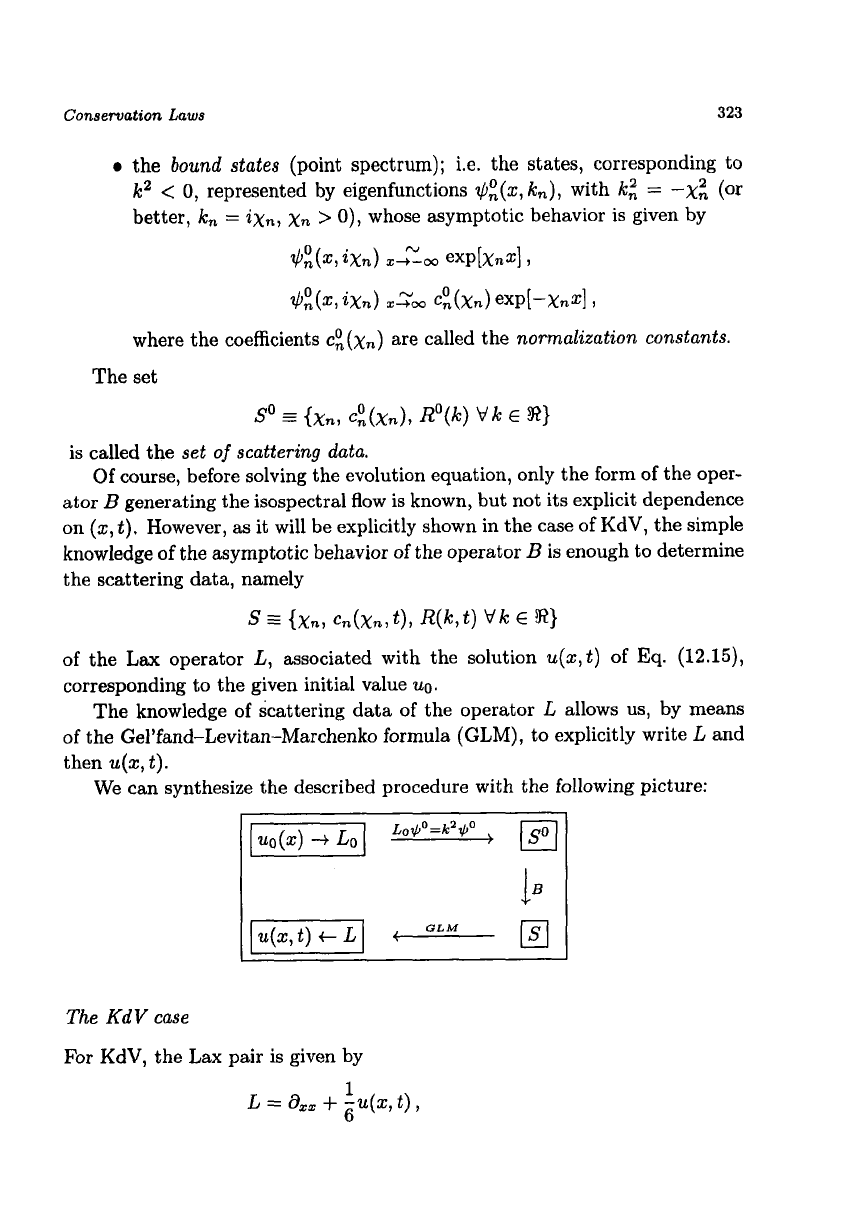
We can synthesize the described procedure with the following picture:
I'
The
Kd
V
case
For KdV, the Lax pair is given by
1
L
=
a,,
+
-u(z,
6
t)
,
