Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

304
Classical Electrodynamics
We, thus, find that the solutions
of
Maxwell's equations are closely related
with the study
of
the wave equation.
11.3.4
Plane
waves
Let
us
look
for
a
solution
F
of
Maxwell's equations in the empty-space
dF
=
0,
d*F=O,
of travelling wave type; that is, such that the fields
@
and
l?
are functions of
(
=
x
-
ct:
E
=
E(x
-
ct)
,
B
=
B(x
-
Ct)
.
We have
dF
=
dxQ AdE
-
dB,
where
E
=
Exdx
+
E,dy
+
E,dz
,
B
=
Bxdy
A
dz
+
B,dz
A
dx
+
B,dx
A
dy
Thus,
dE
=
aEx
-d[
A
dx
+
sd( Ady
+
Fdt dE, Adz
at
at
aE,
aEz
at
at
at
=
-c-dt
A
dx
+
sd(x
-
ct)
A
dy
+
-d(z
-
ct)
A
dz
,
and
aBx
at
at
at
dB
=
-d(
A
dy
A
dz
+
sdt;
A
dz
A
dx
+
-d(
A
dx
A
dy
=
-d(x
-
ct)
A
dy
A
dz
-
csdt
A
dz
A
dx
-
c-dt
A
dx
A
dy
.
at
at
at
Therefore,
dx' AdE
=
c-dt Adz Ady
+
c-dt Adz Adz,
at
at
Geometrical Identification
of
Electromagnetic Field
in
Space- Time
305
and
dF
=
dx’ AdE
-
dB
dE,
aEvdt
A
dx
A
dy
+
c-dt
A
dx
A
dz
=
Cx-
a<
aBx
-
-d(x
-
ct)
A
dy
A
dz
-
A
dz
A
dx
-
c-dt
A
dx
A
dy
a<
=
c
(-
aE,
-
->
aB, dt Adz Ady+c
($
-k
3)
dt Ada: Adz
a<
In this way,
dF
=
0
=+
B,
=
0,
B,
=
E,,
B,
=
-E,.
Similarly,
d* F
=0
+
E,
=O,
B,
=
E,,
B,
=
-Ez.
It follows that a plane electromagnetic wave
has
transverse electric and mag-
netic
fields
that are determined
by
two
independent functions, corresponding
to the two independent polarization states.
Further
Readings
0
R.
Aldovrandi and
J.
G. Pereira,
An Introduction
to
Geometrical
0
N.
V.
Balasubramanian,
J.
W. Linn and
D.
P.
Sen Gupta,
Differential
forms
on
0
W.
L.
Burke,
Applied Differential Geometry
(Cambridge University Press, 1985).
0
H.
Flanders,
Differential
Forms
(Academic Press, New York, 1963).
0
C.
Godbillon,
Gbombtrie Differentielle et M2canique Analytique
(Hermann, Paris,
1969).
0
V.
Guillemin and
S.
Sternberg,
SympEectic Techniques in Physics
(Cambridge
University Press, 1984).
0
B.
Kostant, “Orbits, symplectic structures
and
representation theory,”
Pmc.
U.S.-Japan Seminar
in
Differential Geometry, Kyoto,
1965
(Nippon Hyoronisha
Tokyo, Japan, 1965), p.
71.
Physics
(World Scientific, 1995).
Electromagnetic Networks
(Butterworths, London, 1970).

306
Classical
Electrodynamics
R.
S.
Ingarden and
A.
Jamiolkowski,
Classical Electrodynamics
(Elsevier, Ams-
terdam, 1985).
P.
Libermann and C.-M. Marle,
Syrnplectic Geometry and Analytical Mechanics
(Dordrecht, Reidel, 1987).
A.
Lichnerovich, “New geometrical dynamics,” in
Lecture Notes
in
Mathematics
(Springer, 1975), p. 570.
G.
M.
Marle,
Symplectic Manifolds, Dynarnicul Groups and Hamiltonian Mechan-
ics
(Reidel, Boston, 1976).
J.
Moser, “Various Aspects
of
Integrable Hamiltonian Systems,’’ in
Progr. Math.,
Vol.
8 (Birkhauser, Boston, 1980).
G.
Pichon,
Grovrpes de Lie:
re‘ppresentation line‘aires et applications
(Hermann
Paris-Collection MBthodes).
0
W.
Thirring,
A
course in Mathematical Physics
(Springer Verlag, 1978).
C.
Von Westenholtz,
Diflerential
forms
in
Mathematical Physics
(North-Holland,
1981).

Part
IV
Integrable
Field
Theories

The
last
few decades have shown the exciting prospects
of
tackling non-
linear field theories (in two dimensions) nonperturbatively by exploiting their
complete integrability properties.92193189J16
Let
us
recall that the concept
of
completely integrable Hamiltonian systems
with finitely many degrees
of
freedom goes back to the last century.1359164
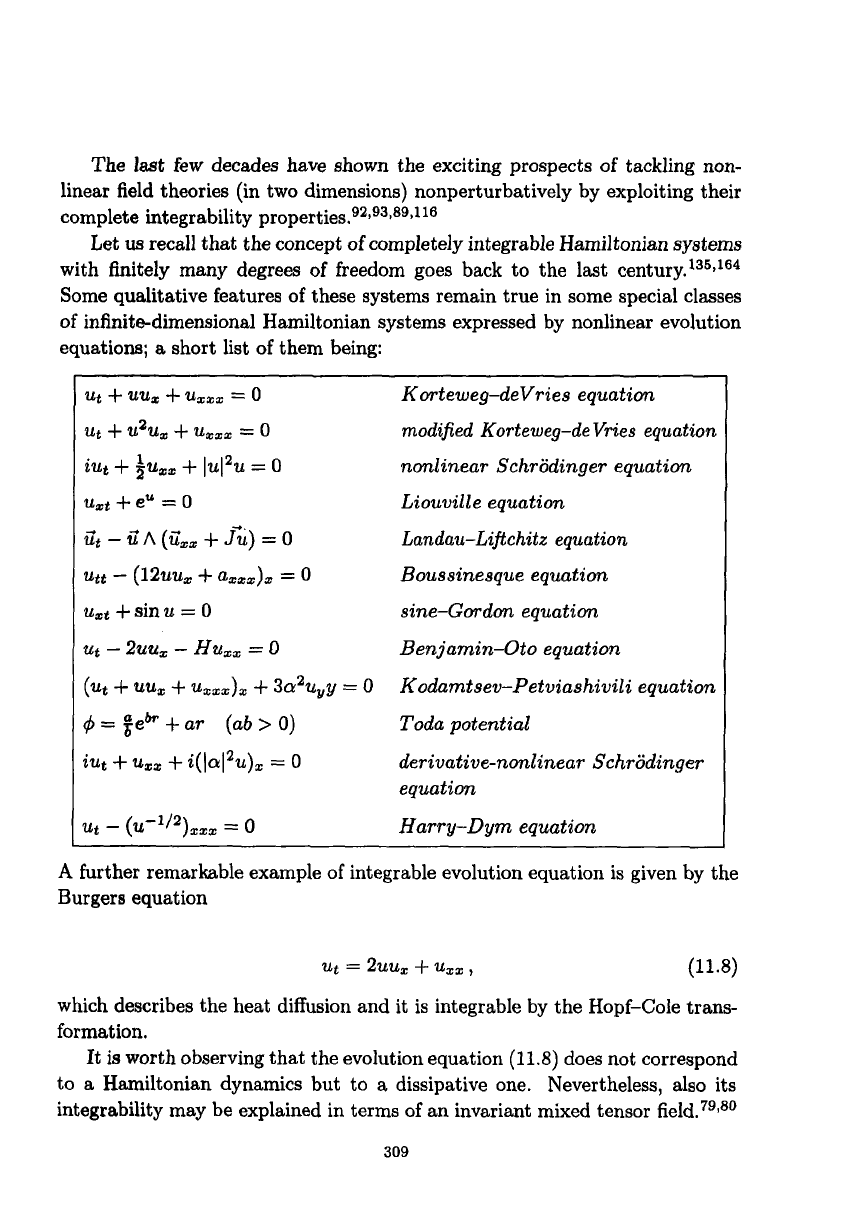
Some qualitative features of these systems remain true in some special classes
of infinite-dimensional Hamiltonian systems expressed by nonlinear evolution
equations;
a
short list of them being:
,
ut
+
~Uz
+
Uzxx
=
0
Ut
+
21%
+
uxxr
=
0
iut
+
guzx
+
Iu12u
=
0
uxt
+
e"
=
0
Gt
-
dA
(Gxz
+
JF)
=
0
utt
-
(1211~~
+
azxz)z
=
0
uxt
+
sin
u
=
0
ut
-
2uuz
-
Huxx
=
0
(Ut
+
UU,
+
uxxz),
+
3a2uvy
=
0
4
=
feb
+
ar (ab
>
0)
iut
+
U,x
+
i((al2u),
=
0
Ut
-
(U-1/2)xxx
=
0
Korteweg-deVries equation
modified Korteweg-de Vries equation
nonlinear Schrodinger equation
Liouville equation
Landau-Liftchitz equation
Boussinesque equation
sine-Gordon equation
Benjamin-Oto equation
Kodamtsev-Petviashivili equation
Toda potential
derivative-nonlinear Schrodinger
equation
Harry-Dym equation
A
further remarkable example of integrable evolution equation is given by the
Burgers equation
ut
=
221212
+
uxx
,
(11.8)
which describes the heat diffusion and it is integrable by the Hopf-Cole trans-
formation.
It
is
worth observing that the evolution equation (11.8) does not correspond
to a Hamiltonian dynamics but to
a
dissipative one. Nevertheless, also its
integrability may be explained in terms
of
an invariant mixed tensor field.
79380
309

310
Classical
Electrodynamics
A
relevant progress in the study of these systems with an infinite-dirnen-
sional phase manifold
hi,
was the introduction
of
the
Lax
~e~rese~tuti~n,l3I
which played an important roIe in formulating the
inverse s~t~e~~g ~et~~~)
universally recognized as one of the most remarkable result of theoretical
physics in the last decades, and
of
the
“AKNS
s~~e~e.”~’
This method al-
lows the integration
of
nonlinear dynamics, both with
a
finitely or infinitely
many degrees of freedom, for which
a
Lax representation can be given,l*lis
this being both of physical and mathematica1 re1evan~e.I~~
On the other hand, the natural arena, for the analysis of the integrab~lity
of dynamical systems,
is
represented by the phase space that
is
endowed with
a
natura~ symplectic structure. In terms of this structure, the scattering data
are interpretable
as
action-angle type variables.
We shall
see
how the integrability of nonlinear field theories can be naturally
explained in terms of mixed tensor fields, rather than symplectic structures,
and how such tensors are linked to the Lax operators. The approach leads to
a theorem of integrability that does not assume a finite number
of
degrees of
freedom and, for
a
dynamical system with finitely many degrees of freedom,
is
equivalent to the classical Liouville theorem.
As
a
working example we will use the Korteweg-de Vries equation
(KdV),
which
is
the most-known completely integrable nonlinear field theory.
Chapter
12
KdV
Equation
The equation
ut
+
uu,
+
u,,,
=
0,
(12.1)
where
u
:
(z,t)
E
R2
-+
IR
is a numerical function depending on the variables
2,
t,
and the indices denote partial derivatives, was derived by
D.
J.
Korteweg
and
G.
de Vries120 in
1985
in order to describe shallow water waves moving in
a
channel without any change of shape,’’O and it is known
as
KdV
equation,
or simply,
Kd
V.
12.1
An
Existence and Uniqueness
Theorem
A
travelling wave type solution
of
KdV,
of the form
u(2,
t)
=
s(x
-
Ct)
,
can be easily found in the hypothesis that
s
vanishes at the infinity together
with its space derivatives.
Indeed, setting
(
=
x
-
ct,
KdV
equation becomes
-cst
+
sst
+
sttt
=
0,
311
KdV
Equation
312
where the apex denotes the derivative with respect to
(.
Thus, integrating and
using the boundary condition, we obtain
1
2
-cs
+
-s2
+
s/I
=
0.
A
first integral
of
the above equation can be found multiplying by
s',
so
that
1
2
-CSd
+
-S2d
+
s"9'
=
0,
and integrating once again
-a2
+
As3
+
d2
=
0,
3
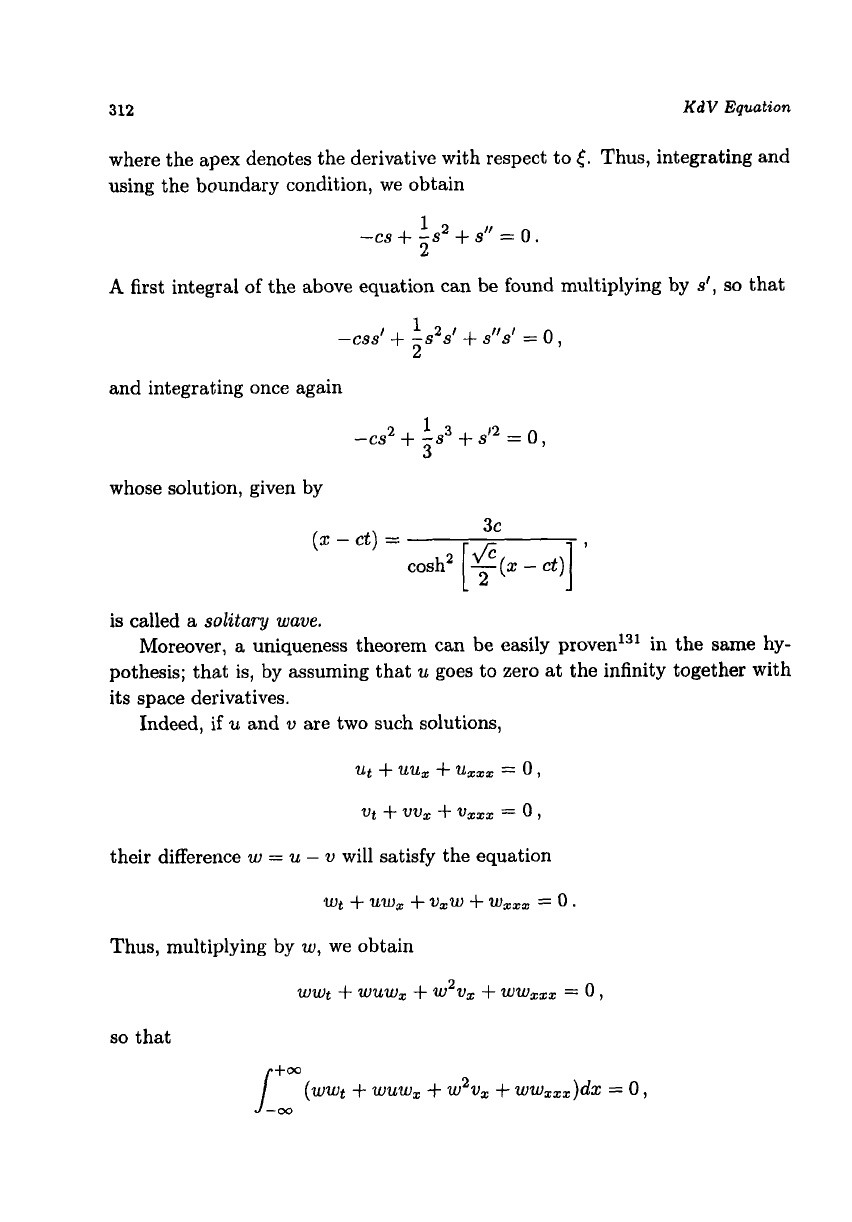
whose solution, given by
is called
a
so2itay
wave.
Moreover,
a
uniqueness theorem can be easily proven'31 in the same hy-
pothesis; that is, by assuming that
u
goes to zero at the infinity together with
its space derivatives.
Indeed, if
u
and
v
are two such solutions,
ut+UUx+uxxx=0,
'Ut
+
'U'UX
+
vxxx
=
0,
their difference
w
=
u
-
v
will satisfy the equation
Wt
+
UW,
+
vzw
+
Wxxx
=
0
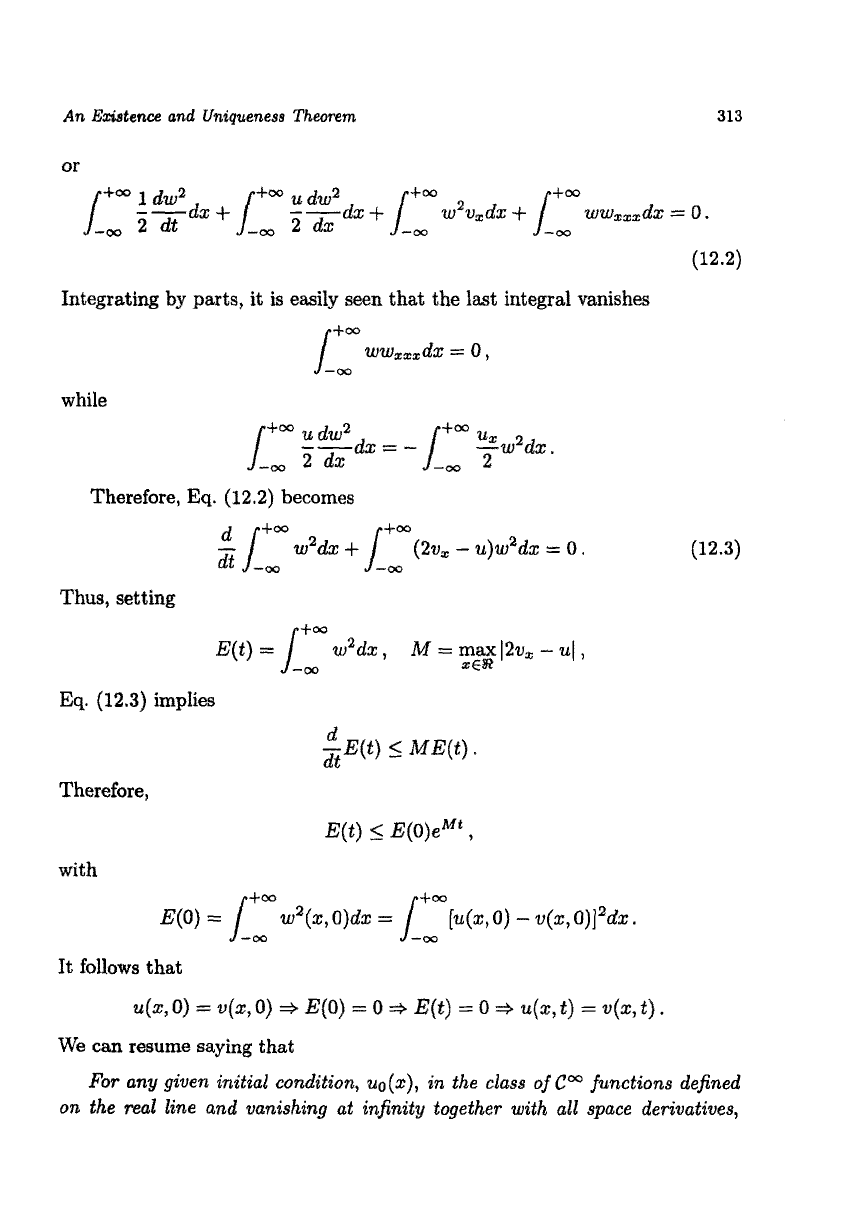
Thus, multiplying by
w,
we obtain
2
wwt
+
WUW,
4-
w
21%
+
wwxxx
=
0,
so
that
+W
(wwt
+
WUW,
+
w2vX
+
wwxxx)dx
=
0,
An
Existence and Uniqueness Theorem
313
or
(12.2)
Integrating
by
parts, it
is
easily seen that the
fast
integral vanishes
wwxxxdx
=
0,
while
Therefore,
Eq.
(12.2) becomes
(12.3)
Thus, setting
r+m
Eq.
(12.3)
implies
d
dt
-E(t)
5
~E(t).
E(t)
5
E(O)Pt,
Therefore,
with
+W
+oo
E(0)
=
Lrn
w2(x, 0)dx
11:
[u(x,
0)
-
v(x, 0)l2dx.
1-
It
follows that
ufx,
0)
=
v(x,
0)
*
E(0)
=
0
3
E(t)
=
0
*
u(x,
t)
=
v(x,
t)
.
We
can
resume
saying
that
For
any
given initial condition,
UO(X),
an the
class
of
C"
functions
defined
on
the
real
line and vanishing at infinity together with
all
space
derivatives,
